Relativistic Corrections to the Maxwellian Distribution for Astrophysical and Fusion Plasmas ()
1. Introduction
The Maxwell distribution is fundamental for statistical behavior of identical particles of mass m, in a environment of temperature
, it starts with the
Boltzmann theoretical thermodynamic law, which is
[1] . Setting
, one obtains the statistical distribution of identical particles of mass
m. In order to obtain a true probability law, one integrates the Boltzmann law on a velocity range v from
, this leads to the correct classical Maxwellian distribution [2] .
The theory of relativity developed by Albert Einstein (1905) imposes that the maximal physical velocity is
, with c is the velocity of light in vacuo.
Taking into account the modification of the velocity limit, for high energy particles, the Maxwell distribution is changed into the Maxwell-Jüttner distribution, (1911) [3] . This paper shows how is the change from standard Maxwellian, with numerical evidences.
Reminding the Maxwell distribution: that is:
(1)
[4] This function
is a normalized probability law:
(2)
[4] At the origin of this article is the interest of the author (since a long time) on how to modify the Maxwellian distribution for particles of mass m, in surrounding environment of temperature T, considering the finite value of the velocity of light c in vacuo, then replacing
into
in the Maxwellian distribution is an interesting physical problem, it has important consequences for high temperatures up from
existing in laboratory fusion plasmas and astrophysical plasmas observed in X rays, notably in Supernovae explosions. The author came across the work of L. Zaninetti (2020) [4] , in his paper changes of variables and the introduction of the Lorentz factor:
are perfectly shown.
Dealing with relativity, the kinetic energy has to be changed according to the famous Albert Einstein formula:
, where
is the rest mass of the particle and
, and the Lorentz factor follows:
(3)
The relativistic energy follows from:
(4)
(5)
(6)
(7)
This is the formula (3) [4] and in [5] formula (10). To install relativity in the Maxwell distribution, I shall follow the Zaninetti way in [4] , his function is:
(8)
It is a not invariant in relativistic transformations. Following: [4] it is necessary to change the variable v in this way:
and
.
Thus the distribution becomes: the relativistic MB in the variable
(9)
The normalization of the distribution is performed:
(10)
This integral is given in [4] formula (7), giving the distribution
this contains a special function: the Meijer G-function. Using Mathematica 12.2 the library has this function ready to use the MeijerG function [6] :
It happens that the distribution PDF of [4] is given by:
(11)
The integral of the PDF follows:
, but written as the PDF [4] , is not analytical, thus can only be numerically integrated. The novelty of this work is to perform all relevant integrals and formal derivatives of these quantities bypassing the use MeijerG functions of the Zaninetti article [4] .
At this stage, it is possible to get new results using Mathematica but also, without using MeijerG functions:
is not integrable analytically,
but can be numerically integrated with the NIntegrate function producing a table with a fixed step, this is done in [4] , thus constructing the DF (distribution function) with accuracy.
If the approximation
, is made it simplifies.
The integral is analytical:
(12)
The asymptotic probability law of the relativistic MB is given by:
(13)
(14)
It can be compared with the data of the numerical integral, for low
values
and
.
It gives the same DF than the function numerically integrated from the PDF [4] , it is a new result.
Figure 1 shows the distribution of the relativistic Maxwell-Boltzmann formula given in Equation (9) for 3 temperatures T.
There are no differences in the Figures for the numerically integrated DF and these obtained with our approximation.
The mode of the relativistic MB is given by deriving and solving:
(15)
(16)
Solving
gives the mode:
The real solution of the cubic equation (the same than obtained, formula (11) in [4] ) without using the Meijer G-function.
(17)
The real root is:
(18)
(19)
![]()
Figure 1. PDF of the relativistic MB as a function of γ for different values of T.
Another approach is possible, to find the distribution function DF, using the v velocity variable with c = 1.
That is
, the PDF function becomes:
(20)
and its derivative
is:
Solving
gives the mode.
2. Main Results
It is accepted that relativistic effects are taken into account with the Maxwell-Jüttner distribution:
(21)
where
.
Following: [4] , m is the mass of the atom, and
is the Boltzmann constant, and the
the temperature of the medium.
Using the Zaninetti formula for the Maxwell-Jüttner distribution and its average value which implies the function [6] :
(22)
Instead it is possible to calculate all relevant quantities as average value or variance of the Maxwell-Jüttner distribution with the useful.
Figure 2 Results obtained by calculating DF with numerical integration [4] .
Figure 3 Results obtained calculating DF by analytical integration, new way to get all quantities appearing in [4] .
Figure 4 Exact formal Maxwell-Jüttner distribution, with the
variable [3]
(23)
It gives:
(24)
![]()
Figure 2. L. Zaninetti DF Distribution Function of the relativistic MB obtained by numerical integration: T = 0.1 red curve, T = 0.5 blue curve T = 1, green curve.
![]()
Figure 3. DF Distribution Function of the relativistic MB obtained by analytical integration: T = 0.1 red curve, T = 0.5, blue curve T = 1, green curve.
![]()
Figure 4. Maxwell-Jüttner relativistic PDF Distribution Function for different Θ red curve:
blue curve
green curve
.
This integral: the distribution function: the DF of [4] , is normalized by:
Thus the resulting
is:
(25)
This DF function fits perfectly the numerical DF of [4] , obtained by numerical integration. It is possible to find the mode of the
, that is solving:
this gives:
1Figure 5 shows the mode, written Mode (Θ) obtained solving
.
It has to be noticed that part of the Mode (Θ), the part containing
is an imaginary number, because a negative square root, for all positive Θ, getting rid of the imaginary part enables a real curve (Figure 5), this occurs in [4] and remains a theoretical problem when solving:
with Mathematica or Maple software.
3. Application to Real Plasmas
I shall use the Zaninetti magnetic B field frequency that is:
inserted in the PDF, whose general formula is:
(26)
This leads to:
(27)
4. Maxwell-Jüttner Distribution with Ee Electric Field
This leads to: it is well known that applying an electric field Ee onto an atom, the interaction energy is:
, this is the linear Stark effect, the factor
appears when the Hamiltonian of the atom plus the energy associated with the electric field Ee:
(28)
is solved.
That is: the Stark energy is given by the mean quantity
,
being the hydrogen wave functions in spherical coordinates, yielding for the energy
, with
The frequencies associated with the linear Stark effect are defined as:
, h is the Planck constant and Ee the electric field are defined by:
(29)
![]()
Figure 5. Maxwell-Jüttner relativistic mode as a function of Θ for different Θ, taking the real part of the theoretical mode(Θ).
(30)
n is the principal quantum number of the atoms in the plasmas, it can be integer when considering atoms in low states, or such as
for atoms (Li, Na, K). For all calculations, the definition of temperature T of the plasmas is
used as in [4] :
, this temperature
, is quite high
compared to the ionization energy of an hydrogen atom expressed in Kelvin
, thus even with a temperature
, most of the atoms should be ionized, part of an hot plasma.
It is still possible define a Stark frequency using the Stark energy:
, that is
, where the length z is a characteristic length, that could be the Debye length
.
I shall use the definition of the distribution
given by [4] , that is Equation (26): changing the frequency
into
for temperatures
and for higher temperatures
z being a length characteristic of the plasma, it could be the Debye length
. The formula from [4] with the magnetic field B frequency distribution is changed with the electric field Ee giving the equation:
(31)
Figure 6 shows frequencies obtained with the Stark energy
is inserted in
, with
.
Figure 7 gives frequencies2,3 Numerically the distribution is:
![]()
Figure 6. Stark frequencies versus variable Ee field using the distribution
these: blue curve T = 0.1, red T = 0.5, green T = 1 in
units, the highest frequencies occur for small Ee field values and z length, here
, and low T temperatures.
![]()
Figure 7. Stark Energy frequencies in the relativistic
T varies from 0.1 to 1 in
units, blue curve T = 1, green curve T = 0.5, red curve T = 0.1.
(32)
Comments of Figure 7: the distribution
shows that the lesser are the values of the energy
the highest are the frequencies
because of the factor
is higher for small values z, if used on atom
scale such as the Bohr radius a0 gives an higher frequency distribution than a z factor of z = 10−2 m, the frequencies from a z near a0 compared to the z = 10−2 m
are enhanced by a factor of fac =
. Using the Maxwell-Jüttner PDF distribution Equation (22) and defining
yields:
(33)
Numerical results are obtained inserting the Planck constant h and electric charge of the electron e, and giving to the electric field
this field E is 26 times higher than the ionizing field for hydrogen
(34)
For a hot plasma,
and a field
the numerical distribution is:
(35)
and the graphical representation is:
Figure 8 gives the energy distribution for a field value
and a z length
.
![]()
Figure 8. Stark frequencies in the Maxwell-Jüttner distribution function of Θ and z the characteristic plasma length.
5. Correct Relativistic M-B Distribution for Any Energy En
Providing an energy written En in the equation, gives the good relativistic behavior of the Maxwell-Boltzmann distribution:
(36)
The same applies to the Maxwell-Jüttner distribution that is:
(37)
Numerically:
(38)
It is readily seen that to get real values it is necessary that any kind of energy En in Joule units has to be
numerically
.
It is possible to check the normalization of this distribution integrating on the variables
with a initial value
,
, and on the
variable Θ, these calculations are quite heavy if one wants an analytical integration. The integrated distributions are obtained with parameters:
and
, these replace integration to infinity and give analytical results with:
and
. This means that instead of an integration of the variables
and Θ towards infinity, the function Integrate works well, if the limits of integration to Infinity, are replaced by a integration with:
rather than
, the same apply for the variable Θ that is again,
replaces
in that way the normalization of
is obtained.
Figure 9 shows the variation of the energy distribution
when any energy is quantized by the relation
.
Defining a thermal energy by:
gives the following figure:
Figure 10 plots the Maxwell Jüttner distribution, for
and
, the temperatures T vary from 0 to 100, these are given in
units,
to get the temperatures in Kelvin one has to multiply T by the factor: 5.92965 × 109.
![]()
Figure 9. Maxwell-Jüttner relativistic distribution of energy
. Different Θ values. Red curve is for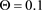 . Other curves are for
and
. These curves merge for
.
. Other curves are for
and
. These curves merge for
.
![]()
Figure 10. Maxwell-Jüttner relativistic distribution of energy
.
6. Conclusions
This article reviews significant progress made for this interesting problem to adapt the Maxwell probability distribution to Einstein relativity theory, (no physical velocity should be greater than c velocity of light), this leads to a correction given partly by the Maxwell-Jüttner distribution. It is known that this correction is important for high temperatures obtained in astrophysical or fusion plasmas, such high temperatures are such that:
.
It is desirable that the manipulation of these quantities: PDF and DF distributions relativity compatible with the correct Maxwell-Jüttner theoretical expression, should give a better handling of real plasmas.
The use of modern software like Mathematica gives an unique way to deal with special functions, all the results obtained by L. Zaninetti with Maple are
found again, with the special function
.
The basic approximation
brings analytical results that fit perfectly the numerical construction of the distribution function DF of [4] . Notebooks developed by the author can be sent to interested readers of this article.
Acknowledgements
Sincere thanks to the referee for his reading of this article and its sound requested changes.4
NOTES
1This equation has a meaning, that is gives a correct Mode(Θ) and the curve representing it, if one deletes its imaginary part
.
2
is the Bohr radius an
the electric charge of an electron, and
the Boltzmann constant,
.
3The electric field is written as Ee or F to distinguish these quantities from the energy written En.
4Mathematica notebooks concerning calculations of this article can be furnished on request.