Hostname: page-component-76fb5796d-25wd4 Total loading time: 0 Render date: 2024-04-26T09:00:49.665Z Has data issue: false hasContentIssue false
  • English
  • Français

Liouville property and quasi-isometries on negatively curved Riemannian surfaces

Part of: Global differential geometry Other generalizations Riemann surfaces

Published online by Cambridge University Press:  10 February 2023

Ana Granados Open the ORCID record for Ana Granados [Opens in a new window] ,
Domingo Pestana Open the ORCID record for Domingo Pestana [Opens in a new window] ,
Ana Portilla Open the ORCID record for Ana Portilla [Opens in a new window] ,
José M. Rodríguez Open the ORCID record for José M. Rodríguez [Opens in a new window]  and
Eva Tourís Open the ORCID record for Eva Tourís [Opens in a new window]
Ana Granados
Affiliation:
Saint Louis University, Madrid Campus Avenida del Valle 34, 28003 Madrid, Spain (ana.granados@slu.edu)
Domingo Pestana
Affiliation:
Departamento de Matemáticas, Universidad Carlos III de Madrid, Avenida de la Universidad 30, 28911 Leganés, Madrid, Spain (dompes@math.uc3m.es)
Ana Portilla
Affiliation:
Saint Louis University, Madrid Campus Avenida del Valle 34, 28003 Madrid, Spain (ana.portilla@slu.edu)
José M. Rodríguez
Affiliation:
Departamento de Matemáticas, Universidad Carlos III de Madrid, Avenida de la Universidad 30, 28911 Leganés, Madrid, Spain (jomaro@math.uc3m.es)
Eva Tourís
Affiliation:
Departamento de Matemáticas, Facultad de Ciencias, Universidad Autónoma de Madrid, Campus de Cantoblanco, 28049 Madrid, Spain (eva.touris@uam.es)
Get access Rights & Permissions [Opens in a new window]

Abstract

Kanai proved powerful results on the stability under quasi-isometries of numerous global properties (including Liouville property) between Riemannian manifolds of bounded geometry. Since his work focuses more on the generality of the spaces considered than on the two-dimensional geometry, Kanai's hypotheses in many cases are not satisfied in the context of Riemann surfaces endowed with the Poincaré metric. In this work we fill that gap for the Liouville property, by proving its stability by quasi-isometries for every Riemann surface (and even Riemannian surfaces with pinched negative curvature). Also, a key result characterizes Riemannian surfaces which are quasi-isometric to $\mathbb {R}$.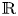

Keywords

Liouville propertyendsquasi-isometryRiemann surfacePoincaré metricpinched negative curvature

MSC classification

Primary: 30F45: Conformal metrics (hyperbolic, Poincaré, distance functions)
Secondary: 53C20: Global Riemannian geometry, including pinching 30F20: Classification theory of Riemann surfaces 31C12: Potential theory on Riemannian manifolds
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 154 , Issue 1 , February 2024 , pp. 131 - 153
Check for updates
Copyright
Copyright © The Author(s), 2023. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alvarez, V. and Rodríguez, J. M.. Structure theorems for Riemann and topological surfaces. J. London Math. Soc. 69 (2004), 153–168.CrossRefGoogle Scholar
Anderson, M. T.. The Dirichlet problem at infinity for manifolds of negative curvature. J. Diff. Geom. 18 (1983), 701–721.Google Scholar
Anderson, M. T. and Schoen, R.. Positive harmonic functions on complete manifolds of negative curvature. Ann. Math. (2) 121 (1985), 429–461.CrossRefGoogle Scholar
Beardon, A. F.. The geometry of discrete groups (New York, Springer-Verlag, 1983).CrossRefGoogle Scholar
Bers, L., An Inequality for Riemann Surfaces. Differential Geometry and Complex Analysis. H. E. Rauch Memorial Volume (Springer-Verlag, New York, 1985).Google Scholar
Bridson, M. R. and Haefliger, A., Metric spaces of non-positive curvature. Vol. 319 of Grundlehren der Mathematischen Wissenschaften (Springer-Verlag, Berlin, Heidelberg, 1999).CrossRefGoogle Scholar
Buser, P.. The collar theorem and examples. Manuscr. Math. 25 (1978), 349–357.CrossRefGoogle Scholar
Buser, P.. Geometry and Spectra of Compact Riemann Surfaces (Boston, Birkhäuser, 1992).Google Scholar
Cantón, A., Granados, A., Portilla, A. and Rodríguez, J. M.. Quasi-isometries and isoperimetric inequalities in planar domains. J. Math. Soc. Japan 67 (2015), 127–157.CrossRefGoogle Scholar
Chavel, I.. Eigenvalues in Riemannian Geometry (New York, Academic Press, 1984).Google Scholar
Cheng., S.-Y. and Yau, S.-T.. Differential equations on Riemannian manifolds and their geometric applications. Comm. Pure Appl. Math. 28 (1975), 333–354.CrossRefGoogle Scholar
Coulhon, T. and L. Saloff-Coste, T.. Variétés riemanniennes isométriques à l'infini. Rev. Mat. Iberoamericana 11 (1995), 687–726.CrossRefGoogle Scholar
Delmotte, T.. Parabolic Harnack inequality and estimates of Markov chains on graphs. Rev. Mat. Iberoamericana 15 (1999), 181–232.CrossRefGoogle Scholar
Freedman, M., Hass, J. and Scott, P.. Closed Geodesics on Surfaces. Bull. London Math. Soc. 14 (1982), 385–391.CrossRefGoogle Scholar
Ghys, E. and de la Harpe, P., Sur les Groupes Hyperboliques d'après Mikhael Gromov. Progress in Mathematics, Vol. 83 (Birkhäuser, Basel, 1990).CrossRefGoogle Scholar
Granados, A., Pestana, D., Portilla, A. and Rodríguez, J. M.. Stability of $p$-parabolicity under quasi-isometries. Math. Nachr. 295 (2022), 536–559.CrossRefGoogle Scholar
Granados, A., Pestana, D., Portilla, A., Rodríguez, J. M. and Tourís, E.. Stability of the volume growth rate under quasi-isometries. Rev. Matem. Complutense 33 (2020), 231–270.CrossRefGoogle Scholar
Gromov, M., Hyperbolic manifolds, groups and actions. Ann. of Math. Studies, Vol. 97 (Princeton, Princeton Univ. Press, 1981), pp. 183–213.CrossRefGoogle Scholar
Gromov, M., Hyperbolic groups, in ‘Essays in group theory’. Edited by Gersten, S. M., M. S. R. I. Publ. Vol. 8 (Springer, 1987), pp. 75–263.Google Scholar
Kanai, M.. Rough isometries and combinatorial approximations of geometries of non-compact Riemannian manifolds. J. Math. Soc. Japan 37 (1985), 391–413.CrossRefGoogle Scholar
Lyons, T.. Instability of the Liouville property for quasi-isometric Riemannian manifolds and reversible Markov chains. J. Diff. Geom. 26 (1987), 33–66.Google Scholar
Moser, J.. On Harnack's theorem for elliptic differential equations. Comm. Pure Appl. Math. 14 (1961), 577–591.CrossRefGoogle Scholar
Portilla, A., Rodríguez, J. M. and Tourís, E.. Structure Theorem for Riemannian surfaces with arbitrary curvature. Math. Z. 271 (2012), 45–62.CrossRefGoogle Scholar
Randol, B.. Cylinders in Riemann surfaces. Comment. Math. Helv. 54 (1979), 1–5.CrossRefGoogle Scholar
Ratcliffe, J. G.. Foundations of Hyperbolic Manifolds (New York, Springer-Verlag, 1994).CrossRefGoogle Scholar
Shimizu, H.. On discontinuous groups operating on the product of upper half-planes. Ann. Math. 77 (1963), 33–71.CrossRefGoogle Scholar
Sullivan, D.. The Dirichlet problem at infinity for a negatively curved manifold. J. Diff. Geom. 18 (1983), 723–732.Google Scholar
Yau, S.-T.. Harmonic functions on complete Riemannian manifolds. Comm. Pure Appl. Math. 28 (1975), 201–228.CrossRefGoogle Scholar