No CrossRef data available.
Article contents
Velocity gradient analysis of a head-on vortex ring collision
Published online by Cambridge University Press: 05 March 2024
Abstract
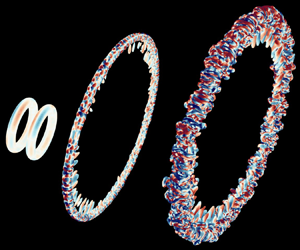
We simulate the head-on collision between vortex rings with circulation Reynolds numbers of 4000 using an adaptive, multiresolution solver based on the lattice Green's function. The simulation fidelity is established with integral metrics representing symmetries and discretization errors. Using the velocity gradient tensor and structural features of local streamlines, we characterize the evolution of the flow with a particular focus on its transition and turbulent decay. Transition is excited by the development of the elliptic instability, which grows during the mutual interaction of the rings as they expand radially at the collision plane. The development of antiparallel secondary vortex filaments along the circumference mediates the proliferation of small-scale turbulence. During turbulent decay, the partitioning of the velocity gradients approaches an equilibrium that is dominated by shearing and agrees well with previous results for forced isotropic turbulence. We also introduce new phase spaces for the velocity gradients that reflect the interplay between shearing and rigid rotation and highlight geometric features of local streamlines. In conjunction with our other analyses, these phase spaces suggest that, while the elliptic instability is the predominant mechanism driving the initial transition, its interplay with other mechanisms, e.g. the Crow instability, becomes more important during turbulent decay. Our analysis also suggests that the geometry-based phase space may be promising for identifying the effects of the elliptic instability and other mechanisms using the structure of local streamlines. Moving forward, characterizing the organization of these mechanisms within vortices and universal features of velocity gradients may aid in modelling turbulent flows.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press
References
Archer, P.J., Thomas, T.G. & Coleman, G.N. 2008 Direct numerical simulation of vortex ring evolution from the laminar to the early turbulent regime. J. Fluid Mech. 598, 201–226.CrossRefGoogle Scholar
Archer, P.J., Thomas, T.G. & Coleman, G.N. 2010 The instability of a vortex ring impinging on a free surface. J. Fluid Mech. 642, 79–94.CrossRefGoogle Scholar
Arvidsson, P.M., Kovács, S.J., Töger, J., Borgquist, R., Heiberg, E., Carlsson, M. & Arheden, H. 2016 Vortex ring behavior provides the epigenetic blueprint for the human heart. Sci. Rep. 6, 22021.CrossRefGoogle ScholarPubMed
Ayala, D. & Protas, B. 2017 Extreme vortex states and the growth of enstrophy in three-dimensional incompressible flows. J. Fluid Mech. 818, 772–806.CrossRefGoogle Scholar
Balakrishna, N., Mathew, J. & Samanta, A. 2020 Inviscid and viscous global stability of vortex rings. J. Fluid Mech. 902, A9.CrossRefGoogle Scholar
Bergdorf, M., Koumoutsakos, P. & Leonard, A. 2007 Direct numerical simulations of vortex rings at 
$Re_\varGamma = 7500$. J. Fluid Mech. 581, 495–505.CrossRefGoogle Scholar
$Re_\varGamma = 7500$. J. Fluid Mech. 581, 495–505.CrossRefGoogle ScholarBlanco-Rodríguez, F.J. & Le Dizès, S. 2016 Elliptic instability of a curved Batchelor vortex. J. Fluid Mech. 804, 224–247.CrossRefGoogle Scholar
Blanco-Rodríguez, F.J. & Le Dizès, S. 2017 Curvature instability of a curved Batchelor vortex. J. Fluid Mech. 814, 397–415.CrossRefGoogle Scholar
Blanco-Rodríguez, F.J., Le Dizès, S., Selçuk, C., Delbende, I. & Rossi, M. 2015 Internal structure of vortex rings and helical vortices. J. Fluid Mech. 785, 219–247.CrossRefGoogle Scholar
Brasey, V. & Hairer, E. 1993 Half-explicit Runge–Kutta methods for differential-algebraic systems of index 2. SIAM J. Numer. Anal. 30 (2), 538–552.CrossRefGoogle Scholar
Bush, J.W.M., Thurber, B.A. & Blanchette, F. 2003 Particle clouds in homogeneous and stratified environments. J. Fluid Mech. 489, 29–54.CrossRefGoogle Scholar
Chakraborty, P., Balachandar, S. & Adrian, R.J. 2005 On the relationships between local vortex identification schemes. J. Fluid Mech. 535, 189–214.CrossRefGoogle Scholar
Chang, C. & Llewellyn Smith, S.G. 2021 Density and surface tension effects on vortex stability. Part 2. Moore–Saffman–Tsai–Widnall instability. J. Fluid Mech. 913, A15.CrossRefGoogle Scholar
Cheng, M., Lou, J. & Lim, T.T. 2016 Evolution of an elliptic vortex ring in a viscous fluid. Phys. Fluids 28 (3), 037104.CrossRefGoogle Scholar
Cheng, M., Lou, J. & Lim, T.T. 2018 Numerical simulation of head-on collision of two coaxial vortex rings. Fluid Dyn. Res. 50 (6), 065513.CrossRefGoogle Scholar
Cheng, M., Lou, J. & Lim, T.T. 2019 Collision and reconnection of viscous elliptic vortex rings. Phys. Fluids 31 (6), 067107.CrossRefGoogle Scholar
Chong, M.S., Perry, A.E. & Cantwell, B.J. 1990 A general classification of three-dimensional flow fields. Phys. Fluids A 2 (5), 765–777.CrossRefGoogle Scholar
Chu, C., Wang, C., Chang, C., Chang, R. & Chang, W. 1995 Head-on collision of two coaxial vortex rings: experiment and computation. J. Fluid Mech. 296, 39–71.CrossRefGoogle Scholar
Crow, S.C. 1970 Stability theory for a pair of trailing vortices. AIAA J. 8 (12), 2172–2179.CrossRefGoogle Scholar
Cummins, C., Seale, M., Macente, A., Certini, D., Mastropaolo, E., Viola, I.M. & Nakayama, N. 2018 A separated vortex ring underlies the flight of the dandelion. Nature 562 (7727), 414–418.CrossRefGoogle ScholarPubMed
Dabiri, J.O. 2005 Flow patterns generated by oblate medusan jellyfish: field measurements and laboratory analyses. J. Exp. Biol. 208 (7), 1257–1265.CrossRefGoogle ScholarPubMed
Das, R. & Girimaji, S.S. 2019 On the Reynolds number dependence of velocity-gradient structure and dynamics. J. Fluid Mech. 861, 163–179.CrossRefGoogle Scholar
Das, R. & Girimaji, S.S. 2020 a Characterization of velocity-gradient dynamics in incompressible turbulence using local streamline geometry. J. Fluid Mech. 895, A5.CrossRefGoogle Scholar
Das, R. & Girimaji, S.S. 2020 b Revisiting turbulence small-scale behavior using velocity gradient triple decomposition. New J. Phys. 22 (6), 063015.CrossRefGoogle Scholar
Das, R. & Girimaji, S.S. 2022 The effect of large-scale forcing on small-scale dynamics of incompressible turbulence. J. Fluid Mech. 941, A34.CrossRefGoogle Scholar
Dazin, A., Dupont, P. & Stanislas, M. 2006 Experimental characterization of the instability of the vortex rings. Part 2. Non-linear phase. Exp. Fluids 41 (3), 401–413.CrossRefGoogle Scholar
Dong, X., Gao, Y. & Liu, C. 2019 New normalized Rortex/vortex identification method. Phys. Fluids 31 (1), 011701.CrossRefGoogle Scholar
Dong, X., Wang, Y., Chen, X., Dong, Y., Zhang, Y. & Liu, C. 2018 Determination of epsilon for Omega vortex identification method. J. Hydrodyn. 30 (4), 541–548.CrossRefGoogle Scholar
Dorschner, B., Yu, K., Mengaldo, G. & Colonius, T. 2020 A fast multi-resolution lattice Green's function method for elliptic difference equations. J. Comput. Phys. 407, 109270.CrossRefGoogle Scholar
Epps, B.P. 2017 Review of vortex identification methods. In 55th AIAA Aerospace Sciences Meeting, pp. 2017–0989.Google Scholar
Fukumoto, Y. & Hattori, Y. 2005 Curvature instability of a vortex ring. J. Fluid Mech. 526, 77–115.CrossRefGoogle Scholar
Gao, Y. & Liu, C. 2018 Rortex and comparison with eigenvalue-based vortex identification criteria. Phys. Fluids 30 (8), 085107.CrossRefGoogle Scholar
Gao, Y. & Liu, C. 2019 Rortex based velocity gradient tensor decomposition. Phys. Fluids 31 (1), 011704.CrossRefGoogle Scholar
Gao, Y., Yu, Y., Liu, J. & Liu, C. 2019 Explicit expressions for Rortex tensor and velocity gradient tensor decomposition. Phys. Fluids 31 (8), 081704.CrossRefGoogle Scholar
Gharib, M., Rambod, E. & Shariff, K. 1998 A universal time scale for vortex ring formation. J. Fluid Mech. 360, 121–140.CrossRefGoogle Scholar
Girimaji, S.S. & Speziale, C.G. 1995 A modified restricted Euler equation for turbulent flows with mean velocity gradients. Phys. Fluids 7 (6), 1438–1446.CrossRefGoogle Scholar
Günther, T. & Theisel, H. 2018 The state of the art in vortex extraction. Comput. Graph. Forum 37 (6), 149–173.CrossRefGoogle Scholar
Hattori, Y., Blanco-Rodríguez, F.J. & Le Dizès, S. 2019 Numerical stability analysis of a vortex ring with swirl. J. Fluid Mech. 878, 5–36.CrossRefGoogle Scholar
Hoffman, J. 2021 Energy stability analysis of turbulent incompressible flow based on the triple decomposition of the velocity gradient tensor. Phys. Fluids 33 (8), 081707.CrossRefGoogle Scholar
Hunt, J.C.R., Wray, A.A. & Moin, P. 1988 Eddies, streams, and convergence zones in turbulent flows. In Proceedings of the 1988 Summer Program, pp. 193–208. Center for Turbulence Research.Google Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.CrossRefGoogle Scholar
Johnson, W. 2005 Model for vortex ring state influence on rotorcraft flight dynamics. NASA Tech. Rep. NASA/TP-2005-213477.Google Scholar
Kang, D., Yun, D. & Protas, B. 2020 Maximum amplification of enstrophy in three-dimensional Navier–Stokes flows. J. Fluid Mech. 893, A22.CrossRefGoogle Scholar
Kerswell, R.R. 2002 Elliptical instability. Annu. Rev. Fluid Mech. 34, 83–113.CrossRefGoogle Scholar
Keylock, C.J. 2018 The Schur decomposition of the velocity gradient tensor for turbulent flows. J. Fluid Mech. 848, 876–905.CrossRefGoogle Scholar
Kida, S., Takaoka, M. & Hussain, F. 1991 Collision of two vortex rings. J. Fluid Mech. 230, 583–646.CrossRefGoogle Scholar
Kolář, V. 2004 2D velocity-field analysis using triple decomposition of motion. In Proceedings of the 15th Australasian Fluid Mechanics Conference, p. AFMC00017. The University of Sydney.Google Scholar
Kolář, V. 2007 Vortex identification: new requirements and limitations. Int. J. Heat Fluid Flow 28 (4), 638–652.CrossRefGoogle Scholar
Kolář, V. & Šístek, J. 2020 Consequences of the close relation between Rortex and swirling strength. Phys. Fluids 32 (9), 091702.CrossRefGoogle Scholar
Kolář, V. & Šístek, J. 2022 Disappearing vortex problem in vortex identification: non-existence for selected criteria. Phys. Fluids 34 (7), 071704.CrossRefGoogle Scholar
Krueger, P.S. & Gharib, M. 2003 The significance of vortex ring formation to the impulse and thrust of a starting jet. Phys. Fluids 15 (5), 1271–1281.CrossRefGoogle Scholar
Kyle, R., Lee, Y.C. & Früh, W. 2020 Propeller and vortex ring state for floating offshore wind turbines during surge. Renew. Energy 155, 645–657.CrossRefGoogle Scholar
Leweke, T., Le Dizès, S. & Williamson, C.H.K. 2016 Dynamics and instabilities of vortex pairs. Annu. Rev. Fluid Mech. 48, 507–541.CrossRefGoogle Scholar
Lim, T. & Nickels, T. 1992 Instability and reconnection in the head-on collision of two vortex rings. Nature 357, 225–227.CrossRefGoogle Scholar
Limbourg, R. & Nedić, J. 2021 Formation of an orifice-generated vortex ring. J. Fluid Mech. 913, A29.CrossRefGoogle Scholar
Liska, S. 2016 Fast lattice Green's function methods for viscous incompressible flows on unbounded domains. PhD Thesis, California Institute of Technology.CrossRefGoogle Scholar
Liska, S. & Colonius, T. 2014 A parallel fast multipole method for elliptic difference equations. J. Comput. Phys. 278, 76–91.CrossRefGoogle Scholar
Liska, S. & Colonius, T. 2016 A fast lattice Green's function method for solving viscous incompressible flows on unbounded domains. J. Comput. Phys. 316, 360–384.CrossRefGoogle Scholar
Liu, C., Gao, Y., Dong, X., Wang, Y., Liu, J., Zhang, Y., Cai, X. & Gui, N. 2019 a Third generation of vortex identification methods: Omega and Liutex/Rortex based systems. J. Hydrodyn. 31 (2), 205–223.CrossRefGoogle Scholar
Liu, C., Gao, Y., Tian, S. & Dong, X. 2018 Rortex—a new vortex vector definition and vorticity tensor and vector decompositions. Phys. Fluids 30 (3), 035103.CrossRefGoogle Scholar
Liu, C., Wang, Y., Yang, Y. & Duan, Z. 2016 New omega vortex identification method. Sci. China Phys. Mech. Astron. 59 (8), 684711.CrossRefGoogle Scholar
Liu, J., Gao, Y. & Liu, C. 2019 b An objective version of the Rortex vector for vortex identification. Phys. Fluids 31 (6), 065112.CrossRefGoogle Scholar
Liu, J., Gao, Y., Wang, Y. & Liu, C. 2019 c Objective Omega vortex identification method. J. Hydrodyn. 31 (3), 455–463.CrossRefGoogle Scholar
Liu, J. & Liu, C. 2019 Modified normalized Rortex/vortex identification method. Phys. Fluids 31 (6), 061704.CrossRefGoogle Scholar
Lopez, J.M. & Bulbeck, C.J. 1993 Behavior of streamwise rib vortices in a three-dimensional mixing layer. Phys. Fluids A 5 (7), 1694–1702.CrossRefGoogle Scholar
Lu, L. & Doering, C.R. 2008 Limits on enstrophy growth for solutions of the three-dimensional Navier–Stokes equations. Indiana Univ. Math. J. 57 (6), 2693–2727.CrossRefGoogle Scholar
McKeown, R., Ostilla-Mónico, R., Pumir, A., Brenner, M.P. & Rubinstein, S.M. 2018 Cascade leading to the emergence of small structures in vortex ring collisions. Phys. Rev. Fluids 3 (12), 124702.CrossRefGoogle Scholar
McKeown, R., Ostilla-Mónico, R., Pumir, A., Brenner, M.P. & Rubinstein, S.M. 2020 Turbulence generation through an iterative cascade of the elliptical instability. Sci. Adv. 6 (9), eaaz2717.CrossRefGoogle ScholarPubMed
Mishra, A., Pumir, A. & Ostilla-Mónico, R. 2021 Instability and disintegration of vortex rings during head-on collisions and wall interactions. Phys. Rev. Fluids 6 (10), 104702.CrossRefGoogle Scholar
Mohseni, K., Ran, H. & Colonius, T. 2001 Numerical experiments on vortex ring formation. J. Fluid Mech. 430, 267–282.CrossRefGoogle Scholar
Moore, D.W. & Saffman, P.G. 1975 The instability of a straight vortex filament in a strain field. Proc. R. Soc. Lond. A 346 (1646), 413–425.Google Scholar
Nagata, R., Watanabe, T., Nagata, K. & da Silva, C.B. 2020 Triple decomposition of velocity gradient tensor in homogeneous isotropic turbulence. Comput. Fluids 198, 104389.CrossRefGoogle Scholar
Nakayama, K. 2017 Topological features and properties associated with development/decay of vortices in isotropic homogeneous turbulence. Phys. Rev. Fluids 2 (1), 014701.CrossRefGoogle Scholar
Nguyen, V.L., Phan, T.T., Duong, V.D. & Le, N.T.P. 2021 Turbulent energy cascade associated with viscous reconnection of two vortex rings. Phys. Fluids 33 (8), 085117.CrossRefGoogle Scholar
O'Farrell, C. & Dabiri, J.O. 2014 Pinch-off of non-axisymmetric vortex rings. J. Fluid Mech. 740, 61–96.CrossRefGoogle Scholar
Oshima, Y. 1978 Head-on collision of two vortex rings. J. Phys. Soc. Japan 44 (1), 328–331.CrossRefGoogle Scholar
Ostilla-Mónico, R., McKeown, R., Brenner, M.P., Rubinstein, S.M. & Pumir, A. 2021 Cascades and reconnection in interacting vortex filaments. Phys. Rev. Fluids 6 (7), 074701.CrossRefGoogle Scholar
Perry, A.E. & Chong, M.S. 1987 A description of eddying motions and flow patterns using critical-point concepts. Annu. Rev. Fluid Mech. 19, 125–155.CrossRefGoogle Scholar
Perry, A.E. & Fairlie, B.D. 1975 Critical points in flow patterns. Adv. Geophys. 18, 299–315.CrossRefGoogle Scholar
Ruggaber, G.J. 2000 Dynamics of particle clouds related to open-water sediment disposal. PhD Thesis, Massachusetts Institute of Technology.Google Scholar
Serrin, J. 1959 Mathematical Principles of Classical Fluid Mechanics, Handbuch der Physik, vol. 8/1, pp. 125–263. Springer.CrossRefGoogle Scholar
Shariff, K. & Leonard, A. 1992 Vortex rings. Annu. Rev. Fluid Mech. 24, 235–279.CrossRefGoogle Scholar
Shariff, K., Verzicco, R. & Orlandi, P. 1994 A numerical study of three-dimensional vortex ring instabilities: viscous corrections and early nonlinear stage. J. Fluid Mech. 279, 351–375.CrossRefGoogle Scholar
Sharma, B., Das, R. & Girimaji, S.S. 2021 Local vortex line topology and geometry in turbulence. J. Fluid Mech. 924, A13.CrossRefGoogle Scholar
Smith, G.B. & Wei, T. 1994 Small-scale structure in colliding off-axis vortex rings. J. Fluid Mech. 259, 281–290.CrossRefGoogle Scholar
Taddeucci, J., Alatorre-Ibarguengoitia, M.A., Palladino, D.M., Scarlato, P. & Camaldo, C. 2015 High-speed imaging of Strombolian eruptions: gas-pyroclast dynamics in initial volcanic jets. Geophys. Res. Lett. 42 (15), 6253–6260.CrossRefGoogle Scholar
Tian, S., Gao, Y., Dong, X. & Liu, C. 2018 Definitions of vortex vector and vortex. J. Fluid Mech. 849, 312–339.CrossRefGoogle Scholar
Tom, J., Carbone, M. & Bragg, A.D. 2021 Exploring the turbulent velocity gradients at different scales from the perspective of the strain-rate eigenframe. J. Fluid Mech. 910, A24.CrossRefGoogle Scholar
Towns, J., et al. 2014 XSEDE: accelerating scientific discovery. Comput. Sci. Eng. 16 (5), 62–74.CrossRefGoogle Scholar
Tsai, C. & Widnall, S.E. 1976 The stability of short waves on a straight vortex filament in a weak externally imposed strain field. J. Fluid Mech. 73 (4), 721–733.CrossRefGoogle Scholar
Walker, J.D.A., Smith, C.R., Cerra, A.W. & Doligalski, T.L. 1987 The impact of a vortex ring on a wall. J. Fluid Mech. 181, 99–140.CrossRefGoogle Scholar
Wang, Y., Gao, Y. & Liu, C. 2018 Letter: Galilean invariance of Rortex. Phys. Fluids 30 (11), 111701.CrossRefGoogle Scholar
Weigand, A. & Gharib, M. 1994 On the decay of a turbulent vortex ring. Phys. Fluids 6 (12), 3806–3808.CrossRefGoogle Scholar
Widnall, S.E., Bliss, D.B. & Tsai, C. 1974 The instability of short waves on a vortex ring. J. Fluid Mech. 66 (1), 35–47.CrossRefGoogle Scholar
Widnall, S.E. & Tsai, C. 1977 The instability of the thin vortex ring of constant vorticity. Phil. Trans. R. Soc. Lond. A 287 (1344), 273–305.Google Scholar
Wu, Y., Zhang, W., Wang, Y., Zou, Z. & Chen, J. 2020 Energy dissipation analysis based on velocity gradient tensor decomposition. Phys. Fluids 32 (3), 035114.CrossRefGoogle Scholar
Yao, J. & Hussain, F. 2020 a On singularity formation via viscous vortex reconnection. J. Fluid Mech. 888, R2.CrossRefGoogle Scholar
Yao, J. & Hussain, F. 2020 b A physical model of turbulence cascade via vortex reconnection sequence and avalanche. J. Fluid Mech. 883, A51.CrossRefGoogle Scholar
Yao, J. & Hussain, F. 2020 c Separation scaling for viscous vortex reconnection. J. Fluid Mech. 900, R4.CrossRefGoogle Scholar
Yao, J., Yang, Y. & Hussain, F. 2021 Dynamics of a trefoil knotted vortex. J. Fluid Mech. 923, A19.CrossRefGoogle Scholar
Yu, K. 2021 Multi-resolution lattice Green's function method for high Reynolds number external flows. PhD Thesis, California Institute of Technology.Google Scholar
Yu, K., Dorschner, B. & Colonius, T. 2022 Multi-resolution lattice Green's function method for incompressible flows. J. Comput. Phys. 459, 110845.CrossRefGoogle Scholar
Zawadzki, I. & Aref, H. 1991 Mixing during vortex ring collision. Phys. Fluids A 3 (5), 1405–1410.CrossRefGoogle Scholar
Zhao, X., Yu, Z., Chapelier, J. & Scalo, C. 2021 Direct numerical and large-eddy simulation of trefoil knotted vortices. J. Fluid Mech. 910, A31.CrossRefGoogle Scholar
Arun and Colonius supplementary movie 1
Evolution of the vortex boundaries throughout the simulation, where the coloring indicates the rotation direction (as in figure 3).
File
10.2 MB
Arun and Colonius supplementary movie 2
Evolution of the vortex boundaries throughout the simulation, where the coloring indicates the relative strength of the shear-rotation correlations (as in figure 12).
File
10.4 MB