For a subshift $(X, \sigma _{X})$ and a subadditive sequence 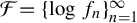${\mathcal F}=\{\log f_{n}\}_{n=1}^{\infty }$ on X, we study equivalent conditions for the existence of 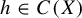$h\in C(X)$ such that 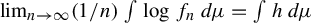$\lim _{n\rightarrow \infty }(1/{n})\int \log f_{n}\, d\kern-1pt\mu =\int h \,d\kern-1pt\mu $ for every invariant measure $\mu $ on X. For this purpose, we first we study necessary and sufficient conditions for ${\mathcal F}$ to be an asymptotically additive sequence in terms of certain properties for periodic points. For a factor map 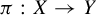$\pi : X\rightarrow Y$ , where 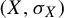$(X, \sigma _{X})$ is an irreducible shift of finite type and 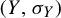$(Y, \sigma _{Y})$ is a subshift, applying our results and the results obtained by Cuneo [Additive, almost additive and asymptotically additive potential sequences are equivalent. Comm. Math. Phys. 37 (3) (2020), 2579–2595] on asymptotically additive sequences, we study the existence of h with regard to a subadditive sequence associated to a relative pressure function. This leads to a characterization of the existence of a certain type of continuous compensation function for a factor map between subshifts. As an application, we study the projection 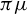$\pi \mu $ of an invariant weak Gibbs measure 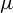$\mu $ for a continuous function on an irreducible shift of finite type.