The free vibration analysis of hybrid porous nanocomposite joined hemispherical–cylindrical–conical shells

Abstract

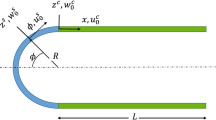
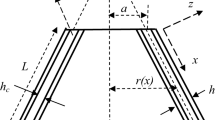
In this research, the natural frequency responses of joined hemispherical–cylindrical–conical shells made of composite three-phase materials have been dealt with in the framework of First-Order Shear Deformation Theory (FOSDT). The joined hemispherical–cylindrical–conical shells are assumed to be made of hybrid porous nanocomposite material with three phases including a matrix of epoxy, macroscale carbon fiber, and nanoscale 3D Graphene Foams (3GFs). For getting the equivalent mechanical properties of the Hybrid Matrix (HM) including polymer epoxy and 3GFs, the well-known rule of the mixture is used. In addition, the effect of porosity throughout the HM is considered using two novel and one well-known porosity distribution pattern. Moreover, the HM is reinforced with transversely isotropic macroscale carbon fibers in which the Halpin–Tsai scheme is used for multiscale homogenization procedure. The governing equations of motion associated with hybrid porous nanocomposite joined hemispherical–cylindrical–conical structures are figured out by implementing Donnell-type shell formulation and Hamilton’s approach. Moreover, an efficient and well-known semi-analytical solution method entitled Generalized Differential Quadrature Method (GDQM) is employed to solve the governing differential equations. To verify the proposed formulation some well-known benchmarks, especially those are composed of homogenous materials have been analyzed, and a good agreement has been achieved. Besides, some other new and applicable problems are considered to investigate the effects of different parameters including various boundary conditions, patterns of porosity distributions, and geometric properties of structure on the vibration behavior of joined shells.

Appendix I

The governing differential equations of joined hemispherical–cylindrical–conical shells in terms of displacement functions are given by

$$ \begin{gathered} \left( {\frac{1}{{R^{2} }}} \right)\{ A_{{11}} \bar{U}^{{cy}} _{{1,\zeta _{{cy}} \zeta _{{cy}} }} R^{2} + \bar{A}_{{11}} \bar{\Psi }_{{\zeta ,\zeta _{{cy}} \zeta _{{cy}} }}^{{cy}} R^{2} \hfill \\ \quad - nR(\bar{A}_{{12}} + \bar{A}_{{66}} )\bar{\Psi }_{{\theta ,\zeta _{{cy}} }}^{{cy}} - nR(A_{{12}} + A_{{66}} )\bar{U}^{{cy}} _{{2,\zeta _{{cy}} }} \hfill \\ \quad + RA_{{12}} \bar{U}^{{cy}} _{{3,\zeta _{{cy}} }} + (\bar{U}^{{cy}} _{1} A_{{22}} + \bar{\Psi }_{\zeta }^{{cy}} \bar{A}_{{22}} ) \hfill \\ \quad - n^{2} A_{{66}} \bar{U}^{{cy}} _{1} - \bar{\Psi }_{\zeta }^{{cy}} n^{2} \bar{A}_{{66}} \} = I_{1} \omega _{n} ^{2} \bar{U}^{{cy}} _{1} + I_{2} \omega _{n} ^{2} \bar{\Psi }_{\zeta }^{{cy}} \hfill \\ \end{gathered} $$

(71)

$$ \begin{gathered} \left( {\frac{1}{{R^{2} }}} \right)\{ \bar{A}_{{66}} \bar{\Psi }_{{\theta ,\zeta _{{cy}} \zeta _{{cy}} }}^{{cy}} R^{2} + A_{{66}} \bar{U}^{{cy}} _{{2,\zeta _{{cy}} \zeta _{{cy}} }} R^{2} \hfill \\ \quad + nR(A_{{12}} + A_{{66}} )\bar{U}^{{cy}} _{{1,\zeta _{{cy}} }} + nR(\bar{A}_{{12}} + \bar{A}_{{66}} )\bar{\Psi }_{{\zeta ,\zeta _{{cy}} }}^{{cy}} \hfill \\ \quad - n\bar{U}^{{cy}} _{2} (\kappa A_{{44}} + A_{{22}} ) + n\bar{U}^{{cy}} _{3} (\kappa A_{{44}} + A_{{22}} )\} \hfill \\ \quad + ( - n^{2} \bar{A}_{{22}} + R\kappa A_{{44}} )\bar{\Psi }_{\theta }^{{cy}} \hfill \\ \;\;\;= I_{1} \omega _{n} ^{2} \bar{U}^{{cy}} _{2} + I_{2} \omega _{n} ^{2} \bar{\Psi }_{\theta }^{{cy}} \hfill \\ \end{gathered} $$

(72)

$$ \begin{gathered} \left( {\frac{1}{{R^{2} }}} \right)\{ \kappa A_{{55}} R^{2} \bar{U}^{{cy}} _{{3,\zeta _{{cy}} \zeta _{{cy}} }} + (R[R\kappa A_{{55}} - \bar{A}_{{12}} )\bar{\Psi }_{{\zeta ,\zeta _{{cy}} }}^{{cy}} \hfill \\ \quad - RA_{{12}} \bar{U}^{{cy}} _{{1,\zeta _{{co}} }} - \bar{U}^{{cy}} _{3} A_{{22}} - \bar{A}_{{22}} n\bar{\Psi }_{\theta }^{{cy}} \hfill \\ \quad + \bar{U}^{{cy}} _{2} (\kappa A_{{44}} + A_{{22}} )n \hfill \\ \quad - ((nA_{{44}} \bar{\Psi }_{\theta }^{{cy}} )R + A_{{44}} n^{2} \bar{U}^{{cy}} _{3} )\kappa \} \hfill \\ \;\;\;= I_{1} \omega _{n} ^{2} \bar{U}^{{cy}} _{3} \hfill \\ \end{gathered} $$

(73)

$$ \begin{gathered} \left( {\frac{1}{{R^{2} }}} \right)\{ \bar{A}_{{11}} \bar{U}^{{cy}} _{{1,\zeta _{{cy}} \zeta _{{cy}} }} R^{2} + \bar{\bar{A}}_{{11}} \bar{\Psi }_{{\zeta ,\zeta _{{cy}} \zeta _{{cy}} }}^{{cy}} R^{2} \hfill \\ \quad - nR(\bar{\bar{A}}_{{12}} + \bar{\bar{A}}_{{66}} )\bar{\Psi }_{{\theta ,\zeta _{{cy}} }}^{{cy}} - nR(\bar{A}_{{12}} + \bar{A}_{{66}} )\bar{U}^{{cy}} _{{2,\zeta _{{cy}} }} \hfill \\ \quad + ( - R^{2} A_{{55}} \kappa + \bar{A}_{{12}} R)\bar{U}^{{cy}} _{3} - n^{2} \bar{A}_{{66}} \bar{U}^{{cy}} _{1} \hfill \\ \quad - R^{2} A_{{55}} \kappa \bar{\Psi }_{\zeta }^{{cy}} - \bar{\Psi }_{\zeta }^{{cy}} n^{2} \bar{\bar{A}}_{{66}} \} \hfill \\ \;\;\;= I_{2} \omega _{n} ^{2} \bar{U}^{{cy}} _{1} + I_{3} \omega _{n} ^{2} \bar{\Psi }_{\zeta }^{{cy}} \hfill \\ \end{gathered} $$

(74)

$$ \begin{gathered} \left( {\frac{1}{{R^{2} }}} \right)\{ \bar{\bar{A}}_{{66}} \bar{\Psi }_{{\theta ,\zeta _{{cy}} \zeta _{{cy}} }}^{{cy}} R^{2} + \bar{A}_{{66}} \bar{U}^{{cy}} _{{2,\zeta _{{cy}} \zeta _{{cy}} }} R^{2} \hfill \\ \quad + nR(\bar{A}_{{12}} + \bar{A}_{{66}} )\bar{U}^{{cy}} _{{1,\zeta _{{cy}} }} + nR(\bar{\bar{A}}_{{12}} + \bar{\bar{A}}_{{66}} )\bar{\Psi }_{{\zeta ,\zeta _{{cy}} }}^{{cy}} \hfill \\ \quad - R^{2} \bar{\Psi }_{\theta }^{{cy}} \kappa A_{{44}} - n^{2} \bar{\bar{A}}_{{22}} \bar{\Psi }_{\theta }^{{cy}} \hfill \\ \quad - \kappa A_{{44}} (n\bar{U}^{{cy}} _{3} - \bar{U}^{{cy}} _{2} )R - \bar{U}^{{cy}} _{2} n^{2} \bar{A}_{{22}} \hfill \\ \quad + \bar{U}^{{cy}} _{3} n\bar{A}_{{22}} \} = I_{2} \omega _{n} ^{2} \bar{U}^{{cy}} _{2} + I_{3} \omega _{n} ^{2} \bar{\Psi }_{\theta }^{{cy}} \hfill \\ \end{gathered} $$

(75)

$$ \begin{gathered} \left( {\frac{1}{{R(\zeta _{{co}} )^{2} }}} \right)\{ A_{{11}} \bar{U}^{{co}} _{{1,\zeta _{{co}} \zeta _{{co}} }} R(\zeta _{{co}} )^{2} + \bar{A}_{{11}} \bar{\Psi }_{{\zeta ,\zeta _{{co}} \zeta _{{co}} }}^{{co}} R(\zeta _{{co}} )^{2} \hfill \\ - nR(\zeta _{{co}} )(\bar{A}_{{12}} + \bar{A}_{{66}} )\bar{\Psi }_{{\theta ,\zeta _{{co}} }}^{{co}} - nR(\zeta _{{co}} )(A_{{12}} + A_{{66}} )\bar{U}^{{co}} _{{2,\zeta _{{co}} }} \hfill \\ \quad + \sin (\Theta )R(\zeta _{{co}} )A_{{11}} \bar{U}^{{co}} _{{1,\zeta _{{co}} }} + \cos (\Theta )R(\zeta _{{co}} )A_{{12}} \bar{U}^{{co}} _{{3,\zeta _{{co}} }} \hfill \\ \quad + \sin (\Theta )R(\zeta _{{co}} )\bar{A}_{{11}} \bar{\Psi }_{{\zeta ,\zeta _{{co}} }}^{{co}} + ( - A_{{22}} \bar{U}^{{co}} _{3} \cos (\Theta ) + ((\bar{A}_{{22}} + \bar{A}_{{66}} )\bar{\Psi }_{\theta }^{{co}} \hfill \\ \quad + \bar{U}^{{co}} _{2} (A_{{22}} + A_{{66}} ))n)\sin (\Theta ) + (\bar{U}^{{co}} _{1} A_{{22}} + \bar{\Psi }_{\zeta }^{{co}} \bar{A}_{{22}} )\cos (\Theta )^{2} \hfill \\ \quad + ( - n^{2} A_{{66}} - A_{{22}} )\bar{U}^{{co}} _{1} - \bar{\Psi }_{\zeta }^{{co}} (n^{2} \bar{A}_{{66}} + \bar{A}_{{22}} )\} = I_{1} \omega _{n} ^{2} \bar{U}^{{co}} _{1} + I_{2} \omega _{n} ^{2} \bar{\Psi }_{\zeta }^{{co}} \hfill \\ \end{gathered} $$

(76)

$$ \begin{gathered} \left( {\frac{1}{{R(\zeta _{{co}} )^{2} }}} \right)\{ \bar{A}_{{66}} \bar{\Psi }_{{\theta ,\zeta _{{co}} \zeta _{{co}} }}^{{co}} R(\zeta _{{co}} )^{2} + A_{{66}} \bar{U}^{{co}} _{{2,\zeta _{{co}} \zeta _{{co}} }} R(\zeta _{{co}} )^{2} \hfill \\ \quad + nR(\zeta _{{co}} )(A_{{12}} + A_{{66}} )\bar{U}^{{co}} _{{1,\zeta _{{co}} }} + nR(\zeta _{{co}} )(\bar{A}_{{12}} + \bar{A}_{{66}} )\bar{\Psi }_{{\zeta ,\zeta _{{co}} }}^{{co}} \hfill \\ \quad + \sin (\Theta )R(\zeta _{{co}} )\bar{A}_{{66}} \bar{\Psi }_{{\theta ,\zeta _{{co}} }}^{{co}} + \sin (\Theta )R(\zeta _{{co}} )A_{{66}} \bar{U}^{{co}} _{{2,\zeta _{{co}} }} \hfill \\ \quad + \cos (\Theta )R(\zeta _{{co}} )\kappa A_{{44}} \bar{\Psi }_{\theta }^{{co}} + (\bar{\Psi }_{\theta }^{{co}} \bar{A}_{{66}} - \bar{U}^{{co}} _{2} (\kappa A_{{44}} - A_{{66}} ))\cos (\Theta )^{2} \hfill \\ \quad + n\bar{U}^{{co}} _{2} (\kappa A_{{44}} + A_{{22}} )\cos (\Theta ) + n((A_{{22}} + A_{{66}} )\bar{U}^{{co}} _{1} \hfill \\ \quad + \bar{\Psi }_{\zeta }^{{co}} (\bar{A}_{{22}} + \bar{A}_{{66}} )\sin (\Theta ) + ( - n^{2} \bar{A}_{{22}} - \bar{A}_{{66}} )\bar{\Psi }_{\theta }^{{co}} - \bar{U}^{{co}} _{2} (n^{2} A_{{22}} + A_{{66}} )\} \hfill \\ \;\;\;= I_{1} \omega _{n} ^{2} \bar{U}^{{co}} _{2} + I_{2} \omega _{n} ^{2} \bar{\Psi }_{\theta }^{{co}} \hfill \\ \end{gathered} $$

(77)

$$ \begin{gathered} \left( {\frac{1}{{R(\zeta _{{co}} )^{2} }}} \right)\{ \kappa A_{{55}} R(\zeta _{{co}} )^{2} \bar{U}^{{co}} _{{3,\zeta _{{co}} \zeta _{{co}} }} + (R(\zeta _{{co}} )[R(\zeta _{{co}} )\kappa A_{{55}} - \bar{A}_{{12}} \cos (\Theta ))\bar{\Psi }_{{\zeta ,\zeta _{{co}} }}^{{co}} \hfill \\ \quad - R(\zeta _{{co}} )\cos (\Theta )A_{{12}} \bar{U}^{{co}} _{{1,\zeta _{{co}} }} + \sin (\Theta )R(\zeta _{{co}} )\kappa A_{{55}} \bar{U}^{{co}} _{{3,\zeta _{{co}} }} \hfill \\ \quad - \bar{U}^{{co}} _{3} \cos (\Theta )^{2} A_{{22}} + (( - A_{{22}} \bar{U}^{{co}} _{1} - \bar{A}_{{22}} \bar{\Psi }_{\zeta }^{{co}} )\sin (\Theta ) \hfill \\ \quad + (\bar{A}_{{22}} \bar{\Psi }_{\theta }^{{co}} + \bar{U}^{{co}} _{2} (\kappa A_{{44}} + A_{{22}} ))n)\cos (\Theta ) \hfill \\ \quad - ((nA_{{44}} \bar{\Psi }_{\theta }^{{co}} - A_{{44}} \bar{\Psi }_{\zeta }^{{co}} \sin (\Theta ))R(\zeta _{{co}} ) + n^{2} \bar{U}^{{co}} _{3} )\kappa \} \hfill \\ \;\;\;= I_{1} \omega _{n} ^{2} \bar{U}^{{co}} _{3} \hfill \\ \end{gathered} $$

(78)

$$ \begin{gathered} \left( {\frac{1}{{R(\zeta _{{co}} )^{2} }}} \right)\{ \bar{A}_{{11}} \bar{U}^{{co}} _{{1,\zeta _{{co}} \zeta _{{co}} }} R(\zeta _{{co}} )^{2} + \bar{\bar{A}}_{{11}} \bar{\Psi }_{{\zeta ,\zeta _{{co}} \zeta _{{co}} }}^{{co}} R(\zeta _{{co}} )^{2} \hfill \\ \quad - nR(\zeta _{{co}} )(\bar{\bar{A}}_{{12}} + \bar{\bar{A}}_{{66}} )\bar{\Psi }_{{\theta ,\zeta _{{co}} }}^{{co}} - nR(\zeta _{{co}} )(\bar{A}_{{12}} + \bar{A}_{{66}} )\bar{U}^{{co}} _{{2,\zeta _{{co}} }} \hfill \\ \quad + ( - R(\zeta _{{co}} )^{2} A_{{55}} \kappa + \bar{A}_{{12}} \cos (\Theta )R(\zeta _{{co}} ))\bar{U}^{{co}} _{3} \hfill \\ \quad + \sin (\Theta )R(\zeta _{{co}} )\bar{A}_{{11}} \bar{U}^{{co}} _{{1,\zeta _{{co}} }} + \sin (\Theta )R(\zeta _{{co}} )\bar{\bar{A}}_{{11}} \bar{\Psi }_{{\zeta ,\zeta _{{co}} }}^{{co}} \hfill \\ \quad - R(\zeta _{{co}} )^{2} A_{{55}} \kappa \bar{\Psi }_{\zeta }^{{co}} + ( - \bar{A}_{{22}} \bar{U}^{{co}} _{3} \cos (\Theta ) \hfill \\ \quad + ((\bar{\bar{A}}_{{22}} + \bar{\bar{A}}_{{66}} )\bar{\Psi }_{\theta }^{{co}} + \bar{U}^{{co}} _{2} (\bar{A}_{{22}} + \bar{A}_{{66}} ))n)\sin (\Theta ) \hfill \\ \quad + (\bar{U}^{{co}} _{1} \bar{A}_{{22}} + \bar{\Psi }_{\zeta }^{{co}} \bar{\bar{A}}_{{22}} )\cos (\Theta )^{2} - (n^{2} A_{{66}} + A_{{22}} )\bar{U}^{{co}} _{1} + \bar{\Psi }_{\zeta }^{{co}} ( - n^{2} \bar{\bar{A}}_{{66}} - \bar{\bar{A}}_{{22}} )\} \hfill \\ \;\;\;= I_{2} \omega _{n} ^{2} \bar{U}^{{co}} _{1} + I_{3} \omega _{n} ^{2} \bar{\Psi }_{\zeta }^{{co}} \hfill \\ \end{gathered} $$

(79)

$$ \begin{gathered} \left( {\frac{1}{{R(\zeta _{{co}} )^{2} }}} \right)\{ \bar{\bar{A}}_{{66}} \bar{\Psi }_{{\theta ,\zeta _{{co}} \zeta _{{co}} }}^{{co}} R(\zeta _{{co}} )^{2} + \bar{A}_{{66}} \bar{U}^{{co}} _{{2,\zeta _{{co}} \zeta _{{co}} }} R(\zeta _{{co}} )^{2} \hfill \\ \quad + nR(\zeta _{{co}} )(\bar{A}_{{12}} + \bar{A}_{{66}} )\bar{U}^{{co}} _{{1,\zeta _{{co}} }} + nR(\zeta _{{co}} )(\bar{\bar{A}}_{{12}} + \bar{\bar{A}}_{{66}} )\bar{\Psi }_{{\zeta ,\zeta _{{co}} }}^{{co}} \hfill \\ \quad + \sin (\Theta )R(\zeta _{{co}} )\bar{\bar{A}}_{{66}} \bar{\Psi }_{{\theta ,\zeta _{{co}} }}^{{co}} + \sin (\Theta )R(\zeta _{{co}} )\bar{A}_{{66}} \bar{U}^{{co}} _{{2,\zeta _{{co}} }} \hfill \\ \quad - R(\zeta _{{co}} )^{2} \bar{\Psi }_{\theta }^{{co}} \kappa A_{{44}} - \kappa A_{{44}} (n\bar{U}^{{co}} _{3} - \cos (\Theta )\bar{U}^{{co}} _{2} )R(\zeta _{{co}} ) \hfill \\ \quad + n((\bar{A}_{{22}} + \bar{A}_{{66}} )\bar{U}^{{co}} _{1} + \bar{\Psi }_{\zeta }^{{co}} (\bar{\bar{A}}_{{22}} + \bar{\bar{A}}_{{66}} )\sin (\Theta ) \hfill \\ \quad + (\bar{\Psi }_{\theta }^{{co}} \bar{\bar{A}}_{{66}} + \bar{U}^{{co}} _{2} (\bar{A}_{{66}} )\cos (\Theta )^{2} + \cos (\Theta )\bar{U}^{{co}} _{3} n\bar{A}_{{22}} \hfill \\ \quad + ( - n^{2} \bar{\bar{A}}_{{22}} - \bar{\bar{A}}_{{66}} )\bar{\Psi }_{\theta }^{{co}} - \bar{U}^{{co}} _{2} (n^{2} \bar{A}_{{22}} + \bar{A}_{{66}} )\} \hfill \\ \;\;\; = I_{2} \omega _{n} ^{2} \bar{U}^{{co}} _{2} + I_{3} \omega _{n} ^{2} \bar{\Psi }_{\theta }^{{co}} \hfill \\ \end{gathered} $$

(80)

$$ \begin{gathered} \left( {\frac{1}{{R^{2} \sin (\zeta _{{hs}} )^{2} }}} \right)\{ ( - \cos (\zeta _{{hs}} )^{2} A_{{11}} + A_{{11}} )\bar{U}^{{hs}} _{{1,\zeta _{{hs}} \zeta _{{hs}} }} + ( - \cos (\zeta _{{hs}} )^{2} \bar{A}_{{11}} + \bar{A}_{{11}} )\bar{\Psi }_{{\zeta ,\zeta _{{hs}} \zeta _{{hs}} }}^{{hs}} \hfill \\ \quad - (\cos (\zeta _{{hs}} )^{2} - 1)(\kappa A_{{55}} + A_{{11}} + A_{{12}} )\bar{U}^{{hs}} _{{3,\zeta _{{hs}} }} - n\sin (\zeta _{{hs}} )(\bar{A}_{{12}} + \bar{A}_{{66}} )\bar{\Psi }_{{\theta ,\zeta _{{hs}} }}^{{hs}} \hfill \\ \quad - n\sin (\zeta _{{hs}} )(\bar{A}_{{12}} + \bar{A}_{{66}} )\bar{U}^{{hs}} _{{2,\zeta _{{hs}} }} + \sin (\zeta _{{hs}} )\cos (\zeta _{{hs}} )A_{{11}} \bar{U}^{{hs}} _{{1,\zeta _{{hs}} }} \hfill \\ \quad + \sin (\zeta _{{hs}} )\cos (\zeta _{{hs}} )\bar{A}_{{11}} \bar{\Psi }_{{\zeta ,\zeta _{{hs}} }}^{{hs}} + ((\kappa A_{{55}} + A_{{12}} - A_{{22}} )\bar{U}^{{hs}} _{1} \hfill \\ \quad + \bar{\Psi }_{\zeta }^{{hs}} ( - \kappa A_{{55}} R + \bar{A}_{{12}} - \bar{A}_{{22}} ))\cos (\zeta _{{hs}} )^{2} + (\bar{U}^{{hs}} _{3} (A_{{11}} + A_{{22}} )\sin (\zeta _{{hs}} ) \hfill \\ \quad + ((\bar{A}_{{22}} + \bar{A}_{{66}} )\bar{\Psi }_{\theta }^{{hs}} + \bar{U}^{{hs}} _{2} (A_{{22}} + A_{{66}} )n)\cos (\zeta _{{hs}} ) + \hfill \\ \quad ( - n^{2} A_{{66}} - \kappa A_{{55}} - A_{{12}} )\bar{U}^{{hs}} _{1} - \bar{\Psi }_{\zeta }^{{hs}} ( - \kappa A_{{55}} R + n^{2} \bar{A}_{{66}} + \bar{A}_{{12}} )\} \hfill \\ \;\;\;= I_{1} \omega _{n} ^{2} \bar{U}^{{hs}} _{1} + I_{2} \omega _{n} ^{2} \bar{\Psi }_{\zeta }^{{hs}} \hfill \\ \end{gathered} $$

(81)

$$ \begin{gathered} \left( {\frac{1}{{R^{2} \sin (\zeta _{{hs}} )^{2} }}} \right)\{ ( - \cos (\zeta _{{hs}} )^{2} \bar{A}_{{66}} + \bar{A}_{{66}} )\bar{\Psi }_{{\theta ,\zeta _{{hs}} \zeta _{{hs}} }}^{{hs}} + ( - \cos (\zeta _{{hs}} )^{2} A_{{66}} + A_{{66}} )\bar{U}^{{hs}} _{{2,\zeta _{{hs}} \zeta _{{hs}} }} \hfill \\ \quad + n\sin (\zeta _{{hs}} )(A_{{12}} + A_{{66}} )\bar{U}^{{hs}} _{{1,\zeta _{{hs}} }} + n\sin (\zeta _{{hs}} )(\bar{A}_{{12}} + \bar{A}_{{66}} )\bar{\Psi }_{{\zeta ,\zeta _{{hs}} }}^{{hs}} \hfill \\ \quad + \sin (\zeta _{{hs}} )\cos (\zeta _{{hs}} )\bar{A}_{{66}} \bar{\Psi }_{{\theta ,\zeta _{{hs}} }}^{{hs}} + \sin (\zeta _{{hs}} )\cos (\zeta _{{hs}} )A_{{66}} \bar{U}^{{hs}} _{{2,\zeta _{{hs}} }} \hfill \\ \quad + (( - \kappa A_{{44}} R - 2\bar{A}_{{66}} )\bar{\Psi }_{\theta }^{{hs}} + \bar{U}^{{hs}} _{2} (\kappa A_{{44}} - 2A_{{66}} ))\cos (\zeta _{{hs}} )^{2} \hfill \\ \quad + ((A_{{22}} + A_{{66}} )\bar{U}^{{hs}} _{1} + \bar{\Psi }_{\zeta }^{{hs}} (\bar{A}_{{22}} + \bar{A}_{{66}} ))n\cos (\zeta _{{hs}} ) + \hfill \\ \quad n\bar{U}^{{hs}} _{3} (\kappa A_{{44}} + A_{{12}} + A_{{22}} )\sin (\zeta _{{hs}} ) + (\kappa A_{{44}} R - n^{2} \bar{A}_{{22}} + \bar{A}_{{66}} )\bar{\Psi }_{\theta }^{{hs}} \hfill \\ \quad - \bar{U}^{{hs}} _{2} (n^{2} A_{{22}} + \kappa A_{{44}} - A_{{66}} )\} = I_{1} \omega _{n} ^{2} \bar{U}^{{hs}} _{2} + I_{2} \omega _{n} ^{2} \bar{\Psi }_{\theta }^{{hs}} \hfill \\ \end{gathered} $$

(82)

$$ \begin{gathered} \left( {\frac{1}{{R^{2} \sin (\zeta _{{hs}} )^{2} }}} \right)\{ ( - \cos (\zeta _{{hs}} )^{2} \kappa A_{{55}} + \kappa A_{{55}} )\bar{U}^{{hs}} _{{3,\zeta _{{hs}} \zeta _{{hs}} }} \hfill \\ \quad + (\cos (\zeta _{{hs}} )^{2} - 1)(\kappa A_{{55}} + A_{{11}} + A_{{12}} )\bar{U}^{{hs}} _{{1,\zeta _{{hs}} }} \hfill \\ \quad + (\cos (\zeta _{{hs}} )^{2} - 1)( - \kappa A_{{55}} R + \bar{A}_{{11}} + \bar{A}_{{12}} )\bar{\Psi }_{{\zeta ,\zeta _{{hs}} }}^{{hs}} \hfill \\ \quad + \sin (\zeta _{{hs}} )\cos (\zeta _{{hs}} )\kappa A_{{55}} \bar{U}^{{hs}} _{{3,\zeta _{{hs}} }} + \bar{U}^{{hs}} _{3} (A_{{11}} + 2A_{{12}} + A_{{22}} )\cos (\zeta _{{hs}} )^{2} \hfill \\ \quad - ((\kappa A_{{55}} + A_{{22}} + A_{{12}} )\bar{U}^{{hs}} _{1} + \bar{\Psi }_{\zeta }^{{hs}} ( - \kappa A_{{55}} R + \bar{A}_{{22}} + \bar{A}_{{12}} ))\sin (\zeta _{{hs}} )\cos (\zeta _{{hs}} ) \hfill \\ \quad + n(( - \kappa A_{{44}} R + \bar{A}_{{22}} + \bar{A}_{{12}} )\bar{\Psi }_{\theta }^{{hs}} + \bar{U}^{{hs}} _{2} (\kappa A_{{44}} + A_{{12}} + A_{{22}} ))\sin (\zeta _{{hs}} ) \hfill \\ \quad - \bar{U}^{{hs}} _{3} (\kappa n^{2} A_{{44}} + A_{{11}} + 2A_{{12}} + A_{{22}} )\} = I_{1} \omega _{n} ^{2} \bar{U}^{{hs}} _{3} \hfill \\ \end{gathered} $$

(83)

$$ \begin{gathered} \left( {\frac{1}{{R^{2} \sin (\zeta _{{hs}} )^{2} }}} \right)\{ ( - \cos (\zeta _{{hs}} )^{2} \bar{A}_{{11}} + \bar{A}_{{11}} )\bar{U}^{{hs}} _{{1,\zeta _{{hs}} \zeta _{{hs}} }} + ( - \cos (\zeta _{{hs}} )^{2} \bar{\bar{A}}_{{11}} + \bar{\bar{A}}_{{11}} )\bar{\Psi }_{{\zeta ,\zeta _{{hs}} \zeta _{{hs}} }}^{{hs}} \hfill \\ \quad + (\cos (\zeta _{{hs}} )^{2} - 1)(\kappa A_{{55}} R + \bar{\bar{A}}_{{11}} + \bar{\bar{A}}_{{12}} )\bar{U}^{{hs}} _{{3,\zeta _{{hs}} }} - n\sin (\zeta _{{hs}} )(\bar{\bar{A}}_{{12}} + \bar{\bar{A}}_{{66}} )\bar{\Psi }_{{\theta ,\zeta _{{hs}} }}^{{hs}} \hfill \\ \quad - n\sin (\zeta _{{hs}} )(\bar{\bar{A}}_{{12}} + \bar{\bar{A}}_{{66}} )\bar{U}^{{hs}} _{{2,\zeta _{{hs}} }} + \sin (\zeta _{{hs}} )\cos (\zeta _{{hs}} )\bar{A}_{{11}} \bar{U}^{{hs}} _{{1,\zeta _{{hs}} }} \hfill \\ \quad + \sin (\zeta _{{hs}} )\cos (\zeta _{{hs}} )\bar{\bar{A}}_{{11}} \bar{\Psi }_{{\zeta ,\zeta _{{hs}} }}^{{hs}} + ((\kappa A_{{55}} R + \bar{A}_{{12}} - \bar{A}_{{22}} )\bar{U}^{{hs}} _{1} \hfill \\ \quad + \bar{\Psi }_{\zeta }^{{hs}} ( - \kappa A_{{55}} R^{2} + \bar{\bar{A}}_{{12}} - \bar{\bar{A}}_{{22}} ))\cos (\zeta _{{hs}} )^{2} + (\bar{U}^{{hs}} _{3} (\bar{A}_{{11}} + \bar{A}_{{22}} )\sin (\zeta _{{hs}} ) \hfill \\ \quad + n((\bar{\bar{A}}_{{22}} + \bar{\bar{A}}_{{66}} )\bar{\Psi }_{\theta }^{{hs}} + \bar{U}^{{hs}} _{2} (\bar{A}_{{22}} + \bar{A}_{{66}} )))\cos (\zeta _{{hs}} ) + \hfill \\ \quad ( - n^{2} \bar{A}_{{66}} - \kappa A_{{55}} R - \bar{A}_{{12}} )\bar{U}^{{hs}} _{1} - \bar{\Psi }_{\zeta }^{{hs}} ( - \kappa A_{{55}} R^{2} + n^{2} \bar{\bar{A}}_{{66}} + \bar{\bar{A}}_{{12}} )\} \hfill \\ \;\;\; = I_{2} \omega _{n} ^{2} \bar{U}^{{hs}} _{1} + I_{3} \omega _{n} ^{2} \bar{\Psi }_{\zeta }^{{hs}} \hfill \\ \end{gathered} $$

(84)

$$ \begin{gathered} \left( {\frac{1}{{R^{2} \sin (\zeta _{{hs}} )^{2} }}} \right)\{ ( - \cos (\zeta _{{hs}} )^{2} \bar{\bar{A}}_{{66}} + \bar{\bar{A}}_{{66}} )\bar{\Psi }_{{\theta ,\zeta _{{hs}} \zeta _{{hs}} }}^{{hs}} + ( - \cos (\zeta _{{hs}} )^{2} \bar{A}_{{66}} + A_{{66}} )\bar{U}^{{hs}} _{{2,\zeta _{{hs}} \zeta _{{hs}} }} \hfill \\ \quad + n\sin (\zeta _{{hs}} )(\bar{A}_{{12}} + \bar{A}_{{66}} )\bar{U}^{{hs}} _{{1,\zeta _{{hs}} }} + n\sin (\zeta _{{hs}} )(\bar{\bar{A}}_{{12}} + \bar{\bar{A}}_{{66}} )\bar{\Psi }_{{\zeta ,\zeta _{{hs}} }}^{{hs}} \hfill \\ \quad + \sin (\zeta _{{hs}} )\cos (\vartheta )\bar{\bar{A}}_{{66}} \bar{\Psi }_{{\theta ,\zeta _{{hs}} }}^{{hs}} + \sin (\zeta _{{hs}} )\cos (\vartheta \zeta _{{hs}} )\bar{A}_{{66}} \bar{U}^{{hs}} _{{2,\zeta _{{hs}} }} \hfill \\ \quad + (( - \kappa A_{{44}} R^{2} - 2\bar{\bar{A}}_{{66}} )\bar{\Psi }_{\theta }^{{hs}} - \bar{U}^{{hs}} _{2} (\kappa A_{{44}} R + 2A_{{66}} ))\cos (\zeta _{{hs}} )^{2} \hfill \\ \quad + ((\bar{A}_{{22}} + \bar{A}_{{66}} )\bar{U}^{{hs}} _{1} + \bar{\Psi }_{\zeta }^{{hs}} (\bar{\bar{A}}_{{22}} + \bar{\bar{A}}_{{66}} ))n\cos (\zeta _{{hs}} ) - \hfill \\ \quad n\bar{U}^{{hs}} _{3} (\kappa A_{{44}} R - \bar{A}_{{12}} - \bar{A}_{{22}} )\sin (\zeta _{{hs}} ) + ( - \kappa A_{{44}} R^{2} - n^{2} \bar{\bar{A}}_{{22}} + \bar{\bar{A}}_{{66}} )\bar{\Psi }_{\theta }^{{hs}} \hfill \\ \quad - \bar{U}^{{hs}} _{2} (n^{2} A_{{22}} - \kappa A_{{44}} R - A_{{66}} )\} = I_{2} \omega _{n} ^{2} \bar{U}^{{hs}} _{2} + I_{3} \omega _{n} ^{2} \bar{\Psi }_{\theta }^{{hs}} \hfill \\ \end{gathered} $$

(85)