Mechanistic insights of neuronal calcium and IP₃ signaling system regulating ATP release during ischemia in progression of Alzheimer’s disease

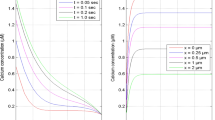
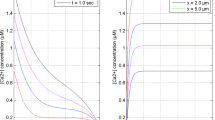
Abstract

The mechanisms of calcium ([Ca²⁺]) signaling in various human cells have been widely analyzed by scientists due to its crucial role in human organs like the heartbeat, muscle contractions, bone activity, brain functionality, etc. No study is reported for interdependent [Ca²⁺] and IP₃ mechanics regulating the release of ATP in neuron cells during Ischemia in Alzheimer’s disease advancement. In the present investigation, a finite element method (FEM) is framed to explore the interdependence of spatiotemporal [Ca²⁺] and IP₃ signaling mechanics and its role in ATP release during Ischemia as well as in the advancement of Alzheimer’s disorder in neuron cells. The results provide us insights of the mutual spatiotemporal impacts of [Ca²⁺] and IP₃ mechanics as well as their contributions to ATP release during Ischemia in neuron cells. The results obtained for the mechanics of interdependent systems differ significantly from the results of simple independent system mechanics and provide new information about the processes of the two systems. From this study, it is concluded that neuronal disorders cannot only be simply attributed to the disturbance caused directly in the processes of calcium signaling mechanics, but also to the disturbances caused in IP₃ regulation mechanisms impacting the calcium regulation in the neuron cell and ATP release.

Appendix: Model equations summary

The FEM is a numerical-cum-analytical technique for solving boundary value problems. It involves the discretization of the domain into a finite number of sub-regions and then the solution of each sub-region is obtained by substituting an interpolation function. The solution of each sub-region is assembled to obtain the solution for the whole region. Here, Galerkin’s approach is used to obtain the variational form. The unsteady state models of [Ca²⁺] and IP₃ diffusion concerning the one-dimensional case in neuron cells are constructed. The present problem is to get the solutions of Eq. (7) coupled with Eqs. (23, 25 and 26) concerning [Ca²⁺] and Eq. (17) coupled with Eqs. (24, 27 and 28) concerning IP₃. The length of the region is considered to be 5 µm, and divided into 40 elements from the source location to 5 µm. The initial and boundary conditions were constructed in light of biophysical conditions. The model equations for [Ca²⁺] and IP₃ dynamics are transformed into the variational form and Galerkin’s finite element procedure was utilized to get the solution. Conveniently, the notations ‘u’ and ‘v’ are used instead of [Ca²⁺] and IP₃ and e = 1, 2, 3,…, 40. Also, e depicts the e^th element and x_i and x_i+1 depict the initial and terminal nodes of the e^th element.

For [Ca²⁺] and IP₃ distribution, shape functions for each element is considered as,

$${\text{u}}^{{\text{(e)}}} {\text{ = q}}_{{1}}^{{\text{(e)}}} {\text{ + q}}_{{2}}^{{\text{(e)}}} {\text{ x}}$$

(32)

$${\text{v}}^{{\text{(e)}}} {\text{ = r}}_{{1}}^{{\text{(e)}}} {\text{ + r}}_{{2}}^{{\text{(e)}}} {\text{ x}}$$

(33)

$${\text{u}}^{{\text{(e)}}} {\text{ = S}}^{{\text{T}}} {\text{q}}^{{\text{(e)}}} {\text{, v}}^{{\text{(e)}}} {\text{ = S}}^{{\text{T}}} {\text{r}}^{{\text{(e)}}}$$

(34)

$$S^{{\text{T}}} { = [}\begin{array}{*{20}c} {1} & {\text{x}} \\ \end{array} {\text{], q}}^{{{\text{(e)}}^{{\text{T}}} }} { = [}\begin{array}{*{20}c} {{\text{q}}_{{1}}^{{\text{(e)}}} } & {{\text{q}}_{{2}}^{{\text{(e)}}} } \\ \end{array} {\text{], r}}^{{{\text{(e)}}^{{\text{T}}} }} { = [}\begin{array}{*{20}c} {{\text{r}}_{{1}}^{{\text{(e)}}} } & {{\text{r}}_{{2}}^{{\text{(e)}}} } \\ \end{array} {]}$$

(35)

Putting nodal conditions in Eq. (34),

$$\overline{u}^{(e)} \, = \,S^{(e)} q^{(e)} {, }\overline{v}^{(e)} \, = \,S^{(e)} r^{(e)} ,$$

(36)

where,

$$\overline{{\text{u}}} ^{{{\text{(e)}}}} = \left[ {\begin{array}{*{20}c} {{\text{u}}_{{\text{i}}} } \\ {{\text{u}}_{{\text{j}}} } \\ \end{array} } \right],{\mkern 1mu} \overline{{\text{v}}} ^{{{\text{(e)}}}} = \left[ {\begin{array}{*{20}c} {{\text{v}}_{{\text{i}}} } \\ {{\text{v}}_{{\text{j}}} } \\ \end{array} } \right]{\mkern 1mu} {\mkern 1mu} {\text{and}}{\mkern 1mu} {\mkern 1mu} S^{{{\text{(e)}}}} = \left[ {\begin{array}{*{20}c} 1 & {{\text{x}}_{{\text{i}}} } \\ 1 & {{\text{x}}_{{\text{j}}} } \\ \end{array} } \right]$$

(37)

From Eq. (36), we get

$$q^{(e)} \, = \,R^{(e)} \, \overline{u}^{(e)} , \, r^{(e)} \, = \,R^{(e)} \, \overline{v}^{(e)}$$

(38)

And

$${\text{R}}^{{\text{(e)}}} {\text{ = S}}^{{{\text{(e)}}^{{ - 1}} }}$$

(39)

Substituting ${\text{q}}^{{\text{(e)}}}$ and ${\text{r}}^{{\text{(e)}}}$ from Eq. (38) in (34),

$${\text{u}}^{{\text{(e)}}} {\text{ = S}}^{{\text{T}}} {\text{ R}}^{{\text{(e)}}} {\overline{\text{u}}}^{{\text{(e)}}} {\text{, v}}^{{\text{(e)}}} {\text{ = S}}^{{\text{T}}} {\text{ R}}^{{\text{(e)}}} {\overline{\text{v}}}^{{\text{(e)}}}$$

(40)

The integrals ${\text{I}}_{{1}}^{{\text{(e)}}}$ and ${\text{I}}_{{2}}^{{\text{(e)}}}$ represent discretized variational forms of Eqs. (7) and (17). These forms are given below;

$$I_{{1}}^{{\text{(e)}}} \, = \, I_{{{\text{a1}}}}^{{\text{(e)}}} - I_{{{\text{b1}}}}^{{\text{(e)}}} + \, I_{{{\text{c1}}}}^{{\text{(e)}}} - I_{{{\text{d1}}}}^{{\text{(e)}}} + \, I_{{{\text{e1}}}}^{{\text{(e)}}} + \, I_{{{\text{f1}}}}^{{\text{(e)}}} - I_{{{\text{g1}}}}^{{\text{(e)}}} + \, I_{{{\text{h1}}}}^{{\text{(e)}}} - I_{{{\text{i1}}}}^{{\text{(e)}}}$$

(41)

where

$${\text{I}}_{{{\text{a1}}}}^{{\text{(e)}}} { = }\int\limits_{{x_{i} }}^{{x_{j} }} {\left\{ {\left( {\frac{{\partial {\text{u}}^{{\text{(e)}}} }}{{\partial {\text{x}}}}} \right)^{2} } \right\}{\text{dx}}}$$

(42)

$${\text{I}}_{{{\text{b1}}}}^{(e)} = \frac{d}{dt}\int\limits_{{x_{i} }}^{{x_{j} }} {\left[ {\frac{{({\text{u}}^{(e)} )}}{{{\text{D}}_{{{\text{Ca}}}} }}} \right] \, dx}$$

(43)

$${\text{I}}_{{{\text{c1}}}}^{{\text{(e)}}} = \frac{{{\text{V}}_{{{\text{IPR}}}} }}{{{\text{D}}_{{{\text{ca}}}} {\text{F}}_{{\text{c}}} }}\int\limits_{{x_{i} }}^{{x_{j} }} {\left[ {\alpha_{1} {\text{u}}^{{\text{(e)}}} + \, \alpha_{2} {\text{v}}^{{\text{(e)}}} + \, \alpha_{3} } \right] \, dx}$$

(44)

$${\text{I}}_{{{\text{d1}}}}^{{\text{(e)}}} = \frac{{{\text{V}}_{{{\text{SERCA}}}} }}{{{\text{D}}_{{{\text{ca}}}} {\text{F}}_{{\text{c}}} }}\int\limits_{{x_{i} }}^{{x_{j} }} {\left[ {\beta_{1} {\text{u}}^{{\text{(e)}}} + \, \beta_{2} } \right] \, dx}$$

(45)

$${\text{I}}_{{{\text{e1}}}}^{{\text{(e)}}} \, = \,\frac{{{\text{V}}_{{{\text{LEAK}}}} }}{{{\text{D}}_{{{\text{ca}}}} {\text{F}}_{{\text{c}}} }}\int\limits_{{{\text{x}}_{{\text{i}}} }}^{{{\text{x}}_{{\text{j}}} }} {\left[ {{\text{[Ca}}^{{2 + }} {]}_{{{\text{ER}}}} \, - \,{\text{u}}^{{\text{(e)}}} } \right]{\text{dx}}}$$

(46)

$${\text{I}}_{{{\text{f1}}}}^{{\text{(e)}}} = \frac{{{\text{V}}_{{{\text{RyR}}}} {\text{P}}_{{0}} }}{{{\text{D}}_{{{\text{ca}}}} {\text{F}}_{{\text{c}}} }}\int\limits_{{{\text{x}}_{{\text{i}}} }}^{{{\text{x}}_{{\text{j}}} }} {\left[ {{\text{[Ca}}^{{2 + }} {]}_{{{\text{ER}}}} - \,{\text{u}}^{{\text{(e)}}} } \right]{\text{dx}}}$$

(47)

$${\text{I}}_{{{\text{g1}}}}^{{\text{(e)}}} \,{ = }\,\frac{{{\text{K}}^{ + } }}{{{\text{D}}_{{{\text{ca}}}} }}\int\limits_{{{\text{x}}_{{\text{i}}} }}^{{{\text{x}}_{{\text{j}}} }} {\left[ {{\text{u}}^{{\text{(e)}}} {\text{ - [Ca}}^{{2 + }} ]_{\infty } } \right]{\text{ dx}}}$$

(48)

$$I_{{{\text{h1}}}}^{{\text{(e)}}} \,{ = }\,\frac{{1}}{{{\text{D}}_{{{\text{ca}}}} }}\int\limits_{{{\text{x}}_{{\text{i}}} }}^{{{\text{x}}_{{\text{j}}} }} {\left[ {{\uptheta }_{{1}} {\text{u}}^{{\text{(e)}}} - {\uptheta }_{{2}} } \right]{\text{ dx}}}$$

(49)

$${\text{I}}_{{{\text{i1}}}}^{{\text{(e)}}} {\text{ = f}}^{{\text{(e)}}} \left( {\frac{{{\upsigma }_{{{\text{Ca}}}} }}{{{\text{D}}_{{{\text{ca}}}} }} - \frac{{{\upsigma }_{{{\text{NCX}}}} }}{{{\text{D}}_{{{\text{ca}}}} }}} \right)_{{\text{ x = 0}}}$$

(50)

$${\text{I}}_{{2}}^{{\text{(e)}}} \, = \,{\text{I}}_{{{\text{a2}}}}^{{\text{(e)}}} - {\text{I}}_{{{\text{b2}}}}^{{\text{(e)}}} \, + {\text{ I}}_{{{\text{c2}}}}^{{\text{(e)}}} - {\text{I}}_{{{\text{d2}}}}^{{\text{(e)}}}$$

(51)

$${\text{I}}_{{{\text{a2}}}}^{{\text{(e)}}} \,{ = }\,\int\limits_{{x_{i} }}^{{x_{j} }} {\left\{ {\left( {\frac{{\partial {\text{v}}^{{\text{(e)}}} }}{{\partial {\text{x}}}}} \right)^{2} } \right\}{\text{ dx}}}$$

(52)

$${\text{I}}_{{{\text{b2}}}}^{{\text{(e)}}} = \frac{d}{dt}\int\limits_{{x_{i} }}^{{x_{j} }} {\left[ {\frac{{{\text{v}}^{{\text{(e)}}} }}{{{\text{D}}_{{\text{i}}} }}} \right]\,dx}$$

(53)

$${\text{I}}_{{{\text{c2}}}}^{{\text{(e)}}} = \frac{{{\text{V}}_{{{\text{PROD}}}} }}{{{\text{D}}_{{\text{i}}} {\text{F}}_{{\text{c}}} }}\int\limits_{{x_{i} }}^{{x_{j} }} {\left[ {\mu_{1} {\text{u}}^{{\text{(e)}}} + \, \mu_{2} } \right] \, dx}$$

(54)

$$I_{d2}^{{\text{(e)}}} = \frac{\lambda }{{{\text{F}}_{{\text{c}}} {\text{D}}_{i} }}\int\limits_{{x_{i} }}^{{x_{j} }} {\left[ {\delta_{1} {\text{u}}^{{\text{(e)}}} \, + \, \delta_{2} {\text{v}}^{{\text{(e)}}} \, + \, \delta_{3} )} \right] \, dx}$$

(55)

The various coefficients $\alpha _{1} ,{\text{ }}\alpha _{2} ,{\text{ }}\alpha _{3} ,{\text{ }}\beta _{1} ,{\text{ }}\beta _{2} ,{\text{ }}\theta _{1} ,{\text{ }}\theta _{2} ,{\text{ }}\mu _{1} ,{\text{ }}\mu _{2} ,{\text{ }}\delta _{1} ,{\text{ }}\delta _{2} ,{\text{ and}},{\text{ }}\delta _{3}$ are determined by the linearization procedure for nonlinear terms of [Ca²⁺] and IP₃ distributions. The boundary conditions are incorporated in the analyzed equations to provide the system of equations as follows.

$$\frac{{dI_{1} }}{{d\overline{u}^{{\text{(e)}}} }} = \sum\limits_{e = 1}^{N} {\overline{Q}^{{\text{(e)}}} } \frac{{dI_{{1}}^{{\text{(e)}}} }}{{d\overline{u}^{{\text{(e)}}} }}\overline{Q}^{{{\text{(e)}}^{{\text{T}}} }} = 0$$

(56)

$$\frac{{dI_{2} }}{{d\overline{v}^{{\text{(e)}}} }} = \sum\limits_{e = 1}^{N} {\overline{Q}^{{\text{(e)}}} } \frac{{dI_{2}^{{\text{(e)}}} }}{{d\overline{v}^{{\text{(e)}}} }}\overline{Q}^{{{\text{(e)}}^{{\text{T}}} }} = 0$$

(57)

where,

$$\overline{Q}^{{\text{(e)}}} = \left[ {\begin{array}{*{20}c} {\begin{array}{*{20}c} 0 \\ {\begin{array}{*{20}c} . \\ {\begin{array}{*{20}c} 0 \\ 1 \\ {\begin{array}{*{20}c} {\begin{array}{*{20}c} 0 \\ 0 \\ \end{array} } \\ {\begin{array}{*{20}c} . \\ 0 \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } & {\begin{array}{*{20}c} 0 \\ {\begin{array}{*{20}c} . \\ {\begin{array}{*{20}c} 0 \\ 0 \\ {\begin{array}{*{20}c} {\begin{array}{*{20}c} 1 \\ 0 \\ \end{array} } \\ {\begin{array}{*{20}c} . \\ 0 \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \right]\,\,{\text{and}}\,\,\overline{u}\, = \,\left[ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\begin{array}{*{20}c} {u_{1} } \\ {u_{2} } \\ \end{array} } \\ {u_{3} } \\ \end{array} } \\ {\begin{array}{*{20}c} . \\ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\begin{array}{*{20}c} . \\ {u_{39} } \\ \end{array} } \\ {u_{40} } \\ \end{array} } \\ {u_{41} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \right],\,\,\overline{v}\, = \,\left[ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\begin{array}{*{20}c} {v_{1} } \\ {v_{2} } \\ \end{array} } \\ {v_{3} } \\ \end{array} } \\ {\begin{array}{*{20}c} . \\ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\begin{array}{*{20}c} . \\ {v_{39} } \\ \end{array} } \\ {v_{40} } \\ \end{array} } \\ {v_{41} } \\ \end{array} } \\ \end{array} } \\ \end{array} } \right]$$

(58)

$$\frac{{dI_{{1}}^{{\text{(e)}}} }}{{d\overline{u}^{{\text{(e)}}} }} = \frac{{dI_{{{\text{a1}}}}^{{\text{(e)}}} }}{{d\overline{u}^{{\text{(e)}}} }} + \frac{d}{dt}\frac{{dI_{{{\text{b1}}}}^{{\text{(e)}}} }}{{d\overline{u}^{{\text{(e)}}} }} + \frac{{dI_{{{\text{c1}}}}^{{\text{(e)}}} }}{{d\overline{u}^{{\text{(e)}}} }} - \frac{{dI_{{d{1}}}^{{\text{(e)}}} }}{{d\overline{u}^{{\text{(e)}}} }} + \frac{{dI_{{{\text{e1}}}}^{{\text{(e)}}} }}{{d\overline{u}^{{\text{(e)}}} }} + \frac{{dI_{{{\text{f1}}}}^{{\text{(e)}}} }}{{d\overline{u}^{{\text{(e)}}} }} - \frac{{dI_{{{\text{g1}}}}^{{\text{(e)}}} }}{{d\overline{u}^{{\text{(e)}}} }} + \frac{{dI_{{{\text{h1}}}}^{{\text{(e)}}} }}{{d\overline{u}^{{\text{(e)}}} }} - \frac{{dI_{{{\text{i1}}}}^{{\text{(e)}}} }}{{d\overline{u}^{{\text{(e)}}} }}$$

(59)

$$\frac{{dI_{{2}}^{{\text{(e)}}} }}{{d\overline{v}^{{\text{(e)}}} }} = \frac{{dI_{{{\text{a2}}}}^{{\text{(e)}}} }}{{d\overline{v}^{{\text{(e)}}} }} + \frac{d}{dt}\frac{{dI_{{{\text{b2}}}}^{{\text{(e)}}} }}{{d\overline{v}^{{\text{(e)}}} }} + \frac{{dI_{{{\text{c2}}}}^{{\text{(e)}}} }}{{d\overline{v}^{{\text{(e)}}} }} - \frac{{dI_{{{\text{d2}}}}^{{\text{(e)}}} }}{{d\overline{v}^{{\text{(e)}}} }}$$

(60)

$$\left[ {\text{A}} \right]_{82 \times 82} \left[ {\begin{array}{*{20}c} {\left[ {\frac{{\partial \overline{u}}}{\partial t}} \right]_{41 \times 1} } \\ {\left[ {\frac{{\partial \overline{v}}}{\partial t}} \right]_{41 \times 1} } \\ \end{array} } \right] + \left[ {\text{B}} \right]_{82 \times 82} \left[ {\begin{array}{*{20}c} {\left[ {\overline{u}} \right]_{41 \times 1} } \\ {\left[ {\overline{v}} \right]_{41 \times 1} } \\ \end{array} } \right] = [{\text{F}}]_{82 \times 1}$$

(61)

Here, A and B are system matrices with F as system vectors. The numerically stable Crank–Nicolson technique is utilized in FEM for time derivatives solution.