Main

Spin currents have recently been measured in graphene using ferromagnetic contacts, an advance that shows promise for room-temperature graphene spintronics8,9,10. Unfortunately, many of the other tools that have been developed for studying spin-dependent phenomena in solid-state nanostructures (optical excitation, spin-resolved Coulomb blockade, and so on) have proven difficult to implement in graphene. The Zeeman-split conductance fluctuations that are the subject of this letter provide a way to detect spin-polarized currents with a straightforward electrical measurement. This is the first report, to our knowledge, of visible spin-splitting of conductance fluctuations in any material.

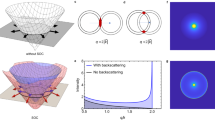
Flakes of graphene were deposited using well-established mechanical exfoliation techniques1 onto silicon wafers with a ∼300 nm surface oxide, then contacted with Cr/Au leads (Fig. 1a). The two flakes used for this experiment (hereafter referred to as A and B) were selected optically and confirmed to be single layer by quantum Hall measurements (see Supplementary Information) showing the characteristic (n+1/2) Landau quantization1. The measurements described below were carried out in a dilution refrigerator with a base temperature of 20 mK. The electron temperature was 40 mK, based on calibration using the Kondo effect in GaAs quantum dots, but the conductance fluctuations presented here were insensitive to temperature below 100 mK. The data presented here are from two-terminal conductance measurements in flake A, with a contact spacing of 3 μm (the phase coherence length was estimated to equal or exceed sample dimensions). Similar spin-resolved conductance fluctuations were observed in flake B, where the availability of multiple contacts enabled four-terminal measurements to eliminate contact effects (see Supplementary Information). A two-axis magnet provided fields up to B∥=12 T in the plane of the graphene and fine control of the out-of-plane component up to (Fig. 1a).

Figure 1: Zeeman effect in graphene.
figure 1

a, Schematic of a single-layer graphene device, showing the orientation of the magnetic fields B∥ and . b, Two-terminal conductance, G, measured as a function of backgate voltage VG at 20 mK, showing the Dirac conductance minimum (). Inset: A sample of conductance fluctuations over a narrow range of gate voltage. c,d, Diagrams of the spin-splitting effect: a simulated conductance trace at zero field c; two offset contributions from spin-up and spin-down at finite field add together in the total conductance d, giving spin-split features. e,f, Two examples of conductance fluctuations spin-splitting in an in-plane field, taken over two ranges of gate voltage (). For both images, a smooth background was subtracted to highlight fluctuations. The offset (indicated by the dashed lines, as guides to the eye) owing to spin is larger around −6 V compared with −2 V because the density of states increases with density. The dashed lines correspond to densities of states of (e) 12×1012 and (f) 7×1012 cm−2 eV−1.

Full size image

Carrier density n was controlled capacitively with a voltage VG on the backgate, inducing a density n=α VG in the graphene sheet; the proportionality constants of each flake were extracted from quantum Hall data. A Dirac resistance peak was observed when VG tuned the carrier density to the charge neutrality point. The conductance, G, in the two-wire measurement was calculated after taking into account an estimated contact resistance of 3.2 kΩ. Conductance fluctuations, δG, were visible superimposed on the Dirac line shape (Fig. 1b), and were extracted by subtracting an ensemble-averaged background (see Supplementary Information). The mean free path ranged from 100 to 200 nm, much smaller than sample dimensions, so transport in the flake was diffusive.

Graphene, like any metallic or semiconducting system, shows ‘universal’ conductance fluctuations when transport is phase coherent11,12,13,14. These aperiodic fluctuations arise from quantum interference between all possible paths that charge carriers take as they traverse the device. The interference pattern is randomized by small changes in the relative phase between trajectories, which depends on the Aharonov–Bohm magnetic flux through the sample as well as on the Fermi wave vector11. The conductance includes contributions from spin-up and spin-down carriers: G=G↑+G↓. In the absence of spin–orbit and many-body interactions, G↑/↓ depends on the spin only through the Fermi wave vector, and therefore the density, of the respective carrier population.

Spin-up and spin-down carriers have the same density at zero field, n↑=n↓. In a simple picture, then, zero-field conductance fluctuations include identical contributions from both populations (Fig. 1c). Applying a magnetic field partially polarizes the graphene—it induces a difference between spin-up and spin-down carrier densities. The total density n=n↑+n↓=α VG is set by the backgate voltage; the difference in the densities n↑−n↓=(1/2)(dn/dε)g μBB is set by the magnetic field, where dn/dε is the density of states and g μBB is the Zeeman energy. Together these yield

As a result, spin-up and spin-down conductance contributions at finite field are offset in gate voltage by VGoffset, leading to Zeeman splitting of interference features in a gate-voltage trace G(VG) (Fig. 1d).

Zeeman splitting of graphene conductance fluctuations can be seen in Fig. 1e,f. The ‘V’ shapes in the data correspond to spin-resolved conductance: left-moving (right-moving) arms reflect interference for a particular density of spin-down (spin-up) carriers. Although conductance fluctuations have been observed for several decades in a wide variety of materials11,13, a direct measurement of their Zeeman splitting has never before been reported. Several factors must conspire to make this effect visible. A low density of states enhances the visibility of conductance fluctuations and reduces the gate-voltage offset between spin-split features; a small spin–orbit interaction is required for the approximation of two independent spin populations to be valid; atomic-scale flatness and precise alignment of the in-plane field reduce the effective Aharonov–Bohm flux entrained by an in-plane magnetic field. The first three factors are naturally present in graphene; the fourth was facilitated by a two-axis magnet.

A statistical analysis of the spin-split offset at particular values of B∥ was obtained using an ensemble of traces, δG(VG), collected for a range of out-of-plane fields, (≪B∥). The offset is barely visible in the raw conductance data (Fig. 2a), but can be seen more clearly by computing an autocorrelation C[δG](ΔVG)=〈δG(VG)δG(VG+ΔVG)〉. Side peaks in the autocorrelation at ΔVG=±VGoffset (Fig. 2b) reflect similar features offset in the conductance traces (Fig. 2a) owing to the difference in spin-up and spin-down carrier densities.

Figure 2: Autocorrelation extraction of spin-splitting.
figure 2

a, Features are doubled in an image of conductance fluctuations at B∥=8 T. b, Autocorrelations of the windows indicated in a show that the spin-split offset (the location of the autocorrelation side peak) changes from VGoffset=0.22 V at VG=−16 V, to VGoffset=0.19 V at VG=−12 V. The autocorrelation function is symmetric, so side peaks appear at ±VGoffset.

Full size image

The side peaks shifted outward with increasing B∥ (Fig. 3a), at the same time as the height of the central peak (C[δG](0), the variance of the fluctuations) dropped by a factor of two (Fig. 3b). The drop in C[δG](0) reflects a suppression of conductance fluctuations when the Zeeman energy, g μBB, exceeds energy broadening owing to temperature or dephasing. This effect has been used to characterize spin degeneracy in other systems15,16,17, where splitting could not be observed directly. The suppression factor of ∼2 indicates that spin degeneracy was intact at zero field, after taking into account broken time-reversal symmetry owing to the small out-of-plane field. The shift of the side-peak location, VGoffset, with B∥ was expected from equation (1)—a statistical confirmation of the linear spin-splitting seen in Fig. 1e,f.

Figure 3: Magnetic-field- and gate-dependence of spin splitting.
figure 3

a, Autocorrelations, C[δG](ΔVG,B∥), of fluctuations in the range VG=−5…−4.4 V, averaged over an ensemble of traces for , demonstrate the in-plane field dependence of side-peak location, VGoffset. The dashed red line is fitted to VGoffset, and corresponds to a density of states 1.04×1013 cm−2 eV−1. b, Variance C[δG](ΔVG=0,B∥) (filled squares) and autocorrelation side-peak height C[δG](ΔVG=VGoffset,B∥) (open squares) from a. The variance drops to the expected 50% of its zero-field value, but the side peaks fall below the expected 25%. The out-of-plane field was large enough to break time-reversal symmetry even at B∥=0. c, Autocorrelations computed for a sliding 0.75-V-wide window in VG (see Fig. 2), and averaged over an ensemble of traces for , show how the density of states depends on density. d, The Fermi velocity vF extracted from side-peak locations, VGoffset, in c is enhanced near the Dirac point. The error bars are primarily due to uncertainty in the charge neutrality point, V0=1.0±0.3 V.

Full size image

The density of states can be extracted directly from VGoffset at a given field using g=2 for graphene (see Supplementary Information) and the value α=8.05±0.05×1010cm−2 V−1 determined from quantum Hall measurements (equation (1)). VGoffset(VG) was recorded over the full gate-voltage range by computing the autocorrelation C[δG](ΔVG) within a sliding window in VG (Fig. 3c). The resulting square-root line shape can be compared to dn/dε expected from graphene’s dispersion relation: , with a Fermi velocity vF that would be independent of density for an ideal Dirac band structure.

As VGoffset can be determined very accurately from C[δG](ΔVG), spin-split fluctuations provide an accurate measure of vF. Experimental error bars were typically one or two per cent of the measured value (Fig. 3d), dominated by uncertainty in the charge neutrality point, V0. Both flakes showed vF>9×105 m s−1 away from the Dirac peak, with vF>1.05×106 throughout most of the gate-voltage range for flake A (Fig. 3d) and for the electron-doped gate-voltage range of flake B (see Supplementary Information). These values are similar to those reported elsewhere18,19,20 but considerably larger than the expected vF≈0.8×106 m s−1 from non-interacting band-structure calculations4. Enhancements in vF have been predicted due to many-body effects21,22,23. On the other hand, differences in the Fermi speeds for flakes A and B suggest that the apparent Fermi speed in realistic graphene samples also depends strongly on disorder and other sample details. Larger values of vF are observed in Fig. 3d at lower densities, similar to a report of infrared spectroscopy measurements19, but in flake B the trend was reversed, providing further evidence for sample-to-sample variations in Fermi speed.

To observe V shapes such as those shown in Fig. 1e,f, interference features must shift, but not otherwise change, as a function of in-plane field. In other words, the primary influence of the magnetic field on the interference must be through its effect on the densities of spin-up and spin-down carriers, rather than a change in Aharonov–Bohm flux. Fields of a tesla or more were required to differentiate the two carrier densities, but the Aharonov–Bohm flux introduced by a few millitesla in was sufficient to alter the observed interference pattern. The drastically different field scales for spin and Aharonov–Bohm effects demanded precise alignment (within 0.05∘) of the B∥ axis to the plane of the graphene flake. The alignment of B∥ was monitored using weak localization, the coherent enhancement of backscattering associated with time-reversal symmetry at (ref. 11). Slight mis-alignment led to a shift in the location of the weak-localization conductance dip as B∥ was raised, and was corrected by offsetting .

In addition to shifting, the weak-localization dip also decreased in magnitude with B∥, by a factor of two at 1 T and below detectable levels above 4 T (Fig. 4a,b). The complete collapse of the weak-localization dip, over a field range where the variance of conductance fluctuations decreases only by a factor of two, implies that time-reversal symmetry is broken even by an in-plane field24,25. The disappearance of symmetry in at finite B∥ (Fig. 4c,d) provided further evidence of time-reversal symmetry breaking24. Similar phenomena have been observed in semiconductor two-dimensional electron gases, and have been associated with finite thickness and nanometre-scale undulations in the two-dimensional electron gas25, allowing B∥ to thread an Aharonov–Bohm flux through the conductor. The B∥-scale of weak-localization collapse in graphene corresponds to an effective thickness of ∼1 nm, in agreement with previous measurements of the intrinsic ripple size in graphene sheets26,27.

Figure 4: Loss of weak localization and symmetry due to in-plane field.
figure 4

a, Averaging out the conductance fluctuations over a range VG=−5…−3 V, a weak localization dip in conductance is present at B=0 but disappears for increasing B∥ or . This indicates a breaking of time-reversal symmetry from either component, but at very different field scales. Curves at different B∥ are vertically offset to align (see Supplementary Information). b, Negative magnetoconductance for is unaffected by B∥. The rectangle encloses the range from a. c,d, Conductance is symmetric in at B∥=0 (c) but not at B∥=2 T (d). These data provide further evidence of time-reversal symmetry breaking by the in-plane field, excluding (for example) that the disappearance of weak localization in a and b is due to decoherence.

Full size image

The ability to distinguish conductance fluctuations associated with spin-up transport from those associated with spin-down transport, using the magnetic-field dependence of their position in gate voltage, may allow the development of interference-based spin filters in graphene. The maximum spin polarization of current injected through such a device is set by the ratio of conductance fluctuation amplitude to the conductance itself. Although this ratio was typically <10% in the devices measured here, it could be increased by using constrictions or other device geometries to decrease the overall conductance, because the amplitude of conductance fluctuations remains large as long as the device size does not exceed the phase coherence length.