Skip to main content
SpringerLink
Log in

Numerical study of integer-order hyperbolic telegraph model arising in physical and related sciences

  • Regular Article
  • Published:
The European Physical Journal Plus Aims and scope Submit manuscript

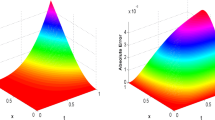
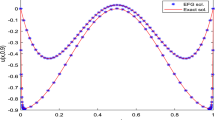
Abstract

More recently, it is discovered in the field of applied sciences and engineering that the telegraph equation is better suited to model reaction-diffusion than the ordinary diffusion equation. In this article, the second-order hyperbolic telegraph equations are analyzed numerically by means of an efficient local differential quadrature method utilizing the radial basis functions. The explicit time integration technique is used to semi-discretize the model in the time direction, while the space derivatives are discretized by the proposed meshless procedure. To test the accuracy and capabilities of the method, five test problems are considered utilizing both rectangular and non-rectangular domains, which show that the proposed scheme solutions are converging extremely quick in comparison with the different existing numerical techniques in the recent literature.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

References

  1. C. Rong-Jun, G. Hong-Xia, Element-free Galerkin (EFG) method for a kind of two-dimensional linear hyperbolic equation. Chin. Phys. B 18(10), 4059 (2009)

    ADS  Google Scholar 

  2. I. Komashynska, M. Al-Smadi, A. Ateiwi, S. Al-Obaidy, Approximate analytical solution by residual power series method for system of fredholm integral equations. Appl. Math. Inf. Sci. 10, 1–11 (2016)

    MathSciNet  Google Scholar 

  3. H. Ahmad, A.R. Seadawy, T.A. Khan, Study on numerical solution of dispersive water wave phenomena by using a reliable modification of variational iteration algorithm. Math. Comput. Simul. 177, 13–23 (2020)

    MathSciNet  Google Scholar 

  4. M.A. Aal, N. Abu-Darwish, O.A. Arqub, M. Al-Smadi, S. Momani, Analytical solutions of fuzzy fractional boundary value problem of order 2\(\alpha \) by using RKHS algorithm. Appl. Math. Inf. Sci. 13(4), 523–533 (2019)

    MathSciNet  Google Scholar 

  5. R. Mohanty, M. Jain, An unconditionally stable alternating direction implicit scheme for the two space dimensional linear hyperbolic equation. Numer. Methods Partial Differ. Equ. Int. J. 17(6), 684–688 (2001)

    MathSciNet  MATH  Google Scholar 

  6. R. Mohanty, New unconditionally stable difference schemes for the solution of multi-dimensional telegraphic equations. Int. J. Comput. Math. 86(12), 2061–2071 (2009)

    MathSciNet  MATH  Google Scholar 

  7. B. Bülbül, M. Sezer, A Taylor matrix method for the solution of a two-dimensional linear hyperbolic equation. Appl. Math. Lett. 24(10), 1716–1720 (2011)

    MathSciNet  MATH  Google Scholar 

  8. M. Dehghan, S. Yousefi, A. Lotfi, The use of He’s variational iteration method for solving the telegraph and fractional telegraph equations. Int. J. Numer. Methods Biomed. Eng. 27(2), 219–231 (2011)

    MathSciNet  MATH  Google Scholar 

  9. R. Jiwari, S. Pandit, R. Mittal, A differential quadrature algorithm to solve the two dimensional linear hyperbolic telegraph equation with Dirichlet and Neumann boundary conditions. Appl. Math. Comput. 218(13), 7279–7294 (2012)

    MathSciNet  MATH  Google Scholar 

  10. R. Mittal, R. Bhatia, A numerical study of two dimensional hyperbolic telegraph equation by modified B-spline differential quadrature method. Appl. Math. Comput. 244, 976–997 (2014)

    MathSciNet  MATH  Google Scholar 

  11. R.M. Hafez, Numerical solution of linear and nonlinear hyperbolic telegraph type equations with variable coefficients using shifted jacobi collocation method. Comput. Appl. Math. 37(4), 5253–5273 (2018)

    MathSciNet  MATH  Google Scholar 

  12. H. Ahmad, Variational iteration method with an auxiliary parameter for solving telegraph equations. J. Nonlinear Anal. Appl. 2(2018), 223–232 (2018)

    Google Scholar 

  13. A.E. Harrak, A. Bergam, Preserving finite-volume schemes for two-time reaction-diffusion model. Appl. Math. Inf. Sci. 14, 41–50 (2020)

    MathSciNet  Google Scholar 

  14. H. Ahmad, A.R. Seadawy, T.A. Khan, P. Thounthong, Analytic approximate solutions for some nonlinear parabolic dynamical wave equations. J. Taibah Univ. Sci. 14(1), 346–358 (2020)

    Google Scholar 

  15. M. Dehghan, A. Shokri, A meshless method for numerical solution of a linear hyperbolic equation with variable coefficients in two space dimensions. Numer. Methods Partial Differ. Equ. Int. J. 25(2), 494–506 (2009)

    MathSciNet  MATH  Google Scholar 

  16. M. Dehghan, A. Ghesmati, Combination of meshless local weak and strong (MLWS) forms to solve the two dimensional hyperbolic telegraph equation. Eng. Anal. Bound. Elem. 34(4), 324–336 (2010)

    MathSciNet  MATH  Google Scholar 

  17. M. Dehghan, R. Salehi, A method based on meshless approach for the numerical solution of the two-space dimensional hyperbolic telegraph equation. Math. Methods Appl. Sci. 35(10), 1220–1233 (2012)

    ADS  MathSciNet  MATH  Google Scholar 

  18. S. Abbasbandy, H.R. Ghehsareh, I. Hashim, A. Alsaedi, A comparison study of meshfree techniques for solving the two-dimensional linear hyperbolic telegraph equation. Eng. Anal. Bound. Elem. 47, 10–20 (2014)

    MathSciNet  MATH  Google Scholar 

  19. D. Rostamy, M. Emamjome, S. Abbasbandy, A meshless technique based on the pseudospectral radial basis functions method for solving the two-dimensional hyperbolic telegraph equation. Eur. Phys. J. Plus 132(6), 263 (2017)

    Google Scholar 

  20. J. Lin, F. Chen, Y. Zhang, J. Lu, An accurate meshless collocation technique for solving two-dimensional hyperbolic telegraph equations in arbitrary domains. Eng. Anal. Bound. Elem. 108, 372–384 (2019)

    MathSciNet  MATH  Google Scholar 

  21. M. Aslefallah, D. Rostamy, Application of the singular boundary method to the two-dimensional telegraph equation on arbitrary domains. J. Eng. Math. 118(1), 1–14 (2019)

    MathSciNet  MATH  Google Scholar 

  22. Y. Zhou, W. Qu, Y. Gu, H. Gao, A hybrid meshless method for the solution of the second order hyperbolic telegraph equation in two space dimensions. Eng. Anal. Bound. Elem. 115, 21–27 (2020)

    MathSciNet  MATH  Google Scholar 

  23. S. Reutskiy, Y. Zhang, J. Lin, H. Sun, Novel numerical method based on cubic B-splines for a class of nonlinear generalized telegraph equations in irregular domains. Alex. Eng. J. 59(1), 77–90 (2020)

    Google Scholar 

  24. I. Ahmad, H. Ahmad, P. Thounthong, Y.-M. Chu, C. Cesarano, Solution of multi-term time-fractional PDE models arising in mathematical biology and physics by local meshless method. Symmetry 12(7), 1195 (2020)

    Google Scholar 

  25. M.H. Srivastava, H. Ahmad, I. Ahmad, P. Thounthong, N. M. Khan, Numerical simulation of three-dimensional fractional-order convection-diffusion PDEs by a local meshless method. Therm. Sci. (00), 210–210 (2020)

  26. I. Ahmad, M.N. Khan, M. Inc, H. Ahmad, K. Nisar, Numerical simulation of simulate an anomalous solute transport model via local meshless method. Alex. Eng. J. 59, 2827–2838 (2020)

    Google Scholar 

  27. R.L. Hardy, Multiquadric equations of topography and other irregular surfaces. J. Geophys. Res. 76(8), 1905–1915 (1971)

    ADS  Google Scholar 

  28. R. Franke, Scattered data interpolation: tests of some methods. Math. Comput. 38(157), 181–200 (1982)

    MathSciNet  MATH  Google Scholar 

  29. E.J. Kansa, Multiquadrics—a scattered data approximation scheme with applications to computational fluid-dynamics-I surface approximations and partial derivative estimates. Comput. Math. Appl. 19(8–9), 127–145 (1990)

    MathSciNet  MATH  Google Scholar 

  30. E.J. Kansa, Multiquadrics—a scattered data approximation scheme with applications to computational fluid-dynamics-II solutions to parabolic, hyperbolic and elliptic partial differential equations. Comput. Math. Appl. 19(8–9), 147–161 (1990)

    MathSciNet  MATH  Google Scholar 

  31. C. Franke, R. Schaback, Convergence order estimates of meshless collocation methods using radial basis functions. Adv. Comput. Math. 8(4), 381–399 (1998)

    MathSciNet  MATH  Google Scholar 

  32. W. Madych, S. Nelson, Multivariate interpolation and conditionally positive definite functions, II. Math. Comput. 54(189), 211–230 (1990)

    ADS  MathSciNet  MATH  Google Scholar 

  33. G.E. Fasshauer, Meshfree Approximation Methods with MATLAB, vol. 6 (World Scientific, Singapore, 2007)

    MATH  Google Scholar 

  34. H. Wendland, Scattered Data Approximation, vol. 17 (Cambridge University Press, Cambridge, 2004)

    MATH  Google Scholar 

  35. G.E. Fasshauer, Newton iteration with multiquadrics for the solution of nonlinear PDEs. Comput. Math. Appl. 43(3–5), 423–438 (2002)

    MathSciNet  MATH  Google Scholar 

  36. G.E. Fasshauer, J.G. Zhang, On choosing “optimal” shape parameters for RBF approximation. Numer. Algorithms 45(1–4), 345–368 (2007)

    ADS  MathSciNet  MATH  Google Scholar 

  37. R. Cavoretto, A. De Rossi, M. Mukhametzhanov, Y.D. Sergeyev, On the search of the shape parameter in radial basis functions using univariate global optimization methods. J. Glob. Optim. 1–23 (2019)

  38. S. Rippa, An algorithm for selecting a good value for the parameter c in radial basis function interpolation. Adv. Comput. Math. 11(2–3), 193–210 (1999)

    MathSciNet  MATH  Google Scholar 

  39. M. Uddin, On the selection of a good value of shape parameter in solving time-dependent partial differential equations using RBF approximation method. Appl. Math. Model. 38(1), 135–144 (2014)

    MathSciNet  MATH  Google Scholar 

  40. R. Cavoretto, A. De Rossi, An adaptive LOOCV-based refinement scheme for RBF collocation methods over irregular domains. Appl. Math. Lett. 103, 106178 (2020)

    MathSciNet  MATH  Google Scholar 

  41. R. Cavoretto, A. De Rossi, A two-stage adaptive scheme based on RBF collocation for solving elliptic PDEs. Comput. Math. Appl. (2020)

  42. J. Biazar, M. Hosami, An interval for the shape parameter in radial basis function approximation. Appl. Math. Comput. 315, 131–149 (2017)

    MathSciNet  MATH  Google Scholar 

  43. M. Nawaz, I. Ahmad, H. Ahmad, A radial basis function collocation method for space-dependent inverse heat problems. J. Appl. Comput. Mech. (2020)

  44. I. Ahmad, S. Zaman, Local meshless differential quadrature collocation method for time-fractional PDEs. Discrete Contin. Dyn. Syst. 13(10), 2641–2654 (2020)

    MathSciNet  Google Scholar 

  45. I. Ahmad, M. Ahsan, Z. Din, M. Ahmad, P. Kumam, An efficient local formulation for time-dependent PDEs. Mathematics 7, 216 (2019)

    Google Scholar 

  46. M.N. Khan, I. Hussain, I. Ahmad, H. Ahmad, A local meshless method for the numerical solution of space-dependent inverse heat problems. Math. Methods Appl. Sci. (2020)

  47. I. Ahmad, M. Ahsan, I. Hussain, P. Kumam, W. Kumam, Numerical simulation of PDEs by local meshless differential quadrature collocation method. Symmetry 11(3), 394 (2019)

    MATH  Google Scholar 

  48. I. Ahmad, A.Q.M. Khaliq, Local RBF method for multi-dimensional partial differential equations. Comput. Math. Appl. 74, 292–324 (2017)

    MathSciNet  MATH  Google Scholar 

  49. C. Shu, Differential Quadrature and Its Application in Engineering (Springer, London, 2000)

    MATH  Google Scholar 

  50. C. Shu, H. Ding, K. Yeo, Local radial basis function-based differential quadrature method and its application to solve two-dimensional incompressible Navier–Stokes equations. Comput. Methods Appl. Mech. Eng. 192, 941–954 (2003)

    ADS  MATH  Google Scholar 

  51. B.K. Singh, P. Kumar, An algorithm based on a new DQM with modified extended cubic B-splines for numerical study of two dimensional hyperbolic telegraph equation. Alex. Eng. J. 57(1), 175–191 (2018)

    Google Scholar 

  52. R. Siraj-ul-islam, B. Šarler et al., Local radial basis function collocation method along with explicit time stepping for hyperbolic partial differential equations. Appl. Numer. Math. 67, 136–151 (2013)

    MathSciNet  MATH  Google Scholar 

  53. M. Rahman, M. Akhtar, A. Shaikh, A. Bhunia, Newton’s P-difference interpolation formula for interval-valued function. Appl. Math. Inf. Sci. 14, 155–167 (2020)

    MathSciNet  Google Scholar 

  54. M. Rehman, Z. Arif, Numerical approximation of bounds of -values for a family of pascal matrices. Appl. Math. Inf. Sci. 13, 271–277 (2019)

    MathSciNet  Google Scholar 

  55. J. Avila, R. Lobato, J. Chuquipoma, M.D. Santos, Exponential stability and numerical results of a coupled system of wave equations with indirect control. Appl. Math. Inf. Sci. 14, 405–414 (2020)

    MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Swabi, Swabi, 23430, Khyber Pakhtunkhwa, Pakistan

    Imtiaz Ahmad

  2. Department of Basic Sciences, University of Engineering and Technology Peshawar, Peshawar, Pakistan

    Hijaz Ahmad

  3. Department of Mathematics, College of Science and Arts, Jouf University, Al-Qurayyat, Saudi Arabia

    Ahmed E. Abouelregal

  4. Renewable Energy Research Centre, Department of Teacher Training in Electrical Engineering, Faculty of Technical Education, King Mongkut’s University of Technology North Bangkok, 1518 Pracharat 1 Road, Bangsue, Bangkok, 10800, Thailand

    Phatiphat Thounthong

  5. Department of Mathematics, Faculty of Science, Sohag University, Sohag, Egypt

    M. Abdel-Aty

Authors
  1. Imtiaz Ahmad
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hijaz Ahmad
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Ahmed E. Abouelregal
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Phatiphat Thounthong
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. M. Abdel-Aty
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Hijaz Ahmad.

Ethics declarations

Conflict of interest

No conflict of interest.

Electronic supplementary material

Below is the link to the electronic supplementary material.

Supplementary material 1 (rar 1870 KB)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ahmad, I., Ahmad, H., Abouelregal, A.E. et al. Numerical study of integer-order hyperbolic telegraph model arising in physical and related sciences. Eur. Phys. J. Plus 135, 759 (2020). https://doi.org/10.1140/epjp/s13360-020-00784-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1140/epjp/s13360-020-00784-z

Search

Navigation