Fifth-Order A-WENO Schemes Based on the Adaptive Diffusion Central-Upwind Rankine-Hugoniot Fluxes

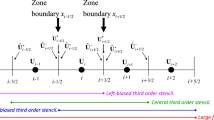
Abstract

We construct new fifth-order alternative WENO (A-WENO) schemes for the Euler equations of gas dynamics. The new scheme is based on a new adaptive diffusion central-upwind Rankine-Hugoniot (CURH) numerical flux. The CURH numerical fluxes have been recently proposed in [Garg et al. J Comput Phys 428, 2021] in the context of second-order semi-discrete finite-volume methods. The proposed adaptive diffusion CURH flux contains a smaller amount of numerical dissipation compared with the adaptive diffusion central numerical flux, which was also developed with the help of the discrete Rankine-Hugoniot conditions and used in the fifth-order A-WENO scheme recently introduced in [Wang et al. SIAM J Sci Comput 42, 2020]. As in that work, we here use the fifth-order characteristic-wise WENO-Z interpolations to evaluate the fifth-order point values required by the numerical fluxes. The resulting one- and two-dimensional schemes are tested on a number of numerical examples, which clearly demonstrate that the new schemes outperform the existing fifth-order A-WENO schemes without compromising the robustness.

Appendix A Adaptive Diffusion Central Flux

In this section, we describe the adaptive diffusion central numerical flux for the 1-D Euler equations of gas dynamics (10) and (11). According to [35], the flux is

$$\begin{aligned} \varvec{{{\mathcal {F}}}}_{{j+\frac{1}{2}}}^{\,\mathrm{FV}}={\frac{1}{2}}\left[ \varvec{F}(\varvec{U}_{{j+\frac{1}{2}}}^-)+\varvec{F}(\varvec{U}_{{j+\frac{1}{2}}}^+)\right] -{\frac{1}{2}}\alpha _{{j+\frac{1}{2}}}\Delta \varvec{U}_{{j+\frac{1}{2}}},\quad \Delta \varvec{U}_{{j+\frac{1}{2}}}:=\varvec{U}_{{j+\frac{1}{2}}}^+-\varvec{U}_{{j+\frac{1}{2}}}^-, \end{aligned}$$

where

$$\begin{aligned} \alpha _{{j+\frac{1}{2}}}=\left\{ \begin{aligned}&\min \left\{ \alpha _{{j+\frac{1}{2}}}^{\mathrm{min}},\kappa \alpha _{{j+\frac{1}{2}}}^{\mathrm{max}}\right\} ,&\theta _{{j+\frac{1}{2}}}\leqslant ({\Delta {x}})^2,\\&\max \left\{ \alpha _{{j+\frac{1}{2}}}^{\mathrm{min}},\min \big (\widehat{\alpha }_{{j+\frac{1}{2}}},\alpha _{{j+\frac{1}{2}}}^{\mathrm{max}}\big )\right\} ,&\text{ otherwise}. \end{aligned}\right. \end{aligned}$$

(A1)

In (A1), $\theta _{{j+\frac{1}{2}}}$ is given by (12),

$$\begin{aligned} \alpha _{{j+\frac{1}{2}}}^{\mathrm{min}}=\min \left\{ \big |u_{{j+\frac{1}{2}}}^-\big |,\big |u_{{j+\frac{1}{2}}}^+\big |\right\} ,\quad \alpha _{{j+\frac{1}{2}}}^{\mathrm{max}}=\max \left\{ \big |u_{{j+\frac{1}{2}}}^-\big |+c_{{j+\frac{1}{2}}}^-,\big |u_{{j+\frac{1}{2}}}^+\big |+c_{{j+\frac{1}{2}}}^+\right\} , \end{aligned}$$

(A2)

and

$$\begin{aligned} \widehat{\alpha }_{{j+\frac{1}{2}}}=\frac{2\big |\Delta F_{{j+\frac{1}{2}}}^{(3)}\big |}{\big |\Delta E_{{j+\frac{1}{2}}}\big |+\max \left\{ \big |\Delta E_{{j+\frac{1}{2}}}\big |,\varepsilon \right\} }. \end{aligned}$$

(A3)

In (A2), $c_{{j+\frac{1}{2}}}^\pm =\sqrt{\gamma p_{{j+\frac{1}{2}}}^\pm /\rho _{{j+\frac{1}{2}}}^\pm }$ with $p_{{j+\frac{1}{2}}}^\pm =(\gamma -1)\left[ E_{{j+\frac{1}{2}}}^\pm -\big ((\rho u)_{{j+\frac{1}{2}}}^\pm \big )^2/(2\rho _{{j+\frac{1}{2}}}^\pm )\right]$. In (A3), $\Delta E_{{j+\frac{1}{2}}}=E_{{j+\frac{1}{2}}}^+-E_{{j+\frac{1}{2}}}^-$ and $\Delta F_{{j+\frac{1}{2}}}^{(3)}=F^{(3)}\big (U_{{j+\frac{1}{2}}}^+\big )-F^{(3)}\big (U_{{j+\frac{1}{2}}}^-\big )$.