Investigating the mechanism of zinc-induced liquid metal embrittlement crack initiation in austenitic microstructure

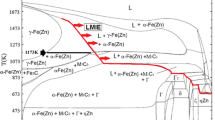
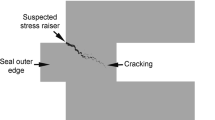
Abstract

Catastrophic brittle failure of ductile materials by liquid metal embrittlement (LME) is a widely documented phenomenon but the fundamentals of its initiation mechanism are poorly understood. The widespread use of Zn-coated advanced high strength steels in the automotive industry has been plagued by Zn-induced LME which is frequently observed in high-temperature forming and welding applications. In this study, numerical modeling and an atomic-scale experimental investigation are used in order to gain insight into the atomistic events that lead to the onset of LME cracking. The results showed that the formation of a stress-induced diffusion wedge (SIDW) at the exposed grain boundary (GB) due to the interdiffusion of Zn-embrittler atoms was the trigger for LME. The formation of the SIDW facilitated the diffusion of the Zn-embrittler atoms into the GBs, which compromised their mechanical integrity. The results show that LME initiation entails several steps: (i) solid-state GB diffusion, (ii) formation of the SIDW, (iii) eventual melting of the SIDW, and (iv) opening of the liquid wedge due to interdiffusion and the application of externally applied stresses.

Graphical Abstract

Appendices

Appendix A

The chemical potential gradient is considered the driving force for governing the grain boundary diffusion equations.

$${\mu }^{i}-{\mu }^{0}=kTln {a}^{i}$$

(A1)

In the case of applied tensile stress $(\sigma )$, the cal potential of any atom of volume $\Omega$ would be changed as [43]:

$${\mu }^{i}-{\mu }^{0}=kTln {a}^{i}+\sigma \Omega$$

(A2)

Considering the activity coefficient, ${\gamma }_{i}= \frac{{a}_{i}}{{C}_{i}}$ where ${C}_{i}$ is the concentration and substituting in Eq. (A2):

$${J}_{i}\propto \frac{\partial {\mu }_{i}}{\partial y}$$

(A3)

$${J}_{i}= -\frac{\delta {D}_{gb}}{kT}{C}_{i}\frac{\partial \mu }{\partial y}=-\frac{{\delta D}_{gb}}{kT} {C}_{i}\frac{\partial }{\partial y} ({\mu }^{0}+kTln ({\gamma }_{i}{C}_{i})+\sigma \Omega )$$

(A4)

where ${D}_{gb}$ and $\delta$ are GB diffusivity and thickness of GB, respectively. It has been assumed that the activity coefficient and atomic volume do not depend on stress or concentration, i.e.:

$${{{\gamma}}}_{{{i}}}\,{{o}}{{r}}\,{\Omega }\ne {{f}}\left({{{C}}}_{{{i}}},{{\sigma}}\right)\ne {{f}}\left({{x}},{{t}}\right)$$

(A5)

Differentiating the second part in the bracket of the right-hand side of Eq. (A4) gives the following equation:

$$\frac{\partial {\mu }_{i}}{\partial y}=kT{\eta }_{i}\frac{1}{{C}_{i}}\frac{\partial {C}_{i}}{\partial y}+\Omega \frac{\partial \sigma }{\partial y}$$

(A6)

where ${\eta }_{i}=\left(1+\frac{\partial ln{\gamma }_{i}}{\partial ln{C}_{i}}\right)$ is the thermodynamic factor [44]. Therefore, the flux equation in the presence of tensile stress can be described by the following equation:

$${J}_{i}= {-\delta {\eta }_{i}D}_{gb}\frac{\partial {C}_{i}}{\partial y}-\frac{\delta {D}_{gb}\Omega }{kT}{C}_{i} \frac{\partial \sigma }{\partial y}$$

(A7)

Based on the Gibbs–Duhem relationship, the thermodynamic factor for both chemical species is the same [44]:

$${\eta }_{Fe}={\eta }_{Zn}=\eta$$

(A8)

The thermodynamic factor is different in each binary system and depends on the composition and temperature. It is known that $\eta >1$ for systems with a negative heat of mixing and $\eta <1$ for the ones with positive heat mixing. The $\eta$ can be calculated using thermodynamic data. For simplicity, the thermodynamic factor has been taken as a constant equal to 1.0. It should be noted that this assumption will not affect the concentration profiles [44]. Therefore, the atomic fluxes along with grain boundary associated with the diffusion of Zn atoms into Fe-substrate (${J}_{Zn}$) and the diffusion of Fe-atom toward zinc layer (${J}_{Fe}$) can be represented as:

$${J}_{Zn}= -\updelta {D}_{gb}^{Zn}\frac{\partial {C}_{gb}^{Zn}}{\partial y}-\frac{{\Omega\updelta D}_{gb}^{Zn}}{kT}{C}_{gb}^{Zn} \frac{\partial \sigma }{\partial y}$$

(A9)

$${J}_{Fe}= -\updelta {D}_{gb}^{Fe}\frac{\partial {C}_{gb}^{Fe}}{\partial y}-\frac{{\Omega\updelta D}_{gb}^{Fe}}{kT}{C}_{gb}^{Fe} \frac{\partial \sigma }{\partial y}$$

(A10)

where ${D}_{gb}^{Zn}$ and ${D}_{gb}^{Fe}$ are grain boundary diffusivities of Zn and Fe atoms, respectively. In this model, the dependence of grain boundary diffusivities on pressure ($p$) and concentration is neglected, e.g.:

$$\frac{\partial D(\sigma )}{\partial p}\equiv 0$$

(A11)

$$\frac{\partial D(C)}{\partial C}\equiv 0$$

(A12)

Fick’s second law can be written down as follows:

$$\frac{\partial {C}_{i}}{\partial t}= -\frac{\partial {J}_{i}}{\partial y}$$

(A13)

$$\frac{\partial {C}_{gb}^{i}}{\partial t}= {\updelta D}_{gb}^{i}\frac{{\partial }^{2}{C}_{gb}^{i}}{\partial {y}^{2}}+\frac{\Omega {\updelta D}_{gb}^{i}}{kT}\frac{\partial }{\partial y} \left({C}_{gb}^{i}\frac{\partial \sigma }{\partial y}\right),\quad i={\rm Zn} \,{\rm and}\, {\rm Fe}$$

(A14)

$$\frac{\partial {C}_{gb}^{i}}{\partial t}={\updelta D}_{gb}^{i}\frac{{\partial }^{2}{C}_{gb}^{i}}{\partial {y}^{2}}+\frac{{\Omega\updelta D}_{gb}^{i}}{kT}\frac{\partial {C}_{gb}^{i}}{\partial y}\frac{\partial \sigma }{\partial y}+\frac{{\Omega\updelta D}_{gb}^{i}}{kT}{C}_{gb}^{i}\frac{{\partial }^{2}\sigma }{\partial {y}^{2}} ,\quad i={\rm Zn} \,and \,{\rm Fe}$$

(A15)

The stress profile along GB, $\sigma (y,t)$, can be represented as:

$${\sigma }_{gb} \left(y,t\right)= {E}^{*}{\int }_{0}^{\infty }K (y,x)\frac{\partial w(x,t)}{\partial x}dx$$

(A16)

$$\left(y,z\right)= \frac{1}{y-z}-\frac{1}{y+z}- \frac{2z(y-z)}{{\left(y+z\right)}^{3}}$$

(A17)

$${E}^{*}=\frac{E}{2\pi (1-{\nu }^{2})}$$

(A18)

It should be noted that the presence of SIDW with the width of $w (x, y)$ must be considered in the continuity equation. Therefore, Eq. (A13) is rewritten as:

$$\frac{\partial {C}_{i}}{\partial t}= -\frac{\partial {J}_{i}}{\partial y}-{C}_{i}^{gb}\frac{\partial w}{\partial t}$$

(A19)

where the second term of RHS of Eq. (A19) describes the role of the SIDW dimension on the diffusion flux. Therefore, Eq. (A15) is written as:

$$\begin{aligned}&\frac{{\partial C_{gb}^{Zn} }}{\partial t} = {\updelta }D_{gb}^{Zn} \frac{{\partial^{2} C_{gb}^{Zn} }}{{\partial y^{2} }} + \frac{{\Omega {\updelta }D_{gb}^{Zn} }}{kT}\frac{{\partial C_{gb}^{Zn} }}{\partial y}\frac{\partial \sigma }{{\partial y}} + \frac{{\Omega {\updelta }D_{gb}^{Zn} }}{kT}C_{gb}^{Zn} \frac{{\partial^{2} \sigma }}{{\partial y^{2} }}\\ &\quad- C_{gb}^{Zn} \frac{\partial w}{{\partial t}} \quad \quad \frac{{\partial w \left( {x,y} \right)}}{\partial t} > 0 \end{aligned}$$

(A20)

$$\begin{aligned}\frac{{\partial C_{gb}^{Fe} }}{\partial t} = {\updelta }D_{gb}^{Fe} \frac{{\partial^{2} C_{gb}^{Fe} }}{{\partial y^{2} }} + \frac{{\Omega {\updelta }D_{gb}^{Fe} }}{kT}\frac{{\partial C_{gb}^{Fe} }}{\partial y}\frac{\partial \sigma }{{\partial y}} + \frac{{\Omega {\updelta }D_{gb}^{Fe} }}{kT}C_{gb}^{Fe} \frac{{\partial^{2} \sigma }}{{\partial y^{2} }}\\&\quad - C_{gb}^{Fe} \frac{\partial w}{{\partial t}} \quad \quad \frac{{\partial w \left( {x,y} \right)}}{\partial t} > 0 \end{aligned}$$

(A21)

Assuming ${C}_{gb}^{Zn}+ {C}_{gb}^{Fe}=1$, Eq. (A21) can be rewritten as the following equations:

$$\frac{\partial }{{\partial t}}\left( {1 - ~C_{{{\text{gb}}}}^{{{\text{Zn}}}} } \right) = \delta D_{{{\text{gb}}}}^{{{\text{Fe}}}} \frac{{\partial ^{2} }}{{\partial y^{2} }}\left( {1 - ~C_{{{\text{gb}}}}^{{{\text{Zn}}}} } \right) + \frac{{\Omega \delta D_{{{\text{gb}}}}^{{{\text{Fe}}}} }}{{kT}}\frac{\partial }{{\partial y}}\left( {1 - ~C_{{{\text{gb}}}}^{{{\text{Zn}}}} } \right)\frac{{\partial \sigma }}{{\partial y}} + \frac{{\Omega \delta D_{{{\text{gb}}}}^{{{\text{Fe}}}} \left( {1 - ~C_{{{\text{gb}}}}^{{{\text{Zn}}}} } \right)}}{{kT}}\frac{{\partial ^{2} \sigma }}{{\partial y^{2} }} - \left( {1 - ~C_{{{\text{gb}}}}^{{{\text{Zn}}}} } \right)\frac{{\partial w~\left( {y,z} \right)}}{{\partial t}}$$

(A22)

$$\begin{aligned}&\frac{\partial {C}_{gb}^{Zn}}{\partial t}=\updelta {D}_{gb}^{Fe}\frac{{\partial }^{2}{C}_{gb}^{Zn}}{\partial {y}^{2}}+\frac{\Omega\updelta {D}_{gb}^{Fe}}{kT}\frac{\partial {C}_{gb}^{Zn}}{\partial y}\frac{\partial \sigma }{\partial y}\\& \quad-\frac{{\Omega\updelta D}_{gb}^{Fe}\left(1- {C}_{gb}^{Zn}\right)}{kT}\frac{{\partial }^{2}\sigma }{\partial {y}^{2}}+\frac{\partial w }{\partial t}-{\frac{\partial w}{\partial t}C}_{gb}^{Zn}\end{aligned}$$

(A23)

Combining Eq. 19 and Eq. 23 yields the following equation:

$$\begin{aligned}&\frac{\partial w \left(y,z\right)}{\partial t}=\updelta \frac{{\partial }^{2}{C}_{gb}^{Zn}}{\partial {y}^{2}} \left({D}_{gb}^{Zn}-{D}_{gb}^{Fe}\right)\\&\quad+\frac{\Omega\updelta }{kT}\frac{\partial {C}_{gb}^{Zn}}{\partial y}\frac{\partial \sigma }{\partial y}\left({D}_{gb}^{Zn}-{D}_{gb}^{Fe}\right)\\&\quad+\frac{\Omega\updelta }{kT}\frac{{\partial }^{2}\sigma }{\partial {y}^{2}}({D}_{gb}^{Zn}{C}_{gb}^{Zn}+{D}_{gb}^{Fe}{C}_{gb}^{Fe})\end{aligned}$$

(A24)

Equation (A24) illustrates the instantaneous dimension of the extra wedge material. Substituting Eq. (A24) into Eq. (A19) yields the following equation:

$$\frac{\partial {C}_{gb}^{Zn}}{\partial t}= \left({D}_{gb}^{Zn}{C}_{gb}^{Fe}+{D}_{gb}^{Fe}{C}_{gb}^{Zn}\right)\frac{{\partial }^{2}{C}_{gb}^{Zn}}{\partial {y}^{2}}+\frac{\Omega \left({D}_{gb}^{Zn}{C}_{gb}^{Fe}{+D}_{gb}^{Fe}{C}_{gb}^{Zn} \right)}{kT}\frac{\partial {C}_{gb}^{Zn}}{\partial y}\frac{\partial \sigma }{\partial y}+\frac{\Omega {C}_{gb}^{Zn}{C}_{gb}^{Fe}\left({D}_{gb}^{Zn}- {D}_{gb}^{Fe}\right)}{kT}\frac{{\partial }^{2}\sigma }{\partial {y}^{2}}$$

(A25)

Appendix B

The finite difference method (FDM) was employed to solve Eqs. 9 (a)-(c). First, ${C}_{i,j}$ is expanded in T direction while ${X}_{i}=i\Delta$ keeps constant.

$${C}_{i,j+1}={C}_{i,j}+\delta T{\left(\frac{\partial C}{\partial T}\right)}_{i,j}+\frac{{\left(\frac{{\partial }^{2}C}{\partial {T}^{2}}\right)}_{i,j}}{2!}{(\delta T)}^{2}+\frac{{\left(\frac{{\partial }^{3}C}{\partial {T}^{2}}\right)}_{i,j}}{3!}{(\delta T)}^{3}+\dots$$

(B1)

With neglecting the higher-order terms, Eq. (B1) can be written as:

$${\left(\frac{\partial C}{\partial T}\right)}_{i,j}=\frac{{C}_{i,j+1}-{C}_{i,j}}{\delta T}$$

(B2)

Similarly, Taylor’s series is applied for the ${C}_{i,j}$ in X direction while ${T}_{j}=j\Delta$ keep constant.

$${C}_{i-1,j}={C}_{i,j}-\delta X{\left(\frac{\partial C}{\partial X}\right)}_{i,j}+\frac{{\left(\frac{{\partial }^{2}C}{\partial {X}^{2}}\right)}_{i,j}}{2!}{(\delta X)}^{2}+\frac{{\left(\frac{{\partial }^{3}C}{\partial {X}^{2}}\right)}_{i,j}}{3!}{(\delta X)}^{3}+\dots$$

(B3)

$${C}_{i+1,j}={C}_{i,j}+\delta X{\left(\frac{\partial C}{\partial X}\right)}_{i,j}+\frac{{\left(\frac{{\partial }^{2}C}{\partial {X}^{2}}\right)}_{i,j}}{2!}{(\delta X)}^{2}+\frac{{\left(\frac{{\partial }^{3}C}{\partial {X}^{2}}\right)}_{i,j}}{3!}{(\delta X)}^{3}+\dots$$

(B4)

Combining Eq. (B3) and Eq. (B4) with neglecting higher orders yields the following set of equations:

$$\frac{{C}_{i-1,j}+{C}_{i+1,j}-2{C}_{i,j}}{{(\delta X)}^{2}}={\left(\frac{{\partial }^{2}C}{\partial {X}^{2}}\right)}_{i,j}$$

(B5)

$$\frac{{C}_{i-1,j}-{C}_{i+1,j}}{\delta X}={\left(\frac{\partial C}{\partial X}\right)}_{i,j}$$

(B6)

By substituting Eqs. (B5) and (B6) into Eqs. 9 (a)-(b) the following equations are obtained:

$$\frac{\partial {{W}}_{{i}} }{\partial {T}}= \left(1-\uptheta \right){({C}}_{{i}+1}-2{{C}}_{{i}}+ {{C}}_{{i}-1})+0.25\left(1-\uptheta \right)\left({{C}}_{{i}+1}- {{C}}_{{i}-1}\right)\left({{S}}_{{i}+1}- {{S}}_{{i}-1}\right)+({{C}}_{{i}}+\uptheta (1-{{C}}_{{i}})){({S}}_{{i}+1}-2{{S}}_{{i}}+ {{S}}_{{i}-1})$$

(B7)

$$\frac{\partial {{C}}_{{i}}}{\partial {T}}= \left[(\theta {{C}}_{{i}}+\left(1-{{C}}_{{i}}\right)){({C}}_{{i}+1}-2{{C}}_{{i}}+ {{C}}_{{i}-1})\right]+0.25\left[(\theta {{C}}_{{i}}+\left(1-{{C}}_{{i}}\right))({{C}}_{{i}+1}- {{C}}_{{i}-1})({{S}}_{{i}+1}- {{S}}_{{i}-1})\right]+\left[{{C}}_{{i}}(1-{{C}}_{{i}})\left(1-\uptheta \right){({S}}_{{i}+1}-2{{S}}_{{i}}+ {{S}}_{{i}-1})\right]$$

(B8)

As mentioned by Klinger and Rabkin [23], for solving Eq. 9 (c), the entire intergro-differentiate equation is discretized using a uniform grid ${{X}}_{{i}}={i}\Delta {x}$ and ${{Y}}_{{i}}={i}\Delta {y}$ as follows:

$$S_{i} = ~\mathop \sum \limits_{{k \ne i,i - 1}} \left[ {\left( {W_{{k + 1}} - W_{k} } \right)F_{{i,k}} + 0.5~\left( {W_{{i + 1}} - W_{{i - 1}} } \right)G_{i}^{{\left( 0 \right)}} + \left( {W_{{i + 1}} - W_{{i - 1}} - 2W_{i} } \right)G_{i}^{{\left( 1 \right)}} } \right]~$$

(B9)

The first term of the right-hand side (RHS) of Eq. (B9) is a linear approximation and the second part of RHS of Eq. (A6) is a parabolic approximation of ${{W}}_{{i}}$. Therefore, Eq. (B9) can be rewritten as follows:

$${{S}}_{{i}}= \sum_{{k}\ne {i},{i}-1}\left[\left({{W}}_{{k}+1}-{{W}}_{{k}}\right){{F}}_{{i},{k}}+0.5 \left({{W}}_{{i}+1}-{{W}}_{{i}-1}\right){{G}}_{{i}}^{(0)}+\left({{W}}_{{i}+1}-{{W}}_{{i}-1}-2{{W}}_{{i}}\right){{G}}_{{i}}^{(1)}\right]$$

(B10)

$${{F}}_{{i},{k}}={ln}\left|\frac{({i}+{k}+1)({i}-{k})}{({i}-{k}-1)({i}+{k})}\right|-\frac{6{i}}{\left({i}+{k}+1\right)\left({i}+{k}\right)}+\frac{2{{i}}^{2}(2{k}+2{i}+1)}{{({i}+{k}+1)}^{2}{({i}+{k})}^{2}}$$

(B11)

$${{G}}_{{i}}^{(0)}=\underset{{i}-1}{\overset{{i}+1}{\int }}\left(\frac{1}{{y}-{z}}-\frac{1}{{y}+{z}}- \frac{2{z}\left({y}-{z}\right)}{{\left({y}+{z}\right)}^{3}}\right){dZ}={ ln}\left|\frac{2{i}+1}{2{i}-1}\right|-\frac{12{i}}{\left(4{{i}}^{2}-1\right)}+\frac{16{{i}}^{3}}{{\left(4{{i}}^{2}-1\right)}^{2}}$$

(B12)

$${{G}}_{{i}}^{(1)}=\underset{{i}-1}{\overset{{i}+1}{\int }}\left(\frac{1}{{y}-{z}}-\frac{1}{{y}+{z}}- \frac{2{z}\left({y}-{z}\right)}{{\left({y}+{z}\right)}^{3}}\right)({z}-{y}){dZ}=-8{i ln}\left|\frac{2{i}+1}{2{i}-1}\right|+\frac{32{{i}}^{2}}{4{{i}}^{2}-1}-\frac{32{{i}}^{4}}{{(4{{i}}^{2}-1)}^{2}}$$

(B13)

Equations (B7), (B8), (B10-13) were solved by coding in MATLAB.