Hostname: page-component-8448b6f56d-qsmjn Total loading time: 0 Render date: 2024-04-23T07:31:32.576Z Has data issue: false hasContentIssue false
  • English
  • Français

Algèbres simples centrales de degré 5 et E8

Published online by Cambridge University Press:  20 November 2018

Philippe Gille
Philippe Gille*
Affiliation:
Département de Mathématiques, CNRS Bât. 425, Université de Paris–Sud F-91405 Orsay Cedex France, courriel: gille@math.u-psud.fr
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

As a consequence of a theorem of Rost-Springer, we establish that the cyclicity problem for central simple algebra of degree 5 on fields containg a fifth root of unity is equivalent to the study of anisotropic elements of order 5 in the split group of type 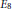${{E}_{8}}$.

Keywords

16S3512G0520G15algèbres simples centralescohomologie galoisienne
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 45 , Issue 3 , 01 September 2002 , pp. 388 - 398
Copyright
Copyright © Canadian Mathematical Society 2002

References

[BS] Borel, A. et de Siebenthal, J., Les sous-groupes fermés de rang maximum des groupes de Lie clos. Comment.Math. Helv. 23 (1949), 23–1949.Google Scholar
[Br] Brauer, R., On normal division algebras of degree 5. Proc. Nat. Acad. Sci. 24 (1938), 24–1938.Google Scholar
[BT] Bruhat, F. et Tits, J., Groupes algébriques sur un corps local III. Compléments et application á la cohomologie galoisienne. J. Fac. Sci. Univ. Tokyo 34 (1987), 34–1987.Google Scholar
[C1] Chernousov, V., The Hasse principle for groups of type E8. Dokl. Akad. Nauk SSSR (5) 306 (1989), 306–1989; traduction anglaise dans Soviet Math. Dokl. (3) 39 (1989), 39–1989.Google Scholar
[C2] Chernousov, V., Remark on the Serre mod-5 invariant for groups of type E8. Mat. Zametki (1) 56 (1994), 56–1994; traduction anglaise dans Math. Notes (1) 56 (1994), 56–1994.Google Scholar
[EKLV] Esnault, H., Kahn, B., Levine, M. et Viehweg, E., The Arason invariant and mod 2 algebraic cycles. J. Amer.Math. Soc. (1) 11 (1998), 11–1998.Google Scholar
[G1] Gille, P., La R-équivalence sur les groupes réductifs définis sur un corps global. Inst. Hautes Études Sci. Publ. Math. 86 (1997), 86–1997.Google Scholar
[G2] Gille, P., Invariants cohomologiques de Rost en caractéristique positive. K-theory 408 (2000), 408–2000.Google Scholar
[G3] Gille, P., Unipotent subgroups of reductive groups in characteristic p > 0. Duke Math. J., á paraître.+0.+Duke+Math.+J.,+á+paraître.>Google Scholar
[G4] Gille, P., An invariant of elements of finite order in semisimple simply connected algebraic groups. J. Group Theory, á paraître.Google Scholar
[Gr] Grothendieck, A., Le groupe de Brauer. I. Algébres d'Azumaya et interprétations diverses, II. Théorie cohomologique. Dix Exposés sur la Cohomologie des Schémas, North-Holland, Amsterdam et Masson, Paris, 46–66 et 67–87.Google Scholar
[Ha] Harder, H., Halbeinfache Gruppenschemata über Dedekindringen. Invent.Math. 4 (1967), 4–1967.Google Scholar
[MS] Merkurjev, A.-S. et Suslin, A.-A., K-cohomology of Severi-Brauer varieties and the norm residue homomorphism. Izv. Akad. Nauk SSSR 46 (1982), 46–1982; traduction dans Math. USSR Izv. 21 (1983), 21–1983.Google Scholar
[Mi] Milne, J. S., Étale Cohomology. Princeton Math. Ser. 33, Princeton, 1980.Google Scholar
[P] Peyre, E., Products of Severi-Brauer varieties and Galois cohomology. Proc. Sympos. Pure Math. 58, Part 2, Amer.Math. Soc., Providence, 1995, 369–401.Google Scholar
[PR] Platonov, V.-P. et Rapinchuk, A.-S., Algebraic Groups and Number Theory (traduction anglaise). Academic Press, 1994.Google Scholar
[RR] Raghunathan, M. S. et Ramanathan, A., Principal bundles on the affine line. Proc. Indian Acad. Sci. 93 (1984), 93–1984.Google Scholar
[RS] Rost, M. et Springer, T. A., On subgroups of type A4 in E8. Travaux en cours.Google Scholar
[Ro] Rowen, L. H., Are p-algebras having cyclic quadratic extensions necessarily cyclic? J. Algebra 215 (1999), 215–1999.Google Scholar
[RoS] Rowen, L. H. et Saltman, D., Dihedral algebras are cyclic. Proc. Amer.Math. Soc. 84 (1982), 84–1982.Google Scholar
[Se1] Serre, J.-P., Cohomologie galoisienne. Lecture Notes in Math. 5, 5e édition, Springer-Verlag, 1994.Google Scholar
[Se2] Serre, J.-P., Cohomologie galoisienne: Progrès et probléemes. Séminaire Bourbaki, exposé 783 (1993–94), Ast érisque 227(1995), 4, 229–257.Google Scholar
[SGA3] Schémas en groupes. Séminaire de Géométrie algébrique de l'I.H.E.S., 1963–1964, dirig é par M. Demazure et A. Grothendieck, Lecture Notes in Math. 151–153, Springer, 1970.Google Scholar
[T] Tignol, J.-P., Metacyclic division algebras of degree 5. Ring Theory 1989 (Jerusalem, 1988/89), Israel Math. Conf. Proc. 1 (1989), 1–1989.Google Scholar
[W] Wedderburn, J. H. M., On division algebras. Trans. Amer. Math. Soc. 22 (1921), 22–1921.Google Scholar