Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Longstaff, W. E.
1986.
A lattice-theoretic equivalent of the invariant subspace problem.
Proceedings of the American Mathematical Society,
Vol. 98,
Issue. 4,
p.
605.
Harrison, K. J.
and
Longstaff, W. E.
1986.
Automorphic images of commutative subspace lattices.
Transactions of the American Mathematical Society,
Vol. 296,
Issue. 1,
p.
217.
Molnar, Lajos
and
Kovács, Endre
2003.
An extension of a characterization of the automorphisms of Hilbert space effect algebras.
Reports on Mathematical Physics,
Vol. 52,
Issue. 1,
p.
141.
Šemrl, Peter
2004.
Applying projective geometry to transformations on rank one idempotents.
Journal of Functional Analysis,
Vol. 210,
Issue. 1,
p.
248.
Chevalier, Georges
2005.
Lattice Approach to Wigner-Type Theorems.
International Journal of Theoretical Physics,
Vol. 44,
Issue. 11,
p.
1905.
Chevalier, Georges
2005.
Automorphisms of An Orthomodular Poset of Projections.
International Journal of Theoretical Physics,
Vol. 44,
Issue. 7,
p.
985.
Fošner, Ajda
2006.
A note on local automorphisms.
Czechoslovak Mathematical Journal,
Vol. 56,
Issue. 3,
p.
981.
Molnár, Lajos
and
Šemrl, Peter
2007.
Elementary operators on self-adjoint operators.
Journal of Mathematical Analysis and Applications,
Vol. 327,
Issue. 1,
p.
302.
Du, Shuanping
Hou, Jinchuan
and
Bai, Zhaofang
2007.
Nonlinear maps preserving similarity on B(H).
Linear Algebra and its Applications,
Vol. 422,
Issue. 2-3,
p.
506.
Molnár, Lajos
and
Šemrl, Peter
2007.
Spectral Order Automorphisms of the Spaces of Hilbert Space Effects and Observables.
Letters in Mathematical Physics,
Vol. 80,
Issue. 3,
p.
239.
Chevalier, Georges
2007.
Handbook of Quantum Logic and Quantum Structures.
p.
429.
Plevnik, Lucijan
2012.
Maps on essentially infinite idempotent operators.
Journal of Mathematical Analysis and Applications,
Vol. 387,
Issue. 1,
p.
24.
Turilova, E.
2014.
Automorphisms of spectral lattices of unbounded positive operators.
Lobachevskii Journal of Mathematics,
Vol. 35,
Issue. 3,
p.
259.
Pankov, Mark
2015.
Geometrical characterization of semilinear isomorphisms of vector spaces and semilinear homeomorphisms of normed spaces.
Linear and Multilinear Algebra,
Vol. 63,
Issue. 4,
p.
695.
Busch, Paul
Lahti, Pekka
Pellonpää, Juha-Pekka
and
Ylinen, Kari
2016.
Quantum Measurement.
p.
499.
Cassinelli, Gianni
and
Lahti, Pekka
2016.
An Axiomatic Basis for Quantum Mechanics.
Foundations of Physics,
Vol. 46,
Issue. 10,
p.
1341.
Wang, Xin Hui
and
Ji, Guo Xing
2019.
Automorphisms on the Poset of Products of Two Projections.
Acta Mathematica Sinica, English Series,
Vol. 35,
Issue. 8,
p.
1393.
Golubić, Iva
and
Marovt, Janko
2020.
Monotone transformations on the cone of all positive semidefinite real matrices.
Mathematica Slovaca,
Vol. 70,
Issue. 3,
p.
733.
Wang, Xinhui
and
Ji, Guoxing
2020.
Star order automorphisms on the poset of type 1 operators.
Journal of Mathematical Analysis and Applications,
Vol. 491,
Issue. 2,
p.
124356.
Petek, Tatjana
and
Radić, Gordana
2020.
A note on equivalence preserving maps.
Linear and Multilinear Algebra,
Vol. 68,
Issue. 11,
p.
2289.
 (X) →
(X) →  (Y) of their lattices of subspaces. A basic theorem of projective geomtry [2, p. 44] asserts that, for spaces of dimension at least 3, any such projectivity is of the form Ψ(M) = SM for a bijection S:X → Y which is semi-linear in the sense that S is an additive mapping for which there exists an isomorphism σ:F→ G such that
(Y) of their lattices of subspaces. A basic theorem of projective geomtry [2, p. 44] asserts that, for spaces of dimension at least 3, any such projectivity is of the form Ψ(M) = SM for a bijection S:X → Y which is semi-linear in the sense that S is an additive mapping for which there exists an isomorphism σ:F→ G such that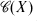 and
and  of closed subspaces are isomorphic if and only if X and Yare isomorphic (i.e., via a bicontinuous linear bijection).
of closed subspaces are isomorphic if and only if X and Yare isomorphic (i.e., via a bicontinuous linear bijection).



