Skip to main content
SpringerLink
Log in

Lagrangian solver for vector fractional diffusion in bounded anisotropic aquifers: Development and application

  • Research Paper
  • Published:
Fractional Calculus and Applied Analysis Aims and scope Submit manuscript

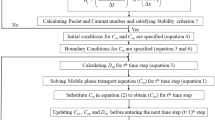
Abstract

Fractional-derivative models (FDMs) are promising tools for characterizing non-Fickian transport in natural geological media. Hydrologic applications of FDMs, however, have been limited in the last two decades, due to the lack of feasible models and solvers to quantify multi-dimensional anomalous diffusion for pollutants in bounded aquifers. This study develops and applies FDM tools to capture vector fractional dispersion for both conservative and reactive pollutants in fractional Brownian motion (fBm) random fields with bounded domains. A d-dimensional anisotropic fBm field for hydraulic conductivity (K) is first generated numerically. A particle-tracking based, fully Lagrangian solver is then developed to approximate particle dynamics in the fBm K fields under various boundary conditions, where the governing equation is the vector FDM subordination to regional flow. Numerical experiments show that the Lagrangian solver can combine nonlocal anomalous transport and local aquifer properties to quantify pollutant transport in bounded aquifers. Application analyses further reveal that the K correlation can significantly enhance the spreading of conservative pollutant particles, and increase the reaction rate by enhancing the mobility and mixing of reactant particles undergoing bimolecular reactions.

Extension of the Lagrangian solver is also discussed, including modeling transient flow, generalizing boundary conditions, and capturing complex chemical reactions. This study therefore provides the hydrologic community an efficient Lagrangian solver to model reactive anomalous transport in bounded anisotropic aquifers with any dimension, size, and boundary conditions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. E.E. Adams, L.W. Gelhar, Field study of dispersion in a heterogeneous aquifer 2. spatial moment analysis. Water Resour. Res. 28, No 12 (1992), 3293–3307.

    Google Scholar 

  2. B. Baeumer, Y. Zhang, R. Schumer, Incorporating super-diffusion due to sub-grid heterogeneity to capture non-Fickian transport. Ground Water 53, No 5 (2015), 699–708.

    Google Scholar 

  3. B. Baeumer, M. Kovács, M.M. Meerschaert, H. Sankaranarayanan, Boundary conditions for fractional diffusion. J. Comput. Appl. Math. 336 (2018), 408–424.

    MathSciNet  MATH  Google Scholar 

  4. B. Baeumer, M. Kovács, H. Sankaranarayanan, Fractional partial differential equations with boundary conditions. J. Differ. Equ. 264, No 2 (2018), 1377–1410.

    MathSciNet  MATH  Google Scholar 

  5. D.A. Benson, The fractional advection-dispersion equation: Development and application. Ph.D. Dissertation Univ. of Nev., Reno (1998)

    Google Scholar 

  6. D.A. Benson, M.M. Meerschaert, B. Baeumer, H.P. Scheffler, Aquifer operator scaling and the effect on solute mixing and dispersion. Water Resour. Res. 42 (2006), W01415; DOI:10.1029/2004WR003755.

    Google Scholar 

  7. J.M. Boggs, S.C. Young, L.M. Beard, Field study of dispersion in a heterogeneous aquifer 1. Overview and site description. Water Resour. Res. 28, No 12 (1992), 3281–3291.

    Google Scholar 

  8. A. Chechkin, V.Y. Gonchar, J. Klafter, R. Metzler, L.V. Tanatarov, Lévy flights in a steep potential well. J. Stat. Phys. 115, No 516 (2004), 1505–1535.

    MATH  Google Scholar 

  9. W. Chen, L. Ye, H.G. Sun, Fractional diffusion equations by the Kansa method. Comput. Math. Appl. 59, No 5 (2010), 1614–1620.

    MathSciNet  MATH  Google Scholar 

  10. W. Chen, G.F. Pang, A new definition of fractional Laplacian with application to modeling three-dimensional nonlocal heat conduction. J. Comput. Phys. 309, No 1 (2016), DOI:10.1016/j.jcp.2016.01.003.

    Google Scholar 

  11. W. Chen, F. Wang, Singular boundary method using time-dependent fundamental solution for transient diffusion problems. Eng. Anal. Bound. Elem. 68, No 7 (2016), 115–123.

    MathSciNet  MATH  Google Scholar 

  12. W. Chen, Y. Liang, X. Hei, Structural derivative based on inverse Mittag-Leffler function for modeling ultraslow diffusion. Fract. Calc. Appl. Anal. 19, No 5 (2016), 1250–1261; DOI:10.1515/fca-2016-0064; https://www.degruyter.com/view/j/fca.2016.19.issue-5/issue-files/fca.2016.19.issue-5.xml

    MathSciNet  MATH  Google Scholar 

  13. V.T. Chow, D.R. Maidment, L.W. Mays, Applied Hydrology. McGraw-Hill Publishing Companies (2013)

    Google Scholar 

  14. O.A. Cirpka, A.J. Valocchi, Debates—Stochastic subsurface hydrology from theory to practice: Does stochastic subsurface hydrology help solving practical problems of contaminant hydrogeology?. Water Resour. Res. 52 (2016), DOI:10.1002/2016WR019087.

  15. C.J.M. Cremer, I. Neuweiler, M. Bechtold, J. Vanderborght, Solute transport in heterogeneous soil with time-dependent boundary conditions. Vadose Zone J. 15, No 6 (2016), DOI:10.2136/vzj2015.11.0144.

    Google Scholar 

  16. F. Delay, P. Ackerer, C. Danquigny, Simulating solute transport in porous or fractured formations using random walk particle tracking. Vadose Zone J. 4, No 2 (2005), 360–379.

    Google Scholar 

  17. M. Dentz, T. Le Borgne, A. Englert, B. Bijeljic, Mixing, spreading and reaction in heterogeneous media: A brief review. J. Contam. Hydrol. 120–121 (2011), 1–17.

    Google Scholar 

  18. G.E. Fogg, Groundwater flow and sand body interconnectedness in a thick multiple-aquifer system. Water Resour. Res. 22 (1986), 679–694.

    Google Scholar 

  19. G.E. Fogg, Y. Zhang, Debates-stochastic subsurface hydrology from theory to practice: A geologic perspective. Water Resour. Res. 53, No 12 (2016), 9235–9245.

    Google Scholar 

  20. R. Gorenflo, F. Mainardi, A. Vivoli, Continuous-time random walk and parametric subordination in fractional diffusion. Chaos Soliton. Fract. 34, No 1 (2007), 87–103.

    MathSciNet  MATH  Google Scholar 

  21. C.T. Green, Y. Zhang, B.C. Jurgens, J.J. Starn, M.K. Landon, Accuracy of travel time distribution (TTD) models as affected by TTD complexity, observation errors, and model and tracer selection. Water Resour. Res. 50, No 7 (2014), 6191–6213.

    Google Scholar 

  22. I. Gupta, A.M. Wilson, B.J. Rostron, Groundwater age, brine migration, and large-sale solute transport in the Alberta Basin. Canada. Geofluids 15 (2015), 608–620.

    Google Scholar 

  23. A.W. Harbaugh, MODFLOW-2005, The U.S. Geological Survey Modular Ground-Water Model - the Ground-Water Flow Process. U.S. Geological Survey Techniques and Methods 6-A16, Reston, Virginia (2005)

    Google Scholar 

  24. K. Kang, S. Redner, Fluctuation-dominated kinetics in diffusion-controlled reactions. Phys. Rev. E 32, No 7 (1985), 435–447.

    Google Scholar 

  25. M. Karamouz, R. Kerachian, B. Zahraie, Monthly water resources and irrigation planning: case study of conjunctive use of surface and groundwater resources. J. Irrig. Drain Eng. 130, No 5 (2004), 391–402.

    Google Scholar 

  26. J.F. Kelly, H. Sankaranarayanan, M.M. Meerschaert, Boundary conditions for two-sided fractional diffusion. J. Comput. Phy. 376 (2019), 1089–1107.

    MathSciNet  MATH  Google Scholar 

  27. J.C. Koch, R.C. Toohey, D.M. Reeves, Tracer-based evidence of heterogeneity in subsurface flow and storage within a boreal hillslope. Hydrol. Porcess. 31, No 13 (2017), 2453–2463.

    Google Scholar 

  28. E.M. LaBolle, G.E. Fogg, A.F.B. Tompson, Random-walk simulation of transport in heterogeneous porous media: Local mass-conservation problem and implementation methods. Water Resour. Res. 32, No 3 (1996), 583–593.

    Google Scholar 

  29. E.M. LaBolle, J. Quastel, G.E. Fogg, J. Gravner, Diffusion processes in composite porous media and their numerical integration by random walks: Generalized stochastic differential equations with discontinuous coefficients. Water Resour. Res. 36 (2000), 651–662.

    Google Scholar 

  30. E.M. LaBolle, G.E. Fogg, Role of molecular diffusion in contaminant migration and recovery in an alluvial aquifer system. Transp. Porous Media 42 (2001), 155–179.

    Google Scholar 

  31. E.M. LaBolle, RWHet: Random Walk Particle Model for Simulating Transport in Heterogeneous Permeable Media, Version 3.2, User’s Manual and Program Documentation. Univ. of Calif., Davis (2006)

    Google Scholar 

  32. B.Q. Lu, Y. Zhang, H.G. Sun, C.M. Zheng, Lagrangian simulation of multi-step and rate-limited chemical reactions in multi-dimensional porous media. Water Sci. Eng. 11, No 2 (2018), 101–113.

    Google Scholar 

  33. B. Mandelbrot, J.W. van Ness, Fractional Brownian motions, fractional noises and applications. SIAM Rev. 10, No 4 (1968), 422–437.

    MathSciNet  MATH  Google Scholar 

  34. M. Marseguerra, A. Zoia, Monte Carlo investigation of anomalous transport in presence of a discontinuity and of an advection field. Physica A 377, No 2 (2007), 448–464.

    Google Scholar 

  35. M.M. Meerschaert, D.A. Benson, B. B. Baeumer, Operator Lévy motion and multiscaling anomalous diffusion. Phys. Rev. E 63 (2001), ID 021112; DOI:10.1103/PhysRevE.63.021112.

    Google Scholar 

  36. F. Obiri-Nyarko, S.J. Grajales-Mesa, G. Malina, An overview of permeable reactive barriers for in situ sustainable groundwater remediation. Chemosphere 111 (2014), 243–259.

    Google Scholar 

  37. L.J. Perez, J.J. Hidalgo, M. Dentz, Upscaling of mixing-limited bimolecular chemical reactions in Poiseuille flow. Water Resour. Res. 55, No 1 (2019), 249–269.

    Google Scholar 

  38. D.M. Reeves, D.A. Benson, M.M. Meerschaert, H.P. Scheffler, Transport of conservative solutes in simulated fracture networks: 2. Ensemble solute transport and the correspondence to operator-stable limit distribution. Water Resour. Res. 44 (2008), W05410; DOI:10.1029/2008WR006858.

    Google Scholar 

  39. K.R. Rehfeldt, J.M. Boggs, L.W. Gelhar, Field study of dispersion in a heterogeneous aquifer 3. Geostatistical analysis of hydraulic conductivity. Water Resour. Res. 28, No 12 (1992), 3309–3324.

    Google Scholar 

  40. P. Salamon, D. Fernàndez-Garcia, J. J. Gómez-Hernández, Modeling mass transfer processes using random walk particle tracking. Water Resour. Res. 42 (2006), W11417; DOI:10.1029/2006WR004927.

    Google Scholar 

  41. X. Sanchez-Vila, D. Fernàndez-Garcia, A. Guadagnini, Interpretation of column experiments of transport of solutes undergoing an irreversible bimolecular reaction using a continuum approximation. Water Resour. Res. 46 (2010), W12510; DOI:10.1029/2010WR009539.

    Google Scholar 

  42. W. Shao, Z. Yang, J. Ni, Y. Su, W. Nie, X. Ma, Comparison of single- and dual-permeability models in simulating the unsaturated hydro-mechanical behavior in a rainfall-triggered landslide. Landslides 15, No 12 (2018), 2449–2464.

    Google Scholar 

  43. E.R. Siirila, R.M. Maxwell, Evaluating effective reaction rates of kinetically driven solutes in large-scale statistically anisotropic media: human health risk implications. Water Resour. Res. 48 (2012), W04527.

    Google Scholar 

  44. R. Sinha, M. Israil, D.C. Singhal, A hydrogeophysical model of the relationship between geoelectric and hydraulic parameters of anisotropic aquifers. Hydrogeol. J. 17, No 5 (2009), ID 495; DOI:10.1007/s10040-008-0424-9.

    Google Scholar 

  45. A.Y. Sun, R.W. Ritzi, D.W. Sims, Characterization and modeling of spatial variability in a complex alluvial aquifer: implications on solute transport. Water Resour. Res. 44 (2008), W04402.

    Google Scholar 

  46. H.G. Sun, A.L. Chang, Y. Zhang, W. Chen, A review on variable-order fractional differential equations: Mathematical foundations, physical models, numerical methods and applications. Frac. Calc. Appl. Anal. 22, No 1 (2019), 27–59; DOI:10.1515/fca-2019-0003; https://www.degruyter.com/view/j/fca.2019.22.issue-1/issue-files/fca.2019.22.issue-1.xml

    MathSciNet  MATH  Google Scholar 

  47. J.J.A. van Kooten, Groundwater contaminant transport including adsorption and first order decay. Stoch. Hydrol. Hydraul. 8, No 3 (1994), 185–205.

    MATH  Google Scholar 

  48. E. Vidal-Henriquez, V. Zykov, E. Bodenschatz, A. Gholami, Convective instability and boundary driven oscillations in a reaction-diffusion-advection model. Chaos 27 (2017), ID 103110; DOI:10.1063/1.4986153.

    MathSciNet  MATH  Google Scholar 

  49. T. Vogel, H.H. Gerke, R. Zhang, M.Th. Van Genuchen, Modeling flow and transport in a two-dimensional dual-permeability system with spatially variable hydraulic properties. J. Hydro. 238, No 1-2 (2000), 78–89.

    Google Scholar 

  50. Y. Wang, S.A. Bradford, J. Simunek, Estimation and upscaling of dual-permeability model parameters for the transport of E. coli D21g in soils with preferential flow. J. Contam. Hydro. 159 (2014), 57–66.

    Google Scholar 

  51. Y. Zhang, D.A. Benson, M.M. Meerschaert, E.M. LaBolle, H.P. Scheffler, Random walk approximation of fractional-order multiscaling anomalous diffusion. Phys. Rev. E 74 (2006), ID 026706; DOI:10.1103/PhysRevE.74.026706.

    Google Scholar 

  52. Y. Zhang, E.M. LaBolle, K. Pohlmann, Monte Carlo approximation of anomalous diffusion in macroscopic heterogeneous media. Water Resour. Res. 45 (2009), W10417; DOI:10.1029/2008WR007448.

    Google Scholar 

  53. Y. Zhang, B. Baeumer, D.M. Reeves, A tempered multiscaling stable model to simulate transport in regional-scale fractured media. Geophy. Res. Lett. 37 (2010), L11405; DOI:10.1029/2010GL043609.

    Google Scholar 

  54. Y. Zhang, C. Papelis, Particle-tracking simulation of fractional diffusion-reaction processes. Phys. Rev. E 84 (2011), 066704; DOI:10.1103/PhysRevE.84.066704.

    Google Scholar 

  55. Y. Zhang, C.T. Green, G.E. Fogg, Subdiffusive transport in alluvial settings: The influence of medium heterogeneity. Adv. Water Resour. 54 (2013), 78–99.

    Google Scholar 

  56. Y. Zhang, M.M. Meerschaert, B. Baeumer, E.M. LaBolle, Modeling mixed retention and early arrivals in multidimensional heterogeneous media using an explicit Lagrangian scheme. Water Resour. Res. 51 (2015), 6311–6337; DOI:10.1002/2015WR016902.

    Google Scholar 

  57. Y. Zhang, C.T. Green, E.M. LaBolle, R.M. Neupauer, H.G. Sun, Bounded fractional diffusion in geological media: Definition and Lagrangian approximation. Water Resour. Res. 52, No 11 (2016), 8561–8577.

    Google Scholar 

  58. Y. Zhang, H.G. Sun, H.H. Stowell, M. Zayernouri, S.E. Hansen, A review of applications of fractional calculus in Earth system dynamics. Chaos Soliton. Frac. 102 (2017), 29–46.

    MathSciNet  MATH  Google Scholar 

  59. Y. Zhang, B. Baeumer, L. Chen, D.M. Reeves, H.S. Sun, A fully subordinated linear flow model for hillslope subsurface stormflow. Water Resour. Res. 53 (2017), 3491–3504; DOI:10.1002/2016WR020192.

    Google Scholar 

  60. Y. Zhang, M.M. Meerschaert, Particle tracking solutions of vector fractional differential equations: A review. Handbook of Fractional Calculus with Applications 3 Numerical Methods (2019), 275–285.

    MathSciNet  Google Scholar 

  61. C. Zheng, M. Bianchi, S.M. Gorelick, Lessons learned from 25 years of research at the MADE site. Ground Water 49, No 5 (2010), 649–662.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. State Key Lab. of Hydrology-Water Resources and Hydraulic Engineering College of Mechanics and Materials, Hohai University, Nanjing, 210098, China

    Yong Zhang & HongGuang Sun

  2. Department of Geological Sciences, University of Alabama, Tuscaloosa, AL 35487, USA

    Yong Zhang

  3. Guangdong Provincial Key Lab. of Soil and Groundwater Pollution Control, School of Environmental Science & Engineering Southern University of Science and Technology, Shenzhen, Guangdong 518055, China

    Chunmiao Zheng

Authors
  1. Yong Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. HongGuang Sun
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Chunmiao Zheng
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yong Zhang.

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhang, Y., Sun, H. & Zheng, C. Lagrangian solver for vector fractional diffusion in bounded anisotropic aquifers: Development and application. FCAA 22, 1607–1640 (2019). https://doi.org/10.1515/fca-2019-0083

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1515/fca-2019-0083

MSC 2010

Key Words and Phrases

Search

Navigation