Lax pair, solitons, breathers and modulation instability of a three-component coupled derivative nonlinear Schrödinger system for a plasma

Abstract

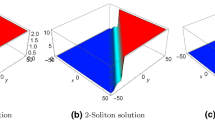
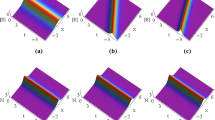
Plasmas are of current interest, supporting some nonlinear phenomena. In this paper, we investigate a three-component coupled derivative nonlinear Schrödinger system for a certain plasma. With respect to the complex envelopes of three fields in the plasma, we construct a Lax pair and obtain some soliton and breather solutions. Based on the soliton solutions, one bell-shaped soliton is presented. We present the interactions between/among the two/three bell-shaped solitons. Widths and velocities of the two solitons are unchanged after the interactions via the asymptotic analysis. When the two/three solitons have the same velocity, bound state of the two/three solitons is formed. Based on breather solutions, interaction between the Akhmediev breather (AB) and soliton, and interaction between the AB and breather-like soliton are shown. We present the interaction between the Kuznetsov–Ma breather and breather-like soliton. We investigate the modulation instability for the plane wave solutions via the linear stability analysis: If the imaginary part of $\Omega$ exists, the modulation instability appears, where $\Omega$ is the frequency of perturbation.

Appendix

We will study the MI for Eq. (3) via the linear stability analysis. Setting the plane wave solution $v[0]={\hat{\kappa }}e^{i\left[ {\hat{\omega }} \xi +\frac{1}{3}{\hat{\omega }}(2{{\hat{\kappa }}}^2\epsilon -3{\hat{\omega }})\varsigma \right] }$, we consider the solutions to be the following form:

$$\begin{aligned} v=({\hat{\kappa }}+{\hat{\varrho }} {\hat{u}})e^{i\left[ {\hat{\omega }} \xi +\frac{1}{3}{\hat{\omega }}(2{{\hat{\kappa }}}^2\epsilon -3{\hat{\omega }})\varsigma \right] }, \end{aligned}$$

(26)

where ${\hat{\varrho }}$ is a small parameter, ${\hat{u}}$ is a function with respect to $\xi$ and $\varsigma$, ${\hat{\omega }}\ne 0$ and ${\hat{\kappa }}$ are real constants. Taking Expression (26) into Eq. (3) can produce the linear system of ${\hat{u}}$ at $O({\hat{\varrho }})$ as

$$\begin{aligned} iv_\varsigma +\frac{2}{3}[{\hat{\kappa }}^2\epsilon {\hat{\omega }}(v+v^*)-i(2{\hat{\kappa }}^2\epsilon -3{\hat{\omega }})v_\xi -i{\hat{\kappa }}^2\epsilon v_\xi ^*]+v_{\xi \xi }=0. \end{aligned}$$

(27)

Then, we set that

$$\begin{aligned} {\hat{u}}={\hat{\sigma }}_{11}(\varsigma )e^{i({\hat{\Lambda }} \xi +{\hat{\Omega }}\varsigma )}+{\hat{\sigma }}_{12}^*(\varsigma )e^{-i({\hat{\Lambda }} \xi +{\hat{\Omega }}\varsigma )}, \end{aligned}$$

(28)

where ${\hat{\sigma }}_{11}(\varsigma )$ and ${\hat{\sigma }}_{12}(\varsigma )$ are the functions of $\varsigma$, ${\hat{\Lambda }}$ is the wave number and ${\hat{\Omega }}$ is the frequency of perturbation. Taking Solution (28) into Eq. (27), we acquire that

$$\begin{aligned} {\hat{\Upsilon }}_\varsigma =i{\hat{D}}{\hat{\Upsilon }}, \end{aligned}$$

(29)

where ${\hat{\Upsilon }}=({\hat{\sigma }}_{11}(\varsigma ),~{\hat{\sigma }}_{12}(\varsigma ))^T$. The matrix ${\hat{D}}$ can be acquired as

$$\begin{aligned} {\hat{D}}=&\begin{pmatrix} \frac{1}{3}[2{\hat{\kappa }}^2\epsilon ({\hat{\omega }}+2{\hat{\Lambda }})-3(2{\hat{\omega }}{\hat{\Lambda }}+{\hat{\Lambda }}^2+{\hat{\Omega }})]&{} \frac{2}{3}{\hat{\kappa }}^2\epsilon ({\hat{\omega }}+{\hat{\Lambda }})] \\ -\frac{2}{3}{\hat{\kappa }}^2\epsilon ({\hat{\omega }}-{\hat{\Lambda }})] &{}-\frac{1}{3}[2{\hat{\kappa }}^2\epsilon ({\hat{\omega }}-2{\hat{\Lambda }})+3(2{\hat{\omega }}{\hat{\Lambda }}-{\hat{\Lambda }}^2+{\hat{\Omega }})] \\ \end{pmatrix}. \end{aligned}$$

(30)

It is easy to see that we get two roots via solving the characteristic equation of the matrix ${\hat{D}}$ as

$$\begin{aligned}&{\hat{\Delta }}_1=\frac{4{\hat{\kappa }}^2{\hat{\Lambda }}\epsilon }{3}-2{\hat{\omega }}{\hat{\Lambda }}-\frac{1}{3}\sqrt{{\hat{\Lambda }}^2(4{\hat{\kappa }}^4-12{\hat{\kappa }}^2{\hat{\omega }}\epsilon +9{\hat{\Lambda }})}-{\hat{\Omega }},\\&{\hat{\Delta }}_2=\frac{4{\hat{\kappa }}^2{\hat{\Lambda }}\epsilon }{3}-2{\hat{\omega }}{\hat{\Lambda }}+\frac{1}{3}\sqrt{{\hat{\Lambda }}^2(4{\hat{\kappa }}^4-12{\hat{\kappa }}^2{\hat{\omega }}\epsilon +9{\hat{\Lambda }})}-{\hat{\Omega }}. \end{aligned}$$

If the characteristic equation of ${\hat{D}}$ has two real roots, thence the solutions are modulationally stable; if the characteristic equation of ${\hat{D}}$ has one pair of the complex conjugate roots. One of this pair of the conjugate complex roots has a negative imaginary part, and accordingly System (1) exhibits the baseband MI. Growth rates of the instability for Eq. (3) and System (1) are different.