Skip to main content
SpringerLink
Log in

Coupled van der Pol and Duffing oscillators: emergence of antimonotonicity and coexisting multiple self-excited and hidden oscillations

  • Regular Article
  • Published:
The European Physical Journal Plus Aims and scope Submit manuscript

Abstract

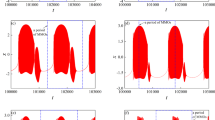
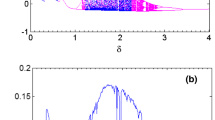
Due to its fundamental and technological relevance, the system formed by a van der Pol oscillator coupled to a Duffing oscillator is the subject of increasing research interest. In this contribution, we study the dynamics of a system composed of a van der Pol oscillator coupled to a Duffing oscillator with two asymmetric potential wells. In the considered coupling scheme, the van der Pol oscillator is disturbed by a signal proportional to the difference of position while the Duffing oscillator is driven by a signal proportional to the speed difference. We use analytical and numerical methods to shed light on the complex behaviors exhibited by the coupled system. We highlight striking phenomena such as hysteresis, parallel bifurcation branches, the coexistence of multiple (i.e., three, four or five) self-excited and hidden attractors, and the coexistence of symmetric and asymmetric bifurcation bubbles depending on the numerical values of initial conditions and parameters. An in-depth study of the coexistence of solutions is carried out by constructing basins of attraction. Sample experimental results captured from STM32F407ZE microcontroller-based digital implementation of the coupled system verify the striking dynamics features observed during the theoretical analysis. It should be noted that the coexistence of a hidden attractor with self-excited others is unique for the coupled oscillators system considered in the context of this work and thus deserves dissemination.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20

Similar content being viewed by others

References

  1. J.C. Sprott, Elegant Chaos: Algebraically Simple Chaotic Flows (World Scientific, 2010)

  2. G.A. Leonov, N.V. Kuznetsov, Hidden attractors in dynamical systems. From hidden oscillations in Hilbert–Kolmogorov, Aizerman, and Kalman problems to hidden chaotic attractor in Chua circuits. Int. J. Bifurc. Chaos 23(1), 1330002 (2013)

    Article  MathSciNet  Google Scholar 

  3. G. Leonov, N. Kuznetsov, T. Mokaev, Homoclinic orbits, and self-excited and hidden attractors in a Lorenz-like system describing convective fluid motion. Eur. Phys. J. Spec. Top. 224(8), 1421–1458 (2015)

    Article  Google Scholar 

  4. S. Zhang et al., Initial offset boosting coexisting attractors in memristive multi-double-scroll Hopfield neural network. Nonlinear Dyn. 102(4), 2821–2841 (2020)

    Article  Google Scholar 

  5. L. Huang et al., Heterogeneous and homogenous multistabilities in a novel 4D memristor-based chaotic system with discrete bifurcation diagrams. Complexity 66, 2020 (2020)

    MATH  Google Scholar 

  6. A.N. Pisarchik, U. Feudel, Control of multistability. Phys. Rep. 540(4), 167–218 (2014)

    Article  ADS  MathSciNet  Google Scholar 

  7. B. Van der Pol, LXXXVIII. On “relaxation-oscillations”. Lond. Edinb. Dublin Philos. Mag. J. Sci. 2(11), 978–992 (1926)

  8. G. Duffing, Erzwungene Schwingungen bei veränderlicher Eigenfrequenz und ihre technische Bedeutung. Vieweg (1918)

  9. P. Woafo, J. Chedjou, H. Fotsin, Dynamics of a system consisting of a van der Pol oscillator coupled to a Duffing oscillator. Phys. Rev. E 54(6), 5929 (1996)

    Article  ADS  Google Scholar 

  10. J. Chedjou et al., Analog simulation of the dynamics of a van der Pol oscillator coupled to a Duffing oscillator. IEEE Trans. Circuits Syst. I Fund. Theory Appl. 48(6), 748–757 (2001)

    Article  Google Scholar 

  11. Y.-J. Han, Dynamics of coupled nonlinear oscillators of different attractors; van der Pol oscillator and damped Duffing oscillator. J. Korean Phys. Soc. 37(1), 3–9 (2000)

    Google Scholar 

  12. J. Kengne et al., Analog circuit implementation and synchronization of a system consisting of a van der Pol oscillator linearly coupled to a Duffing oscillator. Nonlinear Dyn. 70(3), 2163–2173 (2012)

    Article  MathSciNet  Google Scholar 

  13. J. Chedjou, P. Woafo, S. Domngang, Shilnikov Chaos and Dynamics of a Self-Sustained Electromechanical Transducer (2001)

  14. J. Chedjou, et al., Behavior of a Self-Sustained Electromechanical Transducer and Routes to Chaos (2006)

  15. L.A. Low, P.G. Reinhall, D.W. Storti, An investigation of coupled van der Pol oscillators. J. Vib. Acoust. 125(2), 162–169 (2003)

    Article  Google Scholar 

  16. J. Guckenheimer, P. Holmes, Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields, vol. 42 (Springer, Berlin, 2013)

    MATH  Google Scholar 

  17. A. Nayfeh, D. Mook, Nonlinear Oscillations (Willey, New York, 1979)

    MATH  Google Scholar 

  18. A. Wolf et al., Determining Lyapunov exponents from a time series. Phys. D 16(3), 285–317 (1985)

    Article  MathSciNet  Google Scholar 

  19. Q. Lai, L. Wang, Chaos, bifurcation, coexisting attractors and circuit design of a three-dimensional continuous autonomous system. Optik 127(13), 5400–5406 (2016)

    Article  ADS  Google Scholar 

  20. Q. Lai, S. Chen, Research on a new 3D autonomous chaotic system with coexisting attractors. Optik 127(5), 3000–3004 (2016)

    Article  ADS  Google Scholar 

  21. H. Natiq et al., Can hyperchaotic maps with high complexity produce multistability? Chaos Interdiscip. J. Nonlinear Sci. 29(1), 11–103 (2019)

    Article  MathSciNet  Google Scholar 

  22. H. Natiq, S. Banerjee, M. Said, Cosine chaotification technique to enhance chaos and complexity of discrete systems. Eur. Phys. J. Spec. Top. 228(1), 185–194 (2019)

    Article  Google Scholar 

  23. S. Zhang et al., A novel no-equilibrium HR neuron model with hidden homogeneous extreme multistability. Chaos Solitons Fract. 145, 110761 (2021)

    Article  MathSciNet  Google Scholar 

  24. C. Li et al., Infinite multistability in a self-reproducing chaotic system. Int. J. Bifurc. Chaos 27(10), 1750160 (2017)

    Article  MathSciNet  Google Scholar 

  25. H. Natiq et al., Self-excited and hidden attractors in a novel chaotic system with complicated multistability. Eur. Phys. J. Plus 133(12), 1–12 (2018)

    Article  ADS  Google Scholar 

  26. S.P. Dawson et al., Antimonotonicity: inevitable reversals of period-doubling cascades. Phys. Lett. A 162(3), 249–254 (1992)

    Article  ADS  MathSciNet  Google Scholar 

  27. M. Bier, T.C. Bountis, Remerging Feigenbaum trees in dynamical systems. Phys. Lett. A 104(5), 239–244 (1984)

    Article  ADS  MathSciNet  Google Scholar 

  28. L. Kocarev et al., Experimental observation of antimonotonicity in Chua’s circuit. Int. J. Bifurc. Chaos 3(04), 1051–1055 (1993)

    Article  Google Scholar 

  29. K. Rajagopal et al., Dynamical investigation and chaotic associated behaviors of memristor Chua’s circuit with a non-ideal voltage-controlled memristor and its application to voice encryption. AEU Int. J. Electron. Commun. 107, 183–191 (2019)

    Article  Google Scholar 

  30. L. Zhou et al., Various attractors, coexisting attractors and antimonotonicity in a simple fourth-order memristive twin-T oscillator. Int. J. Bifurc. Chaos 28(04), 1850050 (2018)

    Article  MathSciNet  Google Scholar 

  31. B. Bao et al., Third-order RLCM-four-elements-based chaotic circuit and its coexisting bubbles. AEU Int. J. Electron. Commun. 94, 26–35 (2018)

    Article  Google Scholar 

  32. M. Itoh, Synthesis of electronic circuits for simulating nonlinear dynamics. Int. J. Bifurc. Chaos 11(03), 605–653 (2001)

    Article  Google Scholar 

  33. K. Rajagopal, A. Karthikeyan, A.K. Srinivasan, FPGA implementation of novel fractional-order chaotic systems with two equilibriums and no equilibrium and its adaptive sliding mode synchronization. Nonlinear Dyn. 87(4), 2281–2304 (2017)

    Article  Google Scholar 

  34. K. Rajagopal, A. Karthikeyan, A. Srinivasan, Dynamical analysis and FPGA implementation of a chaotic oscillator with fractional-order memristor components. Nonlinear Dyn. 91(3), 1491–1512 (2018)

    Article  Google Scholar 

  35. Z.T. Njitacke et al., Complex dynamics from heterogeneous coupling and electromagnetic effect on two neurons: application in images encryption. Chaos Solitons Fract. 153, 111577 (2021)

    Article  MathSciNet  Google Scholar 

  36. Z. Ju et al., Electromagnetic radiation induced non-chaotic behaviors in a Wilson neuron model. Chin. J. Phys. 77, 214–222 (2022)

    Article  MathSciNet  Google Scholar 

  37. R. Méndez-Ramírez et al., A new simple chaotic Lorenz-type system and its digital realization using a TFT touch-screen display embedded system. Complexity 66, 2017 (2017)

    MathSciNet  MATH  Google Scholar 

  38. T. Lu et al., Controlling coexisting attractors of conditional symmetry. Int. J. Bifurc. Chaos 29(14), 1950207 (2019)

    Article  MathSciNet  Google Scholar 

  39. Z.T. Njitacke et al., Control of coexisting attractors with preselection of the survived attractor in multistable Chua’s system: a case study. Complexity 66, 2020 (2020)

    MATH  Google Scholar 

  40. S. Fang et al., Multistability phenomenon in signal processing, energy harvesting, composite structures, and metamaterials: a review. Mech. Syst. Signal Process. 166, 108419 (2022)

    Article  Google Scholar 

  41. C. Miwadinou et al., Melnikov chaos in a modified Rayleigh–Duffing oscillator with ϕ 6 potential. Int. J. Bifurc. Chaos 26(05), 1650085 (2016)

    Article  MathSciNet  Google Scholar 

  42. J.C. Sprott, A proposed standard for the publication of new chaotic systems. Int. J. Bifurc. Chaos 21(09), 2391–2394 (2011)

    Article  Google Scholar 

Download references

Acknowledgements

This work is partially funded by Center for Nonlinear Systems, Chennai Institute of Technology, India, vide funding number CIT/CNS/2022/RD/006.

Author information

Authors and Affiliations

  1. Center for Nonlinear Systems, Chennai Institute of Technology, Chennai, India

    Ramakrishnan Balamurali & Karthikeyan Rajagopal

  2. Laboratoire d’Automatique et Informatique Appliquée (LAIA), IUT-FV Bandjoun, University of Dschang, Dschang, Cameroon

    Jacques Kengne & Russel Goune Chengui

Authors
  1. Ramakrishnan Balamurali
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jacques Kengne
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Russel Goune Chengui
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Karthikeyan Rajagopal
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jacques Kengne.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Balamurali, R., Kengne, J., Goune Chengui, R. et al. Coupled van der Pol and Duffing oscillators: emergence of antimonotonicity and coexisting multiple self-excited and hidden oscillations. Eur. Phys. J. Plus 137, 789 (2022). https://doi.org/10.1140/epjp/s13360-022-03000-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1140/epjp/s13360-022-03000-2

Search

Navigation