Abstract
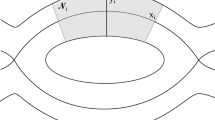
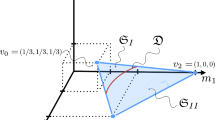
We consider periodic perturbations of conservative systems. The unperturbed systems are assumed to have two nonhyperbolic equilibria connected by a heteroclinic orbit on each level set of conservative quantities. These equilibria construct two normally hyperbolic invariant manifolds in the unperturbed phase space, and by invariant manifold theory there exist two normally hyperbolic, locally invariant manifolds in the perturbed phase space. We extend Melnikov’s method to give a condition under which the stable and unstable manifolds of these locally invariant manifolds intersect transversely. Moreover, when the locally invariant manifolds consist of nonhyperbolic periodic orbits, we show that there can exist heteroclinic orbits connecting periodic orbits near the unperturbed equilibria on distinct level sets. This behavior can occur even when the two unperturbed equilibria on each level set coincide and have a homoclinic orbit. In addition, it yields transition motions between neighborhoods of very distant periodic orbits, which are similar to Arnold diffusion for three or more degree of freedom Hamiltonian systems possessing a sequence of heteroclinic orbits to invariant tori, if there exists a sequence of heteroclinic orbits connecting periodic orbits successively.We illustrate our theory for rotational motions of a periodically forced rigid body. Numerical computations to support the theoretical results are also given.
Similar content being viewed by others
References
Arnold, V. I., On the Nonstability of Dynamical Systems with Many Degrees of Freedom, Soviet Math. Dokl., 1964, vol. 5, no. 3, pp. 581–585 see also: Dokl. Akad. Nauk SSSR, 1964, vol. 156, no. 1, pp. 9–12
Bouabdallah, S., Murrieri, P., and Siegwart, R., Design and Control of an Indoor Micro Quadrotor, in IEEE Internat. Conf. on Robotics and Automation (ICRA’04, New Orleans, La., 26 Apr–1 May 2004), pp. 4393–4396.
Coddington, E. A. and Levinson, N., Theory of Ordinary Differential Equations, New York: McGraw-Hill, 1955.
Doedel, E. and Oldeman, B.E., AUTO-07P: Continuation and Bifurcation Software for Ordinary Differential Equations, available online from https://doi.org/cmvl.cs.concordia.ca/auto (2012).
Eldering, J., Normally Hyperbolic Invariant Manifolds: The Noncompact Case, Paris: Atlantis, 2013.
Fenichel, N., Persistence and Smoothness of Invariant Manifolds for Flows, Indiana Univ. Math. J., 1971/1972, vol. 21, no. 3, pp. 193–226
Fenichel, N., Asymptotic Stability with Rate Conditions, Indiana Univ. Math. J., 1974, vol. 23, no. 12, pp. 1109–1137
Fenichel, N., Asymptotic Stability with Rate Conditions: 2, Indiana Univ. Math. J., 1977, vol. 26, no. 1, pp. 81–93
Gruendler, J., The Existence of Homoclinic Orbits and the Method of Melnikov for Systems in Rn, SIAM J. Math. Anal., 1985, vol. 16, no. 5, pp. 907–931
Gruendler, J., Homoclinic Solutions for Autonomous Dynamical Systems in Arbitrary Dimension, SIAM J. Math. Anal., 1992, vol. 23, no. 3, pp. 702–721
Guckenheimer, J. and Holmes, P.J., Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields, New York: Springer, 1983.
Hairer, E., Nørsett, S. P., and Wanner, G., Solving Ordinary Differential Equations: 1. Nonstiff Problems, 2nd ed., rev., Springer Series in Computational Mathematics, vol. 8, Berlin: Springer, 1993.
Haller, G., Chaos near Resonance, Appl. Math. Sci., vol. 138, New York: Springer, 1999.
Hamel, T., Mahony, R., Lozano, R., and Ostrowski, J., Dynamic Modelling and Configuration Stabilization for an X4-Flyer, IFAC Proceedings Volumes, 2002, vol. 35, no. 1, pp. 217–222
Hirsch, M.W., Pugh, C.C., and Shub, M., Invariant Manifolds, Lecture Notes in Math., vol. 583, New York: Springer, 1977.
Holmes, Ph. J. and Marsden, J. E., Horseshoes and Arnol’d Diffusion for Hamiltonian Systems on Lie Groups, Indiana Univ. Math. J., 1983, vol. 32, no. 2, pp. 273–309
Koiller, J., A Mechanical System with a “Wild” Horseshoe, J. Math. Phys., 1984, vol. 25, no. 5, pp. 1599–1604.
Krishnaprasad, P. S. and Marsden, J. E., Hamiltonian Structures and Stability for Rigid Bodies with Flexible Attachment, Arch. Rational Mech. Anal., 1987, vol. 98, no. 1, pp. 71–93
Palmer, K., Exponential Dichotomies and Transversal Homoclinic Points, J. Differential Equations, 1984, vol. 55, no. 2, pp. 225–256
Sakajo, T. and Yagasaki, K., Chaotic Motion of the N-Vortex Problem on a Sphere: 1. Saddle-Centers in Two-Degree-of-Freedom Hamiltonians, J. Nonlinear Sci., 2008, vol. 18, no. 5, pp. 485–525
Tabarrok, B. and Tong, X., Melnikov’s Method for Rigid Bodies Subject to Small Perturbation Torques, Arch. Appl. Mech., 1996, vol. 66, no. 4, pp. 215–230
Van der Heijden, G. H. M. and Yagasaki, K., Horseshoes for the Nearly Symmetric Heavy Top, J. Appl. Math. Phys (ZAMP), 2014, vol. 65, no. 2, pp. 221–240
Wiggins, S., Global Bifurcations and Chaos: Analytical Methods, Appl. Math. Sci., vol. 73, New York: Springer, 1988.
Wiggins, S., Introduction to Applied Nonlinear Dynamical Systems and Chaos, 2nd ed., Texts Appl. Math., vol. 2, New York: Springer, 2003.
Wiggins, S., Normally Hyperbolic Invariant Manifolds in Dynamical Systems, Appl. Math. Sci., vol. 105, New York: Springer, 1994.
Yagasaki, K., Homoclinic and Heteroclinic Orbits to Invariant Tori in Multi-Degree-of-Freedom Hamiltonian Systems with Saddle-Centres, Nonlinearity, 2005, vol. 18, no. 3, pp. 1331–1350
Yang, R., Krishnaprasad, P. S., and Dayawans, W., Optimal Control of a Rigid Body with Two Oscillators, in Mechanics Days, W., F. Shadwick, P. S. Krishnaprasad, S. Perinkulam, and T. S. Ratiu (Eds.), Providence, R. I.: AMS, 1996, pp. 233–260.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Yagasaki, K. Heteroclinic Transition Motions in Periodic Perturbations of Conservative Systems with an Application to Forced Rigid Body Dynamics. Regul. Chaot. Dyn. 23, 438–457 (2018). https://doi.org/10.1134/S1560354718040056
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S1560354718040056