Skip to main content
SpringerLink
Log in

Travelling waves in microstructure as the exact solutions to the 6th order nonlinear equation

  • Published:
Acoustical Physics Aims and scope Submit manuscript

Abstract

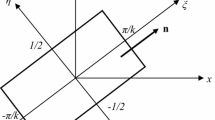
The travelling wave solutions to the nonlinear partial differential equation of 6th order are obtained for a solid having two different spatial scales introduced in the microstructure. The slaving principle method is applied, and the exact explicit solution is found in terms of the doubly periodic Weierstrass elliptic function for the corresponding ODE. Several particular cases are discussed for various parameter values, e.g., the solitary “mexican hat” pulse is found with polarity, depending on microstructure parameters.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. R. Mindlin, Arch. Rat. Mech. Anal. 16, 51 (1964).

    Article  MATH  MathSciNet  Google Scholar 

  2. G. Capriz, Continua with Microstructure (Springer, 1989).

  3. A. C. Eringen, Microcontinuum Field Theories. Foundations and Solids (Springer, 1999).

  4. R. Tenne et al., Topics Appl. Phys. 111, 635 (2007).

    Google Scholar 

  5. A. L. Ivanovsky, J. Inorg. Chem. 53, 1368 (2008).

    Google Scholar 

  6. A. Sachs and J. H. Lee, Nuovo Cim. B 111, 1429 (1996).

    Article  MathSciNet  ADS  Google Scholar 

  7. A. Salupere, J. Engelbrecht, O. Ilison, and L. Ilison, Math. Comp. Simul. 69, 502 (2005).

    Article  MATH  MathSciNet  Google Scholar 

  8. S. Abbasbandy and F. Samadian Zakaria, Nonlinear Dyn. 51, 83 (2008).

    Article  MATH  Google Scholar 

  9. A. Casasso, PhD Thesis (Univ. di Torino, 2009).

  10. A. Casasso and F. Pastrone, Wave Motion (2010), doi: 10.1016/j.wavemoti.2009.12.006.

  11. A. Casasso, F. Pastrone, and A. M. Samsonov, Proc. Estonian Acad. Sci. Phys. Math. 56, 75 (2007).

    MATH  MathSciNet  Google Scholar 

  12. J. Engelbrecht, F. Pastrone, M. Braun, and A. Berezovski, in Universality of Nonclassical Nonlinearity, Ed. by P. P. Delsanto (Springer, 2007), pp. 29–48.

  13. J. L. Ericksen, Int. J. Solids Struct., 371 (1970).

  14. F. Pastrone, Math. Mech. Solids 10, 349 (2005).

    Article  MATH  MathSciNet  Google Scholar 

  15. Mathematica and Wolfram Mathematica are trademarks of Wolfram Research, Inc.; www.wolfram.com.

  16. M. Abramowitz and I. Stegun, Handbook of Mathematical Functions (US Nat. Bureau of Standards, 1964).

  17. A. M. Samsonov, Strain Solitons in Solids and How to Construct Them (Chapman and Hall, CRC, 2001).

  18. L. K. Zarembo and V. A. Krasil’nikov, Sov. Phys. Usp. 102, 549 (1970).

    Google Scholar 

  19. O. V. Rudenko, A. I. Korobov, and M. Yu. Izosimova, Acoust. Phys. 56, 151 (2010).

    Article  ADS  Google Scholar 

  20. L. A. Ostrovsky and O. V. Rudenko, Acoust. Phys. 55, 715 (2009).

    Article  ADS  Google Scholar 

  21. V. Yu. Zaitsev, V. E. Nazarov, and V. I. Talanov, Phys. Usp. 176, 97 (2006).

    Google Scholar 

  22. E. Majewski, in Earthquake Source Asymmetry, Structural Media and Rotation Effects, Ed. by R. Teisseyre, E. Majewski, and M. Takeo (Springer, 2006), pp. 296–300.

  23. A. Berezovski, J. Engelbrecht, and G. Maugin, in Mechanics of Microstructured Solids, Lect. Notes Appl. Comput. Mech., vol. 46 (Springer, 2009), pp. 21–28.

  24. O. V. Rudenko and V. A. Robsman, Dokl. Phys. 47(6), 443 (2002).

    Article  MathSciNet  ADS  Google Scholar 

  25. M. V. Averiyanov, M. S. Basova, and V. A. Khokhlova, Acoust. Phys. 51(5), 495 (2005).

    Article  ADS  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Torino, Torino, 10123, Italy

    A. Casasso & F. Pastrone

  2. The Ioffe Physical Technical Institute of the Russian Academy of Sciences, St. Petersburg, 194021, Russia

    A. M. Samsonov

Authors
  1. A. Casasso
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. F. Pastrone
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. A. M. Samsonov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to F. Pastrone.

Additional information

The article is published in the original.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Casasso, A., Pastrone, F. & Samsonov, A.M. Travelling waves in microstructure as the exact solutions to the 6th order nonlinear equation. Acoust. Phys. 56, 871–876 (2010). https://doi.org/10.1134/S1063771010060114

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S1063771010060114

Keywords

Search

Navigation