Skip to main content
SpringerLink
Log in

A minimal triangulation of complex projective plane admitting a chess colouring of four-dimensional simplices

  • Published:
Proceedings of the Steklov Institute of Mathematics Aims and scope Submit manuscript

Abstract

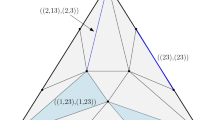
We construct and study a new 15-vertex triangulation X of the complex projective plane ℂP2. The automorphism group of X is isomorphic to S 4 × S 3. We prove that the triangulation X is the minimal (with respect to the number of vertices) triangulation of ℂP2 admitting a chess colouring of four-dimensional simplices. We provide explicit parametrizations for the simplices of X and show that the automorphism group of X can be realized as a group of isometries of the Fubini-Study metric. We find a 33-vertex subdivision \( \bar X \) of the triangulation X such that the classical moment mapping μ: ℂP2 → Δ2 is a simplicial mapping of the triangulation \( \bar X \) onto the barycentric subdivision of the triangle Δ2. We study the relationship of the triangulation X with complex crystallographic groups.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. W. Kühnel and T. F. Banchoff, “The 9-Vertex Complex Projective Plane,” Math. Intell. 5(3), 11–22 (1983).

    Article  MATH  Google Scholar 

  2. W. Kühnel and G. Lassmann, “The Unique 3-Neighborly 4-Manifold with Few Vertices,” J. Comb. Theory A 35, 173–184 (1983).

    Article  MATH  Google Scholar 

  3. B. Bagchi and B. Datta, “On Kühnel’s 9-Vertex Complex Projective Plane,” Geom. Dedicata 50, 1–13 (1994).

    Article  MATH  MathSciNet  Google Scholar 

  4. B. Morin and M. Yoshida, “The Kühnel Triangulation of the Complex Projective Plane from the View Point of Complex Crystallography. I,” Mem. Fac. Sci., Kyushu Univ., Ser. A 45(1), 55–142 (1991).

    MATH  MathSciNet  Google Scholar 

  5. P. Arnoux and A. Marin, “The Kühnel Triangulation of the Complex Projective Plane from the View Point of Complex Crystallography. II,” Mem. Fac. Sci., Kyushu Univ., Ser. A 45(2), 167–244 (1991).

    MATH  MathSciNet  Google Scholar 

  6. S. P. Novikov and I. A. Dynnikov, “Discrete Spectral Symmetries of Low-Dimensional Differential Operators and Difference Operators on Regular Lattices and Two-Dimensional Manifolds,” Usp. Mat. Nauk 52(5), 175–234 (1997) [Russ. Math. Surv. 52, 1057–1116 (1997)].

    MathSciNet  Google Scholar 

  7. I. A. Dynnikov and S. P. Novikov, “Laplace Transforms and Simplicial Connections,” Usp. Mat. Nauk 52(6), 157–158 (1997) [Russ. Math. Surv. 52, 1294–1295 (1997)].

    MathSciNet  Google Scholar 

  8. I. Dynnikov and S. Novikov, “Geometry of the Triangle Equation on Two-Manifolds,” Moscow Math. J. 3(2), 419–438 (2003).

    MATH  MathSciNet  Google Scholar 

  9. T. F. Banchoff and W. Kühnel, “Equilibrium Triangulations of the Complex Projective Plane,” Geom. Dedicata 44, 313–333 (1992).

    Article  MATH  MathSciNet  Google Scholar 

  10. N. Brady, J. McCammond, and J. Meier, “Bounding Edge Degrees in Triangulated 3-Manifolds,” Proc. Am. Math. Soc. 132(1), 291–298 (2004).

    Article  MATH  MathSciNet  Google Scholar 

  11. S. V. Matveev, “Closed Polyhedral 3-Manifolds with K ≥ 0,” Oberwolfach Rep. 3(1), 668–670 (2006).

    Google Scholar 

  12. F. H. Lutz, “Triangulated Surfaces and Higher-Dimensional Manifolds,” Oberwolfach Rep. 3(1), 706–707 (2006).

    Google Scholar 

  13. K. V. Madahar and K. S. Sarkaria, “A Minimal Triangulation of the Hopf Map and Its Application,” Geom. Dedicata 82, 105–114 (2000).

    Article  MATH  MathSciNet  Google Scholar 

  14. M. Joswig, “The Group of Projectivities and Colouring of the Facets of a Simple Polytope,” Usp. Mat. Nauk 56(3), 171–172 (2001) [Russ. Math. Surv. 56, 584–585 (2001)].

    MathSciNet  Google Scholar 

  15. M. Joswig, “Projectivities in Simplicial Complexes and Colorings of Simple Polytopes,” Math. Z. 240(2), 243–259 (2002).

    Article  MATH  MathSciNet  Google Scholar 

  16. M. W. Davis and T. Januszkiewicz, “Convex Polytopes, Coxeter Orbifolds and Torus Actions,” Duke Math. J. 62(2), 417–451 (1991).

    Article  MATH  MathSciNet  Google Scholar 

  17. A. A. Gaifullin, “The Manifold of Isospectral Symmetric Tridiagonal Matrices and Realization of Cycles by Aspherical Manifolds,” Tr. Mat. Inst. im. V.A. Steklova, Ross. Akad. Nauk 263, 44–63 (2008) [Proc. Steklov Inst. Math. 263, 38–56 (2008)].

    Google Scholar 

  18. F. H. Lutz, “Triangulated Manifolds with Few Vertices: Combinatorial Manifolds,” arXiv:math/0506372.

  19. H. S. M. Coxeter and W. O. J. Moser, Generators and Relations for Discrete Groups, 4th ed. (Springer, New York, 1980).

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Moscow State University, Moscow, 119991, Russia

    A. A. Gaifullin

  2. Institute for Information Transmission Problems, Russian Academy of Sciences, Bol’shoi Karetnyi per. 19, Moscow, 127994, Russia

    A. A. Gaifullin

Authors
  1. A. A. Gaifullin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to A. A. Gaifullin.

Additional information

Published in Russian in Trudy Matematicheskogo Instituta imeni V.A. Steklova, 2009, Vol. 266, pp. 33–53.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Gaifullin, A.A. A minimal triangulation of complex projective plane admitting a chess colouring of four-dimensional simplices. Proc. Steklov Inst. Math. 266, 29–48 (2009). https://doi.org/10.1134/S008154380903002X

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S008154380903002X

Keywords

Search

Navigation