Abstract
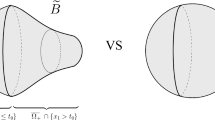
The classical isoperimetric problem for volumes is solved in ℝ×n(1). Minimizers are shown to be invariant under the group O(n) acting standardly on \({\mathbb{S}}\) n, via a symmetrization argument, and are then classified. Solutions are found among two (one-parameter) families: balls and sections of the form [a, b] ×\({\mathbb{S}}\) n. It is shown that the minimizers may be of both types. For n= 2, it is shown that the transition between the two families occurs exactly once. Some results for general n are also presented.
Similar content being viewed by others
References
Almgren, Jr., F. J.: Some interior regularity theorems for minimal surfaces and an extension of Bernstein's problem, Ann. Math. 84 (1966), 277–292.
Almren, Jr., F. J.: Existence and regularity almost everywhere of solutions to elliptic variationa problems with constraints, Mem. Amer. Math. Soc. 4 (165), (1976).
Almgren, Jr., F. J.: Spherical symmetrization, Rend. Circ. Mat. Palermo (2) Suppl.(1987), 11–25, in Proceedings of the International Workshop on Integral Functionals in the Calculus of Variations (Trieste, 1985).
Benjamin, I. and Cao, J.: A new isoperimetric comparison theorem for surfaces of variable curvature, Duke Math. J. 85 (1996), 359–396.
Barbosa, J. L., do Carmo, M. and Eschenburg, J.: Stability of hypersurfaces of constant mean curvature in Riemannians manifolds, Math. Z. 197 (1988), 123–138.
Bombieri, E. and Giusti, E.: Harnack's inequality for elliptic differential equations on minimal surfaces, Invent. Math. 15 (1972), 24–46.
Bavard, C. and Pansu, P.: Sur le volume minimal de R 2, Ann. Sci. École Norm. Sup 19 (4) (1986), 479–490.
Burago, Yu. D. and Zalgaller, V. A.: Geometric Inequalities, Springer-Verlag, New York, 1988.
Federer, H.: Geometric Measure Theory, Springer-Verlag, New York, 1969.
Gallot, S.: Inégalités isopérimétriques et analytiques sur les variétés rimanniennes, Astérisque (1988), No. 163–164, 31–91.
Giusti, E.: Minimal Surfaces and Functions of Bounded Variation, Monogr. Math. 80, Birkhäuser, Boston, 1984.
Gonzalez, E., Massari, U. and Tamanini, I.: On the regularity of boundaries of sets minimizing perimeter with a volume constraint, Indiana Univ. Math. J. 32 (1983), 25–37.
Hsiang, W.-T. and Hsiang, W.-Y.: On the uniqueness of isoperimetric solutions and imbedded soap bubbles in noncompact symmetric spaces, Invent. Math. 85 (1989), 39–58.
Hsiang, W.-Y.: Isoperimetric regions and soap bubbles, In: B. Lawson Jr. and K. Tenenblat (eds), Differential Geometry: A symposium in honor of Manfredo do Carmo, Pitman Monogr. Surveys Pure Appl. Math. 52, Longman Sci. Tech., Harlow, 1991, pp. 229–240.
Hsiang, W.-Y.: On soap bubbles and isoperimetric in noncompact symmetric spaces, I, T ohoku Mat. J. 44 (1992), 151–175.
Johnson, D. and Morgan, F.: Some sharp isoperimetric theorems for Riemannian manifolds, Indiana Univ. Math. J. 49 (2000), 1017–1041.
Kawohl, B.: Rearrangements and Convexity of Level Sets in PDE, Lecture Notes in Math. 1150, Springer-Verlag, Berlin, 1985.
Lieb, E. H. and Loss, M.: Analysis, Grad. Stud. Math. 14, Amer. Math. Soc., Providence, RI, 1997.
Massari, U.: Esistenza and regolarit` a delle ipersuperfici di curvatura media assegnata in R n, Arch. Rat. Mech. Anal. 55 (1974), 357–382.
Morgan, F.: Clusters minimizing area plus length of singular curves, Math. Ann. 299 (1994), 697–714.
Osserman, R.: The isoperimetric inequality, Bull. Amer. Math. Soc. 84 (1978), 1182–1238.
Pansu, P.: Sur la régularité duprofil isopérimétrique des surfaces rimeanniennes compactes, Ann. Inst. Fourier 48 (1998), 247–264.
Pansu, P.: Profil isopérimétrique, métriques périodiques et formes d'équilibre des cristaux, (French) [Isoperimetric profile, periodic metrics and equilibrium shapes of crystals.] ESAIM Control Optim. Calc. Var. 4 (1999), 631–665 (electronic).
Pedrosa, R. H. L.: Minimal partial rearrangements with applications to symmetry properties of optimal composite membranes, Preprint, 2004.
Pedrosa, R. H. L.: Rearrangements and symmetrization in Euclidean and Riemannian spaces, in preparation.
Pedrosa, R. H. L. and Ritoré, M.: Isoperimetric domains in the Riemannian product of a circle with a simply connected space form and applications to free boundary problems, Indiana Univ. Math. J. 48 (1999), 1357–1394.
Pittet, Ch.: The isoperimetric profile of homogeneous Riemannian manifolds'. J. Differential Geom. 54 (2000), 255–302.
Ritoré, M.: Constant geodesic curvature curves and isoperimetric domains in rotationally symmetric surfaces, Comm. Anal. Geom. 9 (2001), 1093–1138.
Ritoré, M. and Ros, A.: Stable constant mean curvature tori and the isoperimetric problem in three space forms, Comm. Math. Helv. 67 (1992), 293–305.
Ritoré, M. and Ros, A.: The space of stable constant mean curvature surfaces and index one minimal surfaces embedded in flat three manifolds, Trans. Amer. Math. Soc. 348 (1996), 391–410.
Ritoré, M. and Ros, A.: Some updates on isoperimetric problems, Math. Intelligencer 24 (2002), 9–14.
Ros, A.: The isoperimetric and Willmore problems. In: Global Differential Geometry: The Mathematical Legacy of Alfred Gray, Bilbao, 2000, Contemp. Math. 288, Amer. Math. Soc., Providence, RI, 2001, pp. 149–161.
Ros, A.: The Isoperimetric problem. Lecture series at the Clay Mathematics Institute Summer School on the Global Theory of Minimal Surfaces, Summer 2001, MSRI, Berkeley, California.
Talenti, G.: The standard isoperimetric theorem. In: P. M. Gruber and J. M. Wills (eds), Handbook of Convex Geometry, Vol. A, North-Holland, Amsterdam, 1993, pp. 73–123.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Pedrosa, R.H.L. The Isoperimetric Problem in Spherical Cylinders. Annals of Global Analysis and Geometry 26, 333–354 (2004). https://doi.org/10.1023/B:AGAG.0000047528.20962.e2
Issue Date:
DOI: https://doi.org/10.1023/B:AGAG.0000047528.20962.e2