Abstract
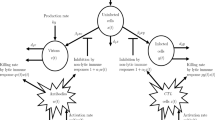
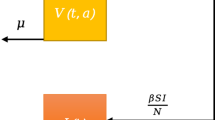
The qualitative behaviors of a system of ordinary differential equations and a system of differential-integral equations, which model the dynamics of disease transmission for tuberculosis (TB), have been studied. It has been shown that the dynamics of both models are governed by a reproductive number. All solutions converge to the origin (the disease-free equilibrium) when this reproductive number is less than or equal to the critical value one. The disease-free equilibrium is unstable and there exists a unique positive (endemic) equilibrium if the reproductive number exceeds one. Moreover, the positive equilibrium is stable. Our results show that the qualitative behaviors predicted by the model with arbitrarily distributed latent stage are similar to those given by the TB model with an exponentially distributed period of latency.
Similar content being viewed by others
REFERENCES
Anderson, R. M. (1982). Population Dynamics of Infections Diseases, Chapman and Hall, London-New York.
Anderson, R. M., and May, R. M. (1982). Population Biology of Infections Diseases, Springer-Verlag, Berlin-Heidelberg-New York.
Anderson, R. M., and May, R. M. (1991). Infectious Diseases of Humans, Oxford University Press, Oxford-New York-Tokyo.
Blower, S. M., McLean, A. R., Porco, T. C., Small, P. M., Hopwell, P. C., Sanchez, M. A, and Moss, A. R. (1995). The intrinsic transmission dynamics of tuberculosis epidemics. Nature Med. 1, 815-821.
Castillo-Chavez, C., and Feng, Z. (1997a). To treat or not to treat: The case of tuberculosis. J. Math. Biol. 35, 629-659.
Castillo-Chavez, C., and Feng, Z. (1997b). Mathematical models for the disease dynamics of tuberculosis. In Arino, O., Axelrod, D., and Kimmel, M. (eds.), Advances in Mathematical Population Dynamics-Molecules, Cells and Man, World Scientific, Singapore, pp. 629-656.
Castillo-Chavez, C., and Feng, Z. (1998). global stability of an age-structure model for TB and its applications to optimal vaccination strategies. Math. Biosci . 151, 135-154.
Castillo-Chavez, C., Hethcote, H. W., Andreason, V., Levin, S. A., and Liu, W. (1988). Cross-immunity in the dynamics of homogeneous and heterogeneous populations. In Gross, L., Hallam, T. G., and Levin, S. A. (eds.), Mathematical Biology, Proceedings, Autumn Course Research Seminars, Trieste, 1986, World Scientific, Singapore, pp. 303-316.
Castillo-Chavez, C., Cooke, K., Huang, W., and Levin, S. A. (1989). Results on the dynamics for models for the sexual transmission of the human immunodeficiency virus. Appl. Math. Lett. 2, 327-331.
Castillo-Chavez, C., Feng, Z., and Capurro, A. F. (2000). A model for TB with exogenous reinfection. Theor. Popul. Biol. 57, 235-247.
Comstook, G. W., and Edwards, P. Q. (1975). The competing risks of tuberculosis and hepatitis for adult tuberculin reactors. Am. Rev. Respir. Dis. 87 (Suppl.), 1-88.
Comstook, G. W., Livesay, V. T., and Woolpert, S. F. (1974). The prognosis of a positive tuberculin reaction in childhood and adolescence. Am. J. Epidemiol. 99, 131-138.
Dietz, K. (1979). Epidemiologic interference of virus populations. J. Math. Biol., 8, 291-300.
Dietz, K., and Schenzle, D. (1985). Proportionate mixing models for age-dependent infection transmission. J. Math. Biol. 22, 117-120.
Feng, Z. (1994). A Mathematical Model for the Dynamics of Childhood Disease Under the Impact of Isolation, Thesis, Arizona State University, Tempe.
Feng, Z., and Thieme, R. H. (1985). Multi-annual outbreaks of childhood disease revisited: The impact of isolation. Math. Biosci. 128, 93-130.
Hadeler, K. P., and Castillo-Chavez, C. (1995). A core group model for disease transmission. Math. Biosci. 128, 41-55.
Hethcote, H. W. (1976). Qualitative analysis for communicable disease models. Math. Biosci. 28, 335-356.
Hethcote, H. W., and Van Ark, J. W. (1987). Epidemiological models for heterogeneous populations: Proportionate mixing, parameter estimation, and immunization programs. Math. Biosci. 84, 85-118.
Hethcote, H W., Stech, H. W., and van den Driessche, P. (1981). Periodicity and stability in epidemic models: A survey. In Busenberg, S., and Cooke, K. L. (eds.), Differential Equations and Applications in Ecology, Epidemics, and Population Problems, Academic Press, New York, pp. 65-82.
Hopewell, P. C. (1994). Overview of clinical tuberculosis. In Bloom, B. R. (ed.), Tuberculosis: Pathogenesis, Protection, and Control, AMS, Washington, DC, pp. 25-46.
Li, M. Y, and Muldowney, J. S. (1995). Global stability for the SEIR model in epidemiology. Math. Biosci. 125, 155-164.
Medical Research Council (1972). BCG and vole bacillus in the prevention of tuberculosis in adolescence and early adult life. Bull. WHO, 46, 3785.
Miller, B. (1993). Preventive therapy for tuberculosis. Med. Clin. North Amer. 77, 1263-1275.
Miller, R. K. (1968). On the linearization of Volterra integral equations. J. Math. Anal. Appl. 23, 198-208.
Miller, R. K. (1971). Nonlinear Volterra Integral Equations, W. A. Benjamin, New York.
Muldowney, J. S. (1990). Compound matrix and ordinary differential equations. Rocky Mont. J. Math. 20, 857-872.
Schenzle, D. (1984). An age-structured model of pre-and post-vaccination measles transmission. IMA J. Math. Appl. Med. Biol. 1, 169-191.
Smith, H. L. (1995). Monotone dynamical systems: An introduction to theory of competitive and cooperative systems. AMS Mathematical Surveys and Monographs 41.
Smith, P. G., and Moss, A. R. (1994). Epidemiology of tuberculosis. In Bloom, B. R. (ed.), Tuberculosis: Pathogenesis, Protection, and Control, AMS, Washington, DC, pp. 47-59.
Stech, H. W., and Williams, M. (1981). Stability in a class of cyclic epidemic models with delay. J. Math. Biol. 11, 95-103.
Thieme, R. H. (1993). Persistence under relaxed point-dissipativity (with applications to an endemic model). SIAM J. Math. Anal. 24, 407-435.
Thieme, R. H., and van den Driessche, P. (1999) Global stability in cyclic epidemic models with disease fatalities. Differential Equations with Application to Biology, Fields Institute Communications, AMS, Vol. 21, pp. 459-472.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Feng, Z., Huang, W. & Castillo-Chavez, C. On the Role of Variable Latent Periods in Mathematical Models for Tuberculosis. Journal of Dynamics and Differential Equations 13, 425–452 (2001). https://doi.org/10.1023/A:1016688209771
Issue Date:
DOI: https://doi.org/10.1023/A:1016688209771