Abstract
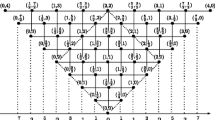
General solutions of relativistic wave equations are studied in terms of functions on the Lorentz group. A close relationship between hyperspherical functions and matrix elements of irreducible representations of the Lorentz group is established. A generalization of the Gel'fand-Yaglom theory for higher-spin equations is given. A two-dimensional complex sphere is associated with each point of Minkowski spacetime. The separation of variables in a general relativistically invariant system is obtained via the hyperspherical functions defined on the surface of the two-dimensional complex sphere. In virtue of this the wave functions are represented in the form of series in hyperspherical functions. Such a description allows one to consider all the physical fields on an equal footing. General solutions of the Dirac and Weyl equations, and also the Maxwell equations in the Majorana-Oppenheimer form, are given in terms of functions on the Lorentz group.
Similar content being viewed by others
REFERENCES
Akhiezer, A. I., and Berestetskii, V. B. (1965). Quantum Electrodynamics, Wiley, New York.
Amar, V., and Dozzio, U. (1972). Gel'fand-Yaglom equations with charge or energy density of definite sign. Nuovo Cimento A 11, 87-99.
Bogoliubov, N. N., and Shirkov, D. V. (1993). Quantum Fields, Nauka, Moscow.
de Broglie, L. (1943). Theorie de particules a spin (methode de fusion), Paris.
Esposito, S. (1998). Covariant Majorana formulation of electrodynamics. Foundations of Physics 28, 231-244.
Gel'fand, I. M., Minlos, R. A., and Ya. Z. Shapiro. (1963). Representations of the Rotation and Lorentz Groups and Their Applications, Pergamon Press, Oxford.
Gel'fand, I. M., and Yaglom, A. M. (1948). General relativistic-invariant equations and infinite-dimensional representations of the Lorentz group. Zhurnal Eksperimental' nai i Teoreticherkoi Fiziki 18, 703-733.
Giannetto, E. (1985). A Majorana-Oppenheimer formulation of quantum electrodynamics. Lettere al Nuovo Cimento 44, 140-144.
Kagan, V. F. (1926). Ueber einige Zahlensysteme, zu denen die Lorentztransformation fürt, Publishing House of Institute of Mathematics, Moscow.
Majorana, E. (unpublished). Scientific Papers, deposited at the “Domus Galileana,” Pisa, quaderno 2, p.101/1; Majorana, E. (unpublished). Scientific Papers, deposited at the “Domus Galileana,” Pisa, quaderno 3, p.11-160; Majorana, E. (unpublished). Scientific Papers, deposited at the “Domus Galileana,” Pisa, quaderno 15, p.16; Majorana, E. (unpublished). Scientific Papers, deposited at the “Domus Galileana,” Pisa, quaderno 17, p. 83-159.
Moses, H. E. (1958). A spinor representation of Maxwell's equations. Nuovo Cimento Supplement 7, 1-18.
Moses, H. E. (1959). Solution of Maxwell's equations in terms of a spinor notation: The direct and inverse problem. Physical Review 113, 1670-1679.
Naimark, M. A. (1964). Linear Representations of the Lorentz Group, Pergamon Press, London.
Oppenheimer, J. R. (1931). Physical Review 38, 725.
Petrov, A. Z. (1969). Einstein Spaces, Pergamon Press, Oxford.
Pletyukhov, V. A., and Strazhev, V. I. (1983). On Dirac-like relativistic wave equations. Russian Journal of Physics 12, 38-41.
Recami, E. (1990). Possible physical meaning of the photon wave-function, according to Ettore Majorana. In Hadronic Mechanics and Non-Potential Interactions, Nova Scientific Publications New York, pp. 231-238.
Rumer, Yu. B., and Fet, A. I. (1977). Group Theory and Quantized Fields [in Russian], Nauka, Moscow.
Ryder, L. (1985). Quantum Field Theory, Cambridge University Press. Cambridge.
Schweber, S. S. (1961). An Introduction to Relativistic Quantum Field Theory, Harper & Row, New York.
Smorodinsky, Ya. A., and Huszar, M. (1970). Representations of the Lorentz group and the generalization of helicity states. Teor. Mat. Fiz. 4(3), 328-340.
Van der Waerden, B. L. (1932). Die Gruppentheoretische Methode in der Quantenmechanik, Springer, Berlin.
Varlamov, V. V. (1999). Fundamental automorphisms of Clifford algebras and an extension of Dabrowski pin groups. Hadronic Journal 22, 497-535.
Varlamov, V. V. (2001a). Discrete symmetries and Clifford algebras. International Journal of Theoretical Physics 40(4), 769-805.
Varlamov, V. V. (2002). About algebraic foundations of Majorana-Oppenheimer quantum electrodynamics and de Broglie-Jordan Neutrino theory of light. Ann. Fond. Broglie 27(2), 273-286.
Varlamov, V. V. (2002b). Hyperspherical functions and linear representations of the Lorentz group. Preprint math-ph/0205013.
Varlamov, V. V. (2002c). A note on the Majorana-Oppenheimer quantum electrodynamics. Preprint math-ph/0206008.
Vilenkin, N. Ya. (1968). Special Functions and the Theory of Group Representations, AMS, Providence, RI.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Varlamov, V.V. General Solutions of Relativistic Wave Equations. International Journal of Theoretical Physics 42, 583–633 (2003). https://doi.org/10.1023/A:1024498001488
Issue Date:
DOI: https://doi.org/10.1023/A:1024498001488