Hostname: page-component-8448b6f56d-jr42d Total loading time: 0 Render date: 2024-04-24T10:11:40.542Z Has data issue: false hasContentIssue false
  • English
  • Français

The zeta function of the beta transformation

Published online by Cambridge University Press:  19 September 2008

Leopold Flatto ,
Jeffrey C. Lagarias  and
Bjorn Poonen
Leopold Flatto
Affiliation:
AT & T Bell Laboratories, Murray Hill, NJ 07974, USA
Jeffrey C. Lagarias
Affiliation:
AT & T Bell Laboratories, Murray Hill, NJ 07974, USA
Bjorn Poonen
Affiliation:
University of California at Berkeley, Berkeley, CA 94720, USA
Get access Rights & Permissions [Opens in a new window]

Abstract

The β-transformation ƒβ(x) = βx(mod 1), for β > 1, has a symbolic dynamics generalizing radix expansions to an integer base. Two important invariants of ƒβ are the (Artin-Mazur) zeta function

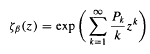
where Pk counts the number of fixed points of , and the lap-counting function 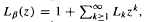where Lk counts the number of monotonic pieces of the kth iterate . For β-transformations these functions are related by ζβ(z) = (1 − z)Lβ(z). The function ζβ(z) is meromorphic in the unit disk, is holomorphic in {z: |z| < 1/β}, has a simple pole at z = 1/β, and has no other singularities with |z| = 1/β. Let M(β) denote the minimum modulus of any pole of ζβ(z) in |z| < 1 other than z = 1/β, and set M(β) = 1 if no other pole exists with |z| < 1. Then Pk = βk + O((M(β)−1+ε)k) for any ε > 0. This paper shows that M(β) is a continuous function, that (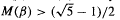 for all β, and that 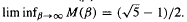An asymptotic formula is derived for M(β) as β → 1+, which implies that M(β) < 1 for all β in an interval (1, 1 + c0). The set 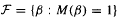is shown to have properties analogous to the set of Pisot numbers. It is closed, totally disconnected, has smallest element ≥ 1 + C0 and contains infinitely many β falling in each interval [n, n + 1) for n ∈ ℤ+. All known members of 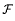are algebraic integers which are either Pisot or Salem numbers.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 14 , Issue 2 , June 1994 , pp. 237 - 266
Copyright
Copyright © Cambridge University Press 1994

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Artin, M. & Mazur, B.. On periodic points. Ann. Math. 81 (1965), 82–99.Google Scholar
[2]Bertrand-Mattas, A., Blanchard, F. & Hansel, G.. Sur la fonction ζ de certain systèmes dynamiques symboliques. Preprint (1992).Google Scholar
[3]Blanchard, F.. β-expansions and symbolic dynamics. Theor. Comput. Sci. 65 (1989), 131–141.Google Scholar
[4]Boyd, D.. Salem numbers of degree four have periodic expansions. In: Théorie des Nombres (Quebec 1987), de Gruyter: Berlin, 1989. pp. 57–64.Google Scholar
[5]Boyd, D.W.. Multiplication modulo one by Salem numbers. Preprint (1992).Google Scholar
[6]Brauer, A.. On algebraic equations with all but one root in the interior of the unit circle. Math. Nachr. 4 (1951), 250–257.Google Scholar
[7]Cantor, D. G.. Power series with integral coefficients. Bull. Amer. Math. Soc. 69 (1963), 362–366.CrossRefGoogle Scholar
[8]Carlson, F.. Über Potenzreihen mit ganzzahlingen Koeffizienten. Math. Z. 9 (1921), 1–13.CrossRefGoogle Scholar
[9]Flatto, L.. Z-numbers and β-transformations. In: Symbolic Dynamics and Its Applications (Walters, P., ed), Contemp. Math. 135 (1992).Google Scholar
[10]Flatto, L. & Lagarias, J. C.. Problem 10351. Amer. Math. Monthly 100 (1993), 952.Google Scholar
[11]Flatto, L. & Lagarias, J. C.. On the range of fractional parts {ξ(p/q)n}. Acta Arithmetica. Submitted.Google Scholar
[12]Frougny, C.. Representation of numbers and finite automata. Math. Sys. Th. 25 (1992), 37–60.Google Scholar
[13]Frougny, C. & Solomyak, B.. Finite β-expansions. Ergod. Th. & Dynam. Sys. 12 (1992), 713–723.Google Scholar
[14]Henrici, P.. Applied and Computational Complex Analysis, Vol. I. John Wiley: New York, 1974.Google Scholar
[15]Hofbauer, F.. β-shifts have unique maximal measures. Monat. Math. 85 (1978), 189–198.Google Scholar
[16]Hofbauer, F. & Keller, G.. Zeta-functions and transfer-operators for piecewise linear transformations. J. reine Angew. 352 (1984), 100–113.Google Scholar
[17]Ito, S. & Takahashi, Y.. Markov subshifts and realization of β-expansions. J. Math. Soc. Japan 26 (1974), 33–55.Google Scholar
[18]Milnor, J. & Thurston, W.. On iterated maps of the interval. In: Springer Lecture Notes in Mathematics 1342, Springer: Berlin, 1988. pp. 465–563.Google Scholar
[19]Odlyzko, A. M. & Poonen, B.. Zeros of polynomials with 0, 1 coefficients. Enseign. Math. 39 (1993), 317–348.Google Scholar
[20]Parry, W.. On the β-expansions of real numbers. Acta. Math. Acad. Sci. Hung. 11 (1960), 401–416.CrossRefGoogle Scholar
[21]Parry, W.. Representations for real numbers. Acta Math. Acad. Sci. Hung. 15 (1964), 95–105.CrossRefGoogle Scholar
[22]Polya, G.. Sur les séries entières à coefficients entiers. Proc. London Math. Soc. 21 (1923), 22–38.Google Scholar
[23]Polya, G. & Szego, G.. Problems and Theorems in Analysis, Vol. II. Springer: Berlin, 1976.Google Scholar
[24]Preston, C.. What you need to know to knead. Adv. Math. 78 (1989), 192–252.CrossRefGoogle Scholar
[25]Renyi, A.. Representations for real numbers and their ergodic properties. Acta Math. Acad. Sci. Hung. 8 (1957), 477–493.CrossRefGoogle Scholar
[26]Salem, R.. Power series with integral coefficients. Duke Math. J. 12 (1945), 153–172.Google Scholar
[27]Schmidt, K.. On periodic expansions of Pisot numbers and Salem numbers. Bull. London Math. Soc. 12 (1980), 269–278.Google Scholar
[28]Solomyak, V.. Substitutions, adic transformations and beta-expansions. In: Symbolic Dynamics and Its Applications, (Walters, P., ed), Contemp. Math. 135 (1992a).Google Scholar
[29]Solomyak, V.. On the spectral theory of adic transformations. Adv. Sov. Math. 9 (1992b), 217–230.Google Scholar
[30]Solomyak, V.. Conjugates of beta-numbers and the zero-free domain for a class of analytic functions. Proc. London Math. Soc. (1994), to appear.Google Scholar
[31]Takahashi, Y.. β-transformations and symbolic dynamics. In: Springer Lecture Notes in Mathematics 330, Springer: Berlin, 1973. pp. 455–464.Google Scholar
[32]Takahashi, Y.. Shift with orbit basis and realization of one-dimensional maps. Osaka Math. J. 20 (1983), 269–278.Google Scholar
[33]Titchmarsh, E. C.. The Theory of Functions, 2nd Edn, Oxford University Press: Oxford, 1939.Google Scholar