Hostname: page-component-848d4c4894-pftt2 Total loading time: 0 Render date: 2024-05-25T06:12:09.748Z Has data issue: false hasContentIssue false
  • English
  • Français

On nonlinear perturbations of linear second order elliptic boundary value problems

Published online by Cambridge University Press:  24 October 2008

P. M. Fitzpatrick
P. M. Fitzpatrick
Affiliation:
University of Maryland
Get access Rights & Permissions [Opens in a new window]

Abstract

Let Ω ⊆ 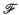n be open and bounded, with ∂Ω smooth. Sufficient conditions on f are given in order that for p > n, the equation

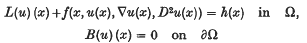
has a solution for every h ∈ Lp; L is a second order symmetric elliptic operator and B represents either the first, second or third boundary value problems. These conditions are in terms of the asymptotic behaviour of f, in its second variable, in relation to those λ for which there is a nontrivial solution of

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 84 , Issue 1 , July 1978 , pp. 143 - 157
Copyright
Copyright © Cambridge Philosophical Society 1978

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Adams, R. A.Sobolev spaces (Academic Press, 1975).Google Scholar
(2)Agmon, S., Douglis, A. and Nirenberg, L.Estimates near the boundary for elliptic partial differential equations satisfying general boundary conditions. Comm. Pure Appl. Math. 12 (1959), 623–727.CrossRefGoogle Scholar
(3)Brézis, H. and Nirenberg, L. Characterizations of the ranges of some nonlinear operators and applications to boundary value problems. (To appear.)Google Scholar
(4)Browder, F. E.Estimates and existence theorems for elliptic boundary value problems. Proc. Natn. Acad. Sci. U.S.A. 45 (1959), 265–372.CrossRefGoogle ScholarPubMed
(5)Cotlar, M. and Cignoli, R.An introduction to functional analysis (North Holland/American Elsevier, 1974).Google Scholar
(6)Cronin, J.Equations with unbounded linearities. J. Differential Equations 14 (1973), 581–596.CrossRefGoogle Scholar
(7)Figueiredo, D. De, The range of nonlinear operators whose linear asymptotes are not invertible. Comment Math. Univ. Carolinae 15 (1974), 415–428.Google Scholar
(8)Fitzpatrick, P. M.Existence results for equations involving noncompact perturbations of Fredholm mappings with applications to differential equations. J. Math. Anal. Appl. (to appear).Google Scholar
(9)Fucik, S., Kucera, M. and Necas, J.Ranges of nonlinear asymptotically linear operators. J. Differential Equations 17 (1975), 375–394.CrossRefGoogle Scholar
(10)Hess, P.On a theorem of Landesman and Lazer. Indiana Univ. Math. J. 23 (1974), 827–830.CrossRefGoogle Scholar
(11)Hetzer, G.Some remarks on φ+-operators and on the coincidence degree for a Fredholm equation with nonlinear perturbation. Ann. Soc. Sci. Bruxelles 89 (1975), 497–508.Google Scholar
(12)Kazden, J. and Warner, F.Remarks on some quasi-linear elliptic equations. Comm. Pure Appl. Math. 28 (1975), 567–597.CrossRefGoogle Scholar
(13)Krasnoselski˘, M. A.Topological methods in the theory of nonlinear integral equations (Pergamon Press, 1964).Google Scholar
(14)Ladyzhenskaia, O. A. and Ubaltseva, N. N.Linear and quasilinear elliptic equations (New York: Academic Press, 1968).Google Scholar
(15)Landesman, E. and Lazer, A.Nonlinear perturbations of linear elliptic boundary value problems at resonance. J. Math. Mech. 19 (1970), 609–623.Google Scholar
(16)Mahwin, J.Existence theorems for nonlinear operator equations and coincidence degree theory for some mappings in locally convex topological vector spaces. J. Differential Equations 12 (1972), 610–636.Google Scholar
(17)Nirenberg, L.An application of generalized degree to a class of nonlinear problems, Troisième Collog. Anal. Funct., Liège, Sept. 1970. Centre Beige de Rech. Math., Vander (1971), 55–71.Google Scholar
(18)Nussbaum, R. D.The fixed point index for local condensing maps. Ann. Mat. Pura Appl. 89 (1970), 217–258.CrossRefGoogle Scholar
(19)Protter, M. H. and Weinberger, H. F.Maximum principles in differential equations (Clifton N.J.: Prentice Hall, 1967).Google Scholar
(20)Sadovski˘, B. N.On a fixed-point principle. Functional Anal. Appl. 2 (1967).Google Scholar
(21)Sadovski˘, B. N.Ultimately compact and condensing mappings. Uspehi Mat. Nauk 27 (1972), 81–146.Google Scholar