Abstract
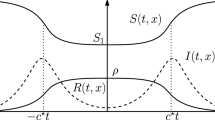
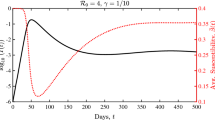
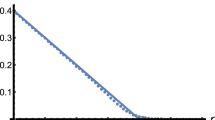
Stochastic differential equations that model an SIS epidemic with multiple pathogen strains are derived from a system of ordinary differential equations. The stochastic model assumes there is demographic variability. The dynamics of the deterministic model are summarized. Then the dynamics of the stochastic model are compared to the deterministic model. In the deterministic model, there can be either disease extinction, competitive exclusion, where only one strain persists, or coexistence, where more than one strain persists. In the stochastic model, all strains are eventually eliminated because the disease-free state is an absorbing state. However, if the population size and the initial number of infected individuals are sufficiently large, it may take a long time until all strains are eliminated. Numerical simulations of the stochastic model show that coexistence cases predicted by the deterministic model are an unlikely occurrence in the stochastic model even for short time periods. In the stochastic model, either disease extinction or competitive exclusion occur. The initial number of infected individuals, the basic reproduction numbers, and other epidemiological parameters are important determinants of the dominant strain in the stochastic epidemic model.
Similar content being viewed by others
References
Ackleh, A. S. and L. J. S. Allen (2003a). Competitive exclusion and coexistence for pathogens in an epidemic model with variable population size. J. Math. Biol. 47, 153–168.
Ackleh, A. S. and L. J. S. Allen (2003b). Competitive exclusion in SIS and SIR epidemic models with total cross immunity and density-dependent host mortality (submitted for publication).
Allen, E. J. (1999). Stochastic differential equations and persistence time for two interacting populations. Dyn. Cont., Discrete Impulsive Syst. 5, 271–281.
Allen, L. J. S. (2003). An Introduction to Stochastic Processes with Applications to Biology, Upper Saddle River, NJ: Prentice-Hall.
Allen, L. J. S. and E. J. Allen (2003). A comparison of three different stochastic population models with regard to persistence time. Theor. Popul. Biol. 64, 439–449.
Allen, L. J. S. and A. M. Burgin (2000). Comparison of deterministic and stochastic SIS and SIR models in discrete time. Math. Biosci. 163, 1–31.
Allen, L. J. S., M. Langlais and C. J. Phillips (2003). The dynamics of two viral infections in a single host population with applications to hantavirus. Math. Biosci. 186, 191–217.
Andreasen, V., J. Lin and S. A. Levin (1997). The dynamics of cocirculating influenza strains conferring partial cross-immunity. J. Math. Biol. 35, 825–842.
Andreasen, V. and A. Pugliese (1995). Pathogen coexistence induced by density dependent host mortality. J. Theor. Biol. 177, 159–165.
Arrigoni, F. and A. Pugliese (2002). Limits of a multi-patch SIS epidemic model. J. Math. Biol. 45, 419–440.
Bailey, N. T. J. (1975). The Mathematical Theory of Infectious Diseases and its Applications, London: Charles Griffin.
Bailey, N. T. J. (1990). The Elements of Stochastic Processes with Applications to the Natural Sciences, New York: John Wiley & Sons.
Ball, F. G. (1999). Stochastic and deterministic models for SIS epidemics among a population partitioned into households. Math. Biosci. 156, 41–67.
Ball, F. G. and P. D. O’Neill (1999). The distribution of general final state random variables for stochastic epidemic models. J. Appl. Prob. 36, 473–491.
Bartlett, M. S. (1960). Stochastic Population Models in Ecology and Epidemiology, New York and London: John Wiley.
Bremermann, H. J. and H. R. Thieme (1989). A competitive exclusion principle for pathogen virulence. J. Math. Biol. 27, 179–190.
Castillo-Chavez, C., W. Huang and J. Li (1996). Competitive exclusion in gonorrhea models and other sexually transmitted diseases. SIAM J. Appl. Math. 56, 494–508.
Castillo-Chavez, C., W. Huang and J. Li (1999). Competitive exclusion and coexistence of multiple strains in an SIS STD model. SIAM J. Appl. Math. 59, 1790–1811.
Castillo-Chavez, C. and J. X. Velasco-Hernández (1998). On the relationship between evolution of virulence and host demography. J. Theor. Biol. 192, 437–444.
Chan, M.-S., F. Mutapi, M. E. J. Woolhouse and V. S. Isham (2000). Stochastic simulation and the detection of immunity to helminth infections. Parasitology 120, 161–169.
Daley, D. J. and J. Gani (1999). Epidemic Modelling: An Introduction, Cambridge Studies in Mathematical Biology, Cambridge: Cambridge University Press.
Dawes, J. H. P. and J. R. Gog (2002). The onset of oscillatory dynamics in models of multiple disease strains. J. Math. Biol. 45, 471–510.
Esteva, L. and C. Vargas (2003). Coexistence of different serotypes of dengue virus. J. Math. Biol. 46, 31–47.
Feng, Z. and J. X. Velasco-Hernández (1997). Competitive exclusion in a vector-host model for the dengue fever. J. Math. Biol. 35, 523–544.
Ferguson, N. M., A. P. Galvani and R. M. Bush (2003). Ecological and immunological determinants of influenza evolution. Nature 422, 428–433.
Finkenstadt, B. F., O. N. Bjornstad and B. T. Grenfell (2002). A stochastic model for extinction and recurrence of epidemics: estimation and inference for measles outbreaks. Biostatistics 3, 4, 493–510.
Gabriel, J.-P., C. Lefèvre and P. Picard (Eds), (1990). Stochastic Processes in Epidemic Theory, Lecture Notes in Biomathematics, New York: Springer-Verlag.
Gard, T. C. (1988). Introduction to Stochastic Differential Equations, NewYork and Basel: Marcel Dekker, Inc.
Gupta, S., K. Trenholme, R. M. Anderson and K. P. Day (1994). Antigenic diversity and the transmission dynamics of Plasmodium falciparum. Science 263, 961–963.
Herbert, J. and V. S. Isham (2000). On stochastic host-parasite interaction models. J. Math. Biol. 40, 343–371.
Hernandez-Suarez, C. M. (2002). A Markov Chain approach to calculate R 0 in stochastic epidemic models. J. Theor. Biol. 215, 83–93.
Hochberg, M. E. and R. D. Holt (1990). The coexistence of competing parasites I. The role of cross species infection. Am. Nat. 136, 517–541.
Isham, V. and M.-S. Chan (1998). A stochastic model of schistosomiasis immunoepidemiology. Math. Biosci. 151, 179–198.
Isham, V. and G. Medley (Eds), (1996). Models for Infectious Human Diseases: Their Structure and Relation to Data, Cambridge: Cambridge University Press.
Jacquez, J. A. and C. P. Simon (1993). The stochastic SI model with recruitment and deaths I. comparison with the closed SIS model. Math. Biosci. 117, 77–125.
Karlin, S. and H. Taylor (1981). A Second Course in Stochastic Processes, New York: Academic Press.
Keeling, M. J., M. E. J. Woolhouse, D. J. Shaw, L. Matthews, M. Chase-Topping, D. T. Haydon, S. J. Cornell, J. Kappey, J. Wilesmith and B. T. Grenfell (2001). Dynamics of the 2001 UK foot and mouth epidemic: stochastic dispersal in a heterogeneous landscape. Science 294, 813–817.
Kirupaharan, N. (2003). Deterministic and stochastic epidemic models with multiple pathogens, PhD Thesis, Texas Tech University, Lubbock, TX, USA.
Kloeden, P. E. and E. Platen (1992). Numerical Solution of Stochastic Differential Equations, New York: Springer.
Kloeden, P. E., E. Platen and H. Schurz (1997). Numerical Solution of Stochastic Differential Equations through Computer Experiments, Berlin: Springer.
Levin, S. A. (1970). Community equilibria and stability, and an extension of the competitive exclusion principle. Am. Nat. 104, 413–423.
Matis, J. H. and T. R. Kiffe (2000). Stochastic Population Models, New York: Springer.
May, R. M. and M. A. Nowak (1994). Superinfection, metapopulation dynamics, and the evolution of virulence. J. Theor. Biol. 170, 95–114.
May, R. M. and M. A. Nowak (1995). Coinfection and the evolution of parasite virulence. Proc. R. Soc. Lond. B 261, 209–215.
Mena-Lorca, J., J. Velasco-Hernández and C. Castillo-Chavez (1999). Density-dependent dynamics and superinfection in an epidemic model. IMA J. Math. Appl. Med. Biol. 16, 307–317.
Mollison, D. (1995). Epidemic Models Their Structure and Relation to Data, Cambridge: Cambridge University Press.
Mosquera, J. and F. R. Adler (1998). Evolution of virulence: a unified framework for coinfection and superinfection. J. Theor. Biol. 195, 293–313.
Nåsell, I. (1996). The quasi-stationary distribution of the closed endemic SIS model. Adv. Appl. Prob. 28, 895–932.
Nåasell, I. (1999). On the quasi-stationary distribution of the stochastic logistic epidemic. Math. Biosci. 156, 21–40.
Nåsell, I. (2002). Endemicity, persistence, and quasi-stationarity, in Mathematical Approaches for Emerging and Reemerging Infectious Diseases An Introduction, C. Castillo-Chavez, S. Blower, P. van den Driessche, D. Kirschner and A.-A. Yakubu (Eds), New York: Springer, pp. 199–227.
Nowak, M. A. and R. M. May (1994). Superinfection and the evolution of parasite virulence. Proc. R. Soc. Lond. B 255, 81–89.
Ortega, J. M. (1987). Matrix Theory, New York and London: Plenum Press.
Pugliese, A. (2002). On the evolutionary coexistence of parasite strains. Math. Biosci. 177, 178, 355–375.
Renshaw, E. (1993). Modelling Biological Populations in Space and Time, Cambridge: Cambridge University Press.
Rohani, P., M. J. Keeling and B. T. Grenfell (2002). The interplay between determinism and stochasticity in childhood diseases. Am. Nat. 159, 469–481.
Swinton, J. (1998). Extinction times and phase transitions for spatially structured closed epidemics. Bull. Math. Biol. 60, 215–230.
Taylor, H. M. and S. Karlin (1998). An Introduction to Stochastic Modeling, 3rd edn, New York: Academic Press.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Kirupaharan, N., Allen, L.J.S. Coexistence of multiple pathogen strains in stochastic epidemic models with density-dependent mortality. Bull. Math. Biol. 66, 841–864 (2004). https://doi.org/10.1016/j.bulm.2003.11.007
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1016/j.bulm.2003.11.007