Skip to main content
SpringerLink
Log in

Application of the mixed formulation method to eliminate shear-locking phenomenon in the Peridynamic Mindlin plate model

  • Published:
Computational Particle Mechanics Aims and scope Submit manuscript

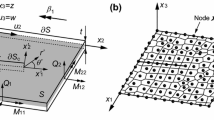
Abstract

Accurately predicting crack propagation in structures remains a key challenge within the finite element method (FEM) framework. Despite developing the local method to mitigate the challenges encountered in the FEM, this method is computationally demanding and inadequate for accurately modeling fracture processes in actual structures. Recently, a powerful nonlocal method, Peridynamics (PD), has been proposed by employing integral equations rather than differential equations to address various discontinuous problems. This study introduces the classical Peridynamic Mindlin plate theory (classical PD) to characterize the kinematics of thick plates. However, the application of the classical PD to extremely thin plate structures results in a shear-locking phenomenon, causing inaccuracies in the solutions. Although the reduced integration with a single-point rule provides an alternative solution to address the shear-locking problem, its sensitivity to the point number in the horizon becomes especially pronounced in the case of discontinuities. To ensure stability and broad applicability, this paper presents a more general mixed formulation method (mixed PD) that can yield accurate results ranging from very thick to thin plate configurations. The efficacy of the mixed PD is demonstrated via several numerical cases.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14

Similar content being viewed by others

References

  1. Onyibo EC, Safaei B (2022) Application of finite element analysis to honeycomb sandwich structures: a review. Rep Mech Eng 3(1):192–209. https://doi.org/10.31181/rme20023032022o

    Article  Google Scholar 

  2. Jafari A, Broumand P, Vahab M, Khalili N (2022) An extended finite element method implementation in comsol multiphysics: solid mechanics. Finite Elem Anal Des 202:103707. https://doi.org/10.1016/j.finel.2021.103707

    Article  MathSciNet  Google Scholar 

  3. Wang Y, Gao F, Cui J (2022) A conforming discontinuous Galerkin finite element method for elliptic interface problems. J Comput Appl Math 412:114304. https://doi.org/10.1016/j.cam.2022.114304

    Article  MathSciNet  MATH  Google Scholar 

  4. Ahn C, Nishizawa Y, Choi W (2020) A finite element method to simulate dislocation stress: a general numerical solution for inclusion problems. AIP Adv 10(1):015111. https://doi.org/10.1063/1.5121149

    Article  Google Scholar 

  5. Borzabadi Farahani E, Sobhani Aragh B, Voges J, Juhre D (2021) On the crack onset and growth in martensitic micro-structures; a phase-field approach. Int J Mech Sci 194:106187. https://doi.org/10.1016/j.ijmecsci.2020.106187

    Article  Google Scholar 

  6. Cockburn B, Karniadakis GE, Shu C-W (2012) Discontinuous Galerkin methods: theory, computation and applications, vol 11. Springer, Berlin. https://doi.org/10.1007/978-3-642-59721-3

    Book  Google Scholar 

  7. BaniHani SM, Al-Oqla FM, Hayajneh M, Mutawe S, Almomani T (2022) A new approach for dynamic crack propagation modeling based on meshless Galerkin method and visibility based criterion. Appl Math Model 107:1–19. https://doi.org/10.1016/j.apm.2022.02.010

    Article  MathSciNet  MATH  Google Scholar 

  8. Liu GR, Dai KY, Nguyen THOIT (2007) A smoothed finite element method for mechanics problems. Comput Mech 39:859–877. https://doi.org/10.1007/s00466-006-0075-4

    Article  MATH  Google Scholar 

  9. Long T, Huang C, Dean H, Liu M (2021) Coupling edge-based smoothed finite element method with smoothed particle hydrodynamics for fluid structure interaction problems. Ocean Eng 225:108772. https://doi.org/10.1016/j.engfracmech.2020.107476

    Article  Google Scholar 

  10. Elices MGGV, Guinea GV, Gomez J, Planas J (2002) The cohesive zone model: advantages, limitations and challenges. Eng Fract Mech 69(2):137–163. https://doi.org/10.1016/s0013-7944(01)00083-2

    Article  Google Scholar 

  11. Agathos K, Bordas SPA, Chatzi E (2019) Improving the conditioning of XFEM/GFEM for fracture mechanics problems through enrichment quasi-orthogonalization. Comput Methods Appl Mech Eng 346:1051–1073. https://doi.org/10.1016/j.cma.2018.08.007

    Article  MathSciNet  MATH  Google Scholar 

  12. Alder BJ, Wainwright TE (1959) Studies in molecular dynamics. I. General method. J Chem Phys 31(2):459–466. https://doi.org/10.1063/1.1730376

    Article  MathSciNet  Google Scholar 

  13. Xiong S, Cao G (2015) Molecular dynamics simulations of mechanical properties of monolayer mos2. Nanotechnology 26(18):185705. https://doi.org/10.1088/0957-4484/26/18/185705

    Article  Google Scholar 

  14. Hospital A, Goi JR, Orozco M, Gelp JL (2015) Molecular dynamics simulations: advances and applications. Adv Appl Bioinform Chem AABC 8:37. https://doi.org/10.2147/AABC.S70333

    Article  Google Scholar 

  15. Wan S, Sinclair RC, Coveney PV (2021) Uncertainty quantification in classical molecular dynamics. Philos Trans R Soc A 379(2197):20200082. https://doi.org/10.5772/intechopen.68507

    Article  Google Scholar 

  16. Hillman M, Pasetto M, Zhou G (2020) Generalized reproducing kernel peridynamics: unification of local and non-local meshfree methods, non-local derivative operations, and an arbitrary-order state-based peridynamic formulation. Comput Part Mech 7:435–469. https://doi.org/10.1007/s40571-019-00266-9

    Article  Google Scholar 

  17. Patnaik S, Sidhardh S, Semperlotti F (2021) Towards a unified approach to nonlocal elasticity via fractional-order mechanics. Int J Mech Sci 189:105992. https://doi.org/10.1016/j.ijmecsci.2020.105992

    Article  MATH  Google Scholar 

  18. Patnaik S, Sidhardh S, Semperlotti F (2021) Displacement-driven approach to nonlocal elasticity. arXiv preprint arXiv:2104.05818. https://doi.org/10.1016/j.euromechsol.2021.104434

  19. Voyiadjis GZ (2019) Handbook of nonlocal continuum mechanics for materials and structures. Springer, Berlin. https://doi.org/10.1007/978-3-319-22977-5

    Book  Google Scholar 

  20. Oterkus E, Oterkus S, Madenci E (2021) Peridynamic modeling, numerical techniques, and applications. Elsevier, Berlin. https://doi.org/10.1016/c2019-0-01174-0

    Book  MATH  Google Scholar 

  21. Blanc N, Frank X, Radjai F, Mayer-Laigle C, Delenne J-Y (2021) Breakage of flawed particles by peridynamic simulations. Comput Part Mech. https://doi.org/10.1007/s40571-021-00390-5

    Article  Google Scholar 

  22. Bai R, Naceur H, Liang G, Zhao J, Yi J, Li X, Yuan S, Huayan P, Luo J (2023) Alleviation of shear locking in the peridynamic timoshenko beam model using the developed mixed formulation method. Comput Part Mech 10(3):627–643. https://doi.org/10.1007/s40571-022-00517-2

    Article  Google Scholar 

  23. Silling SA, Epton M, Weckner O, Ji X, Askari E (2007) Peridynamic states and constitutive modeling. J Elast 88(2):151–184. https://doi.org/10.1007/s10659-007-9125-1

    Article  MathSciNet  MATH  Google Scholar 

  24. Silling SA, Lehoucq RB (2010) Peridynamic theory of solid mechanics. Adv Appl Mech 44:73–168. https://doi.org/10.1016/s0065-2156(10)44002-8

    Article  Google Scholar 

  25. Youn Doh Ha and Florin Bobaru (2010) Studies of dynamic crack propagation and crack branching with peridynamics. Int J Fract 162:229–244. https://doi.org/10.1007/s10704-010-9442-4

    Article  MATH  Google Scholar 

  26. Giannakeas IN (2020) Peridynamic and finite element coupling strategies for the simulation of brittle fracture. Ph.D. thesis, Brunel University London

  27. Silling SA, Lehoucq RB (2008) Convergence of peridynamics to classical elasticity theory. J Elast 93(1):13. https://doi.org/10.1007/s10659-008-9163-3

    Article  MathSciNet  MATH  Google Scholar 

  28. Ugur Yolum and Mehmet Ali Güler (2020) On the peridynamic formulation for an orthotropic mindlin plate under bending. Math Mech Solids 25(2):263–287. https://doi.org/10.1177/1081286519873694

    Article  MathSciNet  MATH  Google Scholar 

  29. Naumenko K, Eremeyev VA (2022) A non-linear direct peridynamics plate theory. Compos Struct 279:114728. https://doi.org/10.1016/j.compstruct.2021.114728

    Article  Google Scholar 

  30. Bazazzadeh S, Zaccariotto M, Galvanetto U (2019) Fatigue degradation strategies to simulate crack propagation using peridynamic based computational methods. Latin Am J Solids Struct. https://doi.org/10.1590/1679-78255022

    Article  Google Scholar 

  31. Giannakeas IN, Papathanasiou TK, Fallah AS, Bahai H (2020) Coupling XFEM and peridynamics for brittle fracture simulation: feasibility and effectiveness. Comput Mech 66(1):103–122. https://doi.org/10.1007/s00466-020-01843-z

    Article  MathSciNet  MATH  Google Scholar 

  32. Pagani A, Enea M, Carrera E (2022) Quasi-static fracture analysis by coupled three-dimensional peridynamics and high order one-dimensional finite elements based on local elasticity. Int J Numer Methods Eng 123(4):1098–1113. https://doi.org/10.1002/nme.6890

    Article  MathSciNet  Google Scholar 

  33. Pagani A, Carrera E (2020) Coupling three-dimensional peridynamics and high-order one-dimensional finite elements based on local elasticity for the linear static analysis of solid beams and thin-walled reinforced structures. Int J Numer Methods Eng 121(22):5066–5081. https://doi.org/10.1002/nme.6510

    Article  MathSciNet  Google Scholar 

  34. Diyaroglu C, Oterkus E, Oterkus S, Madenci E (2015) Peridynamics for bending of beams and plates with transverse shear deformation. Int J Solids Struct 69:152–168. https://doi.org/10.1016/j.ijsolstr.2015.04.040

    Article  Google Scholar 

  35. Yang Z, Oterkus E, Nguyen CT, Oterkus S (2019) Implementation of peridynamic beam and plate formulations in finite element framework. Continuum Mech Thermodyn 31:301–315. https://doi.org/10.1007/s00161-018-0684-0

    Article  MathSciNet  Google Scholar 

  36. Bai R, Liang G, Naceur H, Zhao J, Yi J, Luo J, Wang L, Huayan P (2023) Locking alleviation technique for the peridynamic Reissner-Mindlin plate model: the developed reduced integration method. Arch Appl Mech 93(3):1167–1188. https://doi.org/10.1007/s00419-022-02320-0

    Article  Google Scholar 

  37. Yang Z, Oterkus E, Oterkus S (2021) A novel peridynamic mindlin plate formulation without limitation on material constants. J Peridyn Nonlocal Model 3:287–306. https://doi.org/10.1007/s42102-021-00050-5

    Article  MathSciNet  MATH  Google Scholar 

  38. Carpenter N, Belytschko T, Stolarski H (1986) Locking and shear scaling factors in co bending elements. Comput Struct 22(1):39–52. https://doi.org/10.1016/0045-7949(86)90083-0

    Article  MATH  Google Scholar 

  39. Hernández E, Vellojin J (2021) A locking-free finite element formulation for a non-uniform linear viscoelastic Timoshenko beam. Comput Math Appl 99:305–322. https://doi.org/10.1016/j.camwa.2021.08.014

    Article  MathSciNet  MATH  Google Scholar 

  40. Mukherjee S, Prathap G (2001) Analysis of shear locking in Timoshenko beam elements using the function space approach. Commun Numer Methods Eng 17(6):385–393. https://doi.org/10.1002/cnm.413

    Article  MATH  Google Scholar 

  41. Ping H, Qingyuan H, Xia Y (2016) Order reduction method for locking free isogeometric analysis of Timoshenko beams. Comput Methods Appl Mech Eng 308:1–22. https://doi.org/10.1016/j.cma.2016.05.010

    Article  MathSciNet  MATH  Google Scholar 

  42. Bode T, Weißenfels C, Wriggers P (2020) Mixed peridynamic formulations for compressible and incompressible finite deformations. Comput Mech 65:1365–1376. https://doi.org/10.1007/s00466-020-01824-2

    Article  MathSciNet  MATH  Google Scholar 

  43. Simo JC, Taylor RL, Pister KS (1985) Variational and projection methods for the volume constraint in finite deformation elasto-plasticity. Comput Methods Appl Mech Eng 51(1–3):177–208. https://doi.org/10.1016/0045-7825(85)90033-7

    Article  MathSciNet  MATH  Google Scholar 

  44. Aldakheel F, Hudobivnik B, Soleimani M, Wessels H, Weissenfels C, Marino M (2022) Current trends and open problems in computational mechanics. Springer, Berlin

    Book  MATH  Google Scholar 

  45. Bai R, Naceur H, Liang G, Zhao J, Yi J, Li X, Yuan S, Pu H, Luo J (2022) Alleviation of shear locking in the peridynamic timoshenko beam model using the developed mixed formulation method. Computat Part Mech. https://doi.org/10.1007/s40571-022-00517-2

    Article  Google Scholar 

  46. Oñate E (2013) Structural analysis with the finite element method. Linear statics: volume 2: beams, plates and shells. Springer, Berlin. https://doi.org/10.1007/978-1-4020-8743-1

    Book  MATH  Google Scholar 

  47. Bai R, Naceur H, Zhao J, Yi J, Ma J, Pu H, Luo J (2023) Improved numerical integration for locking treatment in the Peridynamic Timoshenko beam model. Eng Comput. https://doi.org/10.1108/EC-07-2022-0442

  48. Ma H, Zhou J, Liang G (2014) Implicit damping iterative algorithm to solve elastoplastic static and dynamic equations. J Appl Math. https://doi.org/10.1155/2014/486171

    Article  MathSciNet  MATH  Google Scholar 

  49. Zhao M, Li H, Cao S, Xiuli D (2018) An explicit time integration algorithm for linear and non-linear finite element analyses of dynamic and wave problems. Eng Comput. https://doi.org/10.1108/ec-07-2018-0312

    Article  Google Scholar 

  50. Silling SA, Askari E (2005) A meshfree method based on the peridynamic model of solid mechanics. Comput Struct 83(17–18):1526–1535. https://doi.org/10.1016/j.compstruc.2004.11.026

    Article  Google Scholar 

  51. Wang B, Oterkus S, Oterkus E (2020) Determination of horizon size in state-based peridynamics. Continuum Mech Thermodyn. https://doi.org/10.1007/s00161-020-00896-y

    Article  MATH  Google Scholar 

  52. Seleson P, Beneddine S, Prudhomme S (2013) A force-based coupling scheme for peridynamics and classical elasticity. Comput Mater Sci 66:34–49. https://doi.org/10.1016/j.commatsci.2012.05.016

    Article  Google Scholar 

  53. Hui W, Qing-Hua Q (2019) Methods of fundamental solutions in solid mechanics. Elsevier, New York. https://doi.org/10.1016/c2018-0-03684-1

Download references

Acknowledgements

This research is funded by the Entrepreneurship and Innovation Support Program for Chongqing Overseas Returnees (cx2023003), Key Project of Chongqing Natural Science Foundation (cstc2020jcyj-zdxmX0014), Shuguang Program (18SG36), National Natural Science Foundation of China (62303079, 52105244, 62103065), and Fundamental Research Funds for the Central Universities (2023CDJXY-014, 02090052020106).

Author information

Authors and Affiliations

  1. State Key Laboratory of Mechanical Transmission for Advanced Equipment, Chongqing University, Chongqing, 400044, China

    Ruqing Bai, Jinglei Zhao, Jin Yi, Xueping Li, Shujin Yuan, Jun Luo, Liming Wang & Huayan Pu

  2. INSA Hauts-de-France, CNRS UMR 8201-LAMIH, 59313, Valenciennes, France

    Hakim Naceur

Authors
  1. Ruqing Bai
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hakim Naceur
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jinglei Zhao
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Jin Yi
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Xueping Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Shujin Yuan
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. Jun Luo
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. Liming Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  9. Huayan Pu
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

All authors contributed to the study conception and design. Material preparation, data collection and analysis were performed by Ruqing Bai and Hakim Naceur. The first draft of the manuscript was written by Ruqing Bai and all authors commented on previous versions of the manuscript. All authors read and approved the final manuscript.

Corresponding authors

Correspondence to Hakim Naceur or Huayan Pu.

Ethics declarations

Competing Interests

The authors state that this article has no conflict of interest with anybody or any organizations.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bai, R., Naceur, H., Zhao, J. et al. Application of the mixed formulation method to eliminate shear-locking phenomenon in the Peridynamic Mindlin plate model. Comp. Part. Mech. (2023). https://doi.org/10.1007/s40571-023-00677-9

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s40571-023-00677-9

Keywords

Search

Navigation