Skip to main content
SpringerLink
Log in

Bifurcation and chaos in a discrete-time fractional-order logistic model with Allee effect and proportional harvesting

  • Published:
International Journal of Dynamics and Control Aims and scope Submit manuscript

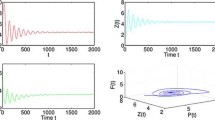
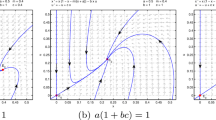
Abstract

The Allee effect and harvesting always get a pivotal role in studying the preservation of a population. In this context, we consider a Caputo fractional-order logistic model with the Allee effect and proportional harvesting. In particular, we implement the piecewise constant arguments (PWCA) method to discretize the fractional model. The dynamics of the obtained discrete-time model are then analyzed. Fixed points and their stability conditions are established. We also show the existence of saddle-node and period-doubling bifurcations in the discrete-time model. These analytical results are then confirmed by some numerical simulations via bifurcation, Cobweb, and maximal Lyapunov exponent diagrams. The occurrence of period-doubling bifurcation route to chaos is also observed numerically. Finally, the occurrence of period-doubling bifurcation is successfully controlled using a hybrid control strategy.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Data availability

Not applicable.

Code availability

Not applicable.

References

  1. Huang J, Liu S, Ruan S, Xiao D (2018) Bifurcations in a discrete predator-prey model with nonmonotonic functional response. J Math Anal Appl 464(1):201–230. https://doi.org/10.1016/j.jmaa.2018.03.074

    Article  MathSciNet  MATH  Google Scholar 

  2. Santra PK, Mahapatra GS (2020) Dynamical study of discrete-tme prey-predator model with constant prey refuge under imprecise biological parameters. J Biol Syst 28(03):681–699. https://doi.org/10.1142/S0218339020500114

    Article  MATH  Google Scholar 

  3. Singh A, Deolia P (2020) Dynamical analysis and chaos control in discrete-time prey-predator model. Commun Nonlinear Sci Numer Simul 90:105313. https://doi.org/10.1016/j.cnsns.2020.105313

    Article  MathSciNet  MATH  Google Scholar 

  4. Ackleh AS, Hossain MI, Veprauskas A, Zhang A (2020) Long-term dynamics of discrete-time predator-prey models: stability of equilibria, cycles and chaos. J Differ Equ Appl. https://doi.org/10.1080/10236198.2020.1786818

    Article  MathSciNet  MATH  Google Scholar 

  5. Alzabut J, Selvam AGM, Dhakshinamoorthy V, Mohammadi H, Rezapour S (2022) On chaos of discrete time fractional order host-immune-tumor cells interaction model. J Appl Math Comput. https://doi.org/10.1007/s12190-022-01715-0

    Article  MathSciNet  MATH  Google Scholar 

  6. Kartal S, Gurcan F (2019) Discretization of conformable fractional differential equations by a piecewise constant approximation. Int J Comput Math 96(9):1849–1860. https://doi.org/10.1080/00207160.2018.1536782

    Article  MathSciNet  MATH  Google Scholar 

  7. Nosrati K, Shafiee M (2018) Fractional-order singular logistic map: stability, bifurcation and chaos analysis. Chaos Solitons Fractals 115:224–238. https://doi.org/10.1016/j.chaos.2018.08.023

    Article  MathSciNet  MATH  Google Scholar 

  8. Wu GC, Baleanu D (2014) Discrete fractional logistic map and its chaos. Nonlinear Dyn 75(1–2):283–287. https://doi.org/10.1007/s11071-013-1065-7

    Article  MathSciNet  MATH  Google Scholar 

  9. Ji YD, Lai L, Zhong SC, Zhang L (2018) Bifurcation and chaos of a new discrete fractional-order logistic map. Commun Nonlinear Sci Numer Simul 57:352–358. https://doi.org/10.1016/j.cnsns.2017.10.009

    Article  MathSciNet  MATH  Google Scholar 

  10. Munkhammar J (2013) Chaos in a fractional order logistic map. Fract Calc Appl Anal 16(3):511–519. https://doi.org/10.2478/s13540-013-0033-8

    Article  MathSciNet  MATH  Google Scholar 

  11. Panigoro HS, Rahmi E (2021) The dynamics of a discrete fractional-order logistic growth model with infectious disease. Contemp Math Appl 3(1):1–18. https://doi.org/10.20473/conmatha.v3i1.26938

    Article  Google Scholar 

  12. Allen LJS (1994) Some discrete-time SI, SIR, and SIS epidemic models. Math Biosci 124(1):83–105. https://doi.org/10.1016/0025-5564(94)90025-6

    Article  MATH  Google Scholar 

  13. Shi R, Chen L (2007) Stage-structured impulsive SI model for pest management. Discret Dyn Nat Soc. https://doi.org/10.1155/2007/97608

    Article  MathSciNet  MATH  Google Scholar 

  14. Panigoro HS, Rahmi E, Achmad N, Mahmud SL, Resmawan R, Nuha AR A discrete-time fractional-order Rosenzweig-Macarthur predator-prey model involving prey refuge. Commun Math Biol Neurosci. https://doi.org/10.28919/cmbn/6586

  15. Ernawati PD, Darti I (2015) Stability analysis of the euler discretization for the harvesting Leslie-Gower predator-prey model. Int J Pure Appl Math 105(2):213–221. https://doi.org/10.12732/ijpam.v105i2.8

    Article  Google Scholar 

  16. Yousef F, Semmar B, Al Nasr K (2022) Dynamics and simulations of discretized Caputo-conformable fractional-order Lotka-Volterra models. Nonlinear Eng 11(1):100–111. https://doi.org/10.1515/nleng-2022-0013

    Article  Google Scholar 

  17. Shabbir MS, Din Q, Alabdan R, Tassaddiq A, Ahmad K (2020) Dynamical complexity in a class of novel discrete-time predator-prey interaction with cannibalism. IEEE Access 8:100226–100240. https://doi.org/10.1109/ACCESS.2020.2995679

    Article  Google Scholar 

  18. Zhao M, Xuan Z, Li C (2016) Dynamics of a discrete-time predator-prey system. Differ Equ. https://doi.org/10.1186/s13662-016-0903-6

    Article  MATH  Google Scholar 

  19. Hu D, Cao H (2015) Bifurcation and chaos in a discrete-time predator-prey system of holling and leslie type. Commun Nonlinear Sci Numer Simul 22(1–3):702–715. https://doi.org/10.1016/j.cnsns.2014.09.010

    Article  MathSciNet  MATH  Google Scholar 

  20. Parsamanesh M, Erfanian M, Mehrshad S (2020) Stability and bifurcations in a discrete-time epidemic model with vaccination and vital dynamics. BMC Bioinform 21(1):1–15. https://doi.org/10.1186/s12859-020-03839-1

    Article  Google Scholar 

  21. Santra PK (2021) Fear effect in discrete prey-predator model incorporating square root functional response. Jambura J Biomath 2(2):51–57. https://doi.org/10.34312/jjbm.v2i2.10444

    Article  Google Scholar 

  22. Tassaddiq A, Shabbir MS, Din Q, Ahmad K, Kazi S (2020) A ratio-dependent nonlinear predator-prey model with certain dynamical results. IEEE Access 8:1–1. https://doi.org/10.1109/access.2020.3030778

    Article  Google Scholar 

  23. Suryanto A, Darti I (2017) Stability analysis and nonstandard Grünwald-Letnikov scheme for a fractional order predator-prey model with ratio-dependent functional response. In: AIP conference proceedings, vol 1913. https://doi.org/10.1063/1.5016645

  24. Dang QA, Hoang MT (2019) Nonstandard finite difference schemes for a general predator-prey system. J Comput Sci 36:101015. https://doi.org/10.1016/j.jocs.2019.07.002

    Article  MathSciNet  Google Scholar 

  25. Diethelm K, Ford NJ, Freed AD (2002) A predictor-corrector approach for the numerical solution of fractional differential equations. Nonlinear Dyn 29(1–4):3–22. https://doi.org/10.1023/A:1016592219341

    Article  MathSciNet  MATH  Google Scholar 

  26. Arciga-Alejandre MP, Sanchez-Ortiz J, Ariza-Hernandez FJ, Catalan-Angeles G (2019) A multi-stage homotopy perturbation method for the fractional Lotka-Volterra model. Symmetry 11(11):1–9. https://doi.org/10.3390/sym11111330

    Article  Google Scholar 

  27. Atta AG, Youssri YH (2022) Advanced shifted first-kind Chebyshev collocation approach for solving the nonlinear time-fractional partial integro-differential equation with a weakly singular kernel. Comput Appl Math 41(8):381. https://doi.org/10.1007/s40314-022-02096-7

    Article  MathSciNet  MATH  Google Scholar 

  28. Abd-Elhameed WM, Youssri YH (2022) Spectral tau solution of the linearized time-fractional KdV-Type equations. AIMS Math 7(8):15138–15158. https://doi.org/10.3934/math.2022830

    Article  MathSciNet  Google Scholar 

  29. El-Sayed AMA, Salman SM (2013) On a discretization process of fractional order Riccati differential equation. J Fract Calc Appl 4(2):251–259

    MATH  Google Scholar 

  30. Agarwal RP, El-Sayed AMA, Salman SM (2013) Fractional-order Chua’s system: discretization, bifurcation and chaos. Adv Difference Equ 2013(1):320. https://doi.org/10.1186/1687-1847-2013-320

    Article  MathSciNet  MATH  Google Scholar 

  31. Kartal S, Gurcan F (2015) Stability and bifurcations analysis of a competition model with piecewise constant arguments. Math Methods Appl Sci 38(9):1855–1866. https://doi.org/10.1002/mma.3196

    Article  MathSciNet  MATH  Google Scholar 

  32. El-Shahed M, Ahmed AM, Abdelstar IME (2017) Stability and bifurcation analysis in a discrete-time predator-prey dynamics model with fractional order. TWMS J. Pure Appl. Math. 83–96(1):83–96

    MathSciNet  MATH  Google Scholar 

  33. El-Shahed M, Nieto JJ, Ahmed A, Abdelstar I (2017) Fractional-order model for biocontrol of the lesser date moth in palm trees and its discretization. Adv Difference Equ 2017(1):295. https://doi.org/10.1186/s13662-017-1349-1

    Article  MathSciNet  MATH  Google Scholar 

  34. Abdeljawad T, Al-Mdallal QM, Jarad F (2019) Fractional logistic models in the frame of fractional operators generated by conformable derivatives. Chaos Solitons Fractals 119:94–101. https://doi.org/10.1016/j.chaos.2018.12.015

    Article  MathSciNet  MATH  Google Scholar 

  35. Berec L, Angulo E, Courchamp F (2007) Multiple Allee effects and population management. Trends Ecol Evol 22(4):185–191. https://doi.org/10.1016/j.tree.2006.12.002

    Article  Google Scholar 

  36. Dennis B (1989) Allee effects: population growth, critical density, and the chance of extinction. Nat Resour Model 3(4):481–538. https://doi.org/10.1111/j.1939-7445.1989.tb00119.x

    Article  MathSciNet  MATH  Google Scholar 

  37. Abbas S, Banerjee M, Momani S (2011) Dynamical analysis of fractional-order modified logistic model. Comput Math Appl 62(3):1098–1104. https://doi.org/10.1016/j.camwa.2011.03.072

    Article  MathSciNet  MATH  Google Scholar 

  38. Liu X, Fan G, Zhang T (2019) Evolutionary dynamics of single species model with Allee effect. Phys A Stat Mech Appl. https://doi.org/10.1016/j.physa.2019.04.010

    Article  MATH  Google Scholar 

  39. Boukal DS, Berec L (2002) Single-species models of the Alee effect: extinction boundaries, sex ratios and mate encounters. J Theor Biol 218(3):375–394. https://doi.org/10.1006/jtbi.2002.3084

    Article  Google Scholar 

  40. Suryanto A, Darti I, Anam S (2017) Stability analysis of a fractional order modified leslie-gower model with additive Allee effect. Int J Math Math Sci 2017(0):1–9. https://doi.org/10.1155/2017/8273430

  41. Allee WC (1931) Animal aggregations, a study in general sociology. The University of Chicago Press, Chicago

    Book  Google Scholar 

  42. Courchamp F, Berec L, Gascoigne J (2008) Allee effects in ecology and conservation. Oxford University Press, New York

  43. Panigoro HS, Rahmi E (2022) Impact of fear and strong Allee effects on the dynamics of a fractional-order Rosenzweig-Macarthur model. In: Banerjee S, Saha A (eds) Nonlinear dynamics and applications. Springer, Cham, pp 611–619

  44. Panigoro HS, Rahmi E, Suryanto A, Darti I (2022) A fractional order predator-prey model with strong allee effect and michaelis-menten type of predator harvesting. In: AIP conference proceedings vol 2498(1), p 020018 https://aip.scitation.org/doi/pdf/10.1063/5.0082684. https://doi.org/10.1063/5.0082684

  45. Matignon D (1996) Stability results for fractional differential equations with applications to control processing. In: Computational engineering in systems applications, p 963–968

  46. Elaydi SN (2007) Discrete chaos: with applications in science and engineering, 2nd edn. Chapman and Hall/CRC, Boca Raton

    Book  Google Scholar 

  47. Li T-Y, Yorke JA (1975) Period three implies chaos. Am Math Mon 82(10):985. https://doi.org/10.2307/2318254

    Article  MathSciNet  MATH  Google Scholar 

  48. Chakraborty P, Sarkar S, Ghosh U (2021) Stability and bifurcation analysis of a discrete prey-predator model with sigmoid functional response and Allee effect. Rend del Circ Mat di Palermo 70(1):253–273. https://doi.org/10.1007/s12215-020-00495-5

    Article  MathSciNet  MATH  Google Scholar 

  49. Yuan LG, Yang QG (2015) Bifurcation, invariant curve and hybrid control in a discrete-time predator-prey system. Appl Math Model 39(8):2345–2362. https://doi.org/10.1016/j.apm.2014.10.040

    Article  MathSciNet  MATH  Google Scholar 

  50. Lin Y, Din Q, Rafaqat M, Elsadany AA, Zeng Y (2020) Dynamics and chaos control for a discrete-time Lotka-Volterra model. IEEE Access 8:126760–126775. https://doi.org/10.1109/ACCESS.2020.3008522

    Article  Google Scholar 

  51. Luo XS, Chen G, Wang BH, Fang JQ (2003) Hybrid control of period-doubling bifurcation and chaos in discrete nonlinear dynamical systems. Chaos Solitons Fractals 18(4):775–783. https://doi.org/10.1016/S0960-0779(03)00028-6

    Article  MATH  Google Scholar 

Download references

Funding

The work of Hasan S. Panigoro was funded by LPPM-UNG via PNBP-Universitas Negeri Gorontalo according to DIPA-UNG No. 023.17.2.677521/2021, under contract No. B/176/UN47.DI/PT.01.03/2022. The work of Agus Suryanto was funded by FMIPA via PNBP-University of Brawijaya according to DIPA-UB No. DIPA-023.17.2.677512/2020, under contract No. 12/UN10.F09/PN/2020.

Author information

Authors and Affiliations

  1. Biomathematics Research Group, Department of Mathematics, Universitas Negeri Gorontalo, Bone Bolango, 96554, Indonesia

    Hasan S. Panigoro

  2. Department of Mathematics, University of Brawijaya, Malang, 65145, Indonesia

    Maya Rayungsari & Agus Suryanto

  3. Department of Mathematics Education, PGRI Wiranegara University, Pasuruan, 67118, Indonesia

    Maya Rayungsari

Authors
  1. Hasan S. Panigoro
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Maya Rayungsari
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Agus Suryanto
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

All authors contributed equally to this article. They read and approved the final manuscript.

Corresponding author

Correspondence to Hasan S. Panigoro.

Ethics declarations

Conflict of interest

All authors declare that they have no conflict of interest.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Panigoro, H.S., Rayungsari, M. & Suryanto, A. Bifurcation and chaos in a discrete-time fractional-order logistic model with Allee effect and proportional harvesting. Int. J. Dynam. Control 11, 1544–1558 (2023). https://doi.org/10.1007/s40435-022-01101-5

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40435-022-01101-5

Keywords

Mathematics Subject Classification

Search

Navigation