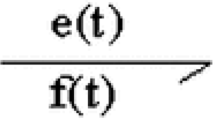Abstract
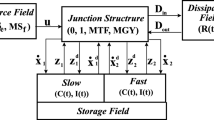
A procedure to obtain a composite control of linear systems with singular perturbations modelled by bond graphs is presented. The state feedback gains of the composite control based on the slow and fast bond graph models are separately designed. The composite control system is formed by: (1) The original Bond Graph in an Integral causality assignment (BGI) with a state feedback of the fast and slow dynamics. (2) An additional bond graph denoted by Singular Perturbed Bond Graph (SPBG) with a state feedback whose storage elements have integral and derivative causality for the slow and fast dynamics, respectively. The advantages of this approach are: (1) From the BGI, the reduced fast models for open and closed loop systems in a direct way are obtained. (2) From the SPBG, the reduced slow models are determined where the change of the causality of the storage elements for the fast dynamics produces inverse matrices required in the traditional approach. (3) The mathematical models are not required. A junction structure of the bond graph with a composite control to determine the mathematical model of the closed loop system is proposed. Finally, the modelling and control of two illustrative examples applying the proposed methodology are described.




























Similar content being viewed by others
References
Kokotovic PV, Khalil HK, O’Reilly J (1986) Singular perturbation methods in control: analysis and design. Academic Press, London
Naidu DS (1988) Singular perturbation methodology. In: Control Systems, IEE Control Engineering Series, vol 34. The Institution of Engineering and Technology, Ed. Peter Peregrinus Ltd, London
Lin K-J (2013) Stabilization of discrete-time fuzzy systems via composite state and output feedback control design. In: International conference on advanced robotics and intelligent systems, Tainan, Taiwan, pp 1–4
Zhang Y, Cheng G, Hu J, Huang Y (2017) Fast and accurate speed regulation of brushless DC motor servo systems via robust composite nonlinear control. In: Proceedings of the 36th Chinese control conference, pp 4924–4928, June 26–28, Dalian, China
Lin H, Ning X, Han B (2017) Composite decoupling control of gimbal servo system in double-gimbaled variable speed CMG via disturbance observer. IEEE/ASME Trans Mechatron 22(1):312–320
Shi Y, Cheng G, Huang Y, Hu J (2015) Improvement of trajectory tracking performance via modified composite nonlinear control. In: Proceedings of the 34th Chinese control conference, pp 5533–5538, July 28–30, Hangzhou, China
Abdelkrim N, Tellili A, Abdelkrin MN (2010) Composite control of a delayed singularly perturbed system by using Lambert-W function. In: 7th international multi-conference on systems, signals and devices, 27–30 June 2010. Amman, Jordan
Shariati A, Taghirad HD, Labibi B (2010) Dealy-Dependent H\(_{\infty }\) control of linear systems with input delay using composite state derivative feedback. In: Proceedings of the 4th international symposium on communications, control and signal processing, ISCCSP 2010, Limassol, Cyprus, 3–5 March
Glielmo L, Corless M (2010) On output feedback control of singularly systems. Appl Math Comput 217:1053–1070
Cai Ch, Zou Y, Zhang D (2004) State feedback design for singularly perturbed system using unified approach. J Control Theory Appl 2(3):276–280
Li T-H, Ling J-L, Kung F-C (1995) Composite sliding-mode control of singular perturbation systems. In: Proceedings of the American control conference, Seattle, Washington, pp 2248–2249
Wang M-S, Li T-HS, Sun Y-Y (1993) Design sliding-mode observers, for singular perturbation systems, pp 2347–2352, Maui, Hi, USA
Suzuki M (1981) Composite controls for singularly perturbed systems. IEEE Trans Autom Control AC–26(2):505–507
Singh H, Naidu DS, Nagurka ML (2000) Unified H\(_{\infty }\) approach for a singularly perturbed aircraft model. In: Proceedings of the American control conference, Chicago, Illinois, pp 1847–1851
Vian JL, Sawan ME (1991) H\(_{\infty }\) Control for singularly perturbed systems. In: Proceedings of the 30th conference on decision and control, Brighton, England, pp 1072–1074
Kim Y-J, Kim B -S, Lim M-T (2003) Composite control for singularly perturbed bilinear systems via successive Galerkin approximation. In: IEEE proceedings-control theory and applications, vol 150, no 5
Zhang B, Gao D, Lu Q, Cao F (2010) Approximation design of composite control for singularly perturbed time-delay via delay compensation. In: Proceedings of the 29th Chinese control conference, 29–31 July, Beijing, China
Xu H, Mukaidani H, Mizukami K (1996) On the near-optimality of composite optimal control for nonstandard singularly perturbed systems. In: Proceedings of 35th IEEE conference on decision and control, Vol 4, 13 December, Kobe, Japan
Mezovar A, Terras T, Fellah MK, Hadjeri S (2012) Composite Sliding mode control of induction motors using singular perturbation theory. Int Rev Autom control 5(6):901–910
Sueur C, Dauphin-Tanguy G (1991) Bond graph approach for structural analysis of MIMO linear systems. J Frankl Inst 328(1):55–70
Brown FT (2001) Engineering system dynamics. Marcel Dekker, Inc., New York
Karnopp DC, Margolis DL, Rosenberg RC (2000) System dynamics modeling and simulation of mechatronic systems. Wiley Interscience, New York
Sueur C, Dauphin-Tanguy G (1991) Bond graph approach to multi-time systems analysis. J Frankl Inst 328(5/6):1005–1026
Dauphin-Tanguy G, Borne P, Lebrun M (1985) Order reduction of multi-time scale systems using bond graphs, the reciprocal system and the singular perturbation method. J Frankl Inst 319(1–2):157–171
Gonzalez G, Barrera N (2013) Quasi-steady state model determination for systems with singular perturbations modelled by bond graphs. Math Comput Model Dyn Syst Methods Tools Appl Eng Relat Sci 19(5):483–503
Gonzalez G, Padilla A (2018) Quasi-steady state model of a class of nonlinear singularly perturbed system in a bond graph approach. Electr Eng J 100:293–302
Gonzalez G, Padilla A (2016) Approximate bond graph models for linear singularly perturbed systems. Math Comput Model Dyn Syst 22(5):412–443
Gonzalez G (2016) A bond graph model of a singularly perturbed LTI MIMO system with a slow state estimated feedback. Proc ImechE Part I J Syst Control Eng 230(8):799–819
Orbak AY, Turkay OS, Eskimat E, Youcef-Toumi K (2003) Model reduction in the physical domain. Proc Inst Mech Eng Part I J Syst Control Eng 217:481–496
Gonzalez G, Herrera MY (2014) The feedback of a singularly perturbed system modelled by bond graphs. In: Proceedings of the IASTED international conference modeling, identification and control (MIC 2014), 17–19 February, Innsbruck, Austria, pp 34–40. https://doi.org/10.2316/P.2014.809-045.
Van Dijk J, Breedveld PC (1991) Simulation of systems models containing zero-order causal paths-I. Classification of zero-order causal paths. J Frankl Inst 328(5/6):959–979
Author information
Authors and Affiliations
Corresponding author
Appendices
Fast models procedures
The bond graph model of a fast reduced model can be obtained by using one of the next two procedures proposed by [23] for the case when a bond graph model has C or I elements of different order of magnitude and R elements of the same order of magnitude (Procedure 1) and when a bond graph model has R elements of different order of magnitude and C or I elements of the same order of magnitude (Procedure 2).
Procedure 1
The fast reduced bond graph is deduced from the global one by suppressing:
-
All the C or I elements with large modulus. The state variables of the slow dynamics.
-
All the R elements causally connected with these C or I elements directly or indirectly through other R elements.
-
All the input sources having no causal connection with the remaining C, I and R elements.
Procedure 2
The fast reduced bond graph is deduced from the global one by suppressing:
-
All the C or I elements causally connected with large valued R elements or large valued R elements in the case of an algebraic loop.
-
All the R elements without causal connection with the remaining C, I or R directly or indirectly through other R elements.
-
All the input sources having no causal connection with the remaining C or I directly or indirectly through other R elements.
Proof of Lemma
From the fourth and fifth lines of (61) with (16) to (19) and their derivatives with respect to time
from ninth and tenth lines of (61) with (16) to (19) and different respect to the time
from the eighth line of (61) with (20)
from the third line of (61) with (20)
and substituting (123) into (124)
where \(Q_{s}=L_{s}\left( I-R_{55}L_{s}\right) ^{-1}\).
From the first line of (61) with (119)
where \(E_{1}^{c}=I-R_{13}^{11}\left( F_{1}^{d}\right) ^{-1}R_{31}^{11}F_{1}\).
Substituting (125) and (124) into (126) with (20)
where \(M_{c}=L\left( I-R_{22}L\right) ^{-1}\). From (127) with (64), (65), (66) and (70), the state equation for the slow dynamics (62) is proved.
From the second line of (61) with (120)
substituting (125) and (124) into (128) with (20)
from (129) with (67), (68), (69) and (71), the state equation for the fast dynamics (63) is proved.
From the eleventh line of (61) with (124), (125) and removing the terms of \( \overset{\bullet }{x}_{2s}^{c}\)
from (77), (78), (79), (80) and (130), (76) is proved.
From (56) with \(u_{s}=G_{0}x_{1}^{s},\) we have
applying the relationships between BGI and SPBG (42) and (45) to (131)
considering that the slow dynamics \(x_{1}^{s}\) is approximated to \(x_{1}\) then it is proved that (133) represents the control signal in a bond graph approach given by (76) of the composite control defined by (10) with (11).
Causal paths
In this section the definition and classification of the zero-causal paths [31] are described.
The classification of zero-order causal paths follows from the research work carried out by Van Dijk and Breedveld [31].
Definitions
Causal path is a sequence of causal bonds between two vertices of the bond graph. The causal strokes must be attached to each bond at “same relative position”.
Causal cycle is a closed causal path.
Topological loop is a signal (flow or effort) loop associated with a causal cycle.
Causal mesh is a closed causal path, usually ends in a port of an element and it has to contain an odd number of gyrators.
A causal cycle is an essential causal cycle if:
-
1.
There is a closed path along a simple junction structure. If more conservation principles than just power conservation are applicable, then the bond graph must represent them explicitly.
-
2.
There is a closed causal path along a weighted junction structure and the loop gain associated with this causal cycle is not equal 1.
In all other cases the causal cycle is not essential.
Zero-order causal path is a causal path with topological loops whose variables are related themselves by means of algebraic assignments [31].
The following classification of zero-order causal can be described [31],
Class 1 zero-order causal path Causal path is between a storage element (or port) with derivative and a storage element (port) with integral causality (Fig. 29a).
Class 2 zero-order causal path Causal path is between elements (ports) whose the constitutive relations are algebraic (algebraic loop) (Fig. 29b).
Class 3 zero-order causal path The closed causal path is an essential causal cycle (Fig. 30a).
Class 4 zero-order causal path Causal cycle is not essential. The loop gain of the topological loops is always \(+\,1\) (Fig. 30b).
Class 5 zero-order causal path The closed causal path is a causal mesh (Fig. 30c).
Rights and permissions
About this article
Cite this article
Gonzalez-A, G., Barrera-G, N., Padilla, J.A. et al. Composite feedback control of linear singularly perturbed systems: a bond graph approach. Int. J. Dynam. Control 9, 689–710 (2021). https://doi.org/10.1007/s40435-020-00690-3
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s40435-020-00690-3