Skip to main content
SpringerLink
Log in

An accurate and cost-efficient numerical approach to analyze the initial and boundary value problems of fractional multi-order

  • Published:
Computational and Applied Mathematics Aims and scope Submit manuscript

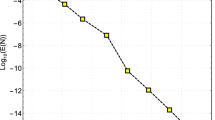
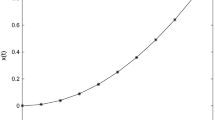
Abstract

This paper proposes an cost-efficient method for the numerical solution of fractional differential equations of multi-order. The suggested algorithm adopts the operational matrix of fractional derivative in term of Caputo sense using modified cubic B-spline functions. The new method leads to a system of algebraic equations that improves the solution accuracy and reduces the computational complexity. Several numerical examples illustrate the applicability of the proposed method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  • Blank L (1995) Numerical treatment of differential equations of fractional order, Manchester centre for computational mathematics numerical analysis Report No. 287, The University of Manchester

  • Bolandtalat A, Babolian E, Jafari H (2016) Numerical solutions of multi-order fractional differential equations by Boubaker polynomials. Open Phys 14:226–230

    Article  Google Scholar 

  • Chang RY, Chen KC, Wang ML (1985) Modified Laguerre operational matrices for fractional calculus and applications. Int J Syst Sci 16(9):1163–1172

    Article  MATH  Google Scholar 

  • Chui CK, Quak E (1992) Wavelets on a bounded interval. Numer Approx Theory 9:53–75

    MathSciNet  MATH  Google Scholar 

  • Dehghan M, Safarpoor M (2016) The dual reciprocity boundary elements method for the linear and nonlinear twodimensional timefractional partial differential equations. Math Methods Appl Sci 39:3979–3995

    Article  MathSciNet  MATH  Google Scholar 

  • Dehghan M, Manafian J, Saadatmandi A (2010) Solving nonlinear fractional partial differential equations using the homotopy analysis method. Numer Methods Partial Differ Equ 26(2):448–479

    MathSciNet  MATH  Google Scholar 

  • Diethelm K (1997) An algorithm for the numerical solution of differential equations of fractional order. Electron Trans Numer Anal 5:1–6

    MathSciNet  MATH  Google Scholar 

  • Diethelm K (2010) The analysis of fractional differential equations, an application-oriented exposition using differential operators of Caputo type. Springer, Berlin

    MATH  Google Scholar 

  • Diethelm K, Ford NJ, Freed AD (2002) A predictor–corrector approach for the numerical solution of fractional differential equations. Nonlinear Dyn 29(1–4):3–22

    Article  MathSciNet  MATH  Google Scholar 

  • Hall CA, Meryer W (1976) Optimal error bounds for cubic spline interpolation. J Approx Theory 16:105–122

    Article  MathSciNet  MATH  Google Scholar 

  • Hashim I, Abdulaziz Q, Momani S (2009) Homotopy analysis method for fractional IVPs. Commun Nonlinear Sci Numer Simul 14:674–684

    Article  MathSciNet  MATH  Google Scholar 

  • Jafari H, Tajadodi H, Baleanu D (2015) A numerical approach for fractional order Riccati differential equation using B-spline operational matrix. Fract Calc Appl Anal 18(2):387–399

    Article  MathSciNet  MATH  Google Scholar 

  • Khan Y, Panjeh Ali Beik S, Sayevand K, Shayeganmanesh A (2015) A numerical scheme for solving differential equations with space and time-fractional coordinate derivatives. Quaest Math. 38(1):41–55

    Article  MathSciNet  MATH  Google Scholar 

  • Khosravian-Arab H, Dehghan M, Eslahchib MR (2015) Fractional Sturm Liouville boundary value problems in unbounded domains: theory and applications. J Comput Phys 299:526–560

    Article  MathSciNet  MATH  Google Scholar 

  • Khosravian-Arab H, Dehghan M, Eslahchib MR (2017) Fractional spectral and pseudospectral methods in unbounded domains: theory and applications. J Comput Phys 338:527–566

    Article  MathSciNet  Google Scholar 

  • Kilbas A, Srivastava HM, Trujillo JJ (2006) Theory and applications of fractional differential equations. Elsevier, Amsterdam

    MATH  Google Scholar 

  • Kumar P, Agrawal OP (2006) An approximate method for numerical solution of fractional differential equations. Signal Proc 86:2602–2610

    Article  MATH  Google Scholar 

  • Lakestani M, Dehghan M (2009) Numerical solution of Fokker-Planck equation using the cubic B-spline scaling functions. Numer Methods Partial Differ Equ 25(2):418–429

    Article  MathSciNet  MATH  Google Scholar 

  • Lakestani M, Razzaghi M, Dehghan M (2005) Solution of nonlinear Fredholm–Hammerstein integral equations by using semiorthogonal spline wavelets. Math Prob Eng 1:113–121

    Article  MathSciNet  MATH  Google Scholar 

  • Lakestani M, Dehghan M, Irandoust-Pakchin S (2012) The construction of operational matrix of fractional derivatives using B-spline functions. Commun Nonlinear Sci Numer Simul 17:1149–1162

    Article  MathSciNet  MATH  Google Scholar 

  • Lakestani M, Razzaghi M, Dehghan M (2006) Semiorthogonal spline wavelets approximation for Fredholm integro-differential equations. Math Prob Eng 1–12, (Article ID 96184)

  • Li Y (2010) Solving a nonlinear fractional differential equation using Chebyshev wavelets. Commun Nonlinear Sci Numer Simul 15:2284–2292

    Article  MathSciNet  MATH  Google Scholar 

  • Li X (2012) Numerical solution of fractional differential equations using cubic B-spline wavelet collocation method. Commun Nonlinear Sci Numer Simul 17:3934–3964

    Article  MathSciNet  MATH  Google Scholar 

  • Lotfi A, Dehghan M, Yousefi SA (2011) A numerical technique for solving fractional optimal control problem. Comput Math Appl 62:1055–1067

    Article  MathSciNet  MATH  Google Scholar 

  • Moghaddam BP, Machado JAT, Behforooz H (2017) An integro quadratic spline approach for a class of variable-order fractional initial value problems. Chaos Solitons Fract 102:354–360

    Article  MathSciNet  MATH  Google Scholar 

  • Oldham KB, Spanier J (1974) The fractional calculus. Academic Press, New York

    MATH  Google Scholar 

  • Patent PD (1976) The effect of quadrature errors in the computation of \(L^{2}\) piecewise polynomial approximations. SIAM J Numer Anal 13(3):344–361

    MathSciNet  MATH  Google Scholar 

  • Podlubny I (1999) Fractional differential equations calculus. Academic Press, New York

    MATH  Google Scholar 

  • Saadatmandi A, Dehghan M (2010) A new operational matrix for solving fractional-order differential equations. Comput Math Appl 59:1326–1336

    Article  MathSciNet  MATH  Google Scholar 

  • Sayevand K, Pichaghchi K (2015) Successive approximation: a survey on stable manifold of fractional differential systems. Fract Calc Appl Anal 18(3):321–341

    Article  MathSciNet  MATH  Google Scholar 

  • Sayevand K, Pichaghchi K (2017) A novel operational matrix method for solving singularly perturbed boundary value problems of fractional multi-order. Int J Comput Math 95:767–796

    Article  MathSciNet  MATH  Google Scholar 

  • ur Rehman M, Khan RA (2011) The Legendre wavelet method for solving fractional differential equations. Commun Nonlinear Sci Numer Simul 16:4163–4173

    Article  MathSciNet  MATH  Google Scholar 

  • Wu JL (2009) A wavelet operational method for solving fractional partial differential equations numerically. Appl Math Comput 214:31–40

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Faculty of Mathematical Sciences, Malayer University, P. O. Box 16846-13114, Malayer, Iran

    K. Sayevand

  2. Department of Electrical Engineering, Institute of Engineering of Polytechnic of Porto, Porto, Portugal

    J. Tenreiro Machado

Authors
  1. K. Sayevand
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. J. Tenreiro Machado
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to K. Sayevand.

Additional information

Communicated by Vasily E. Tarasov.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sayevand, K., Machado, J.T. An accurate and cost-efficient numerical approach to analyze the initial and boundary value problems of fractional multi-order . Comp. Appl. Math. 37, 6582–6600 (2018). https://doi.org/10.1007/s40314-018-0700-9

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40314-018-0700-9

Keywords

Mathematics Subject Classification

Search

Navigation