A Cognitive Information-Based Decision-Making Algorithm Using Interval-Valued q-Rung Picture Fuzzy Numbers and Heronian Mean Operators

Abstract

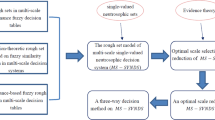
The complexity of the socioeconomic environment means that it is challenging to make decisions that rely on cognitive information. Decision makers normally cannot obtain a precise or sufficient level of knowledge about the problem domain and hence must provide multiple answers with interval values to depict them. This makes cognizing and decision making very difficult. To address this issue, this paper proposes a novel cognitive information-based decision-making algorithm with interval-valued q-rung picture fuzzy (IVq-RPtF) numbers. We first define the concept of the IVq-RPtF set, including the basic definition, operational laws, a score function, and an accuracy function. Considering the interrelationship between attributes, we then present the IVq-RPtF Heronian mean (IVq-RPtFHM) operators using the new operational laws. Moreover, we discuss the properties of IVq-RPtFHM operators, such as monotonicity, commutativity, and idempotency. Finally, we use a numerical example to verify the viability of the proposed method. The results show that the proposed method effectively expresses multiple types of interval cognitive information. The sensitivity analysis of the parameters shows that the ranking results are susceptible to parameter changes, but regardless of how the parameters change, the score values of the four alternatives in our example are in the range of [1.27, 1.66], within the basic scoring range of [1.352–1.472] for the four alternatives. Therefore, our proposed method based on IVq-RPtFHM operators has a stronger information aggregation ability than other methods. Compared with other methods, the proposed cognitive information-based decision-making algorithm is more widely applicable, avoids loss of cognitive information, and conducts a reasonable decision-making process.

Appendices

Appendix 1

• (1) The addition operation of IVq-ROFNs. We need to perform addition operations for the interval MEBDs and NMEBDs of IVq-ROFNs. Specifically, the addition operation between the lower bounds of the MEBDs is $\left( {\left( {u_{1}^{L} } \right)^{q} + \left( {u_{2}^{L} } \right)^{q} - \left( {u_{1}^{L} } \right)^{q} \left( {u_{2}^{L} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$, and the addition operation between the upper bounds of the MEBDs is $\left( {\left( {u_{1}^{U} } \right)^{q} + \left( {u_{2}^{U} } \right)^{q} - \left( {u_{1}^{U} } \right)^{q} \left( {u_{2}^{U} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$. Similarly, the addition between the lower bounds of the NMEBDs is $v_{1}^{L} v_{2}^{L}$, and that between the upper bounds of the MEBDs is $v_{1}^{U} v_{2}^{U}$. Then, we obtain the addition operation of IVq-ROFNs as follows:

  $$A_1 \oplus A_2 = \begin{pmatrix} \begin{aligned} &\begin{bmatrix} \left( \left(u^L_1\right)^q + \left(u^L_2\right)^q - \left(u^L_1\right)^q \left(u^L_2\right)^q \right)^{1 \left / \right. q}, \\ \left( \left(u^U_1\right)^q + \left(u^U_2\right)^q - \left(u^U_1\right)^q \left(u^U_2\right)^q \right)^{1 \left / \right. q} \end{bmatrix} \\ & \left[v^L_1 v^L_2, v^U_1 v^U_2 \right] \end{aligned} \end{pmatrix},$$

• (2) The product operation of IVq-ROFNs. We also need to determine the product operations of the interval MEBDs and NMEBDs of IVq-ROFNs. The product between the lower bounds of the MEBDs is $u_{1}^{L} u_{2}^{L}$ and the corresponding product between the upper bounds is $u_{1}^{U} u_{2}^{U}$. The product between the lower bounds of the NMEBDs is $\left( {\left( {v_{1}^{L} } \right)^{q} + \left( {v_{2}^{L} } \right)^{q} - \left( {v_{1}^{L} } \right)^{q} \left( {v_{2}^{L} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$, and the product between the upper bounds is $\left( {\left( {v_{1}^{U} } \right)^{q} + \left( {v_{2}^{U} } \right)^{q} - \left( {v_{1}^{U} } \right)^{q} \left( {v_{2}^{U} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$. Then, we can obtain the product operation of IVq-ROFNs as follows:

  $$A_1 \otimes A_2 = \begin{pmatrix} \begin{aligned} &\left[ u^L_1 u^L_2, u^U_1 u^U_2 \right], \\ &\begin{bmatrix} \left( \left(v^L_1\right)^q + \left(v^L_2\right)^q - \left(v^L_1\right)^q \left(v^L_2\right)^q \right)^{1 \left / \right. q}, \\ \left( \left(v^U_1\right)^q + \left(v^U_2\right)^q - \left(v^U_1\right)^q \left(v^U_2\right)^q \right)^{1 \left / \right. q} \end{bmatrix}\\ \end{aligned} \end{pmatrix},$$

• (3) The scalar multiplication of IVq-ROFNs. Similarly, the scalar multiplication between the lower bounds of the MEBDs is $\left( {1 - \left( {1 - \left( {u_{1}^{L} } \right)^{q} } \right)^{\lambda } } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$, the corresponding scalar multiplication between the upper bounds is $\left( {1 - \left( {1 - \left( {u_{1}^{U} } \right)^{q} } \right)^{\lambda } } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$, the scalar multiplication between the lower bounds of the NMEBDs is $\left( {v_{1}^{L} } \right)^{\lambda }$, and the scalar multiplication between the upper bounds is $\left( {v_{1}^{U} } \right)^{\lambda }$. Then, we can obtain the scalar multiplication of IVq-ROFNs as follows:

  $$\lambda A_1 = \begin{pmatrix} \begin{aligned} &\begin{bmatrix} \left( 1 - \left(1 - \left( u^L_1 \right)^q\right)^\lambda \right)^{1 \left / \right.q} \\ \left( 1 - \left(1 - \left( u^U_1 \right)^q\right)^\lambda \right)^{1 \left / \right.q} \end{bmatrix} \\ & \left[ \left(v ^L_1\right)^\lambda , \left(v^U_1 \right)^\lambda \right]\end{aligned}\end{pmatrix},$$

• (4) The power operation of IVq-ROFNs. Similar to the above three operational rules, the power operation between the lower bounds of the MEBDs is $\left( {u_{1}^{L} } \right)^{\lambda }$, and the corresponding power operation between the upper bounds is $\left( {u_{1}^{U} } \right)^{\lambda }$. The power operation between the lower bounds of the NMEBDs is $\left( {1 - \left( {1 - \left( {v_{1}^{L} } \right)^{q} } \right)^{\lambda } } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$, and the power operation between the upper bounds is $\left( {1 - \left( {1 - \left( {v_{1}^{U} } \right)^{q} } \right)^{\lambda } } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$. Finally, we can obtain the power operation of IVq-ROFNs as follow

  $$A_1^\lambda = \begin{pmatrix} \begin{aligned} & \left[ \left(v ^L_1\right)^\lambda , \left(v^U_1 \right)^\lambda \right], \\ &\begin{bmatrix} \left( 1 - \left(1 - \left( u^L_1 \right)^q\right)^\lambda \right)^{1 \left / \right.q} \\ \left( 1 - \left(1 - \left( u^U_1 \right)^q\right)^\lambda \right)^{1 \left / \right.q} \end{bmatrix} \end{aligned}\end{pmatrix}.$$

Appendix 2

• (1) The addition operation between IVq-RPtFNs. We need to compute the addition operations for the interval POSMEBDs, NEUMEBDs, and NEGMEBDs of IVq-ROFNs. According to Definition 3, the addition operations of the lower bounds and upper bounds of the POSMEBDs are $\left( {\left( {\Phi_{1}^{L} } \right)^{q} + \left( {\Phi_{2}^{L} } \right)^{q} - \left( {\Phi_{1}^{L} } \right)^{q} \left( {\Phi_{2}^{L} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$ and $\left( {\left( {\Phi_{1}^{U} } \right)^{q} + \left( {\Phi_{2}^{U} } \right)^{q} - \left( {\Phi_{1}^{U} } \right)^{q} \left( {\Phi_{2}^{U} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$, respectively. The addition operations of the lower bounds and upper bounds of the NEUMEBDs are $\Psi_{1}^{L} \Psi_{2}^{L}$ and$\Psi_{1}^{U} \Psi_{2}^{U}$, respectively. The addition operations of the lower bounds and upper bounds of the NEGMEBDs are $\Upsilon_{1}^{L} \Upsilon_{2}^{L}$ and $\Upsilon_{1}^{U} \Upsilon_{2}^{U}$, respectively. From these calculations, we obtain

  $$A_1 \oplus A_2 = \begin{pmatrix} \begin{aligned} &\begin{bmatrix} \left(\left(\Phi^L_1\right)^q + \left(\Phi^L_2\right)^q - \left(\Phi^L_1\right)^q\left(\Phi^L_2\right)^q\right)^{1 \left / \right. q}, \\ \left(\left(\Phi^U_1\right)^q + \left(\Phi^L_2\right)^q - \left(\Phi^U_1\right)^q\left(\Phi^U_2\right)^q\right)^{1 \left / \right. q} \end{bmatrix}, \\& \left[\Psi^L_1 \Psi^L_2, \Psi^U_1 \Psi^U_2\right], \left[\Upsilon^L_1 \Upsilon^L_2, \Upsilon^U_1 \Upsilon^U_2\right] \end{aligned} \end{pmatrix},$$

• (2) The product operation between IVq-RPtFNs. Similarly, the product operations of the lower bounds and upper bounds of the POSMEBDs are $\Phi_{1}^{L} \Phi_{2}^{L}$ and$\Phi_{1}^{U} \Phi_{2}^{U}$, respectively. The product operations of the lower bounds and upper bounds of the NEUMEBDs are $\left( {\left( {\Psi_{1}^{L} } \right)^{q} + \left( {\Psi_{2}^{L} } \right)^{q} - \left( {\Psi_{1}^{L} } \right)^{q} \left( {\Psi_{2}^{L} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$ and $\left( {\left( {\Psi_{1}^{U} } \right)^{q} + \left( {\Psi_{2}^{U} } \right)^{q} - \left( {\Psi_{1}^{U} } \right)^{q} \left( {\Psi_{2}^{U} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$, respectively. The product operations of the lower bounds and upper bounds of the NEGMEBDs are $\left( {\left( {\Upsilon_{1}^{L} } \right)^{q} + \left( {\Upsilon_{2}^{L} } \right)^{q} - \left( {\Upsilon_{1}^{L} } \right)^{q} \left( {\Upsilon_{2}^{L} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$ and $\left( {\left( {\Upsilon_{1}^{U} } \right)^{q} + \left( {\Upsilon_{2}^{U} } \right)^{q} - \left( {\Upsilon_{1}^{U} } \right)^{q} \left( {\Upsilon_{2}^{U} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$, respectively. From these calculations, we obtain

  $$A_1 \otimes A_2 = \begin{pmatrix} \begin{aligned} &\left[ \Phi^L_1 \Phi^L_2, \Phi^U_1 \Phi^U_2\right], \\ & \begin{bmatrix} \left(\left(\Psi^L_1\right)^q + \left(\Psi^L_2\right)^1 - \left(\Psi^L_1\right)^q\left(\Psi^L_2\right)^q\right)^{1 \left /\right. q}, \\ \left(\left(\Psi^U_1\right)^q + \left(\Psi^U_2\right)^1 - \left(\Psi^U_1\right)^q\left(\Psi^U_2\right)^q\right)^{1 \left /\right. q}\end{bmatrix}, \\ & \begin{bmatrix} \left(\left(\Upsilon^L_1\right)^q + \left(\Upsilon^L_2\right)^1 - \left(\Upsilon^L_1\right)^q\left(\Upsilon^L_2\right)^q\right)^{1 \left /\right. q}, \\ \left(\left(\Upsilon^U_1\right)^q + \left(\Upsilon^U_2\right)^1 - \left(\Upsilon^U_1\right)^q\left(\Upsilon^U_2\right)^q\right)^{1 \left /\right. q}\end{bmatrix} \end{aligned} \end{pmatrix},$$

• (3) The scalar multiplication of IVq-RPtFNs. The scalar multiplications of the lower bounds and upper bounds of the POSMEBDs are $\left( {1 - \left( {1 - \left( {\Phi_{1}^{L} } \right)^{q} } \right)^{\lambda } } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$ and $\left( {1 - \left( {1 - \left( {\Phi_{1}^{U} } \right)^{q} } \right)^{\lambda } } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$, respectively. The scalar multiplications of the lower bounds and upper bounds of the NEUMEBDs are $\left( {\Psi_{1}^{L} } \right)^{\lambda }$ and$\left( {\Psi_{1}^{U} } \right)^{\lambda }$, respectively. The scalar multiplications of the lower bounds and upper bounds of the NEGMEBDs are $\left( {\Upsilon_{1}^{L} } \right)^{\lambda }$ and $\left( {\Upsilon_{1}^{U} } \right)^{\lambda }$, respectively. From these calculations, we obtain

  $$\lambda A_1 = \begin{pmatrix} \begin{aligned} &\begin{bmatrix} \left(1 -\left(1 - \left(\Phi^L_1\right)^q\right)^\lambda\right)^{1 \left / \right. q}, \\ \left(1 -\left(1 - \left(\Phi^U_1\right)^q\right)^\lambda\right)^{1 \left / \right. q}\end{bmatrix} \\& \left[\left(\Psi^L_1\right)^\lambda, \left(\Psi^L_1\right)^\lambda \right], \left[\left(\Upsilon^L_1\right)^\lambda, \left(\Upsilon^L_1\right)^\lambda \right] \end{aligned} \end{pmatrix},$$

• (4) The power operation of IVq-RPtFNs. The power operations of the lower bounds and upper bounds of the POSMEBDs are $\left( {\Phi_{1}^{L} } \right)^{\lambda }$ and $\left( {\Phi_{1}^{U} } \right)^{\lambda }$, respectively. The power operations of the lower bounds and upper bounds of the NEUMEBDs are $\left( {1 - \left( {1 - \left( {\Psi_{1}^{L} } \right)^{q} } \right)^{\lambda } } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$ and $\left( {1 - \left( {1 - \left( {\Psi_{1}^{U} } \right)^{q} } \right)^{\lambda } } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$, respectively. The power operations of the lower bounds and upper bounds of the NEGMEBDs are $\left( {1 - \left( {1 - \left( {\Upsilon_{1}^{L} } \right)^{q} } \right)^{\lambda } } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$ and $\left( {1 - \left( {1 - \left( {\Upsilon_{1}^{U} } \right)^{q} } \right)^{\lambda } } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$, respectively. From these calculations, we obtain

  $$A^\lambda_1 = \begin{pmatrix} \begin{aligned} &\left[\left(\Phi^L_1\right)^\lambda , \left(\Phi^U_1\right)^\lambda\right] \\ &\begin{bmatrix} \left(1 -\left(1 - \left(\Psi^L_1\right)^q\right)^\lambda\right)^{1 \left / \right. q}, \\ \left(1 -\left(1 - \left(\Psi^U_1\right)^q\right)^\lambda\right)^{1 \left / \right. q}\end{bmatrix} \\ &\begin{bmatrix} \left(1 -\left(1 - \left(\Upsilon^L_1\right)^q\right)^\lambda\right)^{1 \left / \right. q}, \\ \left(1 -\left(1 - \left(\Upsilon^U_1\right)^q\right)^\lambda\right)^{1 \left / \right. q}\end{bmatrix} \end{aligned}\end{pmatrix}.$$

Appendix 3

Proof

According to Definition 6 we can easily infer that (1), (3), (5), (6), and (7) are clarified, and (2), (4), and (8) need to be further proven as follows:

For (2), $\left( {A_{1} \oplus A_{2} } \right) \oplus A_{3} = A_{1} \oplus \left( {A_{2} \oplus A_{3} } \right)$

Let the degree of positive membership of $\left( {A_{1} \oplus A_{2} } \right) \oplus A_{3}$ and $A_{1} \oplus \left( {A_{2} \oplus A_{3} } \right)$ be $\Phi_{{\left( {A_{1} \oplus A_{2} } \right) \oplus A_{3} }}$ and$\Phi_{{A_{1} \oplus \left( {A_{2} \oplus A_{3} } \right)}}$, the degree of neutral membership of $\left( {A_{1} \oplus A_{2} } \right) \oplus A_{3}$ and $A_{1} \oplus \left( {A_{2} \oplus A_{3} } \right)$ be $\Psi_{{\left( {A_{1} \oplus A_{2} } \right) \oplus A_{3} }}$ and $\Psi_{{A_{1} \oplus \left( {A_{2} \oplus A_{3} } \right)}}$, and the degree of negative membership of $\left( {A_{1} \oplus A_{2} } \right) \oplus A_{3}$ and $A_{1} \oplus \left( {A_{2} \oplus A_{3} } \right)$ be $\Upsilon_{{\left( {A_{1} \oplus A_{2} } \right) \oplus A_{3} }}$ and$\Upsilon_{{A_{1} + \left( {A_{2} + A_{3} } \right)}}$, respectively. Then, we obtain

$$\begin{aligned} &\left[ {\Phi_{{\left( {A_{1} \oplus A_{2} } \right) \oplus A_{3} }}^{L} ,\Phi_{{\left( {A_{1} \oplus A_{2} } \right) \oplus A_{3} }}^{U} } \right] \hfill \\ &= \left[ \begin{aligned} \left( {\left( {\Phi_{1}^{L} } \right)^{q} + \left( {\Phi_{2}^{L} } \right)^{q} - \left( {\Phi_{1}^{L} } \right)^{q} \left( {\Phi_{2}^{L} } \right)^{q} + \left( {\Phi_{3}^{L} } \right)^{q} - \left( {\left( {\Phi_{1}^{L} } \right)^{q} + \left( {\Phi_{2}^{L} } \right)^{q} - \left( {\Phi_{1}^{L} } \right)^{q} \left( {\Phi_{2}^{L} } \right)^{q} } \right)\left( {\Phi_{3}^{L} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} , \hfill \\ \left( {\left( {\Phi_{1}^{U} } \right)^{q} + \left( {\Phi_{2}^{U} } \right)^{q} - \left( {\Phi_{1}^{U} } \right)^{q} \left( {\Phi_{2}^{U} } \right)^{q} + \left( {\Phi_{3}^{U} } \right)^{q} - \left( {\left( {\Phi_{1}^{U} } \right)^{q} + \left( {\Phi_{2}^{U} } \right)^{q} - \left( {\Phi_{1}^{U} } \right)^{q} \left( {\Phi_{2}^{U} } \right)^{q} } \right)\left( {\Phi_{3}^{U} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} \hfill \\ \end{aligned} \right] \hfill \\ &= \left[ \begin{aligned} \left( {\left( {\Phi_{1}^{L} } \right)^{q} + \left( {\Phi_{2}^{L} } \right)^{q} + \left( {\Phi_{3}^{L} } \right)^{q} - \left( {\Phi_{1}^{L} } \right)^{q} \left( {\Phi_{2}^{L} } \right)^{q} - \left( {\Phi_{1}^{L} } \right)^{q} \left( {\Phi_{3}^{L} } \right)^{q} - \left( {\Phi_{2}^{L} } \right)^{q} \left( {\Phi_{3}^{L} } \right)^{q} - \left( {\Phi_{1}^{L} } \right)^{q} \left( {\Phi_{2}^{L} } \right)^{q} \left( {\Phi_{3}^{L} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} , \hfill \\ \left( {\left( {\Phi_{1}^{U} } \right)^{q} + \left( {\Phi_{2}^{U} } \right)^{q} + \left( {\Phi_{3}^{U} } \right)^{q} - \left( {\Phi_{1}^{U} } \right)^{q} \left( {\Phi_{2}^{U} } \right)^{q} - \left( {\Phi_{1}^{U} } \right)^{q} \left( {\Phi_{3}^{U} } \right)^{q} - \left( {\Phi_{2}^{U} } \right)^{q} \left( {\Phi_{3}^{U} } \right)^{q} - \left( {\Phi_{1}^{U} } \right)^{q} \left( {\Phi_{2}^{U} } \right)^{q} \left( {\Phi_{3}^{U} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} \hfill \\ \end{aligned} \right] \hfill \\ \end{aligned}$$

$$\begin{aligned} &\left[ {\Phi_{{A_{1} \oplus \left( {A_{2} \oplus A_{3} } \right)}}^{L} ,\Phi_{{A_{1} \oplus \left( {A_{2} \oplus A_{3} } \right)}}^{U} } \right] \hfill \\ &= \left[ \begin{aligned} \left( {\left( {\Phi_{2}^{L} } \right)^{q} + \left( {\Phi_{3}^{L} } \right)^{q} - \left( {\Phi_{2}^{L} } \right)^{q} \left( {\Phi_{3}^{L} } \right)^{q} + \left( {\Phi_{1}^{L} } \right)^{q} - \left( {\left( {\Phi_{2}^{L} } \right)^{q} + \left( {\Phi_{3}^{L} } \right)^{q} - \left( {\Phi_{2}^{L} } \right)^{q} \left( {\Phi_{3}^{L} } \right)^{q} } \right)\left( {\Phi_{1}^{L} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} , \hfill \\ \left( {\left( {\Phi_{2}^{U} } \right)^{q} + \left( {\Phi_{3}^{U} } \right)^{q} - \left( {\Phi_{2}^{U} } \right)^{q} \left( {\Phi_{3}^{U} } \right)^{q} + \left( {\Phi_{1}^{U} } \right)^{q} - \left( {\left( {\Phi_{2}^{U} } \right)^{q} + \left( {\Phi_{3}^{U} } \right)^{q} - \left( {\Phi_{2}^{U} } \right)^{q} \left( {\Phi_{3}^{U} } \right)^{q} } \right)\left( {\Phi_{1}^{U} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} \hfill \\ \end{aligned} \right] \hfill \\ &= \left[ \begin{aligned} \left( {\left( {\Phi_{1}^{L} } \right)^{q} + \left( {\Phi_{2}^{L} } \right)^{q} + \left( {\Phi_{3}^{L} } \right)^{q} - \left( {\Phi_{1}^{L} } \right)^{q} \left( {\Phi_{2}^{L} } \right)^{q} - \left( {\Phi_{1}^{L} } \right)^{q} \left( {\Phi_{3}^{L} } \right)^{q} - \left( {\Phi_{2}^{L} } \right)^{q} \left( {\Phi_{3}^{L} } \right)^{q} - \left( {\Phi_{1}^{L} } \right)^{q} \left( {\Phi_{2}^{L} } \right)^{q} \left( {\Phi_{3}^{L} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} , \hfill \\ \left( {\left( {\Phi_{1}^{U} } \right)^{q} + \left( {\Phi_{2}^{U} } \right)^{q} + \left( {\Phi_{3}^{U} } \right)^{q} - \left( {\Phi_{1}^{U} } \right)^{q} \left( {\Phi_{2}^{U} } \right)^{q} - \left( {\Phi_{1}^{U} } \right)^{q} \left( {\Phi_{3}^{U} } \right)^{q} - \left( {\Phi_{2}^{U} } \right)^{q} \left( {\Phi_{3}^{U} } \right)^{q} - \left( {\Phi_{1}^{U} } \right)^{q} \left( {\Phi_{2}^{U} } \right)^{q} \left( {\Phi_{3}^{U} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} \hfill \\ \end{aligned} \right] \hfill \\ \end{aligned}$$

Then,

$$\Phi_{{\left( {A_{1} \oplus A_{2} } \right) \oplus A_{3} }} { = }\Phi_{{A_{1} \oplus \left( {A_{2} \oplus A_{3} } \right)}}$$

Similarly, we can deduce that

$$\Psi_{{\left( {A_{1} \oplus A_{2} } \right) \oplus A_{3} }} { = }\Psi_{{A_{1} \oplus \left( {A_{2} \oplus A_{3} } \right)}}$$

$$\Upsilon_{{\left( {A_{1} \oplus A_{2} } \right) \oplus A_{3} }} { = }\Upsilon_{{A_{1} \oplus \left( {A_{2} \oplus A_{3} } \right)}}$$

Therefore, $\left( {A_{1} \oplus A_{2} } \right) \oplus A_{3} = A_{1} \oplus \left( {A_{2} \oplus A_{3} } \right)$

For (4), $\left( {A_{1} \otimes A_{2} } \right) \otimes A_{3} = A_{1} \otimes \left( {A_{2} \otimes A_{3} } \right)$

Let the degree of positive membership of $\left( {A_{1} \otimes A_{2} } \right) \otimes A_{3}$ and $A_{1} \otimes \left( {A_{2} \otimes A_{3} } \right)$ be $\Phi_{{\left( {A_{1} \otimes A_{2} } \right) \otimes A_{3} }}$ and$\Phi_{{A_{1} \otimes \left( {A_{2} \otimes A_{3} } \right)}}$, the degree of neutral membership be $\Psi_{{\left( {A_{1} \otimes A_{2} } \right) \otimes A_{3} }}$ and$\Psi_{{A_{1} \otimes \left( {A_{2} \otimes A_{3} } \right)}}$, and the degree of negative membership be $\Upsilon_{{\left( {A_{1} \otimes A_{2} } \right) \otimes A_{3} }}$ and $\Upsilon_{{A_{1} \otimes \left( {A_{2} \otimes A_{3} } \right)}}$, respectively. Then, we can obtain

$$\begin{aligned} &\left[ {\Psi_{{\left( {A_{1} \otimes A_{2} } \right) \otimes A_{3} }}^{L} ,\Psi_{{\left( {A_{1} \otimes A_{2} } \right) \otimes A_{3} }}^{U} } \right] \hfill \\ &= \left[ \begin{aligned} \left( {\left( {\Psi_{1}^{L} } \right)^{q} + \left( {\Psi_{2}^{L} } \right)^{q} - \left( {\Psi_{1}^{L} } \right)^{q} \left( {\Psi_{2}^{L} } \right)^{q} + \left( {\Psi_{3}^{L} } \right)^{q} - \left( {\left( {\Psi_{1}^{L} } \right)^{q} + \left( {\Psi_{2}^{L} } \right)^{q} - \left( {\Psi_{1}^{L} } \right)^{q} \left( {\Psi_{2}^{L} } \right)^{q} } \right)\left( {\Psi_{3}^{L} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} , \hfill \\ \left( {\left( {\Psi_{1}^{U} } \right)^{q} + \left( {\Psi_{2}^{U} } \right)^{q} - \left( {\Psi_{1}^{U} } \right)^{q} \left( {\Psi_{2}^{U} } \right)^{q} + \left( {\Psi_{3}^{U} } \right)^{q} - \left( {\left( {\Psi_{1}^{U} } \right)^{q} + \left( {\Psi_{2}^{U} } \right)^{q} - \left( {\Psi_{1}^{U} } \right)^{q} \left( {\Psi_{2}^{U} } \right)^{q} } \right)\left( {\Psi_{3}^{U} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} \hfill \\ \end{aligned} \right] \hfill \\ &= \left[ \begin{aligned} \left( {\left( {\Psi_{1}^{L} } \right)^{q} + \left( {\Psi_{2}^{L} } \right)^{q} + \left( {\Psi_{3}^{L} } \right)^{q} - \left( {\Psi_{1}^{L} } \right)^{q} \left( {\Psi_{2}^{L} } \right)^{q} - \left( {\Psi_{1}^{L} } \right)^{q} \left( {\Psi_{3}^{L} } \right)^{q} - \left( {\Psi_{2}^{L} } \right)^{q} \left( {\Psi_{3}^{L} } \right)^{q} - \left( {\Psi_{1}^{L} } \right)^{q} \left( {\Psi_{2}^{L} } \right)^{q} \left( {\Psi_{3}^{L} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} , \hfill \\ \left( {\left( {\Psi_{1}^{U} } \right)^{q} + \left( {\Psi_{2}^{U} } \right)^{q} + \left( {\Psi_{3}^{U} } \right)^{q} - \left( {\Psi_{1}^{U} } \right)^{q} \left( {\Psi_{2}^{U} } \right)^{q} - \left( {\Psi_{1}^{U} } \right)^{q} \left( {\Psi_{3}^{U} } \right)^{q} - \left( {\Psi_{2}^{U} } \right)^{q} \left( {\Psi_{3}^{U} } \right)^{q} - \left( {\Psi_{1}^{U} } \right)^{q} \left( {\Psi_{2}^{U} } \right)^{q} \left( {\Psi_{3}^{U} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} \hfill \\ \end{aligned} \right] \hfill \\ \end{aligned}$$

$$\begin{aligned} &\left[ {\Psi_{{A_{1} \otimes \left( {A_{2} \otimes A_{3} } \right)}}^{L} ,\Psi_{{A_{1} \otimes \left( {A_{2} \otimes A_{3} } \right)}}^{U} } \right] \hfill \\ & = \left[ \begin{aligned} \left( {\left( {\Psi_{2}^{L} } \right)^{q} + \left( {\Psi_{3}^{L} } \right)^{q} - \left( {\Psi_{2}^{L} } \right)^{q} \left( {\Psi_{3}^{L} } \right)^{q} + \left( {\Psi_{1}^{L} } \right)^{q} - \left( {\left( {\Psi_{2}^{L} } \right)^{q} + \left( {\Psi_{3}^{L} } \right)^{q} - \left( {\Psi_{2}^{L} } \right)^{q} \left( {\Psi_{3}^{L} } \right)^{q} } \right)\left( {\Psi_{1}^{L} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} , \hfill \\ \left( {\left( {\Psi_{2}^{U} } \right)^{q} + \left( {\Psi_{3}^{U} } \right)^{q} - \left( {\Psi_{2}^{U} } \right)^{q} \left( {\Psi_{3}^{U} } \right)^{q} + \left( {\Psi_{1}^{U} } \right)^{q} - \left( {\left( {\Psi_{2}^{U} } \right)^{q} + \left( {\Psi_{3}^{U} } \right)^{q} - \left( {\Psi_{2}^{U} } \right)^{q} \left( {\Psi_{3}^{U} } \right)^{q} } \right)\left( {\Psi_{1}^{U} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} \hfill \\ \end{aligned} \right] \hfill \\ & = \left[ \begin{aligned} \left( {\left( {\Psi_{1}^{L} } \right)^{q} + \left( {\Psi_{2}^{L} } \right)^{q} + \left( {\Psi_{3}^{L} } \right)^{q} - \left( {\Psi_{1}^{L} } \right)^{q} \left( {\Psi_{2}^{L} } \right)^{q} - \left( {\Psi_{1}^{L} } \right)^{q} \left( {\Psi_{3}^{L} } \right)^{q} - \left( {\Psi_{2}^{L} } \right)^{q} \left( {\Psi_{3}^{L} } \right)^{q} - \left( {\Psi_{1}^{L} } \right)^{q} \left( {\Psi_{2}^{L} } \right)^{q} \left( {\Psi_{3}^{L} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} , \hfill \\ \left( {\left( {\Psi_{1}^{U} } \right)^{q} + \left( {\Psi_{2}^{U} } \right)^{q} + \left( {\Psi_{3}^{U} } \right)^{q} - \left( {\Psi_{1}^{U} } \right)^{q} \left( {\Psi_{2}^{U} } \right)^{q} - \left( {\Psi_{1}^{U} } \right)^{q} \left( {\Psi_{3}^{U} } \right)^{q} - \left( {\Psi_{2}^{U} } \right)^{q} \left( {\Psi_{3}^{U} } \right)^{q} - \left( {\Psi_{1}^{U} } \right)^{q} \left( {\Psi_{2}^{U} } \right)^{q} \left( {\Psi_{3}^{U} } \right)^{q} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} \hfill \\ \end{aligned} \right] \hfill \\ \end{aligned}$$

Then,

$$\Psi_{{\left( {A_{1} \otimes A_{2} } \right) \otimes A_{3} }} { = }\Psi_{{A_{1} \otimes \left( {A_{2} \otimes A_{3} } \right)}}$$

Similarly, we can deduce that

$$\Upsilon_{{\left( {A_{1} \otimes A_{2} } \right) \otimes A_{3} }} { = }\Upsilon_{{A_{1} \otimes \left( {A_{2} \otimes A_{3} } \right)}}$$

$$\Phi_{{\left( {A_{1} \otimes A_{2} } \right) \otimes A_{3} }} { = }\Phi_{{A_{1} \otimes \left( {A_{2} \otimes A_{3} } \right)}}$$

Therefore, $\left( {A_{1} \otimes A_{2} } \right) \otimes A_{3} = A_{1} \otimes \left( {A_{2} \otimes A_{3} } \right)$

For (8), $A_{1}^{{\lambda_{1} }} \otimes A_{1}^{{\lambda_{2} }} = A_{1}^{{\lambda_{1} + \lambda_{2} }}$

Let $A_{1} = \left\langle {\left[ {\Phi_{1}^{L} ,\Phi_{1}^{U} } \right],\left[ {\Psi_{1}^{L} ,\Psi_{1}^{U} } \right],\left[ {\Upsilon_{1}^{L} ,\Upsilon_{1}^{U} } \right]} \right\rangle$ be IVq-RPtFNs and $\lambda_{1} ,\lambda_{2}$ be nonnegative real numbers. Then, we obtain

$$\begin{aligned} & \left( {\left[ {\Psi_{1}^{L} ,\Psi_{1}^{U} } \right]} \right)^{{\lambda_{1} }} \otimes \left( {\left[ {\Psi_{1}^{L} ,\Psi_{1}^{U} } \right]} \right)^{{\lambda_{2} }} \hfill \\ &{ = } \left[ \begin{aligned} \left( {1 - \left( {1 - \left( {\Psi_{1}^{L} } \right)^{q} } \right)^{{\lambda_{1} }} { + }1 - \left( {1 - \left( {\Psi_{1}^{L} } \right)^{q} } \right)^{{\lambda_{2} }} - \left( {\left( {1 - \left( {1 - \left( {\Psi_{1}^{L} } \right)^{q} } \right)^{{\lambda_{1} }} } \right)\left( {1 - \left( {1 - \left( {\Psi_{1}^{L} } \right)^{q} } \right)^{{\lambda_{2} }} } \right)} \right)} \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} , \hfill \\ \left( {1 - \left( {1 - \left( {\Psi_{1}^{U} } \right)^{q} } \right)^{{\lambda_{1} }} { + }1 - \left( {1 - \left( {\Psi_{1}^{U} } \right)^{q} } \right)^{{\lambda_{2} }} - \left( {\left( {1 - \left( {1 - \left( {\Psi_{1}^{U} } \right)^{q} } \right)^{{\lambda_{1} }} } \right)\left( {1 - \left( {1 - \left( {\Psi_{1}^{U} } \right)^{q} } \right)^{{\lambda_{2} }} } \right)} \right)} \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} \hfill \\ \end{aligned} \right] \hfill \\ & { = } \left[ \begin{aligned} \left( {1 - \left( {1 - \left( {\Psi_{1}^{L} } \right)^{q} } \right)^{{\lambda_{1} }} { + }1 - \left( {1 - \left( {\Psi_{1}^{L} } \right)^{q} } \right)^{{\lambda_{2} }} - \left( {\left( {1 - \left( {1 - \left( {\Psi_{1}^{L} } \right)^{q} } \right)^{{\lambda_{1} }} } \right)\left( {1 - \left( {1 - \left( {\Psi_{1}^{L} } \right)^{q} } \right)^{{\lambda_{2} }} } \right)} \right)} \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} , \hfill \\ \left( {1 - \left( {1 - \left( {\Psi_{1}^{U} } \right)^{q} } \right)^{{\lambda_{1} }} { + }1 - \left( {1 - \left( {\Psi_{1}^{U} } \right)^{q} } \right)^{{\lambda_{2} }} - \left( {\left( {1 - \left( {1 - \left( {\Psi_{1}^{U} } \right)^{q} } \right)^{{\lambda_{1} }} } \right)\left( {1 - \left( {1 - \left( {\Psi_{1}^{U} } \right)^{q} } \right)^{{\lambda_{2} }} } \right)} \right)} \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} \hfill \\ \end{aligned} \right] \hfill \\ & { = } \left[ \begin{aligned} \left( {1 - \left( {1 - \left( {\Psi_{1}^{L} } \right)^{q} } \right)^{{\lambda_{1} }} { + }\left( {1 - \left( {1 - \left( {\Psi_{1}^{L} } \right)^{q} } \right)^{{\lambda_{2} }} } \right)\left( {1 - \left( {\Psi_{1}^{L} } \right)^{q} } \right)^{{\lambda_{1} }} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} , \hfill \\ \left( {1 - \left( {1 - \left( {\Psi_{1}^{U} } \right)^{q} } \right)^{{\lambda_{1} }} { + }\left( {1 - \left( {1 - \left( {\Psi_{1}^{U} } \right)^{q} } \right)^{{\lambda_{2} }} } \right)\left( {1 - \left( {\Psi_{1}^{U} } \right)^{q} } \right)^{{\lambda_{1} }} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} \hfill \\ \end{aligned} \right] \hfill \\ & = \left[ {1 - \left( {1 - \left( {\Psi_{1}^{L} } \right)^{q} } \right)^{{\lambda_{1} { + }\lambda_{2} }} ,1 - \left( {1 - \left( {\Psi_{1}^{U} } \right)^{q} } \right)^{{\lambda_{1} { + }\lambda_{2} }} } \right] \hfill \\ &{ =} \left( {\left[ {\Psi_{1}^{L} ,\Psi_{1}^{U} } \right]} \right)^{{\lambda_{1} { + }\lambda_{2} }} \hfill \\ \end{aligned}$$

Similarly, we can deduce that

$$\left( {\left[ {\Psi_{1}^{L} ,\Psi_{1}^{U} } \right]} \right)^{{\lambda_{1} }} \otimes \left( {\left[ {\Psi_{1}^{L} ,\Psi_{1}^{U} } \right]} \right)^{{\lambda_{2} }} { = }\left( {\left[ {\Psi_{1}^{L} ,\Psi_{1}^{U} } \right]} \right)^{{\lambda_{1} { + }\lambda_{2} }}$$

$$\left( {\left[ {\Upsilon_{1}^{L} ,\Upsilon_{1}^{U} } \right]} \right)^{{\lambda_{1} }} \otimes \left( {\left[ {\Upsilon_{1}^{L} ,\Upsilon_{1}^{U} } \right]} \right)^{{\lambda_{2} }} { = }\left( {\left[ {\Upsilon_{1}^{L} ,\Upsilon_{1}^{U} } \right]} \right)^{{\lambda_{1} { + }\lambda_{2} }}$$

Therefore, $A_{1}^{{\lambda_{1} }} \otimes A_{1}^{{\lambda_{2} }} = A_{1}^{{\lambda_{1} + \lambda_{2} }}$

Appendix 4

Proof

First, based on the power operation of IVq-RPtFNs in Definition 6, we conduct the h power operation of $A_{i}$ and s power operation of $A_{j}$. Specifically, for the h power operation of$A_{i}$, we need to compute the h power operations of the lower bounds and upper bounds of the POSMEBDs, $\left( {\Phi_{i}^{L} } \right)^{h}$ and$\left( {\Phi_{i}^{U} } \right)^{h}$; the h power operations of the lower bounds and upper bounds of the NEUMEBDs, including $\left( {1 - \left( {1 - \left( {\Psi_{i}^{L} } \right)^{q} } \right)^{h} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$ and$\left( {1 - \left( {1 - \left( {\Psi_{i}^{U} } \right)^{q} } \right)^{h} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$; and the h power operations of the lower bounds and upper bounds of the NEGMEBDs, $\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{L} } \right)^{q} } \right)^{h} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$ and$\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{U} } \right)^{q} } \right)^{h} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}}$, respectively.

From these calculations, we obtain

$$A^h_i = \begin{pmatrix} \begin{aligned} &\left[\left(\Phi^L_i\right)^h, \left(\Phi^U_i\right)^h\right] \\ &\begin{bmatrix} \left(1 -\left(1 - \left(\Psi^L_i\right)^q\right)^h \right)^{1 \left / \right. q}, \\ \left(1 -\left(1 - \left(\Psi^U_i\right)^q\right)^h\right)^{1 \left / \right. q}\end{bmatrix} \\ &\begin{bmatrix} \left(1 -\left(1 - \left(\Upsilon^L_i\right)^q\right)^h\right)^{1 \left / \right. q}, \\ \left(1 -\left(1 - \left(\Upsilon^U_i\right)^q\right)^h\right)^{1 \left / \right. q}\end{bmatrix}\end{aligned}\end{pmatrix}$$

 

Similarly, we obtain the s power operation of $A_{j}$ as follows:

$$A^s_i = \begin{pmatrix} \begin{aligned} &\left[\left(\Phi^L_i\right)^s, \left(\Phi^U_i\right)^s\right] \\ &\begin{bmatrix} \left(1 -\left(1 - \left(\Psi^L_i\right)^q\right)^s\right)^{1 \left / \right. q}, \\ \left(1 -\left(1 - \left(\Psi^U_i\right)^q\right)^s\right)^{1 \left / \right. q}\end{bmatrix} \\ &\begin{bmatrix} \left(1 -\left(1 - \left(\Upsilon^L_i\right)^q\right)^s\right)^{1 \left / \right. q}, \\ \left(1 -\left(1 - \left(\Upsilon^U_i\right)^q\right)^s\right)^{1 \left / \right. q}\end{bmatrix}\end{aligned} \end{pmatrix}$$

We easily determine that $A_{i}^{h}$ and $A_{i}^{s}$ are still IVq-RPtFNs.

Then, by the product operation between IVq-RPtFNs, $A_{i}^{h}$ and $A_{i}^{s}$ are multiplied. That is the product operations of the lower bounds and upper bounds in POSMEBDs, NEUMEBDs, and NEGMEBDs as follows:

$$\begin{aligned} & \left( {A_{i} } \right)^{h} \otimes \left( {A_{j} } \right)^{s} \hfill \\ & = \left( \begin{aligned} & \left[ {\left( {\Phi_{i}^{L} } \right)^{h} \left( {\Phi_{j}^{L} } \right)^{h} ,\left( {\Phi_{i}^{U} } \right)^{h} \left( {\Phi_{j}^{U} } \right)^{h} } \right], \hfill \\ &\left[ \begin{aligned} \left( {1 - \left( {1 - \left( {\Psi_{i}^{L} } \right)^{q} } \right)^{h} + 1 - \left( {1 - \left( {\Psi_{j}^{L} } \right)^{q} } \right)^{h} - \left( {1 - \left( {1 - \left( {\Psi_{i}^{L} } \right)^{q} } \right)^{h} } \right)\left( {1 - \left( {1 - \left( {\Psi_{j}^{L} } \right)^{q} } \right)^{h} } \right)} \right)^{\frac{1}{q}} , \hfill \\ \left( {1 - \left( {1 - \left( {\Phi_{i}^{U} } \right)^{q} } \right)^{h} + 1 - \left( {1 - \left( {\Psi_{j}^{U} } \right)^{q} } \right)^{h} - \left( {1 - \left( {1 - \left( {\Psi_{i}^{U} } \right)^{q} } \right)^{h} } \right)\left( {1 - \left( {1 - \left( {\Psi_{j}^{U} } \right)^{q} } \right)^{h} } \right)} \right)^{\frac{1}{q}} \hfill \\ \end{aligned} \right], \hfill \\ & \left[ \begin{aligned} \left( {1 - \left( {1 - \left( {\Upsilon_{i}^{L} } \right)^{q} } \right)^{h} + 1 - \left( {1 - \left( {\Upsilon_{j}^{L} } \right)^{q} } \right)^{h} - \left( {1 - \left( {1 - \left( {\Upsilon_{i}^{L} } \right)^{q} } \right)^{h} } \right)\left( {1 - \left( {1 - \left( {\Upsilon_{j}^{L} } \right)^{q} } \right)^{h} } \right)} \right)^{\frac{1}{q}} , \hfill \\ \left( {1 - \left( {1 - \left( {\Upsilon_{i}^{U} } \right)^{q} } \right)^{h} + 1 - \left( {1 - \left( {\Upsilon_{j}^{U} } \right)^{q} } \right)^{h} - \left( {1 - \left( {1 - \left( {\Upsilon_{i}^{U} } \right)^{q} } \right)^{h} } \right)\left( {1 - \left( {1 - \left( {\Upsilon_{j}^{U} } \right)^{q} } \right)^{h} } \right)} \right)^{\frac{1}{q}} \hfill \\ \end{aligned} \right] \hfill \\ \hfill \\ \end{aligned} \right) \hfill \\ \end{aligned}$$

In addition, as $i = 1,2, \cdots ,n$, we need to perform n product operations between $A_{i}^{h}$ and $A_{i}^{s}$ and add them together. According the addition operation rules of IVq-RPtFNs and the mathematical induction method, we obtain

$$\begin{aligned} &\sum\limits_{i = 1}^{n} {\left( {A_{i} } \right)^{h} \otimes \left( {A_{j} } \right)^{s} } \hfill \\ &= \left( \begin{aligned} & \left[ {\left( {1 - \prod\limits_{i = 1}^{n} {\left( {{1} - \left( {\left( {\Phi_{i}^{L} } \right)^{h} \left( {\Phi_{j}^{L} } \right)^{s} } \right)^{q} } \right)} } \right)^{\frac{1}{q}} ,\left( {1 - \prod\limits_{i = 1}^{n} {\left( {{1} - \left( {\left( {\Phi_{i}^{U} } \right)^{h} \left( {\Phi_{j}^{U} } \right)^{s} } \right)^{q} } \right)} } \right)^{\frac{1}{q}} } \right], \hfill \\ &\left[ {\prod\limits_{i = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{L} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Psi_{j}^{L} } \right)^{q} } \right)^{s} } \right)^{\frac{1}{q}} ,\prod\limits_{i = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{U} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Psi_{j}^{U} } \right)^{q} } \right)^{s} } \right)^{\frac{1}{q}} } } } \right], \hfill \\ & \left[ {\prod\limits_{i = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{L} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Upsilon_{j}^{L} } \right)^{q} } \right)^{s} } \right)^{\frac{1}{q}} ,\prod\limits_{i = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{U} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Upsilon_{j}^{U} } \right)^{q} } \right)^{s} } \right)^{\frac{1}{q}} } } } \right] \hfill \\ \end{aligned} \right) \hfill \\ \end{aligned}$$

Similarly, because$j = 1,2, \cdots ,n{\kern 1pt} {\kern 1pt} (i \ne j)$,

we need to perform n product operations between $A_{i}^{h}$ and $A_{i}^{s}$ and add them together. Thus, we obtain

$$\begin{aligned} & \sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A_{i} } \right)^{h} \otimes \left( {A_{j} } \right)^{s} } } \hfill \\ &= \left( \begin{aligned}& \left[ {\left( {1 - \prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {{1} - \left( {\left( {\Phi_{i}^{L} } \right)^{h} \left( {\Phi_{j}^{L} } \right)^{s} } \right)^{q} } \right)} } } \right)^{\frac{1}{q}} ,\left( {1 - \prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {{1} - \left( {\left( {\Phi_{i}^{U} } \right)^{h} \left( {\Phi_{j}^{U} } \right)^{s} } \right)^{q} } \right)} } } \right)^{\frac{1}{q}} } \right], \hfill \\ &\left[ {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{L} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Psi_{j}^{L} } \right)^{q} } \right)^{s} } \right)^{\frac{1}{q}} } ,\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{U} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Psi_{j}^{U} } \right)^{q} } \right)^{s} } \right)^{\frac{1}{q}} } } } } \right], \hfill \\& \left[ {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{L} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Upsilon_{j}^{L} } \right)^{q} } \right)^{s} } \right)}^{\frac{1}{q}} ,\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{U} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Upsilon_{j}^{U} } \right)^{q} } \right)^{s} } \right)^{\frac{1}{q}} } } } } \right] \hfill \\ \end{aligned} \right) \hfill \\ \end{aligned}$$

Since $\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A_{i} } \right)^{h} \otimes \left( {A_{j} } \right)^{s} } }$ is still an IVq-RPtFN, we conduct scalar multiplication between $\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A_{i} } \right)^{h} \otimes \left( {A_{j} } \right)^{s} } }$ and$\frac{2}{n(n + 1)}$. According to Definition 6, we obtain

$$\frac{2}{n(n + 1)}\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A_{i} } \right)^{h} \otimes \left( {A_{j} } \right)^{s} } } = \left( \begin{aligned} &\left[ \begin{aligned} \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {{1} - \left( {\left( {\Phi_{i}^{L} } \right)^{h} \left( {\Phi_{j}^{L} } \right)^{s} } \right)^{q} } \right)} } } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{\frac{1}{q}} , \hfill \\ \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {{1} - \left( {\left( {\Phi_{i}^{U} } \right)^{h} \left( {\Phi_{j}^{U} } \right)^{s} } \right)^{q} } \right)} } } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{\frac{1}{q}} \hfill \\ \end{aligned} \right], \hfill \\ &\left[ \begin{aligned} \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{L} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Psi_{j}^{L} } \right)^{q} } \right)^{s} } \right)^{\frac{1}{q}} } } } \right)^{{\frac{2}{n(n + 1)}}} , \hfill \\ \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{U} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Psi_{j}^{U} } \right)^{q} } \right)^{s} } \right)^{\frac{1}{q}} } } } \right)^{{\frac{2}{n(n + 1)}}} \hfill \\ \end{aligned} \right], \hfill \\ &\left[ \begin{aligned} \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{L} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Upsilon_{j}^{L} } \right)^{q} } \right)^{s} } \right)^{\frac{1}{q}} } } } \right)^{{\frac{2}{n(n + 1)}}} , \hfill \\ \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{U} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Upsilon_{j}^{U} } \right)^{q} } \right)^{s} } \right)^{\frac{1}{q}} } } } \right)^{{\frac{2}{n(n + 1)}}} \hfill \\ \end{aligned} \right] \hfill \\ \end{aligned} \right)$$

Then, based on the power operation of IVq-RPtFNs, we compute the $\frac{1}{h + s}$ power operation of$\frac{2}{n(n + 1)}\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A_{i} } \right)^{h} \otimes \left( {A_{j} } \right)^{s} } }$, and the result is

$$\left( {\frac{2}{n(n + 1)}\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A_{i} } \right)^{h} \otimes \left( {A_{j} } \right)^{s} } } } \right)^{{\frac{1}{h + s}}} = \left( \begin{aligned}& \left[ \begin{aligned} \left( {\left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {{1} - \left( {\left( {\Phi_{i}^{L} } \right)^{h} \left( {\Phi_{j}^{L} } \right)^{s} } \right)^{q} } \right)} } } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{\frac{1}{q}} } \right)^{{\frac{1}{h + s}}} , \hfill \\ \left( {\left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {{1} - \left( {\left( {\Phi_{i}^{U} } \right)^{h} \left( {\Phi_{j}^{U} } \right)^{s} } \right)^{q} } \right)} } } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{\frac{1}{q}} } \right)^{{\frac{1}{h + s}}} \hfill \\ \end{aligned} \right], \hfill \\ & \left[ \begin{aligned} \left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{L} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Psi_{j}^{L} } \right)^{q} } \right)^{s} } \right)} } } \right)^{{\frac{{2}}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{\frac{1}{q}} , \hfill \\ \left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{U} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Psi_{j}^{U} } \right)^{q} } \right)^{s} } \right)} } } \right)^{{\frac{{2}}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{\frac{1}{q}} \hfill \\ \end{aligned} \right], \hfill \\ &\left[ \begin{aligned} \left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{L} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Upsilon_{j}^{L} } \right)^{q} } \right)^{s} } \right)} } } \right)^{{\frac{{2}}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{\frac{1}{q}} , \hfill \\ \left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{U} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Upsilon_{j}^{U} } \right)^{q} } \right)^{s} } \right)} } } \right)^{{\frac{{2}}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{\frac{1}{q}} \hfill \\ \end{aligned} \right] \hfill \\ \end{aligned} \right)$$

Thus, we have proven Theorem 1.

Appendix 5

The proof of Theorem 2

Proof

Because $A_{i}$ is equal to A for any i, we can obtain.

$$\begin{aligned} {\text{IVq - RPtFHM}}\left( {A_{1} ,A_{2} , \cdots ,A_{n} } \right) &= \left( {\frac{2}{n(n + 1)}\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A_{i} } \right)^{h} \otimes \left( {A_{j} } \right)^{s} } } } \right)^{{\frac{1}{h + s}}} \hfill \\ &= \left( {\frac{2}{n(n + 1)}\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( A \right)^{h} \otimes \left( A \right)^{s} } } } \right)^{{\frac{1}{h + s}}} \hfill \\ &= \left( {\frac{2}{n(n + 1)}\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( A \right)^{h + s} } } } \right)^{{\frac{1}{h + s}}} { = }A \hfill \\ \end{aligned}$$

Therefore,${\text{IVq - RPtFHM}}\left( {A_{1} ,A_{2} , \cdots ,A_{n} } \right) = A$.

The proof of Theorem 3

Proof

Based on Theorem 2, we can obtain

$$\begin{aligned} IVq - RPtFHM\left( {A_{1} ,A_{2} , \cdots ,A_{n} } \right) \hfill &= \left( {\frac{2}{n(n + 1)}\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A_{i} } \right)^{h} \otimes \left( {A_{j} } \right)^{s} } } } \right)^{{\frac{1}{h + s}}} \hfill \\ & \le \left( {\frac{2}{n(n + 1)}\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A^{ + } } \right)^{h} \otimes \left( {A^{ + } } \right)^{s} } } } \right)^{{\frac{1}{h + s}}} \hfill \\ & \le \left( {\frac{2}{n(n + 1)}\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A^{ + } } \right)^{h + s} } } } \right)^{{\frac{1}{h + s}}} { = }A^{ + } \hfill \\ \end{aligned}$$

Similarly, we can obtain $A^{ - } \le {\text{IVq - RPtFHM}}\left( {A_{1} ,A_{2} , \cdots ,A_{n} } \right)$,

Therefore, $A^{ - } \le {\text{IVq - RPtFHM}}\left( {A_{1} ,A_{2} , \cdots ,A_{n} } \right) \le A^{ + }$.

The proof of Theorem 4

Proof

Based on Theorems 3 and 4, for any $i$, there is $\Phi_{{A_{i} }}^{L} \le \Phi_{{B_{i} }}^{L} ,{\kern 1pt} {\kern 1pt} \Phi_{{A_{i} }}^{U} \le \Phi_{{B_{i} }}^{U}$ and$\Psi_{{A_{i} }}^{L} \ge \Psi_{{B_{i} }}^{L} ,{\kern 1pt} {\kern 1pt} \Psi_{{A_{i} }}^{U} \ge \Psi_{{B_{i} }}^{U}$,$\Upsilon_{{A_{i} }}^{L} \ge \Upsilon_{{B_{i} }}^{L} ,{\kern 1pt} {\kern 1pt} \Upsilon_{{A_{i} }}^{U} \ge \Upsilon_{{B_{i} }}^{U}$

Then,

$$\begin{aligned} \left( {\left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {{1} - \left( {\left( {\Phi_{{A_{i} }}^{L} } \right)^{h} \left( {\Phi_{{A_{j} }}^{L} } \right)^{s} } \right)^{q} } \right)} } } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{\frac{1}{q}} } \right)^{{\frac{1}{g + l}}} \le \left( {\left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {{1} - \left( {\left( {\Phi_{{B_{i} }}^{L} } \right)^{h} \left( {\Phi_{{B_{j} }}^{L} } \right)^{s} } \right)^{q} } \right)} } } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{\frac{1}{q}} } \right)^{{\frac{1}{g + l}}} , \hfill \\ \left( {\left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {{1} - \left( {\left( {\Phi_{{A_{i} }}^{U} } \right)^{h} \left( {\Phi_{{A_{j} }}^{U} } \right)^{s} } \right)^{q} } \right)} } } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{\frac{1}{q}} } \right)^{{\frac{1}{g + l}}} \le \left( {\left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {{1} - \left( {\left( {\Phi_{{B_{i} }}^{U} } \right)^{h} \left( {\Phi_{{B_{j} }}^{U} } \right)^{s} } \right)^{q} } \right)} } } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{\frac{1}{q}} } \right)^{{\frac{1}{g + l}}} \hfill \\ \end{aligned}$$

and

$$\begin{aligned} &\left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Phi_{{A_{i} }}^{L} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Phi_{{A_{j} }}^{L} } \right)^{q} } \right)^{s} } \right)} } } \right)^{{\frac{{2}}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{\frac{1}{q}} \hfill \\ & \quad \ge \left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Phi_{{B_{i} }}^{L} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Phi_{{B_{j} }}^{L} } \right)^{q} } \right)^{s} } \right)} } } \right)^{{\frac{{2}}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{\frac{1}{q}} , \hfill \\ &\left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Phi_{{A_{i} }}^{U} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Phi_{{A_{j} }}^{U} } \right)^{q} } \right)^{s} } \right)} } } \right)^{{\frac{{2}}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{\frac{1}{q}} \hfill \\ & \quad \ge \left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Phi_{{B_{i} }}^{U} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Phi_{{B_{j} }}^{U} } \right)^{q} } \right)^{s} } \right)} } } \right)^{{\frac{{2}}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{\frac{1}{q}} \hfill \\ \end{aligned}$$

and

$\begin{aligned} &\left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{{A_{i} }}^{L} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Upsilon_{{A_{j} }}^{L} } \right)^{q} } \right)^{s} } \right)} } } \right)^{{\frac{{2}}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{\frac{1}{q}} \hfill \\ &\quad \ge \left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{{B_{i} }}^{L} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Upsilon_{{B_{j} }}^{L} } \right)^{q} } \right)^{s} } \right)} } } \right)^{{\frac{{2}}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{\frac{1}{q}} , \hfill \\ &\left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{{A_{i} }}^{U} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Upsilon_{{A_{j} }}^{U} } \right)^{q} } \right)^{s} } \right)} } } \right)^{{\frac{{2}}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{\frac{1}{q}} \hfill \\ & \quad \ge \left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{{B_{i} }}^{U} } \right)^{q} } \right)^{h} \left( {1 - \left( {\Upsilon_{{B_{j} }}^{U} } \right)^{q} } \right)^{s} } \right)} } } \right)^{{\frac{{2}}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{\frac{1}{q}} \hfill \\ \end{aligned}$.

Based on Definitions 9 and 10, we can obtain

${\text{IVq - RPtFHM}}\left( {A_{1} ,A_{2} , \cdots ,A_{n} } \right) \le {\text{IVq - RPtFHM}}\left( {B_{1} ,B_{2} , \cdots ,B_{n} } \right)$.

Appendix 6

Proof

Based on the operations of IVq-RPtFNs, we can obtain the following.

First, we carry out scalar multiplication and power operations on $A_{i}$ and $A_{j}$ according to the expression structure in Definition 11. Considering the operations of $A_{i}$ as an example, we first utilize the scalar multiplication of IVq-RPtFNs in Definition 6 to multiply $A_{i}$ and$w_{i}$, that is, to multiply the lower bounds and upper bounds in POSMEBDs, NEUMEBDs, and NEGMEBDs in $A_{i}$ with the real number$w_{i}$. Then, we find that the interval POSMEBD of $A_{i} w_{i}$ is $\left[ {\left( {1 - \left( {1 - \left( {\Phi_{i}^{L} } \right)^{q} } \right)^{{w_{i} }} } \right)^{\frac{1}{q}} ,\left( {1 - \left( {1 - \left( {\Phi_{i}^{U} } \right)^{q} } \right)^{{w_{i} }} } \right)^{\frac{1}{q}} } \right]$, the interval NEUMEBD of $A_{i} w_{i}$ is $\left[ {\left( {\Psi_{i}^{L} } \right)^{{w_{i} }} ,\left( {\Psi_{i}^{U} } \right)^{{w_{i} }} } \right]$, and the interval NEGMEBD of $A_{i} w_{i}$ is $\left[ {\left( {\Upsilon_{i}^{L} } \right)^{{w_{i} }} ,\left( {\Upsilon_{i}^{U} } \right)^{{w_{i} }} } \right]$. Finally, we can obtain

$$A_{i} w_{i} { = }\left( {\left[ {\left( {1 - \left( {1 - \left( {\Phi_{i}^{L} } \right)^{q} } \right)^{{w_{i} }} } \right)^{\frac{1}{q}} ,\left( {1 - \left( {1 - \left( {\Phi_{i}^{U} } \right)^{q} } \right)^{{w_{i} }} } \right)^{\frac{1}{q}} } \right],\left[ {\left( {\Psi_{i}^{L} } \right)^{{w_{i} }} ,\left( {\Psi_{i}^{U} } \right)^{{w_{i} }} } \right],\left[ {\left( {\Upsilon_{i}^{L} } \right)^{{w_{i} }} ,\left( {\Upsilon_{i}^{U} } \right)^{{w_{i} }} } \right]} \right)$$

Then, we introduce the power operation of IVq-RPtFNs in Definition 6 to perform the h power operation of$A_{i} w_{i}$, and we find that the interval POSMEBD of $\left( {A_{i} w_{i} } \right)^{h}$ is$\left[ {\left( {1 - \left( {1 - \left( {\Phi_{i}^{L} } \right)^{q} } \right)^{{w_{i} }} } \right)^{\frac{h}{q}} ,\left( {1 - \left( {1 - \left( {\Phi_{i}^{U} } \right)^{q} } \right)^{{w_{i} }} } \right)^{\frac{h}{q}} } \right]$, the interval NEUMEBD of $\left( {A_{i} w_{i} } \right)^{h}$ is$\left[ {\left( {1 - \left( {1 - \left( {\Psi_{i}^{L} } \right)^{{w_{i} q}} } \right)^{h} } \right)^{\frac{1}{q}} ,\left( {1 - \left( {1 - \left( {\Psi_{i}^{U} } \right)^{{w_{i} q}} } \right)^{h} } \right)^{\frac{1}{q}} } \right]$, and the interval NEGMEBD of $\left( {A_{i} w_{i} } \right)^{h}$ is$\left[ {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{L} } \right)^{{w_{i} q}} } \right)^{h} } \right)^{\frac{1}{q}} ,\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{U} } \right)^{{w_{i} q}} } \right)^{h} } \right)^{\frac{1}{q}} } \right]$. Then, we obtain

$$\left( {A_{i} w_{i} } \right)^{h} { = }\left( \begin{aligned} \left[ {\left( {1 - \left( {1 - \left( {\Phi_{i}^{L} } \right)^{q} } \right)^{{w_{i} }} } \right)^{\frac{h}{q}} ,\left( {1 - \left( {1 - \left( {\Phi_{i}^{U} } \right)^{q} } \right)^{{w_{i} }} } \right)^{\frac{h}{q}} } \right], \hfill \\ \left[ {\left( {1 - \left( {1 - \left( {\Psi_{i}^{L} } \right)^{{w_{i} q}} } \right)^{h} } \right)^{\frac{1}{q}} ,\left( {1 - \left( {1 - \left( {\Psi_{i}^{U} } \right)^{{w_{i} q}} } \right)^{h} } \right)^{\frac{1}{q}} } \right], \hfill \\ \left[ {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{L} } \right)^{{w_{i} q}} } \right)^{h} } \right)^{\frac{1}{q}} ,\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{U} } \right)^{{w_{i} q}} } \right)^{h} } \right)^{\frac{1}{q}} } \right] \hfill \\ \end{aligned} \right)$$

Similarly, we calculate the result of the scalar multiplication between $A_{j}$ and $w_{j}$ as follows:

$$A_{j} w_{j} { = }\left( {\left[ {\left( {1 - \left( {1 - \left( {\Phi_{j}^{L} } \right)^{q} } \right)^{{w_{j} }} } \right)^{\frac{1}{q}} ,\left( {1 - \left( {1 - \left( {\Phi_{j}^{U} } \right)^{q} } \right)^{{w_{j} }} } \right)^{\frac{1}{q}} } \right],\left[ {\left( {\Psi_{j}^{L} } \right)^{{w_{j} }} ,\left( {\Psi_{j}^{U} } \right)^{{w_{j} }} } \right],\left[ {\left( {\Upsilon_{j}^{L} } \right)^{{w_{j} }} ,\left( {\Upsilon_{j}^{U} } \right)^{{w_{j} }} } \right]} \right)$$

and the s power operation of $A_{j} w_{j}$ as

$$\left( {A_{j} w_{j} } \right)^{s} { = }\left( \begin{aligned} & \left[ {\left( {1 - \left( {1 - \left( {\Phi_{j}^{L} } \right)^{q} } \right)^{{w_{j} }} } \right)^{\frac{s}{q}} ,\left( {1 - \left( {1 - \left( {\Phi_{j}^{U} } \right)^{q} } \right)^{{w_{j} }} } \right)^{\frac{s}{q}} } \right], \hfill \\ & \left[ {\left( {1 - \left( {1 - \left( {\Psi_{j}^{L} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} ,\left( {1 - \left( {1 - \left( {\Psi_{j}^{U} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} } \right], \hfill \\ & \left[ {\left( {1 - \left( {1 - \left( {\Upsilon_{j}^{L} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} ,\left( {1 - \left( {1 - \left( {\Upsilon_{j}^{U} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} } \right] \hfill \\ \end{aligned} \right)$$

Then, as the proof step in Theorem 2, we apply the product operations of IVq-RPtFNs in Definition 6 to multiply $\left( {A_{i} w_{i} } \right)^{h}$ and $\left( {A_{j} w_{j} } \right)^{s}$ as follows:

$\begin{aligned}&(A_i w_i)^h \otimes (A_j w_j)^s \\ &=\begin{pmatrix} \begin{aligned}&\begin{bmatrix} \left(1 -\left(1 - \left(\Phi_i^L \right)^q\right)^{w_i}\right)^\frac{h}{q} \left(1 -\left(1 - \left(\Phi_i^L \right)^q\right)^{w_i}\right)^\frac{s}{q}, \\ \left(1 -\left(1 - \left(\Phi_i^U \right)^q\right)^{w_i}\right)^\frac{h}{q} \left(1 -\left(1 - \left(\Phi_i^U \right)^q\right)^{w_i}\right)^\frac{s}{q}\end{bmatrix} \\ & \begin{bmatrix} \begin{aligned} &\begin{pmatrix}\begin{aligned}&\left(\left(1 - \left(1 -\left(\Psi^L_i\right)^{w_iq}\right)^h\right)^\frac{l}{e}\right)^q + \left(\left(1 - \left(1 -\left(\Psi^L_i\right)^{w_iq}\right)^s\right)^\frac{l}{e}\right)^q \\& -\left(\left(1 - \left(1 -\left(\Psi^L_i\right)^{w_iq}\right)^h\right)^\frac{l}{e}\right)^q \left(\left(1 - \left(1 -\left(\Psi^L_i\right)^{w_iq}\right)^s\right)^\frac{l}{q}\right)^q, \end{aligned}\end{pmatrix}^\frac{1}{q} \\ &\begin{pmatrix}\begin{aligned}&\left(\left(1 - \left(1 -\left(\Psi^U_i\right)^{w_iq}\right)^h\right)^\frac{l}{e}\right)^q + \left(\left(1 - \left(1 -\left(\Psi^U_i\right)^{w_iq}\right)^s\right)^\frac{l}{e}\right)^q \\& -\left(\left(1 - \left(1 -\left(\Psi^U_i\right)^{w_iq}\right)^h\right)^\frac{l}{e}\right)^q \left(\left(1 - \left(1 -\left(\Psi^U_i\right)^{w_iq}\right)^s\right)^\frac{l}{q}\right)^q, \end{aligned}\end{pmatrix}^\frac{1}{q} \end{aligned} \end{bmatrix}, \\ &\begin{bmatrix} \begin{aligned} &\begin{pmatrix}\begin{aligned}&\left(\left(1 - \left(1 -\left(\Upsilon^L_i\right)^{w_iq}\right)^h\right)^\frac{l}{e}\right)^q + \left(\left(1 - \left(1 -\left(\Upsilon^L_i\right)^{w_iq}\right)^s\right)^\frac{l}{e}\right)^q \\& -\left(\left(1 - \left(1 -\left(\Upsilon^L_i\right)^{w_iq}\right)^h\right)^\frac{l}{e}\right)^q \left(\left(1 - \left(1 -\left(\Upsilon^L_i\right)^{w_iq}\right)^s\right)^\frac{l}{q}\right)^q, \end{aligned}\end{pmatrix}^\frac{1}{q}, \\ &\begin{pmatrix}\begin{aligned}&\left(\left(1 - \left(1 -\left(\Upsilon^U_i\right)^{w_iq}\right)^h\right)^\frac{l}{e}\right)^q + \left(\left(1 - \left(1 -\left(\Upsilon^U_i\right)^{w_iq}\right)^s\right)^\frac{l}{e}\right)^q \\& -\left(\left(1 - \left(1 -\left(\Upsilon^U_i\right)^{w_iq}\right)^h\right)^\frac{l}{e}\right)^q \left(\left(1 - \left(1 -\left(\Upsilon^U_i\right)^{w_iq}\right)^s\right)^\frac{l}{q}\right)^q, \end{aligned}\end{pmatrix}^\frac{1}{q}, \end{aligned} \end{bmatrix}\end{aligned}\end{pmatrix}\\ & = \begin{pmatrix}\begin{aligned}& \begin{bmatrix}\begin{aligned} \left(1 -\left(1 - \left(\Phi_i^L \right)^q\right)^{w_i}\right)^\frac{h}{q} \left(1 -\left(1 - \left(\Phi_i^L \right)^q\right)^{w_i}\right)^\frac{s}{q}, \\ \left(1 -\left(1 - \left(\Phi_i^U \right)^q\right)^{w_i}\right)^\frac{h}{q} \left(1 -\left(1 - \left(\Phi_i^U \right)^q\right)^{w_i}\right)^\frac{s}{q} \end{aligned}\end{bmatrix}, \\ &\begin{bmatrix} \left(\left(1 -\left(1 - \left(\Psi_i^L \right)^q\right)^{w_i}\right)\left(1 -\left(1 - \left(\Psi_i^L \right)^q\right)^{w_i}\right)\right)^\frac{1}{q}, \\ \left(\left(1 -\left(1 - \left(\Psi_i^U \right)^q\right)^{w_i}\right) \left(1 -\left(1 - \left(\Psi_i^U \right)^q\right)^{w_i}\right)\right)^\frac{1}{q}\end{bmatrix}, \\&\begin{bmatrix} \left(\left(1 -\left(1 - \left(\Upsilon_i^L \right)^q\right)^{w_i}\right)\left(1 -\left(1 - \left(\Upsilon_i^L \right)^q\right)^{w_i}\right)\right)^\frac{1}{q}, \\ \left(\left(1 -\left(1 - \left(\Upsilon_i^U \right)^q\right)^{w_i}\right) \left(1 -\left(1 - \left(\Upsilon_i^U \right)^q\right)^{w_i}\right)\right)^\frac{1}{q}\end{bmatrix} \end{aligned}\end{pmatrix}\end{aligned}$ Similarly, because $i = 1,2, \cdots ,n$, we need to perform n product operations between $\left( {A_{i} w_{i} } \right)^{h}$ and $\left( {A_{j} w_{j} } \right)^{s}$ and add them. Then, we obtain

$$\begin{aligned} & \sum\limits_{i = 1}^{n} {\left( {A_{i} w_{i} } \right)^{h} \otimes \left( {A_{j} w_{j} } \right)^{s} } \hfill \\ &= \left( \begin{aligned} & \left[ \begin{aligned} \left( {1 - \prod\limits_{i = 1}^{n} {\left( {1 - \left( {1 - \left( {1 - \left( {\Phi_{i}^{L} } \right)^{q} } \right)^{{w_{i} }} } \right)^{h} \left( {1 - \left( {1 - \left( {\Phi_{j}^{L} } \right)^{q} } \right)^{{w_{j} }} } \right)^{s} } \right)} } \right)^{\frac{1}{q}} , \hfill \\ \left( {1 - \prod\limits_{i = 1}^{n} {\left( {1 - \left( {1 - \left( {1 - \left( {\Phi_{i}^{U} } \right)^{q} } \right)^{{w_{i} }} } \right)^{h} \left( {1 - \left( {1 - \left( {\Phi_{j}^{U} } \right)^{q} } \right)^{{w_{j} }} } \right)^{s} } \right)} } \right)^{\frac{1}{q}} \hfill \\ \end{aligned} \right], \hfill \\ & \left[ \begin{aligned} \prod\limits_{i = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{L} } \right)^{{w_{i} q}} } \right)^{h} } \right)}^{\frac{1}{q}} \left( {\left( {1 - \left( {\Psi_{j}^{L} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} , \hfill \\ \prod\limits_{i = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{U} } \right)^{{w_{i} q}} } \right)^{h} } \right)}^{\frac{1}{q}} \left( {\left( {1 - \left( {\Psi_{j}^{U} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} \hfill \\ \end{aligned} \right], \hfill \\ & \left[ \begin{aligned} \prod\limits_{i = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{L} } \right)^{{w_{i} q}} } \right)^{h} } \right)}^{\frac{1}{q}} \left( {\left( {1 - \left( {\Upsilon_{j}^{L} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} , \hfill \\ \prod\limits_{i = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{U} } \right)^{{w_{i} q}} } \right)^{h} } \right)}^{\frac{1}{q}} \left( {\left( {1 - \left( {\Upsilon_{j}^{U} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} \hfill \\ \end{aligned} \right] \hfill \\ \end{aligned} \right) \hfill \\ \end{aligned}$$

Furthermore, because $j = 1,2, \cdots ,n{\kern 1pt} {\kern 1pt} (i \ne j)$, we also need to compute n product operations between $\left( {A_{i} w_{i} } \right)^{h}$ and $\left( {A_{j} w_{j} } \right)^{s}$ and add them once more as follows:

$$\begin{aligned} & \sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A_{i} w_{i} } \right)^{h} \otimes \left( {A_{j} w_{j} } \right)^{s} } } \hfill \\ &= \left( \begin{aligned} &\left[ \begin{aligned} \left( {1 - \prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {1 - \left( {\Phi_{i}^{L} } \right)^{q} } \right)^{{w_{i} }} } \right)^{h} \left( {1 - \left( {1 - \left( {\Phi_{j}^{L} } \right)^{q} } \right)^{{w_{j} }} } \right)^{s} } \right)} } } \right)^{\frac{1}{q}} , \hfill \\ \left( {1 - \prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {1 - \left( {\Phi_{i}^{U} } \right)^{q} } \right)^{{w_{i} }} } \right)^{h} \left( {1 - \left( {1 - \left( {\Phi_{j}^{U} } \right)^{q} } \right)^{{w_{j} }} } \right)^{s} } \right)} } } \right)^{\frac{1}{q}} \hfill \\ \end{aligned} \right], \hfill \\ &\left[ \begin{aligned} \prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{L} } \right)^{{w_{i} q}} } \right)^{h} } \right)} }^{\frac{1}{q}} \left( {1 - \left( {1 - \left( {\Psi_{j}^{L} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} , \hfill \\ \prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{U} } \right)^{{w_{i} q}} } \right)^{h} } \right)} }^{\frac{1}{q}} \left( {1 - \left( {1 - \left( {\Psi_{j}^{U} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} \hfill \\ \end{aligned} \right], \hfill \\ &\left[ \begin{aligned} \prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{L} } \right)^{{w_{i} q}} } \right)^{h} } \right)} }^{\frac{1}{q}} \left( {1 - \left( {1 - \left( {\Upsilon_{j}^{L} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} , \hfill \\ \prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{U} } \right)^{{w_{i} q}} } \right)^{h} } \right)} }^{\frac{1}{q}} \left( {1 - \left( {1 - \left( {\Upsilon_{j}^{U} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} \hfill \\ \end{aligned} \right] \hfill \\ \end{aligned} \right) \hfill \\ \end{aligned}$$

Next, we calculate the scalar multiplication between $\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A_{i} w_{i} } \right)^{h} \otimes \left( {A_{j} w_{j} } \right)^{s} } }$ and the real number $\frac{2}{n(n + 1)}$ as follows:

$$\begin{aligned} & \frac{2}{n(n + 1)}\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A_{i} w_{i} } \right)^{h} \otimes \left( {A_{j} w_{j} } \right)^{s} } } \hfill \\ &= \left( \begin{aligned} & \left[ \begin{aligned} \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {1 - \left( {\Phi_{i}^{L} } \right)^{q} } \right)^{{w_{i} }} } \right)^{h} \left( {1 - \left( {1 - \left( {\Phi_{j}^{L} } \right)^{q} } \right)^{{w_{j} }} } \right)^{s} } \right)} } } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{\frac{1}{q}} , \hfill \\ \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {1 - \left( {\Phi_{i}^{U} } \right)^{q} } \right)^{{w_{i} }} } \right)^{h} \left( {1 - \left( {1 - \left( {\Phi_{j}^{U} } \right)^{q} } \right)^{{w_{j} }} } \right)^{s} } \right)} } } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{\frac{1}{q}} \hfill \\ \end{aligned} \right], \hfill \\ &\left[ \begin{aligned} \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{L} } \right)^{{w_{i} q}} } \right)^{h} } \right)^{\frac{1}{q}} } \left( {1 - \left( {1 - \left( {\Psi_{j}^{L} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} } } \right)^{{\frac{2}{n(n + 1)}}} , \hfill \\ \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{U} } \right)^{{w_{i} q}} } \right)^{h} } \right)^{\frac{1}{q}} } \left( {1 - \left( {1 - \left( {\Psi_{j}^{U} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} } } \right)^{{\frac{2}{n(n + 1)}}} \hfill \\ \end{aligned} \right], \hfill \\ &\left[ \begin{aligned} \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{L} } \right)^{{w_{i} q}} } \right)^{h} } \right)^{\frac{1}{q}} } \left( {1 - \left( {1 - \left( {\Upsilon_{j}^{L} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} } } \right)^{{\frac{2}{n(n + 1)}}} , \hfill \\ \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{U} } \right)^{{w_{i} q}} } \right)^{h} } \right)^{\frac{1}{q}} } \left( {1 - \left( {1 - \left( {\Upsilon_{j}^{U} } \right)^{{w_{j} q}} } \right)^{s} } \right)^{\frac{1}{q}} } } \right)^{{\frac{2}{n(n + 1)}}} \hfill \\ \end{aligned} \right] \hfill \\ \end{aligned} \right) \hfill \\ \end{aligned}$$

Finally, we compute the $\frac{1}{h + s}$ power operation of $\frac{2}{n(n + 1)}\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A_{i} w_{i} } \right)^{h} \otimes \left( {A_{j} w_{j} } \right)^{s} } }$ as follows:

$$\begin{aligned} & \left( {\frac{2}{n(n + 1)}\sum\limits_{i = 1}^{n} {\sum\limits_{j = 1}^{n} {\left( {A_{i} w_{i} } \right)^{h} \otimes \left( {A_{j} w_{j} } \right)^{s} } } } \right)^{{\frac{1}{h + s}}} \hfill \\ &= \left( \begin{aligned} &\left[ \begin{aligned} \left( {\left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{i = 1}^{n} {\left( {1 - \left( {1 - \left( {1 - \left( {\Phi_{i}^{L} } \right)^{q} } \right)^{{w_{i} }} } \right)^{h} \left( {1 - \left( {1 - \left( {\Phi_{j}^{L} } \right)^{q} } \right)^{{w_{j} }} } \right)^{s} } \right)} } } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{\frac{1}{q}} } \right)^{{\frac{1}{h + s}}} , \hfill \\ \left( {\left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{i = 1}^{n} {\left( {1 - \left( {1 - \left( {1 - \left( {\Phi_{i}^{U} } \right)^{q} } \right)^{{w_{i} }} } \right)^{h} \left( {1 - \left( {1 - \left( {\Phi_{j}^{U} } \right)^{q} } \right)^{{w_{j} }} } \right)^{s} } \right)} } } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{\frac{1}{q}} } \right)^{{\frac{1}{h + s}}} \hfill \\ \end{aligned} \right], \hfill \\ &\left[ \begin{aligned} \left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{L} } \right)^{{w_{i} q}} } \right)^{h} } \right)} \left( {1 - \left( {1 - \left( {\Psi_{j}^{L} } \right)^{{w_{j} q}} } \right)^{s} } \right)} } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} , \hfill \\ \left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Psi_{i}^{U} } \right)^{{w_{i} q}} } \right)^{h} } \right)} \left( {1 - \left( {1 - \left( {\Psi_{j}^{U} } \right)^{{w_{j} q}} } \right)^{s} } \right)} } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} \hfill \\ \end{aligned} \right], \hfill \\ &\left[ \begin{aligned} \left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{L} } \right)^{{w_{i} q}} } \right)^{h} } \right)} \left( {1 - \left( {1 - \left( {\Upsilon_{j}^{L} } \right)^{{w_{j} q}} } \right)^{s} } \right)} } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} , \hfill \\ \left( {1 - \left( {1 - \left( {\prod\limits_{i = 1}^{n} {\prod\limits_{j = 1}^{n} {\left( {1 - \left( {1 - \left( {\Upsilon_{i}^{U} } \right)^{{w_{i} q}} } \right)^{h} } \right)} \left( {1 - \left( {1 - \left( {\Upsilon_{j}^{U} } \right)^{{w_{j} q}} } \right)^{s} } \right)} } \right)^{{\frac{2}{n(n + 1)}}} } \right)^{{\frac{1}{h + s}}} } \right)^{{{1 \mathord{\left/ {\vphantom {1 q}} \right. \kern-\nulldelimiterspace} q}}} \hfill \\ \end{aligned} \right] \hfill \\ \end{aligned} \right) \hfill \\ \hfill \\ \end{aligned}$$

Therefore, we stopped the proof of Theorem 5.