Initiation and Propagation of Plastic Yielding in Duplex Stainless Steel

Abstract

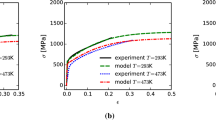
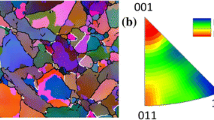
The elastic-plastic behavior of a two-phase stainless steel alloy is explored at the crystal scale for five levels of stress biaxiality. The crystal lattice (elastic) strains were measured with neutron diffraction (ND) using tubular samples subjected to different combinations of axial load and internal pressure to achieve a range of biaxial stress ratios. Finite element simulations were conducted on virtual polycrystals using loading histories that mimicked the experimental protocols. Two-phase microstructures were instantiated based on microscopy images of the grain and phase topologies and on crystallographic orientation distributions from ND. Detailed comparisons were made between the measured and computed lattice strains for several crystal reflections in both phases for scattering vectors in the axial, radial, and hoop directions that confirm the model’s ability to accurately predict the evolving local stress states. The strength-to-stiffness parameter for multiaxial stress states developed for single-phase polycrystals was applied to explain the initiation of yielding across the five levels of stress biaxiality. Finally, building off the multiaxial strength-to-stiffness, the propagation of yielding over the volume of a polycrystal was estimated in terms of an equation that provides the local stress necessary to initiate yielding within crystals in terms of the macroscopic stress.

Appendix A: Reduction of Diffraction Data

The neutron detector registered one-dimensional profiles of diffracted neutron counts. Profiles were comprised of 32 points, corresponding to the 32 detector channels. Each profile was fit using an analytic function. The detector angle, $\phi ,$ was determined from the center of the analytic function. Two peak fit functions, Gaussian and pseudo-Voigt, were considered, both with a sloped background. The pseudo-Voigt profile is a linear combination of Gaussian [$G(\phi )$] and Lorentzian [($L(\phi )$] functions. The Gaussian and Lorentzian distributions are given by

$$ G(\phi )= \frac{2 \sqrt{\ln 2}}{H \sqrt{\pi }} \exp \left[ -4 \ln 2 \frac{(\phi - \mu )^{2}}{H^{2}} \right] , $$

(A1)

$$ L(\phi )= \frac{2}{\pi H} \left[ 1 + \frac{4 (\phi - \mu )^{2}}{H^{2}} \right] ^{-1}, $$

(A2)

where $\mu$ is the mean and H is the full-width at half-maximum. The analytic forms of the Gaussian and pseudo-Voigt peak fitting functions with sloped background are

$$ y^{\text {fit}}= I G(\phi ) + m (\phi - \phi _{1}) + b, $$

(A3)

$$ y^{\text {fit}}= I \left[ \eta L(\phi ) + (1-\eta ) G(\phi ) \right] + m (\phi - \phi _{1}) + b, $$

(A4)

where I is the intensity, $\eta$ is a mixing parameter between 0 and 1, b is the constant background, and m is the background slope. The offset $\phi _{1}$ is the position of the first detector wire and is not a free parameter. The Gaussian function has five degrees of freedom, whereas the pseudo-Voigt has six. Figure A1 shows representative diffraction peaks fit with both functions. The pseudo-Voigt function consistently performs better than the Gaussian function both qualitatively and in terms of the adjusted $R^{2}$ statistic. However, since the peaks are fairly symmetric, both functions perform about equally well with regard to determining the center of the distribution, corresponding to the detector angle. Consequently, both functions perform about equally well in the strain calculation and yield similar results. The pseudo-Voigt function was used for all subsequent data reduction.

Fig. A1

Representative diffraction peaks for the axial scattering vector at zero load for the (a) FCC $\{111\},$ (b) BCC $\{110\},$ (c) FCC $\{200\},$ (d) BCC $\{200\},$ (e) FCC $\{220\},$ and (f) BCC $\{211\}$ crystallographic fibers

There was overlap between the tails of the FCC $\{111\}$ and BCC $\{110\}$ peaks. The overlap region was excluded from the peak fit analysis and each peak was fit separately. Simultaneous fitting of the overlapping peaks was investigated, but did not produce a significant improvement. One of the detector wires (Wire 21) exhibited spurious behavior during some experiments. The 21 data point was therefore excluded from all analyses.

Diffraction peak fitting minimizes the weighted sum-squared error s between the measured data $y^{\text {meas}}$ and fit data $y^{\text {fit}}$

$$ s = \sum _{i=1}^{n} w_{i} \left( y_{i}^{\text {meas}} - y_{i}^{\text {fit}} \right) ^{2}, $$

(A5)

where w represents the weight. The subscript i indexes over the data points corresponding to each of the detector wires. The weighting function is related to the variance of the neutron counts that would arise if the peak were measured multiple times. Since neutron counting is a Poisson process, the variance of the counts is proportional to the number of counts. The weighting function is defined as the reciprocal of one plus the variance

$$ w_{i} = \left( y_{i}^{\text {meas}} + 1 \right) ^{-1}. $$

(A6)

The addition of one to the variance exists to avoid potential division by zero.

The Bragg angle, calculated from the diffraction peak fit, is used to calculate the lattice spacing. The Bragg angle is half the detector angle, $\phi .$ Bragg’s law describes the relationship between the lattice spacing, d,  Bragg angle, $\theta ,$ and wavelength, $\lambda .$

$$ \lambda = 2d \sin \theta . $$

(A7)

The normal component of lattice strain, $\epsilon ,$ aligned with the scattering vector is the change in lattice spacing normalized by the initial lattice spacing

$$ \epsilon = \frac{d - d_{0}}{d_{0}} = \frac{d}{d_{0}} - 1, $$

(A8)

where the subscript $(\cdot )_{0}$ denotes a reference value. Combining Eqs. [A7] and [A8] yields

$$ \epsilon = \frac{\sin \theta _{0}}{\sin \theta } - 1. $$

(A9)

A relative, rather than absolute, reference Bragg angle was used to calculate strain. Since the material is assumed to be initially unstrained, the Bragg angle at zero load may be taken as the reference angle. To improve the precision of the reference Bragg angle, a linear fit was performed of the Bragg angle as a function of applied axial stress for the first six load steps in the elastic regime (0 to 250 MPa). The reference Bragg angle was then determined from the y-intercept of the fit line. This fitting procedure was used to determine a reference Bragg angle for each crystallographic fiber.

Bragg angle uncertainty, $\delta \theta ,$ is half the detector angle uncertainty, $\delta \phi ,$ calculated from the goodness of the diffraction peak fit. The relationship between lattice strain uncertainty, $\delta \epsilon ,$ and Bragg angle uncertainty, $\delta \theta ,$ is obtained by differentiating Eq. [A9] with respect to $\theta$

$$\delta \epsilon = \frac{\sin \theta _{0} \cos \theta }{\sin ^{2}\theta } \delta \theta . $$

(A10)

Bragg angle uncertainty is used with Eq. [A10] to calculate the lattice strain uncertainty. Uncertainty in the reference Bragg angle, $\theta _{0},$ is treated as bias, and is not included in this analysis.