Skip to main content
SpringerLink
Log in

Numerical Simulation of Dual-Phase-Lag Model and Inverse Fractional Single-Phase-Lag Problem for the Non-Fourier Heat Conduction in a Straight Fin

  • Published:
Journal of Thermal Science Aims and scope Submit manuscript

Abstract

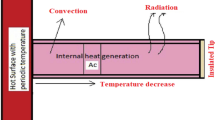
In recent years, many studies have been done on heat transfer in the fin under unsteady boundary conditions using Fourier and non-Fourier models. In this paper, unsteady non-Fourier heat transfer in a straight fin having an internal heat source under periodic temperature at the base was investigated by solving numerically Dual-Phase-Lag and Fractional Single-Phase-Lag models. In this way, the governing equations of these models were presented for heat conduction analysis in the fin, and their results of the temperature distribution were validated using the theoretical results of Single and Dual-Phase-Lag models. After that, for the first time the order of fractional derivation and heat flux relaxation time of the fractional model were obtained for the straight fin problem under periodic temperature at the base using Levenberg-Marquardt parameter estimation method. To solve the inverse fractional heat conduction problem, the numerical results of Dual-Phase-Lag model were used as the inputs. The results obtained from Fractional Single-Phase-Lag model could predict the fin temperature distribution at unsteady boundary condition at the base as well as the Dual-Phase-Lag model could.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Abbreviations

A :

Cross section of arbitrary profile/m2

C T :

Thermal wave speed/m•s−1

c :

Specific heat capacity/J•kg−1•K−1

G :

Generation number

H :

Dimensionless convective heat transfer coefficient

h :

Convective heat transfer coefficient/W•m−2•K−1

i, j, n :

Number of iterations

J :

Sensitivity coefficient

k :

Thermal conductivity/ W•m−1•K−1

L :

Fin length/m

P :

Unknown parameters in inverse problem

p :

Perimeter of fin element/m

q :

Heat flux/W•m−2

q gen :

Internal heat generation/W•m−2

q ∞ :

Internal heat generation at sink temperature/W•m−2

S :

Least squares norm

T :

Fin temperature/°C

T b :

Fin base temperature/°C

T b,m :

Average of fin base temperature/°C

T ∞ :

Ambient temperature/°C

T0 Initial :

temperature of fin/°C

t :

Time/s

X :

Coordinate variable/m

α :

Fin thermal diffusivity/m2•s−1

β :

Order of fractional derivative

Γ :

Dimensionless temperature gradient relaxation time

ε :

Internal heat generation parameter/ K−1

ε :

G Dimensionless internal heat generation parameter

η :

Dimensionless coordinate parameter

θ :

Dimensionless fin temperature

θ ∞ :

Dimensionless ambient temperature

Λ :

Dimensionless heat flux relaxation time

μ :

Damping parameter

ξ :

Dimensionless time

ρ :

Density/kg•m−3

τ q :

Heat flux relaxation time/s

τ T :

Temperature gradient relaxation time/s

φ :

Amplitude of periodic base temperature

ψ :

Dimensionless base temperature frequency

ω :

Base temperature frequency/s−1

ϵ :

Error tolerance

ω :

Weight factor of fractional derivative

References

  1. Fourier J.B., Théorie Analytique de la Chaleur, Paris. 1822 (English Translation by Freeman A., in English: The analytical theory of heat). Dover, New York, 1955.

    Google Scholar 

  2. Cattaneo C., Sur une Forme de l’Équation de la Chaleur Elinant le Paradoxe d’une Propagation Instantance (in English: On a form of the heat equation by eliminating the paradox of an instant propagation). CR Academy of Science Paris, 1958, 247: 431–433.

    MATH  Google Scholar 

  3. Vernotte M.P., Les Paradoxes de la Théorie Continue de l’Équation de la Chaleur (in English: The paradoxes of the continuous theory of heat conduction equation). CR Academy of Science Paris, 1958, 246: 3154–3155.

    MathSciNet  MATH  Google Scholar 

  4. Bai C., Lavine A.S., On the hyperbolic heat conduction and the second law of thermodynamics. ASME Journal of Heat Transfer, 1995, 117(2): 256–263.

    Article  Google Scholar 

  5. Bayazitoglu Y., Peterson G.P., Fundamental issues in hyperbolic heat conduction. Heat Transfer Division (HTD) Series, American Society of Mechanical Engineers, CA., 1992, 227.

    Google Scholar 

  6. Tzou D.Y., A unified field approach for heat conduction from micro-to macro-scales. ASME Journal of Heat Transfer, 1995, 117(1): 8–16.

    Article  Google Scholar 

  7. Tzou D.Y., Macro-to micro-scale heat transfer: the lagging behavior. second ed., Taylor and Francis, Washington, D.C., 1997, pp. 61–122.

    Google Scholar 

  8. Antaki P.J., New interpretation of non-Fourier heat conduction in processed meat. ASME Journal of Heat Transfer, 2005, 127: 189–193.

    Article  Google Scholar 

  9. Zhou J., Zhang Y., Chen J.K., An axisymmetric dualphase-lag bio heat model for laser heating of living tissues. International Journal of Thermal Science, 2009, 48: 1477–1485.

    Article  Google Scholar 

  10. Vadasz P., Heat conduction in nano-fluid suspensions. ASME Journal of Heat Transfer, 2006, 128(6): 465–477.

    Article  Google Scholar 

  11. Al-Nimr M.A., Khadrawi A.F., Thermal behavior of a stagnant gas confined in a horizontal micro channel as described by the dual-phase-lag heat conduction model. International Journal of Thermophysics, 2004, 25(6): 1953–1964.

    Article  ADS  Google Scholar 

  12. Khadrawi A.F., Al-Nimr M.A., Unsteady natural convection fluid flow in a vertical micro channel under the effect of the dual-phase-lag heat conduction model. International Journal of Thermophysics, 2007, 28(4): 1387–1400.

    Article  ADS  Google Scholar 

  13. Kendall T., Haji-Sheikh A., Nnanna A.G.A., Phasechange phenomena in porous media-a non-local thermal equilibrium model. International Journal of Heat Mass Transfer, 2001, 44: 1619–1625.

    Article  MATH  Google Scholar 

  14. Minkowycz W.J., Haji-Sheikh A., Vafai K., On departure from local thermal equilibrium in porous media due to a rapidly changing heat source: the Sparrow number. International Journal of Heat Mass Transfer, 1999, 42: 3373–3385.

    Article  MATH  Google Scholar 

  15. Fangming J., Dengying L., DPL model analysis of non-Fourier heat conduction restricted by continuous boundary interface. Journal of Thermal Science, 2001, 10(1): 69–73.

    Article  Google Scholar 

  16. Yang Y.W., Periodic heat transfer in straight fins. ASME Journal of Heat Transfer, 1972, 94: 310–314.

    Article  Google Scholar 

  17. Eslinger R.G., Chung B.T.F., Periodic heat transfer in radiating and convecting fins or fin arrays. AIAA Journal, 1979, 17: 1134–1140.

    Article  ADS  Google Scholar 

  18. Aziz A., Na T.Y., Periodic heat transfer in fins with variable thermal parameters. International Journal of Heat and Mass Transfer, 1981, 24: 1397–1404.

    Article  MATH  ADS  Google Scholar 

  19. Yang X.X., He H.Z., Periodic heat transfer in the fins with variable thermal parameter. Applied Mathematics and Mechanics, 1996, 17(2): 169–180.

    Article  MATH  Google Scholar 

  20. Al-Sanea S.A., Mujahid A.A., A numerical study of the thermal performance of fins with time-independent boundary conditions including initial transient effects. Warme Stoffubertrag, 1993, 28: 417–424.

    Article  ADS  Google Scholar 

  21. Lin J.Y., The non-Fourier effect on the fin performance under periodic thermal conditions. Journal of Applied Mathematical Modelling, 1998, 22(8): 629–640.

    Article  MATH  Google Scholar 

  22. Lee H.L., Chang W.J., Chen W.L., Yang Y.C., Non-Fourier thermoelastic analysis of an annular fin with variable convection heat transfer coefficient. International Journal of Thermophysics, 2012, 33: 1068–1081.

    Article  ADS  Google Scholar 

  23. Kundu B., Lee K.S., Fourier and non-Fourier heat conduction analysis in the absorber plates of a flat-plate solar collector. Journal of Solar Energy, 2012, 86: 3030–3039.

    Article  Google Scholar 

  24. Ahmadikia H., Rismanian C., Analytical solution of non-Fourier heat conduction problem on a fin under periodic boundary conditions. Journal of Mechanical Science and Technology, 2011, 25(11): 2919–2926.

    Article  Google Scholar 

  25. Askarizadeh H., Ahmadikia H., Periodic heat transfer in convective fins based on dual-phase-lag theory. Journal of Thermophysics and Heat Transfer, 2016, 30(2): 359–368.

    Article  Google Scholar 

  26. Singh S., Kumar D., Rai K.N., Analytical solution of Fourier and non-Fourier heat transfer in longitudinal fin with internal heat generation and periodic boundary condition. International Journal of Thermal Sciences, 2018, 125: 166–175.

    Article  Google Scholar 

  27. Hilfer R., Applications of fractional calculus in physics. World Scientific, Singapore, 2000.

    Book  MATH  Google Scholar 

  28. Compte A., Metzler R., The generalized Cattaneo equation for the description of anomalous transport processes. Journal of Physics A: Mathematical and General, 1997, 30: 7277–7289.

    Article  MathSciNet  MATH  ADS  Google Scholar 

  29. Ghazizadeh H.R., Maerefat M., Azimi A., Modeling non-Fourier behavior of bio heat transfer by fractional single-phase-lag heat conduction constitutive model. The 4th IFAC Workshop on Fractional Differentiation and its Applications (FDA’10), University of Extremadura in Badajoz, Spain, 2010, ISBN: 9788055304878, Article no.: FDA10-053.

    MATH  Google Scholar 

  30. Odibat Z.M., Shawagfeh N.T., Generalized Taylor’s formula. Journal of Applied Mathematics and Computation, 2007, 186: 286–293.

    Article  MathSciNet  MATH  Google Scholar 

  31. Ghazizadeh H.R., Azimi A., Maerefat M., An inverse problem to estimate relaxation parameter and order of fractionally in fractional single-phase-lag heat equation. International Journal of Heat and Mass Transfer, 2012, 55: 2095–2101.

    Article  Google Scholar 

  32. Zhuang Q., Yu B., Jiang, X., An inverse problem of parameter estimation for time-fractional heat conduction in a composite medium using carbon–carbon experimental data. Journal of Physica B: Condensed Matter, 2015, 456: 9–15.

    Article  ADS  Google Scholar 

  33. Yu B., Jiang X., Wang W., Numerical algorithms to estimate relaxation parameters and Caputo fractional derivative for a fractional thermal wave model in spherical composite medium. Journal of Applied Mathematics and Computation, 2016, 274: 106–118.

    Article  MathSciNet  MATH  Google Scholar 

  34. Tang D.W., Araki N., An inverse analysis to estimate relaxation parameters and thermal diffusivity with a universal heat conduction equation. International Journal of Thermophysics, 2000, 22: 553–561.

    Article  Google Scholar 

  35. Huang C.H., Hsien-Wu H., An inverse hyperbolic heat conduction problem in estimating surface heat flux by the conjugate gradient method. Journal of Physics D: Applied Physics, 2006, 39: 4087–4096.

    Article  Google Scholar 

  36. Huang C.H., Lin C., Inverse hyperbolic conduction problem in estimating two unknown surface heat fluxes simultaneously. Journal of Thermophysics and Heat Transfer, 2008, 22: 766–774.

    Article  Google Scholar 

  37. Haghighi G., Malekzadeh M.R., Rahideh P., Vaghefi M., Inverse transient heat conduction problems of a multilayered functionally graded cylinder. Journal of Numerical Heat Transfer, Part A: Applications, 2012, 61: 717–733.

    Article  ADS  Google Scholar 

  38. Tser-Son W., Lee H.L., Chang W.J., Yang Y.C., An inverse hyperbolic heat conduction problem in estimating pulse heat flux with a dual-phase-lag model. International Communications in Heat and Mass Transfer, 2015, 60: 1–8.

    Article  Google Scholar 

  39. Wang C.C., Direct and inverse solutions with non-Fourier effect on the irregular shape. International Journal of Heat and Mass Transfer, 2010, 53: 2685–2693.

    Article  MATH  Google Scholar 

  40. Hsu P.T., Chu Y.H., An inverse non-Fourier heat conduction problem approach for estimating the boundary condition in electronic device. Journal of Applied Mathematical Modeling, 2004, 28: 639–652.

    Article  MATH  Google Scholar 

  41. Azimi A., Bamdad K., Ahmadikia H., Inverse hyperbolic heat conduction in fins with arbitrary profiles. Journal of Numerical Heat Transfer, Part A: Applications, 2012, 61(3): 220–240.

    Article  MATH  ADS  Google Scholar 

  42. Mochnacki B., Paruch M., Estimation of relaxation and thermalization times in microscale heat transfer model. Journal of Theoretical and Applied Mechanics, 2013, 51(4): 837–845.

    MATH  Google Scholar 

  43. Podlubny I., Fractional differential equations. Academic Press, New York, 1999.

    MATH  Google Scholar 

  44. Ozisik M.N., Orlande H.R.B., Inverse heat transfer problems: Fundamentals and applications. Taylor & Francis, New York, 2000.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Shahid Chamran University of Ahvaz, Ahvaz, 6135785311, Iran

    Milad Mozafarifard, Aziz Azimi & Salem Mehrzad

Authors
  1. Milad Mozafarifard
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Aziz Azimi
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Salem Mehrzad
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Aziz Azimi.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Mozafarifard, M., Azimi, A. & Mehrzad, S. Numerical Simulation of Dual-Phase-Lag Model and Inverse Fractional Single-Phase-Lag Problem for the Non-Fourier Heat Conduction in a Straight Fin. J. Therm. Sci. 29, 632–646 (2020). https://doi.org/10.1007/s11630-019-1137-1

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11630-019-1137-1

Keywords

Search

Navigation