Skip to main content
SpringerLink
Log in

New Algorithmic Implementation of Exact Three-Point Difference Schemes for Systems of Nonlinear Ordinary Differential Equations of the Second Order

  • Published:
Ukrainian Mathematical Journal Aims and scope

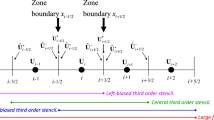
We propose and justify three-point difference schemes of higher orders of accuracy on a nonuniform grid for systems of nonlinear ordinary differential equations of the second order with derivative on the right-hand side and boundary conditions of the first kind. We construct a new approximation of the derivative of solution to the boundary value problem at the nodes of the grid, prove the existence and uniqueness of the solution, and establish the order of accuracy of the difference schemes. We also develop an iterative Newton-type method aimed at finding the solutions of these schemes and propose an algorithm for the automatic selection of grid points guaranteeing the attainment of the required accuracy. In addition, we present numerical examples, which confirm the efficiency and reliability of the proposed algorithm.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. V. L. Makarov and A. A. Samarskii, “Exact three-point difference schemes for second-order nonlinear ordinary differential equations and their implementation,” Sov. Math. Dokl., 41, No. 3, 495–500 (1991).

    Google Scholar 

  2. M. V. Kutniv, V. L. Makarov, and A. A. Samarskii, “Accurate three-point difference schemes for second-order nonlinear ordinary differential equations and their implementation,” Comput. Math. Math. Phys., 39, No. 1, 45–60 (1999).

    MathSciNet  MATH  Google Scholar 

  3. M. V. Kutniv, “Accurate three-point difference schemes for second-order monotone ordinary differential equations and their implementation,” Comput. Math. Math. Phys., 40, No. 3, 368–382 (2000).

    MathSciNet  MATH  Google Scholar 

  4. M. V. Kutniv, “Modified three-point difference schemes of high-accuracy order for second order nonlinear ordinary differential equations,” Comput. Methods Appl. Math., 3, No. 2, 287–312 (2003).

    Article  MathSciNet  Google Scholar 

  5. L. B. Gnativ, M. V. Kutniv, and A. I. Chukhrai, “Generalized three-point difference schemes of high order of accuracy for nonlinear ordinary differential equations of the second order,” Mat. Met. Fiz.-Mekh. Polya, 51, No. 4, 59–69 (2008); English translation: J. Math. Sci., 167, No. 1, 62–75 (2010).

  6. M. Krol and M. V. Kutniv, “Three-point difference schemes of high-order accuracy for second-order nonlinear differential equations with boundary conditions of the third kind,” J. Comput. Appl. Math., No. 2(116), 43–62 (2014).

    Google Scholar 

  7. I. P. Gavrilyuk, M. Hermann, V. L. Makarov, and M. V. Kutniv, Exact and Truncated Difference Schemes for Boundary Value ODEs, Springer AG, Basel (2011).

    Book  Google Scholar 

  8. M. V. Kutniv, “Three-point difference schemes of high accuracy order for systems of nonlinear ordinary differential equations of the second order,” Comput. Math. Math. Phys., 41, No. 6, 860–873 (2001).

    MathSciNet  MATH  Google Scholar 

  9. M. V. Kutniv, “High-order accurate three-point difference schemes for systems of second-order ordinary differential equations with a monotone operator,” Comput. Math. Math. Phys., 42, No. 5, 724–738 (2002).

    MathSciNet  MATH  Google Scholar 

  10. L. B. Gnativ, M. V. Kutniv, and V. L. Makarov, “Generalized three-point difference schemes of high-order accuracy for systems of second order nonlinear ordinary differential equations,” Different. Equat., 45, No. 7, 998–1019 (2009).

    Article  MathSciNet  Google Scholar 

  11. E. Hairer, S. P. Nørsett, and G. Wanner, Solving Ordinary Differential Equations I. Nonstiff Problems, Springer, Berlin (1987).

    Book  Google Scholar 

  12. L. B. Gnativ, M. Krol, and M. V. Kutniv, “Exact three-point difference schemes for second order nonlinear differential equations with boundary conditions of the third kind,” J. Numer. Appl. Math., No. 3(109), 34–52 (2012).

    Google Scholar 

  13. A. A. Samarskii and E. S. Nikolaev, Numerical Methods for Grid Equations, vol. 2, Iterative Methods, Birkhäuser, Basel (1989).

  14. V. A. Trenogin, Functional Analysis [in Russian], Nauka, Moscow (1980).

  15. J. R. Cash and F. Mazzia, “Hybrid mesh selection algorithms based on conditioning for two-point boundary value problems,” J. Numer. Anal., Industrial Appl. Math., 1, No. 6, 81–90 (2006).

    MathSciNet  MATH  Google Scholar 

  16. Ch. Tsitouras and S. N. Papakostas, “Cheap error estimation for Runge–Kutta methods,” SIAM J. Sci. Comput., 20, No. 6, 2067–2088 (1999).

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute for Applied Problems in Mechanics and Mathematics, Ukrainian National Academy of Sciences, Lviv, Ukraine

    M. V. Kutniv

  2. Rzeszów University of Technology, Rzeszów, Poland

    M. V. Kutniv & M. Krol

Authors
  1. M. V. Kutniv
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. M. Krol
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to M. Krol.

Additional information

Translated from Ukrains’kyi Matematychnyi Zhurnal, Vol. 74, No. 2, pp. 204–219, February, 2022. Ukrainian DOI: https://doi.org/10.37863/umzh.v74i2.6935.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kutniv, M.V., Krol, M. New Algorithmic Implementation of Exact Three-Point Difference Schemes for Systems of Nonlinear Ordinary Differential Equations of the Second Order. Ukr Math J 74, 232–250 (2022). https://doi.org/10.1007/s11253-022-02060-y

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11253-022-02060-y

Search

Navigation