Estimation of entanglement in bipartite systems directly from tomograms

Abstract

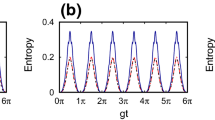
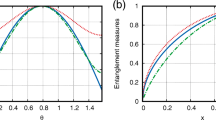
We investigate the advantages of extracting the degree of entanglement in bipartite systems directly from tomograms, as it is the latter that are readily obtained from experiments. This would provide a superior alternative to the standard procedure of assessing the extent of entanglement between subsystems after employing the machinery of state reconstruction from the tomogram. The latter is both cumbersome and involves statistical methods, while a direct inference about entanglement from the tomogram circumvents these limitations. In an earlier paper, we had identified a procedure to obtain a bipartite entanglement indicator directly from tomograms. To assess the efficacy of this indicator, we now carry out a detailed investigation using two nonlinear bipartite models by comparing this tomographic indicator with standard markers of entanglement such as the subsystem linear entropy and the subsystem von Neumann entropy and also with a commonly used indicator obtained from inverse participation ratios. The two-model systems selected for this purpose are a multilevel atom interacting with a radiation field, and a double-well Bose–Einstein condensate. The role played by the specific initial states of these two systems in the performance of the tomographic indicator is also examined. Further, the efficiency of the tomographic entanglement indicator during the dynamical evolution of the system is assessed from a time-series analysis of the difference between this indicator and the subsystem von Neumann entropy.

Appendices

Appendix 1: Reconstructing a single-mode vacuum state from the tomogram

For our present purpose, we start with the tomogram for a zero-photon state obtained numerically from the corresponding density matrix. The aim is to demonstrate how to reconstruct the state from this tomogram. Since we have the exact density matrix (equivalently, state) to compare it with, we can assess the efficiency of the reconstruction program. The procedure we employ is based on iterative maximum likelihood estimates. Here, starting with a trial density matrix, an appropriate transformation outlined in [40] is applied iteratively on the density matrix to achieve a reconstructed state with the maximum possible likelihood of being the true state (here, the vacuum state). It is evident that the choice of the trial state is crucial for the efficiency of this procedure. Figure 14a–c shows the different reconstructed states arising from three different trial density matrices. Figure 14d is the photon number distribution of the true state. It is evident that Fig. 14c is closer to the true state compared to Fig. 14a, b. However, the probability of the zero-photon state is only $\sim 0.85$ compared to 1 in Fig. 14d.

Fig. 14

Photon number distribution of the reconstructed state corresponding to trial density matrix a $0.1\sum _{p=0}^{9} |p\rangle \langle p|$, b $0.2\sum _{p=0}^{4} |p\rangle \langle p|$ and c $0.9 |0\rangle \langle 0| + 0.1 |1\rangle \langle 1|$. d Photon number distribution of the true state $|0\rangle \langle 0|$

More sophisticated techniques will lead to a reconstructed state closer to the zero-photon state. However, these are computationally intensive even in this case and significantly more time-consuming for bipartite and multipartite states. It is therefore useful and efficient to extract as much information about a state directly from tomograms as possible.

Appendix 2: Time evolution in the double-well BEC model

The double-well BEC effective Hamiltonian is given by Eq. (24). We require the state $|\psi (t)\rangle$ corresponding to an initial state that is a direct product of normalized boson-added coherent states of the atoms in the wells A and B, namely,

$$\begin{aligned} |\psi _{m_{1},m_{2}}(0)\rangle = |\alpha _{a}, m_{1}\rangle \otimes |\alpha _{b}, m_{2}\rangle , \end{aligned}$$

(30)

where $\alpha _{a}, \alpha _{b} \in \mathbb {C}$ and $m_{1}, m_{2}$ are nonnegative integers. The dependence of the state on $\alpha _{a}$ and $\alpha _{b}$ has been suppressed on the left-hand side for notational simplicity. The m-PACS $|\alpha , m\rangle$ [defined in Eq. (17)] reduces to the standard oscillator coherent state $|\alpha \rangle$ for $m = 0$.

In the special case $m_{1} = 0, m_{2} = 0$, the state at any time t corresponding to the initial state $\psi _{0,0}(0)$ has been shown in Ref. [22] to be given by

$$\begin{aligned} |\psi _{00} (t)\rangle&= \mathrm{{e}}^{-\tfrac{1}{2}(|\alpha _{a}|^{2} + |\alpha _{b}|^{2})} \sum _{p,q=0}^{\infty } \frac{\beta _{1}^{p}(t) \, \beta _{2}^{q}(t)}{\sqrt{p! q!}} \nonumber \\&\quad \mathrm{{e}}^{- i t (p+q)[\omega _{0} + U (p + q)]} |p\rangle \otimes |q\rangle , \end{aligned}$$

(31)

where

$$\begin{aligned} \left. \begin{array}{lll} \beta _{1}(t) &{} = &{} \alpha _{a} \cos \,(\lambda _{1} t) + (i/\lambda _{1}) ( \lambda \alpha _{b} - \omega _{1} \alpha _{a}) \sin \,(\lambda _{1} t),\\ \beta _{2}(t) &{} = &{} \alpha _{b} \cos \,(\lambda _{1} t) + (i/\lambda _{1}) ( \lambda \alpha _{a} + \omega _{1} \alpha _{b}) \sin \,(\lambda _{1} t), \end{array} \right\} \end{aligned}$$

(32)

and $\lambda _{1}=(\lambda ^{2}+ \omega _{1}^{2})^{1/2}$. It can then be shown [9] that the state vector at time t is given by

$$\begin{aligned} |\psi _{m_{1},m_{2}}(t)\rangle = M_{m_{1},m_{2}}(t)|\psi _{00}(t)\rangle , \end{aligned}$$

(33)

where the operator $M_{m_{1},m_{2}}(t)$ is as follows. Let k, l, p, q denote nonnegative integers, and let

$$\begin{aligned} s = k+l+p+q, \, \overline{p} =(k+m_{2}-l), \,\overline{q} =(l+m_{1}-k). \end{aligned}$$

(34)

Further, let

$$\begin{aligned} \kappa = \big [m_{1}! m_{2}! L_{m_{1}}(-|\alpha _{a}|^{2}) L_{m_{2}}(-|\alpha _{b}|^{2})\big ]^{-1/2} \end{aligned}$$

(35)

and $\Gamma =\cos ^{-1}(\omega _{1}/\lambda _{1})$. Then

$$\begin{aligned} M_{m_{1},m_{2}}(t)= & {} \kappa \left\{ \sum _{k=0}^{m_{1}}\sum _{l=0}^{m_{2}}\sum _{p=0}^{\overline{p}}\sum _{q=0}^{\overline{q}}(-1)^{k-p} {\genfrac(){0.0pt}1{m_{1}}{k}} {\genfrac(){0.0pt}1{m_{2}}{l}} \, {\genfrac(){0.0pt}1{\overline{p}}{p}} \, {\genfrac(){0.0pt}1{\overline{q}}{q}}\, \mathrm{{e}}^{2i(l-k)\lambda _{1} t}\right. \nonumber \\&\,\left. \times \big (\cos \,\tfrac{1}{2}\Gamma \big )^{s} \big (\sin \,\tfrac{1}{2}\Gamma \big )^{2(m_{1}+m_{2})- s} (a^{\dagger })^{p+\overline{q}-q} \,(b^{\dagger })^{q+\overline{p}-p}\right\} \nonumber \\&\,\times \mathrm{{e}}^{-i\omega _{0} t(m_{1}+m_{2}) +i\lambda _{1}t (m_{1}- m_{2})} \mathrm{{e}}^{-i U t (m_{1}+m_{2}) (2 N_\mathrm{{tot}} + m_{1}+m_{2})}. \end{aligned}$$

(36)