Skip to main content
SpringerLink
Log in

Chaotic heteroclinic tangles with the degenerate Melnikov function

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

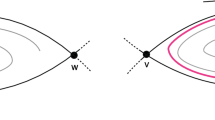
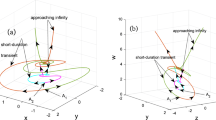
This work establishes the heteroclinic tangles when the Melnikov function is degenerate. By deriving the higher order Melnikov functions, we obtain an explicit formula of the second-order Melnikov function \(E_1(t_0)\), which essentially facilitates the evaluation and application to the concrete differential equations. Two examples manifest this efficient method to characterize chaotic heteroclinic tangle.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Data Availability

Data of this study will be available from the corresponding author on reasonable request.

References

  1. Poincaré, H.: Mémoire sur les courbes définies par une équation différetielle. J. Math. 7, 375–422 (1881)

    MATH  Google Scholar 

  2. Poincaré, H.: Mémoire sur les courbes définies par une équation différetielle. J. Math. 8, 251–296 (1882)

    MATH  Google Scholar 

  3. Smale, S.: Differential dynamical systems. Bull. Am. Math. Soc. 73(6), 747–817 (1967)

    Article  Google Scholar 

  4. Wang, Q.D., Oksasoglu, A.: Rank one chaos: theory and applications. Int. J. Bifurcat. Chaos 18(5), 1261–1319 (2008)

    Article  MathSciNet  Google Scholar 

  5. Wang, Q.D., Oksasoglu, A.: Periodic occurrence of chaotic behavior of homoclinic tangles. Phys. D 239(7), 387–395 (2010)

    Article  MathSciNet  Google Scholar 

  6. Wang, Q.D., Oksasoglu, A.: Dynamics of homoclinic tangles in periodically perturbed second-order equations. J. Differ. Equ. 250(2), 710–751 (2011)

    Article  MathSciNet  Google Scholar 

  7. Chen, F.J., Oksasoglu, A., Wang, Q.D.: Heteroclinic tangles in time-periodic equations. J. Differ. Equ. 254(3), 1137–1171 (2013)

    Article  MathSciNet  Google Scholar 

  8. Young, L.-S.: What are SRB measures, and which dynamical systems have them? J. Stat. Phys. 108, 733–754 (2002)

    Article  MathSciNet  Google Scholar 

  9. Wang, Q.D., Young, L.-S.: Toward a theory of rank one attractors. Ann. Math. 167(2), 349–480 (2008)

    Article  MathSciNet  Google Scholar 

  10. Melnikov, V.K.: On the stability of the center for time periodic perturbations. Trans. Moscow Math. Soc. 12, 1–57 (1963)

    MathSciNet  Google Scholar 

  11. Guckenheimer, J., Holmes, P.: Nonlinear Oscillations, Dynamical Systems and bifurcations of Vector Fields. Springer-Verlag, New York (1983)

    Book  Google Scholar 

  12. Buica, A., Gasull, A., Yang, J.Z.: The third order Melnikov function of a quadratic center under quadratic perturbations. J. Math. Anal. Appl. 331(1), 443–454 (2007)

    Article  MathSciNet  Google Scholar 

  13. Cristina, S.-T., Tasso, J.K.: Higher-order Melnikov theory for adiabatic systems. J. Math. Phys. 37(2), 6220–6249 (1996)

    MathSciNet  MATH  Google Scholar 

  14. Iliev, I.D.: Higher order Melnikov functions for degenerate cubic Hamiltonians. Adv. Differ. Equ. 1(4), 689–708 (1996)

    MathSciNet  MATH  Google Scholar 

  15. Iliev, I.D., Perko, L.M.: Higher order bifurcations of limit cycles. J. Differ. Equ. 154(2), 339–363 (1999)

    Article  MathSciNet  Google Scholar 

  16. Yagasaki, K.: Higher-order Melnikov method and chaos for two-degree-of-freedom Hamiltonian systems with saddle-centers. Discret. Contin. Dyn. Syst. 29(1), 387–402 (2011)

    Article  MathSciNet  Google Scholar 

  17. Zhang, Zh.F., Li, B.Y.: High order melnikov functions and the problem of uniformity in global bifurcation. Ann. Mat. Pura Appl. 161(4), 181–212 (1992)

    MathSciNet  MATH  Google Scholar 

  18. Chen, F.J., Wang, Q.D.: High order Melnikov method for time-periodic equations. Adv. Nonlinear Stud. 17(4), 793–818 (2017)

    Article  MathSciNet  Google Scholar 

  19. Chen, F.J., Wang, Q.D.: High order melnikov method: theory and application. J. Differ. Equ. 267(2), 1095–1128 (2019)

    Article  MathSciNet  Google Scholar 

  20. Wang, Q.D.: Exponentially small splitting: a direct approach. J. Differ. Equ. 269(1), 954–1036 (2020)

    Article  MathSciNet  Google Scholar 

  21. Holmes, P.J.: A nonlinear oscillator with a strange attractor. Philos. Trans. R. Soc. London Ser. A 292(1394), 419–448 (1979)

    Article  MathSciNet  Google Scholar 

  22. Anna, L.H., Vered, R.K.: Symmetry-breaking perturbations and strange attractors. Phys. Rev. E 55(5), 4964–4978 (1997)

    Article  MathSciNet  Google Scholar 

  23. Awrejcewicz, J., Holicke, M.M.: Melnikov’s method and stick-slip chaotic oscillations in very weekly forced mechanical systems. Int. J. Bifurcat. Chaos 9(3), 505–518 (1999)

    Article  Google Scholar 

  24. Awrejcewicz, J., Holicke, M.M.: Analytical prediction of stick-slip chaos in a double self-excited Duffing-type oscillator. Math. Probl. Eng. 2006, 1–79 (2006)

    MathSciNet  MATH  Google Scholar 

  25. Awrejcewicz, J., Pyryev, Y.: Chaos prediction in the Duffing type system with friction using Melnikov’s functions. Nonlinear Anal. Real World Appl. 7(1), 12–24 (2006)

    Article  MathSciNet  Google Scholar 

  26. Awrejcewicz, J., Holicke, M.M.: Smooth and Nonsmooth High Dimensional Chaos and the Melnikov-Type Methods. World Scientific, Singapore (2007)

    Book  Google Scholar 

Download references

Acknowledgements

The authors would like to thank the anonymous reviewers for their careful reading, useful comments and constructive suggestions for the improvement of the manuscript of the present research work.

Funding

The paper is supported by the National Natural Science Foundation of China (Grant No. 11471289).

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Zhejiang University, No.38 Zheda Road, Hangzhou, 310027, Zhejiang, China

    Yi Zhong

  2. Department of Mathematics, Zhejiang Normal University, No. 688 Yingbin Avenue, Jinhua, 321004, Zhejiang, China

    Fengjuan Chen

Authors
  1. Yi Zhong
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Fengjuan Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yi Zhong.

Ethics declarations

Conflict interest

On behalf of all authors, the corresponding author states that there is no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhong, Y., Chen, F. Chaotic heteroclinic tangles with the degenerate Melnikov function. Nonlinear Dyn 108, 697–709 (2022). https://doi.org/10.1007/s11071-022-07220-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-022-07220-0

Keywords

Mathematics Subject Classification

Search

Navigation