Nonlinear resonant response of the cable-stayed beam with one-to-one internal resonance in veering and crossover regions

Abstract

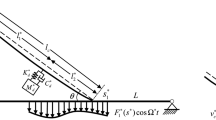
In this study, the large amplitude vibration of the cable-stayed beam subjected to external excitation is investigated. Emphasis is focused on the one-to-one resonant interaction between hybrid and hybrid/local modes, which may be activated in the veering and crossover regions. The Hamilton’s principle and multi-mode discretization are applied to obtain the discrete model governing the in-plane vibration of the cable-stayed beam. Then, the method of multiple scales is applied to solve the equation of motion, and the displacement of the cable-stayed beam and corresponding modulation equations are determined. In the following, the equilibrium solution of the modulation equations and the associated stability are examined to discuss the periodic motion of the cable-stayed beam. Whereas the shooting method is applied to investigate the dynamic solution to qualitatively illustrate the non-periodic motion. Numerical simulations are performed to verify the periodic solution and examine the nonlinear dynamics of the cable-stayed beam. Particular attention is placed on the chaotic dynamic of the cable-stayed beam in unstable region. It is shown that the equilibrium and dynamic solutions may undergo different bifurcations, i.e., Hopf, torus and cyclic-fold bifurcations.

Appendix

The second-order nonlinear interaction coefficients of the modulation equations can be expressed as [14]:

$$\begin{aligned} K_{hh}= & {} \sum ^{\infty }_{k=1}\bigg [(\varLambda _{hhk}+\varLambda _{hkh})\varLambda _{khh}\bigg (\frac{2}{\omega ^2_k}+\frac{1}{\omega ^2_k-4\omega ^2_h}\bigg )\bigg ]\nonumber \\&+\,3\varGamma _{hhhh},~~~~h=m,n, \end{aligned}$$

(59)

$$\begin{aligned} K_{mn}= & {} \sum ^{\infty }_{k=1}\bigg [(\varLambda _{mmk} +\,\varLambda _{mkm})\frac{2\varLambda _{knn}}{\omega _k^2}\nonumber \\&+(\varLambda _{mnk}+\varLambda _{mkn})(\varLambda _{kmn}+\varLambda _{knm})\nonumber \\&\bigg (\frac{1}{\omega ^2_k-(\omega _n+\omega _m)^2}+\frac{1}{\omega ^2_k-(\omega _n-\omega _m)^2}\bigg )\bigg ]\nonumber \\&+\,2(\varGamma _{mnnm}+\varGamma _{mnmn}+\varGamma _{mmnn}),\nonumber \\ K_1= & {} \sum ^{\infty }_{k=1}\bigg [(\varLambda _{nmk}+\varLambda _{nkm})\frac{\varLambda _{knn}}{\omega ^2_k-4\omega ^2_n}\nonumber \\&+\,(\varLambda _{nnk}+\varLambda _{nkn})\frac{(\varLambda _{kmn}+\varLambda _{knm})}{\omega ^2_k}\bigg ]\nonumber \\&+\,\varGamma _{nnnm}+\varGamma _{nnmn}+\varGamma _{nmnn}, \end{aligned}$$

(60)

$$\begin{aligned} K_2= & {} \sum ^{\infty }_{k=1}\bigg [(\varLambda _{nmk}+\varLambda _{nkm})\varLambda _{kmm}\bigg (\frac{2}{\omega ^2_k}+\frac{1}{\omega ^2_k-4\omega ^2_m}\bigg )\bigg ]\nonumber \\&+\,3\varGamma _{nmmm}, \end{aligned}$$

(61)

$$\begin{aligned} K_3= & {} \sum ^{\infty }_{k=1}\bigg [(\varLambda _{nnk}+\varLambda _{nkn})\frac{\varLambda _{kmm}}{\omega ^2_k-4\omega ^2_m}\nonumber \\&+\,(\varLambda _{nmk}+\varLambda _{nkm})\frac{\varLambda _{kmn}+\varLambda _{knm}}{\omega ^2_k}\bigg ]\nonumber \\&+\,\varGamma _{nmmn}+\varGamma _{nmnm}+\varGamma _{nnmm}. \end{aligned}$$

(62)