Skip to main content
SpringerLink
Log in

Oscillatory behavior in discrete slow power-law models

  • Original paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

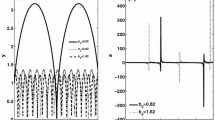
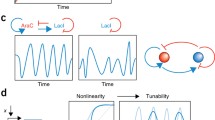
Abstract

Discrete mathematical slow oscillatory models are proposed to describe biological interactions between two populations by considering power-law functions. Conditions for slow convergence to the equilibrium point are imposed on model parameters. Moreover, to obtain oscillatory solutions we prove that model exponents may be parameterized by only one parameter. As a by-product, we also discover a family of functions that can be regarded as a two-dimensional generalization of the Schwarzian derivative. Diverse particular model cases are analyzed numerically in order to show orbital solutions. Finally, applications for biochemical and population models are presented.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

References

  1. Savageau, M.A.: Introduction to S-systems and the underlying power-law formalism. Math. Comput. Model. 11, 546–551 (1988)

    Article  MathSciNet  Google Scholar 

  2. Voit, E.O.: Canonical Nonlinear Modeling: S-systems Approach to Understanding Complexity. Van Nostrand Reinhold, New York (1991)

    MATH  Google Scholar 

  3. Komarova, S.V., Smith, R.J., Dixon, S.J., Sims, S.M., Wahl, L.M.: Mathematical model predicts a critical role for osteoclast autocrine regulation in the control of bone remodeling. Bone 33(2), 206–215 (2003)

    Article  Google Scholar 

  4. Savageau, M.A., Lomnitz, J.G.: Deconstructing Complex Nonlinear Models in System Design Space In Discrete and Topological Models in Molecular Biology. Springer, Berlin (2014)

    MATH  Google Scholar 

  5. Vera, J., Balsa-Canto, E., Wellstead, P., Banga, J.R., Wolkenhauer, O.: Power-law models of signal transduction pathways. Cell Signal. 19(7), 1531–1541 (2007)

    Article  Google Scholar 

  6. Boros, B., Hofbauer, J., Müller, S.: On global stability of the Lotka reactions with generalized mass-action kinetics. Acta Appl. Math. 151(1), 53–80 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  7. Dancsó, A., Farkas, H., Farkas, M., Szabó, G.: Investigations into a class of generalized two-dimensional Lotka–Volterra schemes. Acta Appl. Math. 23(2), 103–127 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  8. Hatton, I.A., McCann, K.S., Fryxell, J.M., Davies, T.J., Smerlak, M., Sinclair, A.R., Loreau, M.: The predator-prey power law: biomass scaling across terrestrial and aquatic biomes. Science 349(6252), aac6284 (2015)

    Article  Google Scholar 

  9. Müller, S., Regensburger, G.: Generalized mass action systems: complex balancing equilibria and sign vectors of the stoichiometric and kinetic-order subspaces. SIAM J. Appl. Math. 72, 1926–1947 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  10. Gupta, A., Banerjee, T., Dutta, P.S.: Increased persistence via asynchrony in oscillating ecological populations with long-range interaction. Phys. Rev. E 96(4), 042202 (2017)

    Article  Google Scholar 

  11. Hopkins, F., Von Brentano, P.: High n-state population and delayed photon emission from beam-foil interaction. J. Phys. B. Atom. Mol. 9(5), 775–778 (1976)

    Article  Google Scholar 

  12. Wiuf, C., Feliu, E.: Power-law kinetics and determinant criteria for the preclusion of multistationarity in networks of interacting species. SIAM J. Appl. Dyn. Syst. 12(4), 1685–1721 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  13. Wang, Y., Gao, S., Yu, Y., Xu, Z.: The discovery of population interaction with a power law distribution in brain storm optimization. Memet. Comput. 11(1), 65–87 (2019)

    Article  Google Scholar 

  14. Bacheler, N.M., Shertzer, K.W., Cheshire, R.T., MacMahan, J.H.: Tropical storms influence the movement behavior of a demersal oceanic fish species. Sci. Rep. 9(1), 1–3 (2019)

    Article  Google Scholar 

  15. Daido, H.: Discrete-time population dynamics of interacting self-oscillators. Prog. Theor. Phys. 75(6), 1460–1463 (1986)

    Article  MathSciNet  Google Scholar 

  16. Kuperman, M.N., Laguna, M.F., Abramson, G., Monjeau, J.A., Lanata, J.L.: Metapopulation oscillations from satiation of predators. Physica A 527(1–8), 121288 (2019)

    Article  MathSciNet  Google Scholar 

  17. Mohd, M.H.: Diversity in interaction strength promotes rich dynamical behaviours in a three-species ecological system. Appl. Math. Comput. 353, 243–253 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  18. Neverova, G.P., Zhdanova, O.L., Ghosh, B., Frisman, E.Y.: Dynamics of a discrete-time stage-structured predator-prey system with Holling type II response function. Nonlinear Dyn. 98(1), 427–446 (2019)

    Article  MATH  Google Scholar 

  19. Jana, D.: Chaotic dynamics of a discrete predator-prey system with prey refuge. Appl. Math. Comput. 224, 848–865 (2013)

    MathSciNet  MATH  Google Scholar 

  20. Lyu., X., Gao, Q., Luo, G., : Dynamic characteristics of a mechanical impact oscillator with a clearance. Int. J. Mech. Sci. 178, 105605 (2020)

  21. Shibata, T.: Global and local structures of oscillatory bifurcation curves. J. Spectr. Theory 9(3), 991–1003 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  22. Sun, K., Zhang, T., Tian, Y.: Theoretical study and control optimization of an integrated pest management predator–prey model with power growth rate. Math. Biosci. 279, 13–26 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  23. Tang, S., Tang, B., Wang, A., Xiao, Y.: Holling II predator–prey impulsive semi-dynamic model with complex Poincaré map. Nonlinear Dyn. 81(3), 1575–1596 (2015)

    Article  MATH  Google Scholar 

  24. Yin, W., Voit, E.O.: Construction and customization of stable oscillation models in biology. J. Biol. Syst. 16(04), 463–478 (2008)

    Article  MATH  Google Scholar 

  25. Hilker, F.M., Liz, E.: Proportional threshold harvesting in discrete-time population models. J. Math. Biol. 79, 1927–1951 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  26. Izzo, G., Vecchio, A.: A discrete time version for models of population dynamics in the presence of an infection. J. Comput. Appl. Math. 210(1–2), 210–221 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  27. Zeigler, B.P.: Persistence and patchiness of predator–prey systems induced by discrete event population exchange mechanisms. J. Theor. Biol. 67(4), 687–713 (1977)

    Article  MathSciNet  Google Scholar 

  28. Crowe, K.M.: A nonlinear ergodic theorem for discrete systems. J. Math. Biol. 32, 179–191 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  29. Lewis, S.J.: A note on the strong ergodic theorem of some discrete models. J. Differ. Equ. Appl. 3(1), 55–63 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  30. Elaydi, S., Sacker, R.: Global stability of periodic orbits of nonautonomous difference equations and populations biology. J. Differ. Equ. 208, 258–273 (2005)

    Article  MATH  Google Scholar 

  31. Kon, R.: A note on attenuant cycles of population models with periodic carrying capacity. J. Differ. Equ. Appl. 10(8), 791–793 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  32. Liz, E.: Local stability implies global stability in some one-dimensional discrete single-species models. Discrete Contin. Dyn. B 7(1), 191–199 (2007)

    MathSciNet  MATH  Google Scholar 

  33. Solis, F., Chen, B., Kojouharov, H.: A classification of slow convergence near parametric periodic points of discrete dynamical systems. Int. J. Comput. Math. 93(6), 1011–1021 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  34. Solis, F.J., Chen-Charpentier, B.M., Kojouharov, H.V.: Multidimensional discrete dynamical systems with slow behavior. Differ. Equ. Dyn. Syst. (2017). https://doi.org/10.1007/s12591-017-0388-0

    Article  Google Scholar 

  35. Jerez, S., Chen, B.: Stability analysis of a Komarova type model for the interactions of osteoblast and osteoclast cells during bone remodeling. Math. Biosci. 264, 29–37 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  36. Baker, G.L., Blackburn, J.A.: The Pendulum: A Case Study in Physics. Oxford University Press, Oxford (2005)

    MATH  Google Scholar 

  37. Dzyubak, L., Dzyubak, O., Awrejcewicz, J.: Controlling and stabilizing unpredictable behaviour of metabolic reactions and carcinogenesis in biological systems. J. Nonlinear Dyn. 97, 1853–1866 (2019)

    Article  Google Scholar 

  38. Sims, N.A., Martin, T.J.: Coupling the activities of bone formation and resorption: a multitude of signals within the basic multicellular unit. BoneKEy Rep. 3, 1–10 (2014)

    Google Scholar 

  39. Raggatt, L.J., Partridge, N.C.: Cellular and molecular mechanisms of bone remodeling. J. Biol. Chem. 285(33), 25103–25108 (2010)

    Article  Google Scholar 

  40. Zumsande, M., Stiefs, D., Siegmund, S., Gross, T.: General analysis of mathematical models for bone remodeling. Bone 48(4), 910–917 (2011)

    Article  Google Scholar 

  41. Kenkre, J., Bassett, J.: The bone remodelling cycle. Ann. Clin. Biochem. 55(3), 308–327 (2018)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. CIMAT, 36000, Guanajuato, GTO, Mexico

    Silvia Jerez, Emilene Pliego & Francisco J. Solis

Authors
  1. Silvia Jerez
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Emilene Pliego
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Francisco J. Solis
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Francisco J. Solis.

Ethics declarations

Conflicts of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

This work was supported by CONACyT, Mexico Project CB2016-286437.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Jerez, S., Pliego, E. & Solis, F.J. Oscillatory behavior in discrete slow power-law models. Nonlinear Dyn 102, 1553–1566 (2020). https://doi.org/10.1007/s11071-020-05982-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-020-05982-z

Keywords

Mathematics Subject Classification

Search

Navigation