Skip to main content
SpringerLink
Log in

Dynamical system method for investigating existence and dynamical property of solution of nonlinear time-fractional PDEs

  • Original paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

Abstract

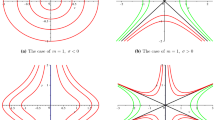
In this paper, based on the dynamical system method, a new approach for investigating solutions of nonlinear time-fractional partial differential equations (PDEs) is introduced. By proposing a novel technique with separation variables, the phase portraits of system derived from the nonlinear time-fractional PDEs are analyzed, and the issue of existence for the solution of the time-fractional PDEs is considered. Moreover, the dynamical properties of the solution of the time-fractional PDEs are studied in detail. As examples, three nonlinear time-fractional models such as the reaction–diffusion model, the biology population model and the fluid model are studied by using this new approach. In some special parametric conditions, exact solutions of these models are obtained. The dynamical properties of some exact solutions are illustrated by graphs. Compared with the results in current studies, the results obtained in this paper are new.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

References

  1. Daftardar-Gejji, V., Jafari, H.: Adomian decomposition: a tool for solving a system of fractional differential equations. J. Math. Anal. Appl. 301(2), 508–518 (2005)

    MathSciNet  MATH  Google Scholar 

  2. Bakkyaraj, T., Sahadevan, R.: An approximate solution to some classes of fractional nonlinear partial differential difference equation using a domian decomposition method. J. Fract. Calc. Appl. 5(1), 37–52 (2014)

    MathSciNet  Google Scholar 

  3. Bakkyaraj, T., Sahadevan, R.: Approximate analytical solution of two coupled time fractional nonlinear Schrodinger equations. Int. J. Appl. Comput. Math. 2(1), 113–135 (2016)

    MathSciNet  MATH  Google Scholar 

  4. Bakkyaraj, T., Sahadevan, R.: On solutions of two coupled fractional time derivative Hirota equations. Nonlinear Dyn. 77(4), 1309–1322 (2014)

    MathSciNet  Google Scholar 

  5. Eslami, M., Vajargah, B.F., Mirzazadeh, M., Biswas, A.: Applications of first integral method to fractional partial differential equations. Indian J. Phys. 88(2), 177–184 (2014)

    Google Scholar 

  6. Sahadevan, R., Bakkyaraj, T.: Invariant analysis of time fractional generalized Burgers and Korteweg-de Vries equations. J. Math. Anal. Appl. 393(2), 341–347 (2012)

    MathSciNet  MATH  Google Scholar 

  7. Bakkyaraj, T., Sahadevan, R.: Invariant analysis of nonlinear fractional ordinary differential equations with Riemann-Liouville derivative. Nonlinear Dyn. 80(1), 447–455 (2015)

    MathSciNet  MATH  Google Scholar 

  8. Odibat, Z.M., Shaher, M.: The variational iteration method: an efficient scheme for handling fractional partial differential equations in fluid mechanics. Comput. Math. Appl. 58, 2199–2208 (2009)

    MathSciNet  MATH  Google Scholar 

  9. Wu, G., Lee, E.W.M.: Fractional variational iteration method and its application. Phys. Lett. A 374(25), 2506–2509 (2010)

    MathSciNet  MATH  Google Scholar 

  10. Momani, S., Zaid, O.: Comparison between the homotopy perturbation method and the variational iteration method for linear fractional partial differential equations. Comput. Math. Appl. 54(7), 910–919 (2007)

    MathSciNet  MATH  Google Scholar 

  11. Sahadevan, R., Bakkyaraj, T.: Invariant subspace method and exact solutions of certain nonlinear time fractional partial differential equations. Fract. Calc. Appl. Anal. 18(1), 146–162 (2015)

    MathSciNet  MATH  Google Scholar 

  12. Harris, P.A., Garra, R.: Analytic solution of nonlinear fractional Burgers-type equation by invariant subspace method. Nonlinear Stud. 20(4), 471–481 (2013)

    MathSciNet  MATH  Google Scholar 

  13. Sahadevan, R., Prakash, P.: Exact solution of certain time fractional nonlinear partial differential equations. Nonlinear Dyn. 85(1), 659–673 (2016)

    MathSciNet  MATH  Google Scholar 

  14. Artale Harris, P., Garra, R.: Nonlinear time-fractional dispersive equations. Commun. Appl. Ind. Math. 6(1), e-487 (2014)

    MathSciNet  MATH  Google Scholar 

  15. Elsayed, M.E.Z., Yasser, A.A., Reham, M.A.S.: The fractional complex transformation for nonlinear fractional partial differential equations in the mathematical physics. J. Assoc. Arab Univ. Basic Appl. Sci. 19, 59–69 (2016)

    Google Scholar 

  16. Li, Z.B., Zhu, W.H., He, J.H.: Exact solutions of time-fractional heat conduction equation by the fractional complex transform. Thermal Sci. 16(2), 335–338 (2012)

    Google Scholar 

  17. Li, Z.B., He, J.H.: Fractional complex transform for fractional differential equations. Math. Comput. Appl. 15(5), 970–973 (2010)

    MathSciNet  MATH  Google Scholar 

  18. Kaplan, M., Bekir, A.: A novel analytical method for time-fractional differential equations. Optik 127, 8209–8214 (2016)

    Google Scholar 

  19. Chen, J., Liu, F., Anh, V.: Analytical solution for the time-fractional telegraph equation by the method of separating variables. J. Math. Anal. Appl. 338, 1364–1377 (2008)

    MathSciNet  MATH  Google Scholar 

  20. Jiang, H., Liu, F., Turner, I., Burrage, K.: Analytical solutions for the multi-term time-fractional diffusion-wave/diffusion equations in a finite domain. Comput. Math. Appl. 64, 3377–3388 (2012)

    MathSciNet  MATH  Google Scholar 

  21. Luchko, Y.: Some uniqueness and existence results for the initial-boundary-value problems for the generalized time-fractional diffusion equation. Comput. Math. Appl. 59, 1766–1772 (2010)

    MathSciNet  MATH  Google Scholar 

  22. Jumarie, G.: Fractional partial differential equations and modified Riemann-Liouville derivative new methods for solution. J. Appl. Math. Comput. 24(1–2), 31–48 (2007)

    MathSciNet  MATH  Google Scholar 

  23. Jumarie, G.: Modified Riemann-Liouville derivative and fractional Taylor series of non-differentiable functions further results. Comput. Math. Appl. 51(9–10), 1367–1376 (2006)

    MathSciNet  MATH  Google Scholar 

  24. Jumarie, G.: Cauchy’s integral formula via the modified Riemann-Liouville derivative for analytic functions of fractional order. Appl. Math. Lett. 23(12), 1444–1450 (2010)

    MathSciNet  MATH  Google Scholar 

  25. He, J.H.: Geometrical explanation of the fractional complex transform and derivative chain rule for fractional calculus. Phys. Lett. A 376, 257–259 (2012)

    MathSciNet  MATH  Google Scholar 

  26. Tarasov, V.E.: On chain rule for fractional derivatives. Commun. Nonlinear Sci. Numer. Simul. 30(1), 1–4 (2016)

    MathSciNet  Google Scholar 

  27. Rui, W.: Applications of homogenous balanced principle on investigating exact solutions to a series of time fractional nonlinear PDEs. Commun. Nonlinear Sci. Numer. Simul. 47, 253–266 (2017)

    MathSciNet  Google Scholar 

  28. Rui, W.: Applications of integral bifurcation method together with homogeneous balanced principle on investigating exact solutions of time fractional nonlinear PDEs. Nonlinear Dyn. 91, 679–712 (2018)

    MathSciNet  Google Scholar 

  29. Wu, C., Rui, W.: Method of separation variables combined with homogenous balanced principle for searching exact solutions of nonlinear time-fractional biological population model. Commun. Nonlinear Sci. Numer. Simul. 63, 88–100 (2018)

    MathSciNet  Google Scholar 

  30. Rui, W.: Ideal of invariant subspace combined with elementary integral method for investigating exact solutions of time-fractional NPDEs. Appl. Math. Comput. 339, 158–171 (2018)

    MathSciNet  MATH  Google Scholar 

  31. Li, J., Liu, Z.: Smooth and non-smooth traveling waves in a nonlinearly dispersive equation. Appl. Math. Model. 25(1), 41–56 (2000)

    MATH  Google Scholar 

  32. Li, J., Zhang, L.: Bifurcations of travelling wave solutions in generalized Pochhammer–Chree equation. Chaos Solitons Fractals 14(4), 581–593 (2002)

    MathSciNet  MATH  Google Scholar 

  33. Li, J., Li, H., Li, S.: Bifurcations of travelling wave solutions for the generalized Kadomtsev–Petviashili equation. Chaos Solitons Fractals 20, 725–734 (2004)

    MathSciNet  MATH  Google Scholar 

  34. Li, J., Chen, G.: Bifurcations of traveling wave solutions for four classes of nonlinear wave equations. Int. J. Bifurc. Chaos 15(2), 3973–3998 (2005)

    MathSciNet  MATH  Google Scholar 

  35. Gumey, W.S.C., Nisbet, R.M.: The regulation of inhomogenous populations. J. Theor. Biol. 52, 441–457 (1975)

    Google Scholar 

  36. El-Sayed, A.M.A., Rida, S.Z., Arafa, A.A.M.: Exact solutions of fractional-order biological population model. Commun. Theor. Phys. 52(6), 992–996 (2009)

    MathSciNet  MATH  Google Scholar 

  37. Li, Y., Li, Z., Zhang, Y.: Homotopy perturbation method to fractional biological population equation. Fract. Differ. Calc. 1, 117–124 (2011)

    MathSciNet  MATH  Google Scholar 

  38. Lu, Y.G.: Hölder estimates of solutions of biological population equations. Appl. Math. Lett. 13(6), 123–126 (2000)

    MathSciNet  MATH  Google Scholar 

  39. Gurtin, M.E., Maccamy, R.C.: On the diffusion of biological populations. Math. Biosci. 33(1–2), 35–49 (1977)

    MathSciNet  MATH  Google Scholar 

  40. Bear, J.: Dynamics of Fluids in Porou Media. American Elsevier, New York (1972)

    MATH  Google Scholar 

  41. Okubo, A.: Diffusion and Ecological Problem. Mathematical Models, Biomathematics 10. Springer, berlin (1980)

    Google Scholar 

  42. Zhang, S., Zhang, H.Q.: Fractional sub-equation method and its applications to nonlinear fractional PDEs. Phys. Lett. A 375(7), 1069–1073 (2011)

    MathSciNet  MATH  Google Scholar 

  43. Blasiak, S.: Time-fractional heat transfer equations in modeling of the non-contacting face seals. Int. J. Heat Mass Transf. 100, 79–88 (2016)

    Google Scholar 

  44. Malaguti, L., Marcelli, C.: Sharp profiles in degenerate and doubly degenerate Fisher-KPP equations. J. Differ. Equ. 195(2), 471–496 (2003)

    MathSciNet  MATH  Google Scholar 

  45. de Pablo, A., Vázquez, J.L.: Travelling waves and finite propagation in a reaction-diffusion equation. J. Differ. Equ. 93(1), 19–61 (1991)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

This study was funded by the National Natural Science Foundation of China (Grant No. 11361023), the Natural Science Foundation in Chongqing City of China (Grant No. cstc2018jcyjAX0766) and the Special Support Program for High-level Talents in Chongqing City of China (Grant No. cstc2018kjcxljrc0049).

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Chongqing Normal University, Chongqing, 401331, People’s Republic of China

    Weiguo Rui

Authors
  1. Weiguo Rui
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Weiguo Rui.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Rui, W. Dynamical system method for investigating existence and dynamical property of solution of nonlinear time-fractional PDEs. Nonlinear Dyn 99, 2421–2440 (2020). https://doi.org/10.1007/s11071-019-05410-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-019-05410-x

Keywords

Search

Navigation