Primary resonance analysis and vibration suppression for the harmonically excited nonlinear suspension system using a pair of symmetric viscoelastic buffers

Abstract

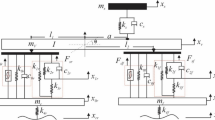
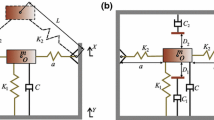
To reduce the severity of high-magnitude vibrations and shock, end-stop buffers was used for the most suspension cabs or seats of work vehicles. This paper employs a pair of symmetric linear viscoelastic end-stops to improve the performance of a single-degree-of-freedom nonlinear suspension system under primary resonance conditions, which has cubic nonlinearity. Firstly, a piecewise symmetry tri-nonlinear model is introduced. The frequency response of relative displacement corresponding to the steady-state motion is obtained by applying the multiple-scale method, which is found to be the same with the averaging method solution. And it is further verified by numerical simulation. Its stability is then studied. Subsequently, a design criterion is proposed for jump avoidance, which is caused by the saddle-node bifurcation. Also, parametric studies are carried out to illustrate effects of design parameters for the end-stop on the isolation performance at primary resonance, including responses of the relative displacement and the absolute acceleration. The results show that with dynamic parameters properly designed by using viscoelastic end-stops, the relative displacement response can be effectively suppressed and the jump can be eliminated for both hardening and softening primary isolators. Besides, the end-stop can effectively attenuate the absolute acceleration response for a hardening primary isolator, while more damping is needed to attenuate that for a softening primary isolator, although the degree of the softening nonlinearity is mitigated. It is suggested that a moderate stiffness compared to that of the primary isolator and also a high damping of the end-stop be beneficial to both vibration isolation and jump avoidance under primary resonance conditions.

Appendices

Appendix A

Using the averaging method

$$\begin{aligned} Y= & {} \bar{{A}}(\tau )\cos ({\lambda \tau +{\bar{\gamma }}(\tau )}) \end{aligned}$$

(A1)

$$\begin{aligned} {\dot{Y}}= & {} -\,\bar{{A}}\lambda \sin ({\lambda \tau +{\bar{\gamma }}}) \end{aligned}$$

(A2)

Then, the following equation is implied

$$\begin{aligned} \dot{\bar{{A}}}\cos ({\lambda \tau +{\bar{\gamma }}}) -\bar{{A}}\dot{{\bar{\gamma }}} \sin ({\lambda \tau +{\bar{\gamma }}})=0 \end{aligned}$$

(A3)

And the acceleration is

$$\begin{aligned} {\ddot{Y}}= & {} -\,\dot{\bar{{A}}}\lambda \sin ({\lambda \tau +{\bar{\gamma }}}) -\bar{{A}}\lambda ^{2}\cos ({\lambda \tau +{\bar{\gamma }}}) \nonumber \\&-\,\bar{{A}}\dot{{\bar{\gamma }}} \lambda \cos ({\lambda \tau +{\bar{\gamma }}}) \end{aligned}$$

(A4)

Considering $\varphi =\lambda \tau +{\bar{\gamma }}$ and defining $\varphi _0 =\left\{ {\begin{array}{ll} \arccos \frac{d}{\bar{{A}}}\in \left( {0,\frac{\pi }{2}} \right) &{} \bar{{A}}>d \\ 0 &{} \bar{{A}}\le d \\ \end{array}} \right.$, substitute (A1), (A2) and (A4) into Eq. (2)

$$\begin{aligned}&-\,\dot{\bar{{A}}}\lambda \sin \varphi -\bar{{A}}\lambda ({\lambda +\dot{{\bar{\gamma }}}}) \cos \varphi +\bar{{A}}\cos \varphi \nonumber \\&\qquad +\,\mu \bar{{A}}^{3}\cos ^{3}\varphi -2\xi _1 \bar{{A}}\lambda \sin \varphi +G_1 \nonumber \\&\quad =\lambda ^{2}\cos ({\varphi -{\bar{\gamma }}} ) \end{aligned}$$

(A5)

where

$$\begin{aligned} G_1 =\left\{ {\begin{array}{ll} \rho ^{2} ({\bar{{A}}\cos \varphi -d})-2\xi _2 \bar{{A}}\lambda \sin \varphi &{} 0<\varphi<\varphi _0 \quad \hbox {or}\quad 2\pi -\varphi _0<\varphi<2\pi \\ 0 &{} \varphi _0 \le \varphi \le \pi -\varphi _0\quad \hbox {or}\quad \pi +\varphi _0 \le \varphi \le 2\pi -\varphi _0 \\ \rho ^{2} ({\bar{{A}}\cos \varphi +d})-2\xi _2 \bar{{A}}\lambda \sin \varphi &{} \pi -\varphi _0<\varphi <\pi +\varphi _0 \end{array}} \right. \end{aligned}$$

Because only $\varphi _0 \le \varphi \le \pi -\varphi _0$ or $\pi +\varphi _0 \le \varphi \le 2\pi -\varphi _0$ can be true when $\varphi _0 =0$, $G_{1}$ is true under both conditions of $A>d$ and $A\le d$.

Solving Eqs. (A3) and (A5) for $\dot{\bar{{A}}}$ and $\dot{{\bar{\gamma }}}$, we have

$$\begin{aligned} \lambda \dot{\bar{{A}}}= & {} -\,\bar{{A}}({\lambda ^{2}-1})\cos \varphi \sin \varphi +\mu \bar{{A}}^{3}\cos ^{3}\varphi \sin \varphi \nonumber \\&-\,2\xi _1 \bar{{A}}\lambda \sin ^{2}\varphi +G_1 \sin \varphi \nonumber \\&-\,\lambda ^{2}\cos ({\varphi -{\bar{\gamma }}})\sin \varphi \end{aligned}$$

(A6)

$$\begin{aligned} \lambda \bar{{A}}\dot{{\bar{\gamma }}}= & {} -\bar{{A}} ({\lambda ^{2}-1}) \cos ^{2}\varphi +\mu \bar{{A}}^{3}\cos ^{4}\varphi \nonumber \\&-\,2\xi _1 \bar{{A}}\lambda \sin \varphi \cos \varphi +G_1 \cos \varphi \nonumber \\&-\,\lambda ^{2}\cos ({\varphi -{\bar{\gamma }}})\cos \varphi \end{aligned}$$

(A7)

Averaging them over the period $2\pi /\lambda$, we have

$$\begin{aligned} \lambda \dot{\bar{{A}}}= & {} -\,\xi _1 \lambda \bar{{A}}-\frac{1}{\pi } \xi _2 \lambda \bar{{A}} ({2\varphi _0 -\sin 2\varphi _0}) \nonumber \\&-\,\frac{1}{2}\lambda ^{2}\sin {\bar{\gamma }} \end{aligned}$$

(A8)

$$\begin{aligned} \lambda \bar{{A}}\dot{{\bar{\gamma }}}= & {} -\frac{1}{2}\bar{{A}} ({\lambda ^{2}-1})+\frac{3}{8}\mu \bar{{A}}^{3} \nonumber \\&+\,\frac{1}{2\pi } ({2\rho ^{2}\bar{{A}}\varphi _0 -4d\rho ^{2}\sin \varphi _0 +\rho ^{2}\bar{{A}}\sin 2\varphi _0}) \nonumber \\&-\, \frac{1}{2}\lambda ^{2}\cos {\bar{\gamma }} \end{aligned}$$

(A9)

At the steady-state condition, the derivatives ${\dot{A}}$ and $\dot{{\bar{\gamma }}}$ can be considered to be zero. Then,

$$\begin{aligned} \lambda ^{2}\sin {\bar{\gamma }}= & {} -2\xi _1 \lambda \bar{{A}}-\frac{2}{\pi } \xi _2 \lambda \bar{{A}} ({2\varphi _0 -\sin 2\varphi _0}) \end{aligned}$$

(A10)

$$\begin{aligned} \lambda ^{2}\cos {\bar{\gamma }}= & {} -\bar{{A}}({\lambda ^{2}-1}) +\frac{3}{4}\mu \bar{{A}}^{3} \nonumber \\&+\frac{1}{\pi } \left( 2\rho ^{2}\bar{{A}}\varphi _0 -4d\rho ^{2}\sin \varphi _0 \right. \nonumber \\&\left. \quad +\rho ^{2}\bar{{A}}\sin 2\varphi _0\right) \end{aligned}$$

(A11)

Therefore,

$$\begin{aligned}&\left[ -\bar{{A}}({\lambda ^{2}-1})+\frac{3}{4}\mu \bar{{A}}^{3} \right. \nonumber \\&\quad \left. +\frac{\rho ^{2}}{\pi } ({2\bar{{A}}\varphi _0 -4d\sin \varphi _0 +\bar{{A}}\sin 2\varphi _0})\right] ^{2} \nonumber \\&\quad +\bar{{A}}^{2}\lambda ^{2}\left[ {2\xi _1 +\frac{2}{\pi } \xi _2 ({2\varphi _0 -\sin 2\varphi _0})} \right] ^{2}=\lambda ^{4}\nonumber \\ \end{aligned}$$

(A12)

Appendix B

$$\begin{aligned} R_1^{\prime } (A)= & {} \frac{2a_2 \rho ^{2}}{A} \end{aligned}$$

(B1)

$$\begin{aligned} R_2^{\prime } (A)= & {} \frac{4a_2 \xi _2}{A} \end{aligned}$$

(B2)