Global dynamics of a stochastic avian–human influenza epidemic model with logistic growth for avian population

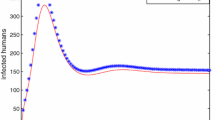
Abstract

In this paper, a stochastic avian–human influenza epidemic model with logistic growth for avian population is investigated. This model describes the transmission of avian influenza among avian population and human population in random environments. The dynamical behavior of this model is discussed. Firstly, the existence and uniqueness of the global positive solution are obtained. Then persistence in the mean and extinction of the infected avian population is studied. Furthermore, sufficient conditions for the existence of an ergodic stationary distribution of stochastic avian–human influenza model are obtained. We find a threshold of this stochastic model which determines the outcome of the disease in case the white noises are small. Results show that environmental white noise is helpful for disease control. Finally, numerical simulations validate the analytical results.

Appendix

We introduce some results concerning the stationary distribution. For more details see [20].

Let X(t) be a homogeneous Markov process in $E^l$ ($E^l$ denotes euclidean l-space) satisfying the stochastic equation

$$\begin{aligned} \mathrm{d} X(t)=h(X) \mathrm{d} t+\sum _{m=1}^{k}g_\mathrm{m}(X) \mathrm{d} B_\mathrm{m}(t). \end{aligned}$$

The diffusion matrix is

$$\begin{aligned} \bar{A}(x)=(\bar{a}_{ij}(x)), \quad \bar{a}_{ij}(x)=\sum _{m=1}^{k}g_\mathrm{m}^{(i)}(x)g_\mathrm{m}^{(j)}(x). \end{aligned}$$

Assumption 1

There is a bounded domain $U\subset E^l$ with regular boundary $\Gamma$, which has the properties that

• (B1) In the domain U and some neighborhood thereof, the smallest eigenvalue of the diffusion matrix $\bar{A}(x)$ is bounded away from zero.

• (B2) If $x\in E^l{\setminus } U$, the mean time $\tau$ at which a path issuing from x reaches the set U is finite, and $\sup _{x\in \mathbb {K}}\mathbb {E}_x\tau <+\infty$ for every compact subset $\mathbb {K}\in E^l$.

Lemma 4

(See [18]) If Assumption 1 holds, then the Markov process X(t) has a stationary distribution $\mu (\cdot )$. Let $f(\cdot )$ be a function integrable with respect to the measure $\mu$. Then

$$\begin{aligned} \mathbb {P}\left\{ \lim _{t\rightarrow \infty }\frac{1}{t}\int _{0}^{t}f(X(s)) \mathrm{d} s=\int _{E^l}f(x)\mu ( \mathrm{d} x)\right\} =1. \end{aligned}$$

In order to verify (B1), we only need to show that F is uniformly elliptical in U, where $F(u)=h(x)u_x+0.5\,\text {trace}(\bar{A}(x)u_{xx})$, that is to say, there is $M>0$ such that

$$\begin{aligned} \sum _{i,j=1}^{k}\bar{a}_{ij}(x)\xi _i\xi _{j}>M|\xi |^2,\quad x\in U,\quad \xi \in \mathbb {R}^k. \end{aligned}$$

(19)

(see Chapter 3 of [23] and Rayleigh’s principle in [24]). To verify (B2), it suffices to prove that there exist a neighborhood U and a nonnegative $C^2$-function such that for any $x \in E^l{\setminus } U, \mathcal {L}V$ is negative (see [21]).