Estimation and elimination of eigenvalue splitting and vibration instability of ring-shaped periodic structure subjected to three-axis angular velocity components

Abstract

Stable operation is one of the most crucial requirements for resonators in vibratory gyroscopes and ultrasonic motors, but eigenvalue splitting can deteriorate operation stability. This work aims at the estimation and elimination of eigenvalue splitting and vibration instability of resonators arranged in a fashion of ring-shaped periodic structures (RPS). An analytical model is developed by Hamilton’s principle, where in-plane bending displacements, grouped supports and angular velocity components applied about three orthogonal directions are incorporated. Eigensolutions for the proposed rotational and mirror symmetric topologies are formulated by perturbation-superposition method, based on which eigenvalue splitting, vibration instability and their evolution with grouped supports and angular velocity are examined. The results verify the behaviors of splitting and instability share similar rules with those RPS having equally-spaced supports, but they change remarkably with grouping patterns. The dependences of grouping patterns and parameters on vibrations are demonstrated based on sample RPS. The splitting and instability are estimated by eigensolutions, and they can be suppressed or even eliminated by the proposed two types of topologies. Comparisons between the two topologies are made in terms of the requirements from engineering practice. Main results are also compared with those in the open literature.

Appendices

Appendix 1

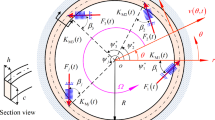
This section calculates the kinetic energy with the method in Ref. [19]. The point q₁ in Fig. 1 is expressed as

$$ \varvec{U}_{1} = \varvec{r}_{\text{0}} + \varvec{r}_{{\text{q}_{0} }} + \varvec{U}_{0} $$

(39)

where $\varvec{r}_{\text{0}}$, $\varvec{r}_{{\text{q}_{0} }}$ and $\varvec{U}_{0}$ are displacement vectors of the geometric center relative to the origin of inertial coordinates, the arbitrary point with respect to the geometric center of the unformed ring, and the instantaneous position on the deformed ring relative to ${\text{q}}_{0}$. $\varvec{r}_{{\text{q}_{0} }}$ is written in o-xyz as

$$ \varvec{r}_{{{\text{q}}_{0} }} = x_{{{\text{q}}_{0} }} {\mathbf{i}} + y_{{{\text{q}}_{0} }} {\mathbf{j}} $$

(40)

where $x_{{{\text{q}}_{0} }}$ and $y_{{{\text{q}}_{0} }}$ are the coordinates. $\varvec{U}_{0}$ is written as

$$ \varvec{U}_{0} = U_{0x} {\mathbf{i}} + U_{0y} {\mathbf{j}} $$

(41)

where $U_{0x}$ and $U_{0y}$ are vibration displacements about x and y axes, respectively.

In the inertial coordinates, the revolution speed can be expressed as

$$ \varvec{\varOmega}_{ 0} = \varOmega_{x 0} {\mathbf{i}} + \varOmega_{y 0} {\mathbf{j}} + \varOmega_{z 0} {\mathbf{k}} $$

(42)

Assuming the speed of origin is

$$ \varvec{v}_{o} = \frac{{\text{d}\varvec{r}_{ 0} }}{{\text{d}t}} = v_{0x} {\mathbf{i}} + v_{0y} {\mathbf{j}} + v_{0z} {\mathbf{k}} $$

(43)

where $v_{0x}$, $v_{0y}$ and $v_{0z}$ are components in the three orthogonal directions, respectively. The speed of ${\text{q}}_{0}$ is

$$ \varvec{v}_{{\text{q}_{0} }} = v_{{\text{q}_{0x} }} {\mathbf{i}} + v_{{\text{q}_{0y} }} {\mathbf{j}} + v_{{\text{q}_{0z} }} {\mathbf{k}} $$

(44)

where

$$ v_{{{\text{q}}_{0x} }} = v_{0x} + \dot{U}_{0x} - y_{{{\text{q}}_{0} }} \varOmega_{z0} - U_{0y} \varOmega_{z0} ,\;v_{{{\text{q}}_{0y} }} = v_{0y} + \dot{U}_{0y} - x_{{{\text{q}}_{0} }} \varOmega_{z0} + U_{0x} \varOmega_{z0} $$

$$ v_{{\text{q}_{0z} }} = v_{0z} + y_{{\text{q}_{0} }} \varOmega_{x 0} + U_{0y} \varOmega_{x 0} - x_{{{\text{q}}_{0} }} \varOmega_{y 0} - U_{0x} \varOmega_{y 0} $$

where “·” means the first derivative of variable versus time. Forming the dot product of velocities yields

$$ \begin{aligned} \varvec{v}_{{{\text{q}}_{0} }} \varvec{.v}_{{{\text{q}}_{0} }} & = \dot{U}_{0x}^{2} + \dot{U}_{0y}^{2} + 2\varOmega_{z0} (U_{0x} \dot{U}_{0y} - \dot{U}_{0x} U_{0y} ) + (\varOmega_{y0}^{2} + \varOmega_{z0}^{2} )U_{0x}^{2} \\ & \quad + \,(\varOmega_{x0}^{2} + \varOmega_{z0}^{2} )U_{0y}^{2} - 2\varOmega_{x0} \varOmega_{y0} y_{{{\text{q}}_{0} }} U_{0x} - 2\varOmega_{x0} \varOmega_{y0} x_{{{\text{q}}_{0} }} U_{0y} + 2(\varOmega_{x0}^{2} + \varOmega_{z0}^{2} )y_{{{\text{q}}_{0} }} U_{0y} \\ & \quad + \,2(v_{0x} - \varOmega_{z0} y_{{{\text{q}}_{0} }} )\dot{U}_{0x} + 2(v_{0y} + \varOmega_{z0} x_{{{\text{q}}_{0} }} )\dot{U}_{0y} + 2(\varOmega_{z0} v_{0y} - \varOmega_{y0} v_{0z} )U_{0x} \\ & \quad - \,2(\varOmega_{z0} v_{0x} - \varOmega_{x0} v_{0z} )U_{0y} - 2\varOmega_{x0} \varOmega_{y0} U_{0x} U_{0y} + 2(\varOmega_{y0}^{2} + \varOmega_{z0}^{2} )x_{{{\text{q}}_{0} }} U_{0x} \\ \end{aligned} $$

(45)

Equation (45) excludes the terms being irrelevant to the governing equation of motion. By introducing polar coordinates, the displacements are rewritten as

$$ \left[ {\begin{array}{*{20}c} {U_{{\text{0}x}} } \\ {U_{{\text{0}y}} } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} {\cos \theta } & { - \,\sin \theta } \\ {\sin \theta } & {\cos \theta } \\ \end{array} } \right]\left[ {\begin{array}{*{20}c} v \\ u \\ \end{array} } \right] $$

(46)

Position of q₀ is defined as

$$ \left[ {\begin{array}{*{20}c} {x_{{\text{q}_{0} }} } \\ {y_{{\text{q}_{0} }} } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} {R\cos \theta } \\ {R\sin \theta } \\ \end{array} } \right] $$

(47)

Substituting Eqs. (46) and (47) into (45) yields

$$ \begin{aligned} \varvec{v}_{{{\text{q}}_{0} }} .\varvec{v}_{{{\text{q}}_{0} }} & = \dot{u}^{2} + \dot{v}^{2} + 2\varOmega_{z0} (\dot{u}v - u\dot{v}) + (\varOmega_{y0}^{2} + \varOmega_{z0}^{2} )(v\cos \theta - u\sin \theta )^{2} \\ & \quad + \,2v_{0x} (\dot{v}\cos \theta - \dot{u}\sin \theta ) + 2v_{0y} (\dot{v}\sin \theta + \dot{u}\sin \theta ) + 2R(\varOmega_{z0} \dot{u} + \varOmega_{z0}^{2} v) \\ & \quad - \,2\varOmega_{x0} \varOmega_{y0} \left[ {R(v\sin 2\theta + u\cos 2\theta ) + (v\cos \theta - u\sin \theta )(v\sin \theta + u\cos \theta )} \right] \\ & \quad + \,2(\varOmega_{z0} v_{0y} - \varOmega_{y0} v_{0z} )(v\cos \theta - u\sin \theta ) + 2(\varOmega_{x0} v_{0z} - \varOmega_{z0} v_{0x} )(v\sin \theta + u\cos \theta ) \\ & \quad + \,(\varOmega_{x0}^{2} + \varOmega_{z0}^{2} )(v\sin \theta + u\cos \theta )^{2} + 2\varOmega_{x0}^{2} R(v\sin \theta + u\cos \theta )\sin \theta \\ & \quad + \,2\varOmega_{y0}^{2} R(v\cos \theta - u\sin \theta )\cos \theta \\ \end{aligned} $$

(48)

Appendix 2

See Tables 4, 5, 6, 7 and 8

Table 4 Eigenvalues of rotational symmetric RPS (pure-imaginary $r_{n0}$, 2n/N₁ = int)

Table 5 Eigenvalues of rotational symmetric RPS (impure-imaginary $r_{n0}$, 2n/N₁ = int)

Table 6 Eigenvalues of rotational symmetric RPS with 2n/N₁${ \ne }$ int

Table 7 Eigenvalues of mirror symmetric RPS (pure-imaginary $r_{n0}$)

Table 8 Eigenvalues of mirror symmetric RPS (impure-imaginary $r_{n0}$)

.