Skip to main content
SpringerLink
Log in

On the Full Idle Time in Multiphase Queueing Systems

  • Published:
Lithuanian Mathematical Journal Aims and scope Submit manuscript

Abstract

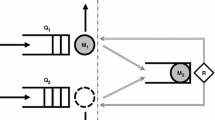
The modern queueing theory is a powerful tool for a quantitative and qualitative analysis of communication systems, computer networks, transportation systems, and many other technical systems. The paper is designated to the analysis of queueing systems arising in the network theory and communications theory (such as the so-called multiphase queueing systems, tandem queues, or series of queueing systems). We present heavy traffic limit theorems for the full idle time in multiphase queueing systems. We prove functional limit theorems for values of the full idle time of a queueing system, which is its important probability characteristic.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

REFERENCES

  1. P. Billingsley, Convergence of Probability Measures, Wiley, New York (1968).

    MATH  Google Scholar 

  2. D. Iglehart, Weak convergence in queueing theory, Adv. Appl. Probab., 5, 570–594 (1973).

    MATH  MathSciNet  Google Scholar 

  3. D. Iglehart and W. Whitt, Multiple channel queues in heavy traffic, I, Adv. Appl. Probab., 2, 150–177 (1973).

    MathSciNet  Google Scholar 

  4. F. Karpelevich and A. Kreinin, Heavy Traffic Limits for Multiphase Queues, Amer. Math. Soc., Providence, Rhode Island (1994).

    MATH  Google Scholar 

  5. O. Kella, Concavity and reflected Levy process, J. Appl. Probab., 29(1), 209–215 (1992).

    MATH  MathSciNet  Google Scholar 

  6. P. Milch and M. Waggoner, A random walk approach to a shutdown queueing system, SIAM J. Appl. Math., 19, 103–115 (1970).

    Article  MathSciNet  MATH  Google Scholar 

  7. Minkevicius, Weak convergence in multiphase queues, Lith. Math. J., 26, (1986), 347–351.

    Article  MATH  MathSciNet  Google Scholar 

  8. M. Pike, Some numerical results for the queueing system D/Ek/1, J. R. Statist. Soc. Ser. B, 25, 477–488 (1963).

    MATH  MathSciNet  Google Scholar 

  9. N. Prabhu, Stochastic Storage Processes, Springer, New York (1980).

    MATH  Google Scholar 

  10. A. Puhalskii, Moderate deviations for queues in critical loading, Queueing Systems Theory Appl., 31(3–4), 359–392 (1999).

    MATH  MathSciNet  Google Scholar 

  11. M. Ridel, Conditions for stationarity in a single server queueing system, Zastos. Mat., 15(1), 17–24 (1976).

    MathSciNet  Google Scholar 

  12. L. Takacs, Occupation time problems in the theory of queues, in: Lecture Notes in Econom. and Math. Systems, 98, Springer, Berlin (1974), pp. 91–131.

    Google Scholar 

  13. W. Whitt, Limits for cumulative input processes to queues, Probab. Engrg. Inform. Sci., 14(2), 123–150 (2000).

    MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Mathematics and Informatics, Akademijos 4, LT-08663, Vilnius, Lithuania

    S. Minkevicius

  2. Vilnius Gediminas Technical University, Sauletekio 11, LT-10223, Vilnius, Lithuania

    S. Minkevicius

Authors
  1. S. Minkevicius
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

__________

Translated from Lietuvos Matematikos Rinkinys, Vol. 45, No. 3, pp. 367–386, July–September, 2005.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Minkevicius, S. On the Full Idle Time in Multiphase Queueing Systems. Lith Math J 45, 299–314 (2005). https://doi.org/10.1007/s10986-005-0032-5

Download citation

  • Received:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10986-005-0032-5

Keywords

Search

Navigation