Boltzmann Entropy of a Freely Expanding Quantum Ideal Gas

Abstract

We study the time evolution of the Boltzmann entropy of a microstate during the non-equilibrium free expansion of a one-dimensional quantum ideal gas. This quantum Boltzmann entropy, $S_B$, essentially counts the “number” of independent wavefunctions (microstates) giving rise to a specified macrostate. It generally depends on the choice of macrovariables, such as the type and amount of coarse-graining, specifying a non-equilibrium macrostate of the system, but its extensive part agrees with the thermodynamic entropy in thermal equilibrium macrostates. We examine two choices of macrovariables: the U-macrovariables are local observables in position space, while the f-macrovariables also include structure in momentum space. For the quantum gas, we use a non-classical choice of the f-macrovariables. For both choices, the corresponding entropies $s_B^f$ and $s_B^U$ grow and eventually saturate. As in the classical case, the growth rate of $s_B^f$ depends on the momentum coarse-graining scale. If the gas is initially at equilibrium and is then released to expand to occupy twice the initial volume, the per-particle increase in the entropy for the f-macrostate, $\Delta s_B^f$, satisfies $\log {2}\le \Delta s_B^f\le 2\log {2}$ for fermions, and $0\le \Delta s_B^f\le \log {2}$ for bosons. For the same initial conditions, the change in the entropy $\Delta s_B^U$ for the U-macrostate is greater than $\Delta s_B^f$ when the gas is in the quantum regime where the final stationary state is not at thermal equilibrium.

Appendices

Marginals of the wavepacket density

Here we establish the fact mentioned in Sec. (5.2) that the marginals of the wave-packet density, $D_\alpha$, after integrating either over momentum or space, correspond respectively to the coarse-grained particle density and momentum density. Let us consider the momentum marginal first i.e. sum over r

$$\begin{aligned} \begin{aligned} D_v(v, t)&= \sum _r D(r, v, t) = \sum _{r, m, n} \langle r, v | m \rangle {\tilde{\rho }}_1(p_m, p_n, t) \langle n | r, v \rangle \\&= \frac{1}{K} \sum _{r=1}^K \sum _{m, n \in {\mathscr {R}}_v} e^{2\pi i (m-n) r/K} {\tilde{\rho }}_1(p_m, p_n, t) \\&= \sum _{n \in {\mathscr {R}}_v} {\tilde{\rho }}_1(p_n, p_n, 0). \end{aligned} \end{aligned}$$

(63)

Let us now consider the r-marginal i.e. sum over v

$$\begin{aligned}&D_r(r, t) = \sum _v D(r, v, t) = \int \frac{dx dx'}{L K}\sum _v \sum _{m, n \in {\mathscr {R}}_v} Q_{m n}(x, x', t), \end{aligned}$$

(64)

$$\begin{aligned}&Q_{m n}(x, x', t) = e^{2\pi i(-m x+n x')/L} e^{i (m - n) 2\pi r/K} \rho _1(x, x', t). \end{aligned}$$

(65)

Note that $m, n \in {\mathscr {R}}_v$ which is given by

$$\begin{aligned} {\mathscr {R}}_v = \left\{ v-\displaystyle {\frac{K-1}{2}}, \ldots , v+\frac{K-1}{2} \right\} . \end{aligned}$$

(66)

We substitute $m=v+{{\tilde{m}}}$ and $n=v+{{\tilde{n}}}$ to get rid of the v dependence in the sums over m and n. We thus get

$$\begin{aligned} D_r(r, t) = \int \frac{dx dx'}{L K} \sum _v \sum _{{{\tilde{m}}}, {{\tilde{n}}} \in {\mathscr {R}}_0} e^{2\pi i v(x'-x)/L} Q_{{{\tilde{m}}} {{\tilde{n}}}}(x, x', t). \end{aligned}$$

(67)

Now since v takes values $\vartheta K$ where $\vartheta$ is an integer that runs from $-\infty$ to $+\infty$, the sum over v can be done and gives

$$\begin{aligned} \begin{aligned} D_r(r, t)&= \int \frac{dx dx'}{ K^2}\sum _{{{\tilde{m}}}, {{\tilde{n}}} \in {\mathscr {R}}_0} \delta (x-x') Q_{{{\tilde{m}}} {{\tilde{n}}}}(x, x', t) \\&= \int \limits _0^L \frac{dx}{ K^2} |{\sum _{{{\tilde{n}}}= -(K-1)/2}^{(K-1)/2} \exp [ 2 \pi i {{\tilde{n}}} \left( \frac{x}{L} - \frac{r}{K} \right) ]}|^2 \rho _1(x,x, t) \\&= \int \limits _0^L dx \,h_K\left( \frac{x}{L} - \frac{r}{K} \right) n(x, t), \end{aligned} \end{aligned}$$

(68)

where n(x, t) is the particle density and $h_K(x) = \displaystyle {\frac{1}{K^2}} \left( \frac{\sin \pi K z}{\sin \pi z} \right) ^2$.

Dependence on K

Here we briefly discuss the dependence of our results on the coarse-graining parameter K.

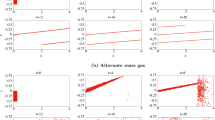
Fermions: In Fig. 13, we plot the saturation value $s_B^f(\infty )$ for different K and for the low and intermediate temperatures. We observe that as long as $K \ll L$, the saturation value does not vary strongly with K. Also, the variation becomes smaller with increasing temperature.

Bosons: The strong dependence on K can be understood from Fig. 14 which shows the variation of the saturation value of the f-macrostate entropy per particle with K. Here, we can clearly see that the variation is stronger for bosons as compared to fermions. As a result, the K values used in our numerical study are not small enough compared to the system size L for bosons, especially in the low-temperature case.

Fig. 13

Fermions: The late time value of the f-macrostate entropy per particle, $s^f_B(\infty )$ as a function of the coarse-graining scale K for the low and intermediate temperatures. It seems that we require $K /L \ll 1$ for the final value to not vary strongly with K. The variation also goes down with increasing temperature

Fig. 14

Bosons: The late time value of the f-macrostate entropy per particle, $s^f_B(\infty )$ as a function of the coarse-graining scale K for the low and intermediate temperatures. It seems that we require $K /L \ll 1$ for the final value to not vary strongly with K. Also note that the variation with K is stronger for bosons as compared to fermions, so a relatively smaller K/L may be required for bosons. Here also, the variation goes down with increasing temperature

Glossary

• f, U: denote the two macrovariables studied in this work.

• $S_B^f, S_B^U$: the Boltzmann entropies corresponding to the two macrovariables.

• $s_B^f, s_B^U$: Boltzmann entropies per particle.

• X: Phase space point.

• ${|{\Phi }\rangle }$: Pure state wavefunction.

• N, E, L: The total number of particles, the total energy, and the system size (Circle perimeter) respectively.

• $T, \beta , \mu$: The temperature, the inverse temperature, and the chemical potential respectively.

• a: Fraction of the circle occupied by the gas at time $t=0$. In most cases $a=1/2$.

• m: Mass of the particles.

• ${\mathscr {H}}$: Hilbert space.

• ${\mathscr {H}}_E$: Subspace of the full Hilbert space corresponding to the energy E.

• ${\hat{M}}_k$: Generic self-adjoint operators.

• $\nu , {\mathscr {H}}_{\nu }$: Generic macrostate and the corresponding macro-space.

• $|{\mathscr {H}}_{\nu }|$: Denotes the dimension of the ${\mathscr {H}}_{\nu }$ macro-space.

• ${\hat{P}}_{\nu }$: Projection operator onto the ${\mathscr {H}}_{\nu }$ macro-space.

• $S_{\nu }$: Boltzmann entropy corresponding to the $\nu$ macrostate.

• ${\mathscr {H}}_{eq}$: Equilibrium macro-space.

• ${\hat{\rho }}_{\text {GC}}, Z_{\text {GC}}$: Generalized canonical density operator and the corresponding partition function.

• $\lambda _k$: Lagrange multipliers (for satisfying the constraints) in the expression for ${\hat{\rho }}_{\text {GC}}$.

• $S_{\text {GvN}}$: The Gibbs-von Neumann entropy of the system

• $\delta , A$: Denote the size and number respectively of the coarse-grained cells in the U-macrostate.

• $\ell$: Labels the coarse-grained cells in the U-macrostate.

• ${\hat{N}}_{\ell }, {\hat{P}}_{\ell }, {\hat{E}}_{\ell }$: Denote the particle number, the momentum, and the energy operators respectively corresponding to the $\ell ^{\text {th}}$ cell in the U-macrostate.

• K: Denotes the coarse-graining scale in the f-macrostate.

• ${|{\psi _{\alpha }}\rangle } \equiv {|{r, v}\rangle }$: The wavepacket basis state localized in position around $x = r L/K$ and in momentum around $p = 2 \pi \hbar v/L$.

• ${\hat{n}}_{\alpha }, D_{\alpha }(t) \equiv D(r, v, t)$: Denote the occupation number operator and the average occupancy (wavepacket density) respectively of the wavepacket basis state ${|{\psi _{\alpha }}\rangle }$.

• ${|{\chi _s}\rangle }, e_s, n_s$: Denote the box energy eigenfunction, eigenvalue, and occupancy of the $s^{\text {th}}$ level respectively.

• ${|{\varphi _n}\rangle }, p_n, \epsilon _n$: Denote the circle energy eigenfunction, momentum, and energy eigenvalue of the $n^{\text {th}}$ level respectively.

• ${\hat{\Psi }}_x, {\hat{b}}_n$: Denote the annihilation operator in the position and in momentum space respectively.

• ${\hat{\rho }}_N$: The N-particle density operator.

• ${\hat{\rho }}_1, \rho _1(x, x'), {\tilde{\rho }}_1 (p_m, p_n)$: The single-particle density operator and its matrix elements in the position and momentum space respectively.

• V: Transformation matrix from the box to the circle basis.

• $f(e_s, \beta , \mu )$: Denotes the Fermi/Bose function.

• ${\hat{\rho }}_1^P, {\hat{\rho }}_1^M$: Denote the single-particle density operator corresponding to the pure and mixed state initial conditions respectively.

• $P(\{ n_s \})$: Denotes the grand canonical probability distribution of the configuration $\{ n_s \}$.

• w(x, p, t): The Wigner function on the real line.

• $q_n$: Denotes the half-integer momenta on the circle.

• $w(x, q_n, t)$: The Wigner function on the circle.

• ${\hat{N}}, {\hat{P}}, {\hat{E}}$: Denote the total particle number, the total momentum, and the total energy operators respectively.

• ${\hat{n}}(x, t), {\hat{p}}(x, t), {\hat{e}}(x, t)$: Denote the local particle, momentum, and energy density operators respectively.

• n(x, t), p(x, t), e(x, t): Denote the expectation values of the local particle, momentum, and energy density operators respectively.

• ${\mathscr {R}}_v$: The set of K integers centered around v.

• $v_{\pm }$: The two end points of the set ${\mathscr {R}}_v$.

• $G_K(q, x)$: The localized kernel that, when integrated over the Wigner function, yields the wavepacket density.

• $D_r(r, t), D_v(v,t)$: The respective marginals of D(r, v, t).

• $h_K(x)$: The localized kernel that, when integrated with the particle density n(x, t), yields the coarse-grained marginal $D_r(r, t)$.

• ${\hat{\rho }}_N^{\star }$: Maximal N-particle density operator subject to the wavepacket density constraints.

• $\lambda _{\alpha }$: Lagrange multipliers (for satisfying the constraints) in the expression for ${\hat{\rho }}_N^{\star }$.

• $\Delta s_B^f, \Delta s_B^U$: The final change in the entropies of f and U macrostates respectively.

• $\rho$: Particle density N/L.

• $\lambda _{\text {th}}$: The thermal De-Broglie wavelength.

• $\tau _p$: The period of oscillations of the particle density.

• $v_{\textrm{f}}$: Fermi velocity.

• $\tau$: The period of oscillations of the Boltzmann entropy $S_B^f$.

• $\tau _{rec}$: The recurrence period.