Skip to main content
SpringerLink
Log in

One-Dimensional Infinite Memory Imitation Models with Noise

  • Published:
Journal of Statistical Physics Aims and scope Submit manuscript

Abstract

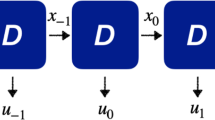
In this paper we study stochastic process indexed by \(\mathbb {Z}\) constructed from certain transition kernels depending on the whole past. These kernels prescribe that, at any time, the current state is selected by looking only at a previous random instant. We characterize uniqueness in terms of simple concepts concerning families of stochastic matrices, generalizing the results previously obtained in De Santis and Piccioni (J Stat Phys 150(6):1017–1029, 2013).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

References

  1. Berbee, H.: Chains with infinite connections: uniqueness and Markov representation. Probab. Theory Relat. Fields 76(2), 243–253 (1987)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  2. Boudiba, M.A.: La chaîne de Feller \(X_{n+1}=\vert X_n-Y_{n+1}\vert \) et les chaînes associées. Ann. Sci. Univ. Clermont-Ferrand II Probab. Appl. 5, 91–132 (1986)

    MathSciNet  Google Scholar 

  3. Bramson, M., Kalikow, S.: Nonuniqueness in \(g\)-functions. Israel J. Math. 84(1–2), 153–160 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  4. Comets, F., Fernández, R., Ferrari, P.A.: Processes with long memory: regenerative construction and perfect simulation. Ann. Appl. Probab. 12(3), 921–943 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  5. De Santis, E., Lissandrelli, A.: Developments in perfect simulation of Gibbs measures through a new result for the extinction of Galton–Watson-like processes. J. Stat. Phys. 147(2), 231–251 (2012)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  6. De Santis, E., Maffei, A.: Perfect simulation for the infinite random cluster model, Ising and Potts models at low or high temperature. Probab. Theory Relat. Fields (2015). doi:10.1007/s00440-014-0608-2

  7. De Santis, E., Piccioni, M.: Exact simulation for discrete time spin systems and unilateral fields. Methodol. Comput. Appl. Probab. 10(1), 105–120 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  8. De Santis, E., Piccioni, M.: Backward coalescence times for perfect simulation of chains with infinite memory. J. Appl. Probab. 49(2), 319–337 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  9. De Santis, E., Piccioni, M.: Perfect simulation of autoregressive models with infinite memory. J. Stat. Phys. 150(6), 1017–1029 (2013)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  10. Feller, W.: An Introduction to Probability Theory and Its Applications, vol. II, 2nd edn. Wiley, New York (1971)

    MATH  Google Scholar 

  11. Fernández, R., Maillard, G.: Chains and specifications. Markov Process. Relat. Fields 10(3), 435–456 (2004)

    MATH  Google Scholar 

  12. Fernández, R., Maillard, G.: Chains with complete connections: general theory, uniqueness, loss of memory and mixing properties. J. Stat. Phys. 118(3–4), 555–588 (2005)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  13. Gallo, S.: Chains with unbounded variable length memory: perfect simulation and a visible regeneration scheme. Adv. Appl. Probab. 43(3), 735–759 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  14. Gallo, S., Garcia, N.L.: Perfect simulation for locally continuous chains of infinite order. Stoch. Process. Appl. 123(11), 3877–3902 (2013)

    Article  MathSciNet  Google Scholar 

  15. Galves, A., Löcherbach, E., Orlandi, E.: Perfect simulation of infinite range Gibbs measures and coupling with their finite range approximations. J. Stat. Phys. 138(1–3), 476–495 (2010)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  16. Georgii, H.-O.: Gibbs Measures and Phase Transitions. de Gruyter Studies in Mathematics, vol. 9. Walter de Gruyter & Co., Berlin (1988)

    Book  Google Scholar 

  17. Grimmett, G.: The random-cluster model. In: Probability on Discrete Structures, vol. 110. Encyclopaedia Mathematical Sciences, pp. 73–12. Springer, Berlin (2004)

  18. Iosifescu, M., Grigorescu, Ş.: Dependence with Complete Connections and Its Applications. Cambridge Tracts in Mathematics. Cambridge University Press, Cambridge (1990)

    Google Scholar 

  19. Johansson, A., Öberg, A.: Square summability of variations of \(g\)-functions and uniqueness of \(g\)-measures. Math. Res. Lett. 10(5–6), 587–601 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  20. Keane, M.: Strongly mixing \(g\)-measures. Invent. Math. 16, 309–324 (1972)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  21. Lalley, S.P.: Regenerative representation for one-dimensional Gibbs states. Ann. Probab. 14(4), 1262–1271 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  22. Lindvall, T.: Lectures on the Coupling Method. Wiley Series in Probability and Mathematical Statistics. Wiley, New York (1992)

    MATH  Google Scholar 

  23. Peigné, M., Woess, W.: Stochastic dynamical systems with weak contractivity properties I. Strong and local contractivity. Colloq. Math. 125(1), 31–54 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  24. Propp, J.G., Wilson, D.B.: Exact sampling with coupled Markov chains and applications to statistical mechanics. Random Struct. Algorithms 9(1–2), 223–252 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  25. Stenflo, Ö.: Uniqueness in \(g\)-measures. Nonlinearity 16(2), 403–410 (2003)

    Article  MathSciNet  ADS  MATH  Google Scholar 

  26. Walters, P.: Ruelle’s operator theorem and \(g\)-measures. Trans. Am. Math. Soc. 214, 375–387 (1975)

    MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Dipartimento di Matematica, Università di Roma La Sapienza, Rome, Italy

    Emilio De Santis & Mauro Piccioni

Authors
  1. Emilio De Santis
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Mauro Piccioni
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Emilio De Santis.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

De Santis, E., Piccioni, M. One-Dimensional Infinite Memory Imitation Models with Noise. J Stat Phys 161, 346–364 (2015). https://doi.org/10.1007/s10955-015-1335-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10955-015-1335-5

Keywords

Mathematics Subject Classification

Search

Navigation