An Analysis of the Transition Zone Between the Various Scaling Regimes in the Small-World Model

Abstract

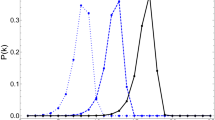
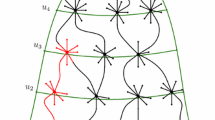
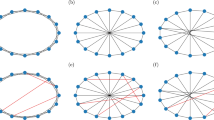
We analyse the so-called small-world network model (originally devised by Strogatz and Watts), treating it, among other things, as a case study of non-linear coupled difference or differential equations. We derive a system of evolution equations containing more of the previously neglected (possibly relevant) non-linear terms. As an exact solution of this entangled system of equations is out of question we develop a (as we think, promising) method of enclosing the “exact” solutions for the expected quantities by upper and lower bounds, which represent solutions of a slightly simpler system of differential equation. Furthermore we discuss the relation between difference and differential equations and scrutinize the limits of the spreading idea for random graphs. We then show that there exists in fact a “broad” (with respect to scaling exponents) crossover zone, smoothly interpolating between linear and logarithmic scaling of the diameter or average distance. We are able to corroborate earlier findings in certain regions of phase or parameter space (as e.g. the finite size scaling ansatz) but find also deviations for other choices of the parameters. Our analysis is supplemented by a variety of numerical calculations, which, among other things, quantify the effect of various approximations being made. With the help of our analytical results we manage to calculate another important network characteristic, the (fractal) dimension, and provide numerical values for the case of the small-world network.