Skip to main content
SpringerLink
Log in

The Compact and Accuracy Preserving Limiter for High-Order Finite Volume Schemes Solving Compressible Flows

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

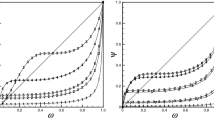
Abstract

In this paper, a new limiter namely the compact weighted biased average procedure (CWBAP) is proposed for the shock capturing of high-order finite volume methods on unstructured girds. The new feature of this limiter is that all its operations are based on the information within a compact stencil. This limiter is an improvement of the original weighted biased average procedure (WBAP) which is not compact due to its successive limiting feature when applied to high-order schemes. This improvement mainly relies on two new techniques: a successive secondary reconstruction and a two-step WBAP limiter. The new limiter can preserve the original order of accuracy of the high-order finite volume schemes when simulating smooth flow without shock waves, and it shows improvements in accuracy, resolution and convergence property over the original WBAP limiter according to the numerical results of a number of test cases. Furthermore, the compactness of this limiter reduces the amount of data transfer in parallel computing. When implemented into the newly developed high-order finite volume schemes based on the compact stencil, the efficiency of the parallel computing can be effectively improved.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

Data Availability

The datasets are available from the corresponding author on reasonable request.

References

  1. Barth, T., Frederickson, P.: Higher-order solution of the Euler equations on unstructured grids using quadratic reconstruction, in: 28th Aerosp. Sci. Meet., American Institute of Aeronautics and Astronautics, 1990.

  2. Delanaye, M., Liu, Y.: Quadratic reconstruction finite volume schemes on 3D arbitrary unstructured polyhedral grids, in: 14th Comput. Fluid Dyn. Conf., American Institute of Aeronautics and Astronautics, 1999.

  3. Ollivier-Gooch, C., Van Altena, M.: A high-order-accurate unstructured mesh finite-volume scheme for the advection-diffusion equation. J. Comput. Phys. 181, 729–752 (2002)

    MATH  Google Scholar 

  4. Friedrich, O.: Weighted essentially non-oscillatory schemes for the interpolation of mean values on unstructured grids. J. Comput. Phys. 144, 194–212 (1998)

    MathSciNet  MATH  Google Scholar 

  5. Hu, C., Shu, C.-W.: Weighted essentially non-oscillatory schemes on triangular meshes. J. Comput. Phys. 150, 97–127 (1999)

    MathSciNet  MATH  Google Scholar 

  6. Dumbser, M., Käser, M., Titarev, V.A., Toro, E.F.: Quadrature-free non-oscillatory finite volume schemes on unstructured meshes for nonlinear hyperbolic systems. J. Comput. Phys. 226, 204–243 (2007)

    MathSciNet  MATH  Google Scholar 

  7. Reed, W.H., Hill, T.: Triangular mesh methods for the neutron transport equation, Los Alamos Report LA-UR-73–479.

  8. Cockburn, B., Shu, C.-W.: TVB Runge-kutta local projection discontinuous galerkin finite element method for conservation laws II: general framework. Math. Comput. 52, 411 (1989)

    MathSciNet  MATH  Google Scholar 

  9. Cockburn, B., Lin, S.-Y., Shu, C.-W.: TVB Runge-Kutta local projection discontinuous Galerkin finite element method for conservation laws III: One-dimensional systems. J. Comput. Phys. 84, 90–113 (1989)

    MathSciNet  MATH  Google Scholar 

  10. Cockburn, B., Hou, S., Shu, C.-W.: The Runge-Kutta local projection discontinuous galerkin finite element method for conservation laws IV: the multidimensional case. Math. Comput. 54, 545 (1990)

    MathSciNet  MATH  Google Scholar 

  11. Cockburn, B., Shu, C.-W.: Runge-Kutta discontinuous Galerkin methods for convection-dominated problems. J. Sci. Comput. 16, 173–261 (2001)

    MathSciNet  MATH  Google Scholar 

  12. Dumbser, M., Balsara, D.S., Toro, E.F., Munz, C.-D.: A unified framework for the construction of one-step finite volume and discontinuous Galerkin schemes on unstructured meshes. J. Comput. Phys. 227, 8209–8253 (2008)

    MathSciNet  MATH  Google Scholar 

  13. Dumbser, M.: Arbitrary high-order PNPM schemes on unstructured meshes for the compressible Navier-Stokes equations. Comput. Fluids. 39, 60–76 (2010)

    MathSciNet  MATH  Google Scholar 

  14. Dumbser, M., Zanotti, O.: Very high-order PNPM schemes on unstructured meshes for the resistive relativistic MHD equations. J. Comput. Phys. 228, 6991–7006 (2009)

    MathSciNet  MATH  Google Scholar 

  15. Wang, Z.J.: Spectral (finite) volume method for conservation laws on unstructured grids. Basic formulation. J. Comput. Phys. 178, 210–251 (2002)

    MathSciNet  MATH  Google Scholar 

  16. Wang, Z.J., Liu, Y.: Spectral (finite) volume method for conservation laws on unstructured grids: II. Extension to two-dimensional scalar equation. J. Comput. Phys. 179, 665–697 (2002)

    MathSciNet  MATH  Google Scholar 

  17. Wang, Z.J., Liu, Y.: Spectral (finite) volume method for conservation laws on unstructured grids III: One dimensional systems and partition optimization. J. Sci. Comput. 20, 137–157 (2004)

    MathSciNet  MATH  Google Scholar 

  18. Wang, Z.J., Zhang, L., Liu, Y.: Spectral (finite) volume method for conservation laws on unstructured grids IV: extension to two-dimensional systems. J. Comput. Phys. 194, 716–741 (2004)

    MathSciNet  MATH  Google Scholar 

  19. Liu, Y., Vinokur, M., Wang, Z.J.: Spectral difference method for unstructured grids I: Basic formulation. J. Comput. Phys. 216, 780–801 (2006)

    MathSciNet  MATH  Google Scholar 

  20. Wang, Z.J., Liu, Y., May, G., Jameson, A.: Spectral difference method for unstructured grids ii: extension to the Euler equations. J. Sci. Comput. 32, 45–71 (2007)

    MathSciNet  MATH  Google Scholar 

  21. May, G., Jameson, A.: A Spectral Difference Method for the Euler and Navier-Stokes Equations on Unstructured Meshes, in: 44th AIAA Aerosp. Sci. Meet. Exhib., American Institute of Aeronautics and Astronautics, 2006.

  22. Huynh, H.T.: A Flux Reconstruction Approach to High-Order Schemes Including Discontinuous Galerkin Methods, in: 18th AIAA Comput. Fluid Dyn. Conf., American Institute of Aeronautics and Astronautics, 2007.

  23. Wang, Z.J., Gao, H.: A unifying lifting collocation penalty formulation including the discontinuous Galerkin, spectral volume/difference methods for conservation laws on mixed grids. J. Comput. Phys. 228, 8161–8186 (2009)

    MathSciNet  MATH  Google Scholar 

  24. van Leer, B.: Towards the ultimate conservative difference scheme. V. A second-order sequel to Godunov’s method. J. Comput. Phys. 32, 101–136 (1979)

    MATH  Google Scholar 

  25. Harten, A.: High resolution schemes for hyperbolic conservation laws. J. Comput. Phys. 49, 357–393 (1983)

    MathSciNet  MATH  Google Scholar 

  26. Harten, A., Engquist, B., Osher, S., Chakravarthy, S.R.: Uniformly high-order accurate essentially non-oscillatory schemes, III. J. Comput. Phys. 71, 231–303 (1987)

    MathSciNet  MATH  Google Scholar 

  27. Jiang, G.-S., Shu, C.-W.: Efficient implementation of weighted ENO schemes. J. Comput. Phys. 126, 202–228 (1996)

    MathSciNet  MATH  Google Scholar 

  28. Liu, X.-D., Osher, S., Chan, T.: Weighted essentially non-oscillatory schemes. J. Comput. Phys. 115, 200–212 (1994)

    MathSciNet  MATH  Google Scholar 

  29. Suresh, A., Huynh, H.T.: Accurate monotonicity-preserving schemes with Runge-Kutta time stepping. J. Comput. Phys. 136, 83–99 (1997)

    MathSciNet  MATH  Google Scholar 

  30. Kim, K.H., Kim, C.: Accurate, efficient and monotonic numerical methods for multi-dimensional compressible flows. J. Comput. Phys. 208, 570–615 (2005)

    MATH  Google Scholar 

  31. Sun, Z., Inaba, S., Xiao, F.: Boundary Variation Diminishing (BVD) reconstruction: a new approach to improve Godunov schemes. J. Comput. Phys. 322, 309–325 (2016)

    MathSciNet  MATH  Google Scholar 

  32. Cheng, L., Deng, X., Xie, B., Jiang, Y., Xiao, F.: Low-dissipation BVD schemes for single and multi-phase compressible flows on unstructured grids. J. Comput. Phys. 428, 110088 (2021)

    MathSciNet  MATH  Google Scholar 

  33. Zhang, X., Shu, C.-W.: On maximum-principle-satisfying high-order schemes for scalar conservation laws. J. Comput. Phys. 229, 3091–3120 (2010)

    MathSciNet  MATH  Google Scholar 

  34. Barth, T., Jespersen, D.: The design and application of upwind schemes on unstructured meshes, in: 27th Aerosp. Sci. Meet., American Institute of Aeronautics and Astronautics, 1989.

  35. Venkatakrishnan, V.: Convergence to steady state solutions of the Euler equations on unstructured grids with limiters. J. Comput. Phys. 118, 120–130 (1995)

    MATH  Google Scholar 

  36. Michalak, C., Ollivier-Gooch, C.: Accuracy preserving limiter for the high-order accurate solution of the Euler equations. J. Comput. Phys. 228, 8693–8711 (2009)

    MathSciNet  MATH  Google Scholar 

  37. Abgrall, R.: On essentially non-oscillatory schemes on unstructured meshes: analysis and implementation. J. Comput. Phys. 114, 45–58 (1994)

    MathSciNet  MATH  Google Scholar 

  38. Park, J.S., Yoon, S.-H., Kim, C.: Multi-dimensional limiting process for hyperbolic conservation laws on unstructured grids. J. Comput. Phys. 229, 788–812 (2010)

    MathSciNet  MATH  Google Scholar 

  39. Park, J.S., Kim, C.: Higher-order multi-dimensional limiting strategy for discontinuous Galerkin methods in compressible inviscid and viscous flows. Comput. Fluids. 96, 377–396 (2014)

    MathSciNet  MATH  Google Scholar 

  40. Zhu, J., Qiu, J.: Hermite WENO schemes and their application as limiters for Runge-Kutta discontinuous galerkin method, iii: unstructured meshes. J. Sci. Comput. 39, 293–321 (2009)

    MathSciNet  MATH  Google Scholar 

  41. Luo, H., Baum, J.D., Löhner, R.: A Hermite WENO-based limiter for discontinuous Galerkin method on unstructured grids. J. Comput. Phys. 225, 686–713 (2007)

    MathSciNet  MATH  Google Scholar 

  42. Krivodonova, L.: Limiters for high-order discontinuous Galerkin methods. J. Comput. Phys. 226, 879–896 (2007)

    MathSciNet  MATH  Google Scholar 

  43. Wang, Q., Ren, Y.-X., Li, W.: Compact high-order finite volume method on unstructured grids I: basic formulations and one-dimensional schemes. J. Comput. Phys. 314, 863–882 (2016)

    MathSciNet  MATH  Google Scholar 

  44. Wang, Q., Ren, Y.-X., Li, W.: Compact high-order finite volume method on unstructured grids II: extension to two-dimensional Euler equations. J. Comput. Phys. 314, 883–908 (2016)

    MathSciNet  MATH  Google Scholar 

  45. Wang, Q., Ren, Y.-X., Pan, J., Li, W.: Compact high-order finite volume method on unstructured grids III: Variational reconstruction. J. Comput. Phys. 337, 1–26 (2017)

    MathSciNet  MATH  Google Scholar 

  46. Zhong, X., Shu, C.-W.: A simple weighted essentially nonoscillatory limiter for Runge-Kutta discontinuous Galerkin methods. J. Comput. Phys. 232, 397–415 (2013)

    MathSciNet  Google Scholar 

  47. Zhu, J., Zhong, X., Shu, C.-W., Qiu, J.: Runge-Kutta discontinuous Galerkin method using a new type of WENO limiters on unstructured meshes. J. Comput. Phys. 248, 200–220 (2013)

    MathSciNet  MATH  Google Scholar 

  48. Zhu, J., Zhong, X., Shu, C.-W., Qiu, J.: Runge-Kutta discontinuous galerkin method with a simple and compact hermite WENO limiter, commun. Comput. Phys. 19, 944–969 (2016)

    MathSciNet  MATH  Google Scholar 

  49. Zhu, J., Zhong, X., Shu, C.-W., Qiu, J.: Runge-Kutta discontinuous galerkin method with a simple and compact hermite WENO limiter on unstructured meshes, commun. Comput. Phys. 21, 623–649 (2017)

    MathSciNet  MATH  Google Scholar 

  50. Zhu, H., Qiu, J., Zhu, J.: A simple, high-order and compact WENO limiter for RKDG method. Comput. Math. with Appl. 79, 317–336 (2020)

    MathSciNet  MATH  Google Scholar 

  51. Dumbser, M., Zanotti, O., Loubère, R., Diot, S.: A posteriori subcell limiting of the discontinuous Galerkin finite element method for hyperbolic conservation laws. J. Comput. Phys. 278, 47–75 (2014)

    MathSciNet  MATH  Google Scholar 

  52. Vuik, M.J., Ryan, J.K.: Multiwavelet troubled-cell indicator for discontinuity detection of discontinuous Galerkin schemes. J. Comput. Phys. 270, 138–160 (2014)

    MathSciNet  MATH  Google Scholar 

  53. Vuik, M.J., Ryan, J.K.: Automated parameters for troubled-cell indicators using outlier detection. SIAM J. Sci. Comput. 38, A84–A104 (2016)

    MathSciNet  MATH  Google Scholar 

  54. Fu, G., Shu, C.-W.: A new troubled-cell indicator for discontinuous Galerkin methods for hyperbolic conservation laws. J. Comput. Phys. 347, 305–327 (2017)

    MathSciNet  MATH  Google Scholar 

  55. Li, W., Ren, Y.-X., Lei, G., Luo, H.: The multi-dimensional limiters for solving hyperbolic conservation laws on unstructured grids. J. Comput. Phys. 230, 7775–7795 (2011)

    MathSciNet  MATH  Google Scholar 

  56. Li, W., Ren, Y.-X.: The multi-dimensional limiters for solving hyperbolic conservation laws on unstructured grids II: Extension to high-order finite volume schemes. J. Comput. Phys. 231, 4053–4077 (2012)

    MathSciNet  MATH  Google Scholar 

  57. Li, W., Ren, Y.-X.: High-order k -exact WENO finite volume schemes for solving gas dynamic Euler equations on unstructured grids. Int. J. Numer. Methods Fluids. 70, 742–763 (2012)

    MathSciNet  MATH  Google Scholar 

  58. Roe, P.L.: Approximate Riemann solvers, parameter vectors, and difference schemes. J. Comput. Phys. 43, 357–372 (1981)

    MathSciNet  MATH  Google Scholar 

  59. Sanders, R., Morano, E., Druguet, M.-C.: Multidimensional dissipation for upwind schemes: stability and applications to gas dynamics. J. Comput. Phys. 145, 511–537 (1998)

    MathSciNet  MATH  Google Scholar 

  60. Ferracina, L., Spijker, M.N.: Strong stability of singly-diagonally-implicit Runge-Kutta methods. Appl. Numer. Math. 58, 1675–1686 (2008)

    MathSciNet  MATH  Google Scholar 

  61. Luo, H., Baum, J.D., Löhner, R.: A discontinuous Galerkin method based on a Taylor basis for the compressible flows on arbitrary grids. J. Comput. Phys. 227, 8875–8893 (2008)

    MathSciNet  MATH  Google Scholar 

  62. Li, W., Ren, Y.-X.: The multi-dimensional limiters for discontinuous Galerkin method on unstructured grids. Comput. Fluids. 96, 368–376 (2014)

    MathSciNet  MATH  Google Scholar 

  63. Sod, G.A.: A survey of several finite difference methods for systems of nonlinear hyperbolic conservation laws. J. Comput. Phys. 27, 1–31 (1978)

    MathSciNet  MATH  Google Scholar 

  64. Lax, P.D.: Weak solutions of nonlinear hyperbolic equations and their numerical computation. Commun. Pure Appl. Math. 7, 159–193 (1954)

    MathSciNet  MATH  Google Scholar 

  65. Woodward, P., Colella, P.: The numerical simulation of two-dimensional fluid flow with strong shocks. J. Comput. Phys. 54, 115–173 (1984)

    MathSciNet  MATH  Google Scholar 

  66. Park, S.-H., Kwon, J.: An improved HLLE method for hypersonic viscous flows, in: 15th AIAA Comput. Fluid Dyn. Conference American Institute of Aeronautics and Astronautics, 2001.

  67. Lax, P.D., Liu, X.-D.: Solution of two-dimensional Riemann problems of gas dynamics by positive schemes. SIAM J. Sci. Comput. 19, 319–340 (1998)

    MathSciNet  MATH  Google Scholar 

  68. Zingg, D.W., De Rango, S., Nemec, M., Pulliam, T.H.: Comparison of several spatial discretizations for the navier-stokes equations. J. Comput. Phys. 160, 683–704 (2000)

    MATH  Google Scholar 

Download references

Acknowledgements

This work was supported by National Natural Science Foundation of China (92152201) and the national numerical wind tunnel project.

Author information

Authors and Affiliations

  1. Department of Engineering Mechanics, AML, Tsinghua University, Beijing, 100084, China

    Zhuohang Wu & Yu-xin Ren

Authors
  1. Zhuohang Wu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yu-xin Ren
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

All authors contributed to the study conception and numerical method. The computation is carried out by ZW. The first draft of the manuscript was written by ZW, which was revised by Y-xR. All authors read and approved the final manuscript.”

Corresponding author

Correspondence to Yu-xin Ren.

Ethics declarations

Conflict of interest

The authors have no relevant financial or non-financial interests to disclose.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wu, Z., Ren, Yx. The Compact and Accuracy Preserving Limiter for High-Order Finite Volume Schemes Solving Compressible Flows. J Sci Comput 96, 77 (2023). https://doi.org/10.1007/s10915-023-02298-z

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-023-02298-z

Keywords

Search

Navigation