Hubble diagrams in the Jordan and Einstein frames

Abstract

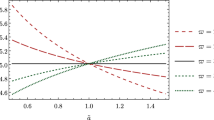
Different models in cosmology generally predict different Hubble diagrams. Then, the comparison between the Hubble diagrams may be used as a way for distinguishing between different cosmological scenarios. But that is not always the case because there is no guarantee that two different models always have different Hubble diagrams. It may be possible for two physically-inequivalent models to have the same Hubble diagrams. In that case, the Hubble diagram cannot be used to differentiate between two models and it is necessary to find another way to distinguish between them. Therefore, the question of whether two different scenarios are distinguishable by using the Hubble diagrams is an important question which would not have an obvious answer. The Jordan and Einstein frames of f(R) theories of gravity are inequivalent, provided that the metricity condition holds in both frames. In the present paper it is argued that if the time-variation of particle masses in the Einstein frame is taken into consideration, the Hubble diagram derived practically from type Ia supernova surveys does not enable us to differentiate between these two frames. Nevertheless, we show that by waiting long enough to measure the change in Hubble diagram it is possible to differentiate between two frames. In other words, the Hubble diagram cannot be employed alone to differentiate between two frames but comparison between the rates of changes in Hubble diagrams can provide a way to do so.

Appendix

In this section we propose two practical methods to discern between inertial and freely-falling observers in the Einstein frame.

A simple experiment is similar to the twin paradox. Consider two spaceships. Assume one of them always keeps its own engine off and the other one turns on its engine and takes a large number of trips and each time comes back to the first. The first spaceship is a freely-falling observer because it is not acted on by a well-known non-gravitational forces, but the second one cannot be a freely-falling observer because its engine exerts a non-gravitational force on it. If for each trip the time measured by the first spaceship is longer than the time of the travelling spaceship, this freely-falling observer is an inertial observer because its world-line has the extremum length. But, if there is a trip in which the time measured by the second spaceship is longer than the first, the freely-falling spaceship cannot be an inertial observer. It can only occur in the Einstein frame and not in the Jordan frame.

Besides the above example, the following argument shows that the Universality of Free Fall can be violated for freely-falling observers in the Einstein frame. The Universality of Free Fall asserts that the relative accelerations of two freely-falling particles located at the same point always vanish independent of their masses and their relative velocities. Obviously, in General Relativity and also in the Jordan frame for freely-falling observers which follow geodesics the Universality of Free Fall and local Lorentz invariance are satisfied. We will argue that in the Einstein frame the relative accelerations of two freely-falling particles located at the same point can take non-vanishing values depending on their relative velocities. Therefore, the test of The Universality of Free Fall can reveal in which frames we are living. Equation (16) can be expressed as

$$\begin{aligned} {\tilde{u}}^{\mu }{\tilde{\nabla }}_{\mu }({\tilde{u}}_{\nu })= -\frac{\partial _{\nu }{\tilde{m}}}{{\tilde{m}}}-\frac{\partial _{\mu }{\tilde{m}}}{{\tilde{m}}}{\tilde{u}}^{\mu }{\tilde{u}}_{\nu }\equiv a_{\nu }. \end{aligned}$$

(58)

Contracting the left hand side with ${\tilde{u}}^{\nu }$ identically yields zero, i.e. ${\tilde{u}}^{\nu }a_{\nu }=0$. It is then possible to employ the Fermi–Walker (or Fermi normal) coordinates near each curve that satisfies the above equation [75]. We use $x^{\mu }$ to denote the Fermi-Walker coordinates near the world-line of a freely-falling observer. Then the world-line of the observer can be described by $d x^{0}=dt$ and $x^{i}=0$, where dt is the proper time along the world-line and $i=1,2,3$. In these coordinates, the metric near the world-line can be expressed as [75]

$$\begin{aligned} {\tilde{g}}_{00}= & {} -(1+2a_{i}(t)x^{i}+(a_{i}(t)x^{i})^2+{\tilde{R}}_{0i0j}(t)x^{i}x^{j}+{\mathcal {O}}(3)),\nonumber \\ {\tilde{g}}_{0i}= & {} -\frac{2}{3}{\tilde{R}}_{0jik}(t)x^{j}x^{k}+{\mathcal {O}}(3),\nonumber \\ {\tilde{g}}_{ij}= & {} \delta _{ij}-\frac{1}{3}{\tilde{R}}_{ikjm}(t)x^{k}x^{m}+{\mathcal {O}}(3), \end{aligned}$$

(59)

where $a_{i}(t)$ are the spatial components of $a_{\nu }$ on the world-line and ${\tilde{R}}_{\mu \nu \alpha \beta }(t)$ are the components of the Riemann tensor evaluated again on the world-line. It is no difficult to see that the metric reduces to ${\tilde{g}}_{00}=-1$ and ${\tilde{g}}_{ij}=\delta _{ij}$ on the world-line and the non-vanishing Christoffel symbols on the world-line are ${\tilde{\Gamma }}^{0}_{0i}={\tilde{\Gamma }}^{i}_{00}=a_{i}(t)$. Since the four-velocity ${\tilde{u}}^{\mu }$ on the world-line is (1, 0, 0, 0) we get

$$\begin{aligned} a_{i}(t)=-\frac{\partial _{i}{\tilde{m}}(x^0=t,x^i=0)}{{\tilde{m}}(x^0=t,x^i=0)}. \end{aligned}$$

(60)

Now consider another freely-falling test particle whose velocity relative to our observer does not vanish when its world-line intersects the world-line of the observer. Near the observer the world-line of this particle can be described by the Fermi-Walker coordinates as $x^{\mu }(s)$, where s is the proper time along the particle world-line. The acceleration of the particle with respect to the observer at the intersection point is

$$\begin{aligned} A^{i}\equiv & {} \frac{d^2 x^i(s)}{(d x^0(s))^2}=\frac{d^2 x^i(s)}{d t^2}\nonumber \\= & {} -\left( \frac{d s}{d t}\right) ^2\frac{d^2t}{d s^2}\frac{d x^i}{d t}+\left( \frac{d s}{d t}\right) ^2\frac{d^2 x^i}{d s^2}. \end{aligned}$$

(61)

Assuming that the velocity of the particle relative to the observer is $\frac{d x^i}{d t}=v^i$ at the intersection point, we have

$$\begin{aligned} \left( \frac{d s}{d t}\right) ^2=\left( \frac{d s}{d x^0}\right) ^2=1-v^2, \end{aligned}$$

(62)

where $v^2=v^iv_{i}$. But, the world-line of the particle must also satisfy the equation of motion (58). Since the intersection point is also on the observer world-line and on this world-line the only non-vanishing Christoffel symbols are ${\tilde{\Gamma }}^{0}_{0i}={\tilde{\Gamma }}^{i}_{00}=a_{i}(t)$, Eq. (58) yields

$$\begin{aligned} \frac{d^2 t}{d s^2}=\frac{d^2 x^0}{d s^2}=- & {} 2a_{j}\frac{d x^0}{d s}\frac{d x^j}{d s}-\frac{d x^0}{d s}\frac{d x^{\mu }}{d s}\frac{\partial _{\mu } {\tilde{m}}}{{\tilde{m}}}\nonumber \\- & {} \frac{\partial ^0 {\tilde{m}}}{{\tilde{m}}}, \end{aligned}$$

(63)

and

$$\begin{aligned} \frac{d^2 x^i}{d s^2}=-a^i \left( \frac{d x^0}{d s}\right) ^2-\frac{d x^i}{d s}\frac{d x^{\mu }}{d s}\frac{\partial _{\mu } {\tilde{m}}}{{\tilde{m}}}-\frac{\partial ^i {\tilde{m}}}{{\tilde{m}}}. \end{aligned}$$

(64)

Taking Eqs. (60) and (62) into account, one can obtain

$$\begin{aligned} \frac{d^2 t}{d s^2}=-\frac{v^2}{1-v^2}\frac{\partial _{0} {\tilde{m}}}{{\tilde{m}}}+\frac{v^j}{1-v^2}\frac{\partial _{j} {\tilde{m}}}{{\tilde{m}}} , \end{aligned}$$

(65)

and

$$\begin{aligned} \frac{d^2 x^i}{d s^2}=-\frac{v^i}{1-v^2}\frac{\partial _{0} {\tilde{m}}}{{\tilde{m}}}+\left( \frac{v^2}{1-v^2}\delta ^{ij}-\frac{v^iv^j}{1-v^2}\right) \frac{\partial _{j} {\tilde{m}}}{{\tilde{m}}} . \end{aligned}$$

(66)

Substituting the relations (62), (65) and (66) in Eq. (61), we find

$$\begin{aligned} A^i=-(1-v^2)v^i\frac{\partial _{0} {\tilde{m}}}{{\tilde{m}}}+(v^2\delta ^{ij}-2v^i v^j)\frac{\partial _{j} {\tilde{m}}}{{\tilde{m}}}. \end{aligned}$$

(67)

It is not difficult to see that not only the acceleration of particle with respect to the observer does not vanish, but also freely-falling test particles, with different velocities relative to the freely-falling observer, have different accelerations with respect to the observer. It means that the Universality of Free Fall is violated for freely-falling observers in the Einstein frame; whereas, in the Jordan frame in contrast to the Einstein frame, the acceleration of all freely-falling particles is always zero with respect to a freely-falling observer.