Heisenberg Uncertainty Relations as Statistical Invariants

Surely, one would like to be able to deduce the quantitative laws of quantum mechanics directly from their anschaulich foundations, that is, essentially, relation ${\delta }p\,{\delta }q \thicksim h$. (Werner Heisenberg [8], p. 196)

Abstract

For a simple set of observables we can express, in terms of transition probabilities alone, the Heisenberg uncertainty relations, so that they are proven to be not only necessary, but sufficient too, in order for the given observables to admit a quantum model. Furthermore distinguished characterizations of strictly complex and real quantum models, with some ancillary results, are presented and discussed.

Appendix

1. Results quoted from [1].

Theorem 7

The following assertions are equivalent:

• (i) the transition matrices P, Q, R admit a complex Hilbert space model;

• (ii) the transition matrices P, Q, R admit a spin model;

• (iii) $\cos ^2\alpha +\cos ^2\beta +\cos ^2\gamma -1\le 2\;\cos \alpha \;\cos \beta \;\cos \gamma ;$

• (iv) $-1\le \frac{\cos ^2\frac{\alpha }{2}+\cos ^2\frac{\beta }{2}+\cos ^2\frac{\gamma }{2}\;-\;1}{2\cos \frac{\alpha }{2}\cos \frac{\beta }{2}\cos \frac{\gamma }{2}}\le 1;$

• (v) $-1\;\le \;\frac{p\;+\;q\;+\;r\;-\;1}{2\sqrt{p\;q\;r}}\;\le \;1;$

• (vi) $[\sqrt{pq}-\sqrt{(1-p)(1-q)}]^2\;\le \;r\;\le \;[\sqrt{pq}+\sqrt{(1-p)(1-q)}]^2$

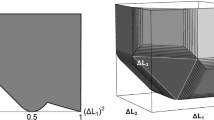
Proposition 3

Three vectors $a,b,c\in S^{(2)}$ satisfying

$$\begin{aligned} \cos \alpha =\cos \widehat{ab},\;\cos \beta =\cos \widehat{bc},\;\cos \gamma =\cos \widehat{ca} \end{aligned}$$

exist if and only if

$$\begin{aligned} \cos ^2\alpha +\cos ^2\beta +\cos ^2\gamma -1\le 2\;\cos \alpha \;\cos \beta \;\cos \gamma . \end{aligned}$$

Theorem 9

The transition matrices P, Q, R admit a real Hilbert space model if and only if $\frac{p\;+\;q\;+\;r\;-\;1}{2\sqrt{p\;q\;r}}\;=\;+1$ or $\frac{p\;+\;q\;+\;r\;-\;1}{2\sqrt{p\;q\;r}}\;=\;-1$ or equivalently $\sqrt{r}\;=\;\sqrt{pq}+\sqrt{(1-p)(1-q)}$ or $\sqrt{r}\;=\;\left| \sqrt{pq}-\sqrt{(1-p)(1-q)}\right|$.

Theorem 10

The transition matrices P, Q, R admit a real Hilbert space model if and only if they admit a spin model defined by a coplanar triple of vectors in $S^{(2)}$.

2. Proof of Lemma 4.1

Putting for simplicity $z_{+\ }:=\frac{z_1+z_2}{2}$ and $z_{-\ }:=\frac{z_1-z_2}{2},$ by equation (10) we get

$$\begin{aligned} \langle {\hat{Z}}\rangle =z_{+\ }+z_{-\ }\langle u_Z\cdot \sigma \rangle \end{aligned}$$

and, since $\langle (u_Z\cdot \sigma )^2\rangle =\langle {\hat{1}}\rangle =1,$

$$\begin{aligned} \langle {\hat{Z}}^2\rangle =(z^2)_{+\ }+2z_{+\ }z_{-\ }\langle u_Z\cdot \sigma \rangle , \end{aligned}$$

from which we obtain

$$\begin{aligned} Var ({\hat{Z}})=z^2_{-{\ }} Var (u_Z\cdot \sigma ). \end{aligned}$$

Further, with easily understood notations, we have

$$\begin{aligned} \frac{1}{2}\langle \{{\hat{X}},{\hat{Y}}\}\rangle \;=\;x_{+\ }y_{+\ }+\;x_{-\ }y_{+\ }\langle u_X\cdot \sigma \rangle \;+\;x_{+\ }y_{-\ }\langle u_Y\cdot \sigma \rangle \;+\;\frac{1}{2}\langle \{u_X\cdot \sigma ,u_Y\cdot \sigma \}\rangle , \end{aligned}$$

so that

$$\begin{aligned} \frac{1}{2}\langle \{{\hat{X}},{\hat{Y}}\}\rangle -\langle {\hat{X}}\rangle \langle {\hat{Y}}\rangle \;=\;x_{-\ }y_{-\ }\left( \frac{1}{2}\langle \{u_X\cdot \sigma ,u_Y\cdot \sigma \}\rangle -\langle u_X\cdot \sigma \rangle \langle u_Y\cdot \sigma \rangle \right) ; \end{aligned}$$

last, quite directly, we get

$$\begin{aligned} \frac{1}{2i}\langle [{\hat{X}},{\hat{Y}}]\rangle \;=\;x_{-\ }y_{-\ }\frac{1}{2i}\langle [u_X\cdot \sigma ,u_Y\cdot \sigma ]\rangle . \end{aligned}$$

$\square$

3. Proof of Lemma 4.2

By definition $u\cdot \sigma =\left[ \begin{matrix}u_3&{}u_1-iu_2\\ u_1+iu_2&{}-u_3\end{matrix}\right] ,$ so that for every state $\psi$ one has

$$\begin{aligned} \langle u\cdot \sigma \rangle= & {} \langle (u\cdot \sigma )\psi |\psi \rangle =[\overline{\psi _1}\;\;\;\overline{\psi _2}][u_3\psi _1+(u_1-iu_2)\psi _2\;\;\;\;\;(u_1+iu_2)\psi _1-u_3\psi _2]^T\\= & {} 2u_1\mathfrak {R}(\overline{\psi _1}\psi _2)+2u_2\mathfrak {I}(\overline{\psi _1}\psi _2)+u_3(\left| \psi _1\right| ^2-\left| \psi _2\right| ^{2.}). \end{aligned}$$

Since¹⁷ as known $\psi _1(w)=\left[ \begin{array}{ll}\sqrt{\frac{1+w_3}{2}}&{}\frac{w_1+i\;w_2}{\sqrt{2(1+w_3)}}\\ \end{array}\right] ^T$ and $\psi _2(w)=\left[ \begin{array}{ll}\sqrt{\frac{1-w_3}{2}}&{}-\frac{w_1+i\;w_2}{\sqrt{2(1-w_3)}}\\ \end{array}\right] ^T,$ putting them in the former formula, with easy calculations we get $\langle u\cdot \sigma \rangle _{\psi _1(w)}=u\cdot w$ and $\langle u\cdot \sigma \rangle _{\psi _2(w)}=-u\cdot w$ as asserted in Eq. (19). Further it is soon seen that $(u\cdot \sigma )^2={\hat{1}},$ so that $\langle (u\cdot \sigma )^2\rangle =1,$ thus, in the said states, $Var (u\cdot \sigma )=\langle u\cdot \sigma \rangle ^2-(\langle u\cdot \sigma \rangle )^2=1-(u\cdot w)^2$ as stated in Eq. (20), that therefore is proven. Further, due to¹⁸ $[\sigma _j,\sigma _k]=2i\varepsilon _{ jkl }\sigma _l$ for every j, k,  one has $\frac{1}{2i}[u\cdot \sigma ,v\cdot \sigma ]= Det \left[ \begin{matrix}\sigma _1&{}\sigma _2&{}\sigma _3\\ u_1&{}u_2&{}u_3\\ v_1&{}v_2&{}v_3\end{matrix}\right] =(u\times v)\cdot \sigma ,$ so that, taking the averages in the states $\psi _k(w)$ and considering that $\langle \sigma _k\rangle =w_k$ for $k=1,2,3$, (21) is proven. Lastly, due to $\{\sigma _h,\sigma _k\}=2\delta _{ hk }{\hat{1}}$ for every h, k,  we have $\{\sigma \cdot u,\sigma \cdot v\}=2u\cdot v{\hat{1}}$ so that, taking the averages in whichever state, we get Eq. (22) and the proof of the lemma is complete. $\square$

4. With the notations of Sect. 3, thanks to the trigonometric identity $\cos \theta =2\cos ^2\frac{\theta }{2}-1,$ we can write $\cos \alpha =2p-1,\;\cos \beta =2q-1,\;\cos \gamma =2r-1,$ so that

$$\begin{aligned}&1-\cos ^2\alpha -\cos ^2\beta -\cos ^2\gamma +2\;\cos \alpha \;\cos \beta \;\cos \gamma \\&\quad =1-(2p-1)^2-(2q-1)^2-(2r-1)^2+2(2p-1)(2q-1)(2r-1) \end{aligned}$$

that suitably simplified becomes $4(4pqr-(p+q+r-1)^2)$ as asserted. $\square$