Mechanical and Optical Properties of Anisotropic Single-Crystal Prisms

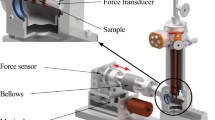
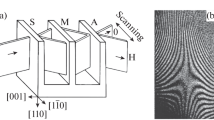
Abstract

The great interest in scintillating crystals, is related to their applications in the high energy physics, biomedicine and security. For this reason a complete characterization and understanding of their structural, optical and mechanical properties at the macroscopic level is necessary. We must give a complete theoretical characterization of the mechanics and optics of bulk prismatic single-crystal bodies in order to design experiments. This work shall deal solely with the theoretical description within the framework of linear theories.

Appendix

In this appendix we show how we obtained the linear relations (52) and (54) between the angles δ, φ and the applied stress T ₃₃.

We begin with (52): by using (46) into (47):

$$\tan\delta=\frac{B_{12}}{B_{1}-B_{11}}=\frac {2B_{12}}{B_{22}-B_{11}+\sqrt{(B_{22}-B_{11})^{2}+4B_{12}^{2}}}; $$

then, since the components of B depends on T ₃₃, by setting:

$$u(T_{33})=\frac{2B_{12}}{B_{22}-B_{11}+\sqrt {(B_{22}-B_{11})^{2}+4B_{12}^{2}}}, $$

we arrive at the non linear relation:

$$ \delta(T_{33})=\tan^{-1}\bigl(u(T_{33}) \bigr). $$

(85)

We take the Taylor expansion of (85) about T ₃₃=0:

$$\delta(T_{33})=\delta(0)+\delta'(0)T_{33}+o \bigl(T_{33}^{2}\bigr),\qquad(\cdot )'= \frac{d}{dT_{33}}, $$

where δ(0)=δ ₀, the value of the angle in the unstressed state and:

$$\delta'=\frac{1}{1+u^{2}}u'. $$

If we set:

$$v(T_{33})=2B_{12},\qquad w(T_{33})=B_{22}-B_{11} , $$

we can rewrite u as follows:

$$u=\frac{v}{w+\sqrt{v^{2}+w^{2}}}, $$

and accordingly:

$$u'=\biggl(\frac{v}{w+\sqrt{v^{2}+w^{2}}}\biggr)'= \frac{v'(w+\sqrt {v^{2}+w^{2}})-v\bigl(w'+{\frac{vv'+ww'}{\sqrt {v^{2}+w^{2}}}}\bigr)}{(w+\sqrt{v^{2}+w^{2}})^{2}}. $$

Since for T ₃₃=0 we have:

$$u=\tan\delta_{0},\qquad v=2B^{0}_{12},\qquad w=B^{0}_{22}-B^{0}_{11}, $$

and

$$v'=\mathbb {M}_{1233},\qquad w'=\mathbb {M}_{2233}- \mathbb {M}_{1133}, $$

then we have:

$$\frac{1}{1+u^{2}}(0)=\frac{1}{1+\tan^{2}\delta_{0}}=\frac{\cos^{2}\delta _{0}}{\sin^{2}\delta_{0}+\cos^{2}\delta_{0}}=\cos^{2} \delta_{0}, $$

and:

$$u'(0)=\frac{\mathbb {M}_{1233}(w+\sqrt{v^{2}+w^{2}})-v\bigl(\mathbb {M}_{2233}-\mathbb {M}_{1133}+{\frac{v\mathbb {M}_{1233}+w(\mathbb {M}_{2233}-\mathbb {M}_{1133})}{\sqrt{v^{2}+w^{2}}}}\bigr)}{(w+\sqrt{v^{2}+w^{2}})^{2}}. $$

By using again (46) and (47) we finally arrive at:

$$M=\delta'(0)=\frac{2\cos^{2}\delta_{0}}{B^{0}_{1}-B^{0}_{11}}\biggl(\mathbb {M}_{1233}+\tan \delta_{0}\frac{(\mathbb {M}_{2233}-\mathbb {M}_{1133})(B^{0}_{1}-B^{0}_{11})+4B^{0}_{12}\mathbb {M}_{1233}}{B^{0}_{1}-B^{0}_{2}}\biggr). $$

For (54) we start from (48):

$$ \varphi(T_{33})=\sin^{-1}\sqrt{ \frac{B_{2}-B_{3}}{B_{1}-B_{3}}}, $$

(86)

where the eigenvalues are given by:

$$\begin{aligned} B_{1} =&\frac{1}{2}\bigl(B^{0}_{11}+B^{0}_{22}+( \mathbb {M}_{1133}+\mathbb {M}_{2233})T_{33}\bigr) \\ &{}-\sqrt{\biggl(\frac{B^{0}_{11}-B^{0}_{22}+(\mathbb {M}_{1133}+\mathbb {M}_{2233})T_{33}}{2}\biggr)^{2}+ \bigl(B_{12}^{0}+\mathbb {M}_{1233}T_{33} \bigr)^{2}}, \\ B_{2} =&\frac{1}{2}\bigl(B^{0}_{11}+B^{0}_{22}+( \mathbb {M}_{1133}+\mathbb {M}_{2233})T_{33}\bigr) \\ &{}+\sqrt{\biggl(\frac{B^{0}_{11}-B^{0}_{22}+(\mathbb {M}_{1133}+\mathbb {M}_{2233})T_{33}}{2}\biggr)^{2}+ \bigl(B_{12}^{0}+\mathbb {M}_{1233}T_{33} \bigr)^{2}}, \\ B_{3} =&B^{0}_{33}+\mathbb {M}_{3333}T_{33} , \end{aligned}$$

and we can set:

$$\begin{aligned} u(T_{33}) =&\frac{1}{2}\bigl(B^{0}_{11}+B^{0}_{22}-2B_{33}+( \mathbb {M}_{1133}+\mathbb {M}_{2233}-2\mathbb {M}_{3333})T_{33} \bigr), \\ w(T_{33}) =&\biggl(\frac{B^{0}_{11}-B^{0}_{22}+(\mathbb {M}_{1133}+\mathbb {M}_{2233})T_{33}}{2}\biggr)^{2}+ \bigl(B_{12}^{0}+\mathbb {M}_{1233}T_{33} \bigr)^{2}, \end{aligned}$$

to write (86) as:

$$ \varphi(T_{33})=\sin^{-1}\sqrt{ \frac{u+\sqrt{w}}{u-\sqrt{w}}}. $$

(87)

By linearizing (87) about T ₃₃=0 we get

$$\varphi(T_{33})=\varphi(0)+\varphi'(0)T_{33}+o \bigl(T_{33}^{2}\bigr), $$

where φ(0)=φ ₀ the angle at the unstressed state and where by the chain rule:

$$ \varphi'(T_{33})=\sqrt{\frac{u+\sqrt{w}}{\sqrt{w}}} \frac{w'u-u'w}{2\sqrt{w}(u-\sqrt{w})^{2}}\sqrt{\frac{u-\sqrt{w}}{u+\sqrt {w}}}. $$

(88)

By evaluating (88) for T ₃₃=0 we get the (54) with:

$$ K=\frac{(B^{0}_{11}-B^{0}_{22})(\mathbb {M}_{1133}-\mathbb {M}_{2233})+B^{0}_{12}\mathbb {M}_{1233}}{B^{0}_{1}+B^{0}_{2}-2B^{0}_{3}}. $$

(89)

A similar analysis can be done for the other two cases to obtain:

$$\begin{aligned} K =&\bigl(2B^{0}_{3}-B^{0}_{1}-B^{0}_{2} \bigr)\frac{((B^{0}_{11}-B^{0}_{22})(\mathbb {M}_{1133}-\mathbb {M}_{2233})+4B^{0}_{12}\mathbb {M}_{1233})}{(2B^{0}_{3}+B^{0}_{1}-3B^{0}_{2})(B^{0}_{1}-B^{0}_{2})} \\ &{}+\frac{2(B^{0}_{1}-B^{0}_{2})(\mathbb {M}_{1133}+\mathbb {M}_{2233}-2\mathbb {M}_{3333})}{ 2B^{0}_{3}+B^{0}_{1}-3B^{0}_{2}}, \end{aligned}$$

(90)

when B ₃>B ₁>B ₂ and

$$K=\hat{K}\sin^{4}\varphi_{0} $$

when B ₁>B ₃>B ₂, where $\hat{K}$ is given by (90).