A fractional Adams–Simpson-type method for nonlinear fractional ordinary differential equations with non-smooth data

Abstract

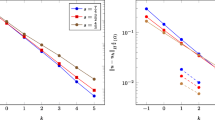
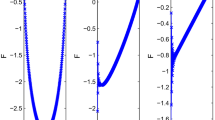
We propose a fractional Adams–Simpson-type method for nonlinear fractional ordinary differential equations with fractional order $\alpha \in (0,1)$. In our method, a nonuniform mesh is used so that the optimal convergence order can be recovered for non-smooth data. By developing a modified fractional Grönwall inequality, we prove that the method is unconditionally convergent under the local Lipschitz condition of the nonlinear term, and show that with a proper mesh parameter, the method can achieve the optimal convergence order $3+\alpha$ even if the given data is not smooth. Under very mild conditions, the nonlinear stability of the method is analyzed by using a perturbation technique. The extensions of the method to multi-term nonlinear fractional ordinary differential equations and multi-order nonlinear fractional ordinary differential systems are also discussed. Numerical results confirm the theoretical analysis results and demonstrate the effectiveness of the method for non-smooth data.

A Appendix

In this appendix, we prove Lemma 4.1.

Proof

It is clear that

$$\begin{aligned} g(t)-g(t_{0})=\int _{t_{0}}^{t} g^{\prime }(\xi )\, {\textrm{d}}\xi = t\int _{0}^{1} g^{\prime }(st)\, {\textrm{d}}s, \qquad t>0. \end{aligned}$$

(A.1)

This proves

$$\begin{aligned} R_{0,0}g(t)= t\int _{0}^{1} g^{\prime }(st)\, {\textrm{d}}s, \qquad t>0, \end{aligned}$$

(A.2)

and so (4.5) is proved. By Taylor expansion with integral remainder,

$$\begin{aligned} g(t_{k})= & {} \sum _{l=0}^{1} \frac{1}{l!}g^{(l)}(t)(t_{k}-t)^{l} +\int _{t}^{t_{k}} g^{(2)}(\xi ) (t_{k}-\xi )\, {\textrm{d}} \xi , \qquad k\ge 0,~t>0, \nonumber \\ \end{aligned}$$

(A.3)

$$\begin{aligned} g(t_{k})= & {} \sum _{l=0}^{2} \frac{1}{l!}g^{(l)}(t)(t_{k}-t)^{l} +\frac{1}{2}\int _{t}^{t_{k}} g^{(3)}(\xi ) (t_{k}-\xi )^{2}\, {\textrm{d}} \xi , \qquad k\ge 0,~t>0. \nonumber \\ \end{aligned}$$

(A.4)

This implies

$$\begin{aligned} R_{1,1}g(t)= & {} g(t)-\sum _{k=0}^{1} l_{k,1,1}(t)g(t_{k})\nonumber \\= & {} g(t)-\sum _{l=0}^{1} \frac{1}{l!}g^{(l)}(t)\sum _{k=0}^{1} l_{k,1,1}(t)(t_{k}-t)^{l}-\sum _{k=0}^{1} l_{k,1,1}(t)\int _{t}^{t_{k}} g^{(2)}(\xi ) (t_{k}-\xi )\, {\textrm{d}} \xi \nonumber \\= & {} -\sum _{k=0}^{1} l_{k,1,1}(t) (t-t_{k})^{2} \int _{0}^{1} g^{(2)}(t_{k}(1-s)+ts) s \, {\textrm{d}} s, \qquad t>0, \end{aligned}$$

(A.5)

$$\begin{aligned} R_{2,2}g(t)= & {} g(t)-\sum _{k=0}^{2} l_{k,2,2}(t)g(t_{k})\nonumber \\= & {} g(t)-\sum _{l=0}^{1} \frac{1}{l!}g^{(l)}(t)\sum _{k=0}^{2} l_{k,2,2}(t)(t_{k}-t)^{l}-\sum _{k=0}^{2} l_{k,2,2}(t)\int _{t}^{t_{k}} g^{(2)}(\xi ) (t_{k}-\xi )\, {\textrm{d}} \xi \nonumber \\= & {} -\sum _{k=0}^{2} l_{k,2,2}(t) (t-t_{k})^{2} \int _{0}^{1} g^{(2)}(t_{k}(1-s)+ts) s \, {\textrm{d}} s, \qquad t>0, \end{aligned}$$

(A.6)

and

$$\begin{aligned} R_{2,q}g(t)= & {} g(t)-\sum _{k=q-2}^{q} l_{k,2,q}(t)g(t_{k})\nonumber \\= & {} g(t)-\sum _{l=0}^{2} \frac{1}{l!} g^{(l)}(t)\sum _{k=q-2}^{q} l_{k,2,q}(t)(t_{k}-t)^{l}\nonumber \\{} & {} -\frac{1}{2}\sum _{k=q-2}^{q} l_{k,2,q}(t)\int _{t}^{t_{k}} g^{(3)}(\xi ) (t_{k}-\xi )^{2}\, {\textrm{d}} \xi \nonumber \\= & {} \frac{1}{2}\sum _{k=q-2}^{q} l_{k,2,q}(t) (t-t_{k})^{3} \int _{0}^{1} g^{(3)}(t_{k}(1-s)+ts) s^{2} \, {\textrm{d}} s,\nonumber \\{} & {} \quad q\ge 2,~t>0. \end{aligned}$$

(A.7)

This proves (4.6)–(4.8). $\square$