Application of nonlocal elasticity continuum damping models in nonlinear dynamic analysis

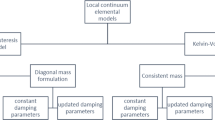
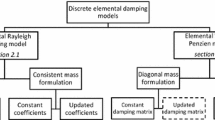
Abstract

In order to have a more generic representation of the damping phenomenon in seismic analysis of structures, in this paper, the already existing nonlocal elasticity continuum damping models have been adapted and extended to the inelastic domain. Adaptations of two nonlocal elasticity based damping models, Russell’s spatial hysteresis model and the extended Sorrentino model, into the nonlinear dynamic analysis is presented. Galerkin based finite element schemes are developed and the numerical implementation of the models are outlined. The performances of the nonlocal elasticity-based damping models are illustrated by studying the nonlinear dynamic responses of a four-story Reinforced Concrete frame designed to the Eurocodes. The incremental dynamic analysis study presented illustrates the fact that the proposed models are devoid of the large spurious damping actions commonly exhibited by the popular classical Rayleigh damping model. It has also been shown that in a nonlinear dynamic analysis, the proposed adaptation of the nonlocal elasticity continuum damping models might be a more realistic alternative to the popular classical Rayleigh damping model. The proposed models may be easily implemented in an existing software framework capable of solving both ordinary and integro-differential equations without adding markedly to the computational effort.

Appendices

Appendix 1: Derivation of damping coefficients for the nonlocal damping models

Only the coefficient matrix for the symmetric upper triangular coefficient matrix is given here, where $c_{ij}$ refers to the ith row and jth column element.

1.1 Russell’s damping coefficients

These coefficients are computed using Eq. (11) for Gaussian kernel function by applying the classical Hermitian cubic shape functions. For details on the variables in the coefficient matrix refer Sect. 3.1.

$$ \begin{aligned} \Re_{\theta } & = \sqrt 2 be^{{ - \left( {\frac{1}{2}\frac{{L^{2} }}{{b^{2} }}} \right)}} L^{2} + erf\left( {\frac{L}{\sqrt 2 b}} \right)\sqrt \pi L^{3} - 6\sqrt 2 bL^{2} - 8\sqrt 2 b^{3} \\ & \quad + 9L\sqrt \pi \left( {erf\left( {\frac{L}{\sqrt 2 b}} \right)} \right)b^{2} + 8\sqrt 2 e^{{\left( { - \frac{1}{2}\frac{{L^{2} }}{{b^{2} }}} \right)}} b^{3} \\ \Re_{\eta } & = 5\sqrt 2 be^{{ - \left( {\frac{1}{2}\frac{{L^{2} }}{{b^{2} }}} \right)}} L^{2} - 20\sqrt 2 bL^{2} - 24\sqrt 2 b^{3} \left( {1 - e^{{ - \left( {\frac{1}{2}\frac{{L^{2} }}{{b^{2} }}} \right)}} } \right) \\ & \quad + 27L\sqrt \pi erf\left( {\frac{1}{2}\frac{L\sqrt 2 }{b}} \right)b^{2} + 4erf\left( {\frac{L}{\sqrt 2 b}} \right)\sqrt \pi L^{3} \\ \Re_{\lambda } & = \sqrt 2 be^{{ - \left( {\frac{1}{2}\frac{{L^{2} }}{{b^{2} }}} \right)}} L^{2} - 16\sqrt 2 bL^{2} - 24\sqrt 2 b^{3} \left( {1 - e^{{ - \left( {\frac{1}{2}\frac{{L^{2} }}{{b^{2} }}} \right)}} } \right) \\ & \quad + 27L\sqrt \pi erf\left( {\frac{1}{2}\frac{L\sqrt 2 }{b}} \right)b^{2} + 2erf\left( {\frac{L}{\sqrt 2 b}} \right)\sqrt \pi L^{3} \\ c_{11} & = \frac{{6b^{2} a}}{{L^{6} \sqrt \pi }}\Re_{\theta } \\ c_{12} & = \frac{{3b^{2} a}}{{L^{5} \sqrt \pi }}\Re_{\theta } \\ c_{13} & = - c_{11} \\ c_{14} & = c_{12} \\ c_{22} & = \frac{{b^{2} a}}{{2L^{4} \sqrt \pi }}\Re_{\eta } \\ c_{23} & = - c_{12} \\ c_{24} & = \frac{{b^{2} a}}{{2L^{4} \sqrt \pi }}\Re_{\lambda } \\ c_{33} & = c_{11} \\ c_{34} & = - c_{14} \\ c_{44} & = c_{22} \\ \end{aligned} $$

1.2 Extended Sorrentino damping coefficients

The internal direct damping coefficient matrix obtained by computing Eq. (27) using classical Hermitian cubic shape functions are given as follows.

$$ \begin{aligned} c_{11} & = - \frac{{\left( {288\left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) - 24\alpha^{3} L_{e}^{3} + \frac{{288\alpha L_{e} }}{{e^{{\alpha L_{e} }} }} + 72\alpha^{2} L_{e}^{2} \left( {\frac{1}{{e^{{\alpha L_{e} }} }} + 1} \right) - 288} \right)}}{{\alpha^{4} L_{e}^{6} }} \\ c_{12} & = \frac{{ - \left( {144\left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) - 12\alpha^{3} L_{e}^{3} + 144\alpha L_{e} \left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) + 36\alpha^{2} L_{e}^{2} \left( {\frac{1}{{e^{{\alpha L_{e} }} }} + 1} \right) - 144} \right)}}{{\alpha^{4} L_{e}^{5} }} \\ c_{13} & = \frac{{\left( {288\left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) - 24\alpha^{3} L_{e}^{3} + 288\alpha L_{e} \left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) + 72\alpha^{2} L_{e}^{2} \left( {\frac{1}{{e^{{\alpha L_{e} }} }} + 1} \right) - 288} \right)}}{{\alpha^{4} L_{e}^{6} }} \\ c_{14} & = - \frac{{\left( {144\left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) - 12\alpha^{3} L_{e}^{3} + 144\alpha L_{e} \left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) + 36\alpha^{2} L_{e}^{2} \left( {\frac{1}{{e^{{\alpha L_{e} }} }} + 1} \right) - 144} \right)}}{{\alpha^{4} L_{e}^{5} }} \\ c_{22} & = - \frac{{\left( {4\left( {2\alpha L_{e} + 3} \right)\left( {\frac{6}{{e^{{\alpha L_{e} }} }}} \right) - \alpha^{2} L_{e}^{2} + 4\alpha L_{e} + 2\alpha L_{e} \left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) - 6} \right)}}{{\alpha^{4} L_{e}^{4} }} \\ c_{23} & = \frac{{\left( {144\left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) - 12\alpha^{3} L_{e}^{3} + 144\alpha L_{e} \left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) + 36\alpha^{2} L_{e}^{2} \left( {\frac{1}{{e^{{\alpha L_{e} }} }} + 1} \right) - 144} \right)}}{{\alpha^{4} L_{e}^{5} }} \\ c_{24} & = - \frac{{\left( {72\left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) - 4\alpha^{3} L_{e}^{3} + 72\alpha L_{e} \left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) + \alpha^{2} L_{e}^{2} \left( {\frac{20}{{e^{{\alpha L_{e} }} }} + 16} \right) - 72} \right)}}{{\alpha^{4} L_{e}^{4} }} \\ c_{33} & = - \frac{{\left( {288\left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) - 24\alpha^{3} L_{e}^{3} + \frac{{288\alpha L_{e} }}{{e^{{\alpha L_{e} }} }} + 72\alpha^{2} L_{e}^{2} \left( {\frac{1}{{e^{{\alpha L_{e} }} }} + 1} \right) - 288} \right)}}{{\alpha^{4} L_{e}^{6} }} \\ c_{34} & = \frac{{\left( {144\left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) - 12\alpha^{3} L_{e}^{3} + 144\alpha L_{e} \left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) + 36\alpha^{2} L_{e}^{2} \left( {\frac{1}{{e^{{\alpha L_{e} }} }} + 1} \right) - 144} \right)}}{{\alpha^{4} L_{e}^{5} }} \\ c_{44} & = - \frac{{\left( {4\left( {2\alpha L_{e} + 3} \right)\left( {\frac{6}{{e^{{\alpha L_{e} }} }}} \right) - \alpha^{2} L_{e}^{2} + 4\alpha L_{e} + 2\alpha L_{e} \left( {\frac{1}{{e^{{\alpha L_{e} }} }}} \right) - 6} \right)}}{{\alpha^{4} L_{e}^{4} }} \\ \end{aligned} $$

Appendix 2: Computation of elemental frequencies using consistent mass matrix (Puthanpurayil et al. 2016)

Elemental frequencies of beam elements are computed assuming a free–free boundary condition. The consistent mass matrix is given as,

$$ {\mathbf{M}} = \frac{{\rho A_{e} l_{e} }}{420}\left[ {\begin{array}{*{20}c} {4l_{e}^{2} } & { - 3l_{e}^{2} } \\ { - 3l_{e}^{2} } & {4l_{e}^{2} } \\ \end{array} } \right] $$

(62)

and the flexibility matrix with plastic hinge spring flexibility $f_{s}$ in series is given as,

$$ {\mathbf{F}} = \left[ {\begin{array}{*{20}c} {\frac{{2l_{e} }}{{6EI_{e} }} + f_{s} } & {\frac{{l_{e} }}{{6EI_{e} }}} \\ {\frac{{l_{e} }}{{6EI_{e} }}} & {\frac{{2l_{e} }}{{6EI_{e} }} + f_{s} } \\ \end{array} } \right] $$

(63)

Now, elemental frequencies can be computed by solving Eq. 64 given below as,

$$ \left[ {\begin{array}{*{20}c} {\frac{{2l_{e} }}{{6EI_{e} }} + f_{s} } & {\frac{{l_{e} }}{{6EI_{e} }}} \\ {\frac{{l_{e} }}{{6EI_{e} }}} & {\frac{{2l_{e} }}{{6EI_{e} }} + f_{s} } \\ \end{array} } \right]\frac{{\rho A_{e} l_{e}^{3} }}{420}\left[ {\begin{array}{*{20}c} 4 & { - 3} \\ { - 3} & 4 \\ \end{array} } \right]\left\{ {\begin{array}{*{20}c} {\varphi_{e,1} } \\ {\varphi_{e,2} } \\ \end{array} } \right\} = \frac{1}{{\omega_{e}^{2} }}\left[ {\begin{array}{*{20}c} 1 & 0 \\ 0 & 1 \\ \end{array} } \right] $$

(64)

Solving Eq. 64 gives the elemental frequencies and elemental mode shapes for the element under consideration.

Appendix 3: Frame details (Puthanpurayil et al. 2016)

The frame details are described in this section for easier readability of the paper and is directly adopted from Puthanpurayil et al. (2016).

3.1 Material property (Dolsek 2010)

Adopted value for the present study is given as below:

$$ \begin{aligned} & {\text{Mean}}\,{\text{concrete}}\,{\text{strength}} = 33\,{\text{MPa}} \\ & {\text{Modulus}}\,{\text{of}}\,{\text{elasticity}} = 3.1 \times 10^{10} \,{\text{N/m}}^{2} \\ \end{aligned} $$

See Fig. 7.

Fig. 7

Four story frame

3.2 Geometric properties (Arede 1997)

Member number	Width of the member (mm)	Depth of the member (mm)
1, 6, 11, 16, 17, 12, 7, 2, 3, 8, 13, 18	450	450
4, 5, 9, 10, 14, 15, 19, 20	300	450

3.3 Nodal mass (Arede 1997)

Floor level	Mass per node (kg)
1st floor	29,800
2nd–4th floor	29,500

3.4 Yield rotations

Yield rotations are computed as described in Puthanpurayil et al. (2016).

Member number	Yield rotation (ith node) (positive/negative) (rad)	Yield rotation (jth node) (positive/negative) (rad)
1, 2, 3	0.04	0.0074
6, 8, 11, 13, 16, 18	0.0064	0.0064
7	0.0054	0.0061
12, 17	0.0061	0.0061
4, 9	0.0093	0.0093
5, 10	0.0062	0.0062
14, 19	0.009	0.009
15, 20	0.006	0.006